1. Which statement is not true? A. Rational numbers are closed under division. B. Odd numbers are closed under multiplication. C. Prime numbers are closed under subtraction. D. Integers are closed under addition
Answers
Answer:
integers are closed under division
Step-by-step explanation:
Integers are not closed under division.Let us consider two integers i and j.
Then need not necessarily be an integer. For example, 2 and 3 are integers but is not an integer. So the first option , namely, integers are closed under division is not true.
On the other hand, the remaining three options given are correct.
Related Questions
A ruler cost x pence a pen costs 10 pence more than the ruler write an expression in terms of x for the cost of the pen
Answers
Answer:
(A ruler) = X (a pen) = Y
y = x+10
Step-by-step explanation:
Answer:
A ruler cost x pence.
Let the cost of the ruler be x pencePen costs 10 pence more than the ruler
Therefore, the pen cost (x+10) pence(x+10) is the right answer.the 9th and 12 terms of an arithmetic progression are 50 and 65 respectively. find the sum of it's first 70 terms.
Answers
bvmnb n m mn
b bn. Step-by-step explanation:
Jackson wrote different patterns for the rule subtract 5 select all the patterns he could have written
Answers
When Jackson wrote different patterns for the rule "subtract 5", the patterns that he could have written include
A. 27, 22, 17, 12, 7
D. 100, 95, 90, 85, 80
What is the expression regarding the pattern?It is important to note that an expression is simply used to show the relationship between the variables that are provided or the data given regarding an information. In this case, it is vital to note that they have at least two terms which have to be related by through an operator.
Some of the mathematical operations that are illustrated in this case include addition, subtraction, etc. In this case, when Jackson wrote different patterns for the rule "subtract 5", the patterns that he could have written include 27, 22, 17, 12, 7 and 100, 95, 90, 85, 80. In this cases, there are difference of 5.
Learn more about expressions on:
https://brainly.com/question/723406
#SPJ1
Complete question
Jackson wrote different patterns for the rule "subtract 5". Select all of the patterns that he could have written.
27, 22, 17, 12, 7
5, 10, 15, 20, 25
55, 50, 35, 30, 25
100, 95, 90, 85, 80
75, 65, 55, 45, 35
A caterpillar crawling in a straight line across a coordinate plane starts at point (-3, -4) and ends at point (25, 38). Counting the start point and end point, how many points with integer coordinates does the caterpillar touch?
Answers
Answer:
15 points
Step-by-step explanation:
The start and end point of the caterpillar path are;
Start point = (-3, -4)
End point = (25, 38)
The slope of the caterpillar's path, 'm', given the 'x', and y-coordinates of two points is given by the following formula;
\(Slope, \, m =\dfrac{y_{2}-y_{1}}{x_{2}-x_{1}}\)
Therefore, m = (38 - (-4))/(25 - (-3)) = 1.5
The equation of the caterpillar's path in point and slope form is given as follows;
y - (-4) = 1.5 × (x - (-3))
y + 4 = 1.5 × (x + 3)
y + 4 = 1.5·x + 4.5
y = 1.5·x + 4.5 - 4 = 1.5·x + 0.5
y = 1.5·x + 0.5
Given that the 0.5 is added to the product of 'x' and 1.5, the points that have both integer points for 'x', and 'y' are the points that have odd number values of 'x', as follows;
(-3, -4), (-1, -1), (1, 2), (3, 5), (5, 8), (7, 11), (9, 14), (11, 17), (13, 20), (15, 23), (17, 26), (19, 29), (21, 32), (23, 35), (25, 38)
Therefore, we have 15 points counting the start and end points with integer coordinates
A sequence can be generated by using an= 3an-1, where a1 = 10 and n is a whole number greater than 1.
What are the first 3 terms in the sequence?
A. 3, 13, 23
B. 10, 30, 90
C. 10, 13, 16
D. 3, 30, 300

Answers
Answer:
B
Step-by-step explanation:
using the recursive rule \(a_{n}\) = 3\(a_{n-1}\) and a₁ = 10, then
a₁ = 10
a₂ = 3a₁ = 3 × 10 = 30
a₃ = 3a₂ = 3 × 30 = 90
the first 3 terms are 10 , 30 , 90
TrianglesABC and DEF are
Similar
Not similar

Answers
Answer:
Similar
Step-by-step explanation:
We can say that the triangles are similar by looking at the angle measurements
Angle B = 35
Angle F = 55
Both angles A and D = 90 because these are right triangles
We can say the measure of angle E = 35 because the sum of angles in a triangle equal to 180
Same rule applies to angle C
How many people will get candy?

Answers
Answer:
22 people
Step-by-step explanation:
Answer:
22
Step-by-step explanation:
First:
Convert any mixed numbers to fractions.
Then your initial equation becomes:
11/4
Applying the fractions formula for division,
11x8
4x1
Simplifying 88/4, the answer is
22Which rectangle has side lengths of 5 units and 4 units?
A(3, 3), B(3, 6), C(8, 6), D(8, 3)
A(3, 3), B(3, 7), C(8, 7), D(8, 3)
A(3, 3), B(3, 7), C(7, 7), D(7, 3)
A(3, 3), B(3, 8), C(8, 8), D(8, 3)
Answers
The rectangle that will side lengths of 5units and 4 units will have coordinate of A(3, 3), B(3, 7), C(8, 7), D(8, 3) ( option B)
What is distance between two points?Distance between two points is the length of the line segment that connects the two points in a plane. The formula to find the distance between the two points is usually given by d=√((x2 – x1)² + (y2 – y1)²).
For a rectangle, the opposite sides are equal this means AB = C D and CB = AD
To verify the answer, The distance between AB = √((3– 3)² + (7– 3)²).
line AB = √0+16= 4units
line AD = √((8– 3)² + (3– 3)²).
line AD = √25+0
line AD = 5 units
therefore the sides of the rectangle are 5units and 4 units.
learn more about distance between two points from
https://brainly.com/question/7243416
#SPJ1
On the SAT exam a total of 25 minutes is allotted for students to answer 20 math questions without the use of a calculator. A guidance counselor would like to know if the students in his school are prepared to complete this portion of the exam in the timeframe allotted. To investigate, the counselor selects a random sample of 35 students and administers this portion of the test. The students are instructed to turn in their test as soon as they have completed the questions. The mean amount of time taken by the students is 23.5 minutes with a standard deviation of 4.8 minutes. The counselor would like to know if the data provide convincing evidence that the true mean amount of time needed for all students of this school to complete this portion of the test is less than 25 minutes and therefore tests the hypotheses H0: μ = 25 versus Ha: μ < 25, where μ = the true mean amount of time needed by students at this school to complete this portion of the exam. The conditions for inference are met. What are the appropriate test statistic and P-value?
Answers
The P-value is between 0.025 and 0.05. and t = -1.85
On the SAT exam a total of 25 minutes is allotted for students to answer 20 math questions without the use of a calculator.
Therefore tests the hypotheses:
\(H_0\) : μ = 25 versus Ha: μ < 25,
where μ = the true mean amount of time needed by students at this school to complete this portion of the exam.
The alternative hypothesis is:
\(H_1:\mu < 25\)
The test statistic is given by:
\(t=\frac{x-\mu}{\frac{s}{\sqrt{n} } }\)
The parameters are:
'x' is the sample mean. \(\mu\) is the value tested at the null hypothesis.s is the standard deviation of the sample.n is the sample size.the values of the parameters are:
x = 23.5 , \(\mu=25\) , s = 4.8, n = 35
Plug all the values in above formula of t- statistic is:
\(t = \frac{23.5-25}{\frac{4.8}{\sqrt{35} } }\)
t = -1.85
Using a t-distribution , with a left-tailed test, as we are testing if the mean is less than a value and 35 - 1 = 34 df, the p-value is of 0.0365.
t = –1.85; the P-value is between 0.025 and 0.05.
Learn more about t-distribution at:
https://brainly.com/question/13574945
#SPJ1
anyone can solve this?
\( \sqrt[4] {a}^{3 \:} to \: power\)
Answers
The value of the expression \(\sqrt[4]{a}^3\) when evaluated is \(a^\frac34\)
How to evaluate the expressionFrom the question, we have the following parameters that can be used in our computation:
\(\sqrt[4]{a}^3\)
Consider an expression expressed as xⁿ
The expression can be expanded as
xⁿ = x * x * x .... in n places
Using the above as a guide, we have the following:
\(\sqrt[4]{a}^3 = \sqrt[4]{a} * \sqrt[4]{a} * \sqrt[4]{a}\)
Apply the exponent rule of indices
\(\sqrt[4]{a}^3 = a^\frac14 * a^\frac14 * a^\frac14\)
Apply the power rule of indices
\(\sqrt[4]{a}^3 = a^\frac34\)
Hence, the expression \(\sqrt[4]{a}^3\) when evaluated is \(a^\frac34\)
Read more about expression at
https://brainly.com/question/32302948
#SPJ1
Noah has a coin jar containing d dimes and a quarters worth a total of $5.00. Select all the equations that represent this situation

Answers
Answer:
C AND D
Step-by-step explanation:
JUST PRESS C AND D AND ITS RIGHT LOL
The equation that represents the situation will be 0.10d + 0.25 = 5 and 10d + 25q = 500. Then the correct options are C and D.
How to convert the sentence into an expression?The act of converting a specified statement into an expression or equation is known as a sentence-to-equation transformation.
A relationship between two or more parameters that, when shown on a graph, produces a linear model. The degree of the variable will be one.
Noah has a coin jar containing dimes (d) and quarters (q) worth a total of $5.00.
The equation that represents the situation will be given as,
0.10d + 0.25 = 5
10d + 25q = 500
The equation that represents the situation will be 0.10d + 0.25 = 5 and 10d + 25q = 500. Then the correct options are C and D.
More about the conversion of sentence into equation link is given below.
https://brainly.com/question/10606712
#SPJ5
Two times the sum of a number and 8 is negative 48 what is the number?
Answers
Answer:
-32
Step-by-step explanation:
Let the number be x.
\(2(x+8)=-48 \\ \\ x+8=-24 \\ \\ x=-32\)
Find the center and radius of the circle represented by the equation below.

Answers
Answer:
centre = (5, - 6 ) , radius = 7
Step-by-step explanation:
the equation of a circle in standard form is
(x - h)² + (y - k)² = r²
where (h, k ) are the coordinates of the centre and r is the radius
given
x² + y² - 10x + 12y + 12 = 0 ( subtract 12 from both sides )
x² + y² - 10x + 12y = - 12 ( collect terms in x/ y )
x² - 10x + y² + 12y = - 12
using the method of completing the square
add ( half the coefficient of the x/ y terms )² to both sides
x² + 2(- 5)x + 25 + y² + 2(6)y + 36 = - 12 + 25 + 36
(x - 5)² + (y + 6)² = 49 = 7² ← in standard form
with centre (5, - 6 ) and radius = 7
Answer:
Center = (5, -6)
Radius = 7
Step-by-step explanation:
To find the center and the radius of the circle represented by the given equation, rewrite the equation in standard form by completing the square.
To complete the square, begin by moving the constant to the right side of the equation and collecting like terms on the left side of the equation:
\(x^2-10x+y^2+12y=-12\)
Add the square of half the coefficient of the term in x and the term in y to both sides of the equation:
\(x^2-10x+\left(\dfrac{-10}{2}\right)^2+y^2+12y+\left(\dfrac{12}{2}\right)^2=-12+\left(\dfrac{-10}{2}\right)^2+\left(\dfrac{12}{2}\right)^2\)
Simplify:
\(x^2-10x+(-5)^2+y^2+12y+(6)^2=-12+(-5)^2+(6)^2\)
\(x^2-10x+25+y^2+12y+36=-12+25+36\)
\(x^2-10x+25+y^2+12y+36=49\)
Factor the perfect square trinomials on the left side:
\((x-5)^2+(y+6)^2=49\)
The standard equation of a circle is:
\(\boxed{(x-h)^2+(y-k)^2=r^2}\)
where:
(h, k) is the center.r is the radius.Comparing this with the rewritten given equation, we get
\(h = 5\)\(k = -6\)\(r^2 = 49 \implies r=7\)Therefore, the center of the circle is (5, -6) and its radius is r = 7.
Value of X. Please give step by step so I understand

Answers
Answer:
The value of x is 15.
Step-by-step explanation:
Since the base is 40 inches long, and the two triangles are congruent - both are right triangles and have sides x and their bases equal - you know that each base of a triangle is 20 inches long. Using the Pythagorean Theorem, you can get that \(x^2+20^2=25^2\). Solve for x by isolating the variable on one side: \(x^{2} =25^{2} - 20^{2}\) ⇒ \(x^{2} = 625 - 400\) ⇒ \(x^{2} = 225\) ⇒ \(x^{2} =225\) ⇒ \(x=\sqrt{225}\) ⇒ \(x=15\) inches.
Find the product of 5√10 and 4√6 in simplest form. Also, determine whether the
result is rational or irrational and explain your answer.

Answers
Answer:
Step-by-step explanation:
5√10×4√6=(5×4)√(10×6)=20√60=20√(4×15)=(20×2)√15=40√15≈77.4596669241...
The result is irrational because it can not be written as the ratio of two integers and its decimal expansion 77.4596669241
Convert the linear equation 8x-2y=12 into function form.

Answers
Answer:
C
Step-by-step explanation:
get the y alone on one side: 2y=8x-12
divide by two on both sides: y=4x-6
Answer:
y=4x-6
Step-by-step explanation:
Your goal is to isolate the y variable to get it back into function form.
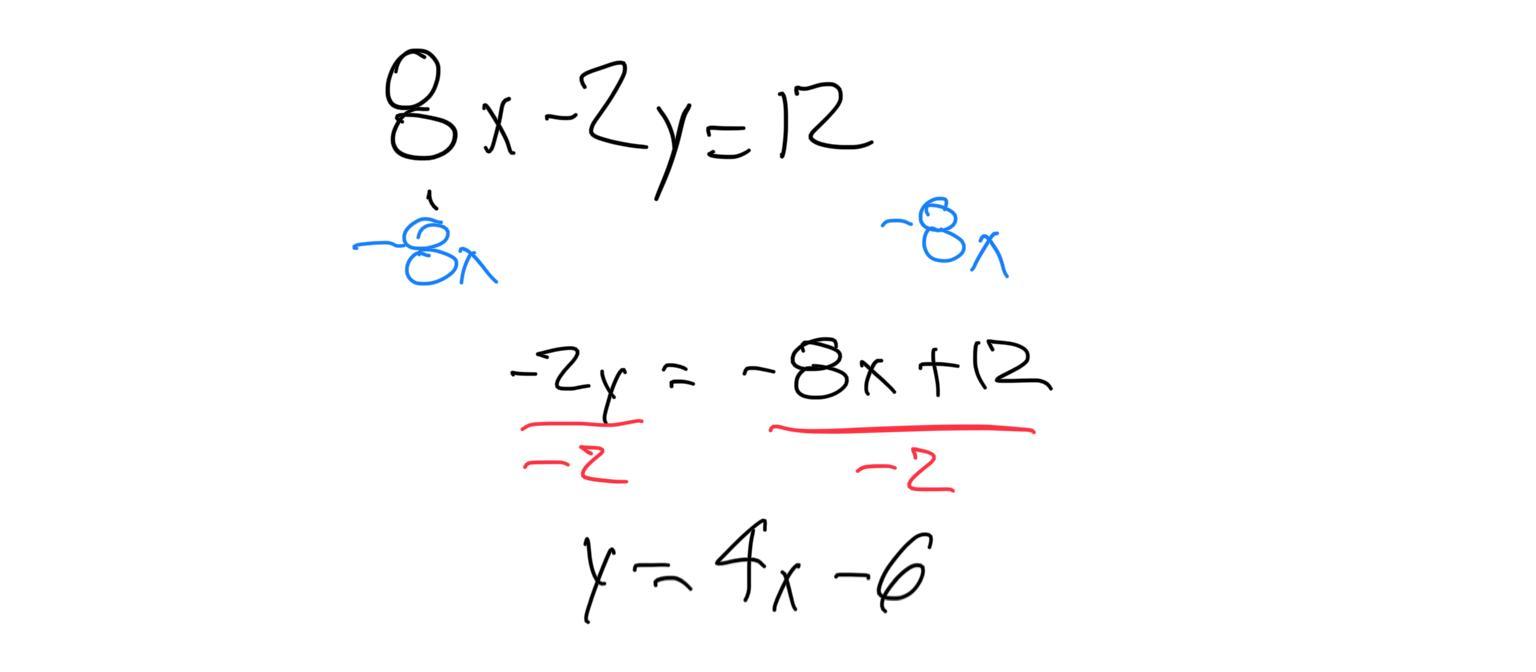
The original price of a desk was $80. And its price was marked
down to $70 during the holiday. What was the percentage of
discount for the desk during the holiday?
Answers
Answer:
Required Answer:-Amount of discount =80-70=10$
percentage:-
\({:}\longrightarrow\)\(\sf {\dfrac{10}{80}}\times 100 \)
\({:}\longrightarrow\)\(\sf {\dfrac {10}{4}}5 \)
\({:}\longrightarrow\)\(\sf {\dfrac {50}{4}}\)
\({:}\longrightarrow\)\(\sf 12.5\% \)
Answer: the answer is 12.5
Step-by-step explanation:
There are six pizzas there are 36 players on the team. If each member has one slice and each pizza has eight slices how much pizza in fraction form will be left over.
Answers
There will be 12 slices of pizza left over, which can be expressed as 3/2 or 1 1/2 slices in fraction form.
To find out how much pizza will be left over, we need to calculate the total number of slices available and subtract the number of slices consumed by the players.
Given that there are six pizzas and each pizza has eight slices, the total number of slices available is 6 pizzas * 8 slices/pizza = 48 slices.
Since there are 36 players on the team, and each player has one slice, the total number of slices consumed is 36 slices.
To determine the remaining slices, we subtract the consumed slices from the total available slices: 48 slices - 36 slices = 12 slices.
Therefore, there will be 12 slices of pizza left over.
To express this as a fraction, we can write it as 12/1, which simplifies to 12. This means there will be 12 whole slices of pizza left over.
If you would like to express it as a fraction in its simplest form, you can write it as 12/8. By dividing both the numerator and denominator by their greatest common divisor, which is 4, we get 3/2. So, in fraction form, there will be 3/2 or 1 1/2 slices of pizza left over.
for more questions on fraction
https://brainly.com/question/78672
#SPJ8
Today Olivia is carrying her history textbook and her lunch in her backpack. If the history textbook weighs 1/2 of a pound and her lunch weighs 2/3 of a pound, how much weight is in Olivia's backpack?
Answers
Weight is in Olivia's backpack is 5/6 pound.
As in the given problem olivia's history book weights 1/2 of a pound and
her lunch weighs 2/3 of a pound.
As both the units are same,
Thus total weight must be summation of both history book and her lunch box.
total weight is =1/2 +2/3 pound
= (3+2)/6
that is equal to 5/6 pound.
Learn more about summation here:
https://brainly.com/question/21021524
#SPJ4
x+y=9.35 5x-y=8.75
how to solve this math problem
Answers
Answer:
x=3.01667
Step-by-step explanation:
\(x+y=9.35\\5x-y=8.75\\By\ adding\ the\ system\ of\ equations:\\(x+y)+(5x-y)=9.35+8.75\\6x=18.1\\x=3.01667\)
HELP PLEASEEEEEEEE !!!

Answers
Explanation:
ABD is a right triangle (Angle ADB is a right angle)
Using the Pythagoras Theorem,
AB^2 = AD^2 + BD^2
AB^2 = 9^2 + 12^2
AB^2 = 81 + 144
AB^2 = 225
AB = 15
In triangle ABD,
sin(Angle A) = BD/AB
sin(Angle A) = 12/15
Angle A = sin^(-1)[12/15]
Angle A ≈ 53.13 degrees
In triangle ABC,
cos(Angle A) = AB/AC
cos(53.13) = 15/AC
AC = 15/cos(53.13)
AC ≈ 25
DC = AC - AD = 25 - 9 = 16
please help!
a. 8x10^3
b. 8x10^12
c. 8
d. 8x10^9

Answers
Mr.Davis drives 496 miles in eight hours. At this rate, how many miles does he drive in six hours?
Is it 372?
Answers
Answer:
Step-by-step explanation:
496m=8h
Figure for 1 hour.
496/8=62
Now do
62x6=372m
Yes, you are correct.
A geometric series has the third term 36 , and sixth term 972 . Find the first term of the series
Answers
Answer:
the first term is 4
Step-by-step explanation:
Given
G(3) = 36
G(6) = 972
Solution:
General formula for geometric series
G(x) = AB^x
From given data: G(6)/G(3) = 972/36 = 27
From formula: G(6)/G(3) = AB^6/(AB^3) = B^3
Therefore
B^3 = 27
B=3
Hence
G(1) = AB^1 = AB^3/B^2=36/3^2=36/9=4
Ans: the first term is 4
Answer:
a₁ = 4
Step-by-step explanation:
The n th term of a geometric series is
\(a_{n}\) = a₁ \(r^{n-1}\)
where a₁ is the first term and r the common ratio
Given a₃ = 36 and a₆ = 972 , then
ar² = 36 → (1)
a\(r^{5}\) = 972 → (2)
Divide (2) by (1)
\(\frac{ar^{5} }{ar^{2} }\) = \(\frac{972}{36}\) , that is
r³ = 27 ( take the cube root of both sides )
r = \(\sqrt[3]{27}\) = 3
Substitute r = 3 into (1)
9a = 36 ( divide both sides by 9 )
a = 4
The first term is 4
If m∠J = 44°, what is m∠I?
Answers
Answer:
6666
Step-by-step explanation:
Nvm I found the answer
Answers
Find the value of x in the figure below if MP is parallel to RA.

Answers
Answer: 4.5 units
Step-by-step explanation:
Answer:
4.5 units
Step-by-step explanation:
You would use this equation to find the value of x: \(\frac{18}{x}\) = \(\frac{20}{5}\)
Which two relationships describes angles 1 and 2? Choose from (adjacent angles, complementary angles, supplementary angles, or vertical angles) relationship 1:
Relationship 2:

Answers
Hence ,the sum of ∠1 and ∠2 is 90 degrees this is a relationship between ∠1 and ∠2.
What is the angle?In Euclidean geometry, an angle is the figure formed by two rays, called the sides of the angle, sharing a common endpoint, called the vertex of the angle. Angles formed by two rays lie in the plane that contains the rays. Angles are also formed by the intersection of two planes. These are called dihedral angles.
What is the adjacent angles, complementary angles, supplementary angles, or vertical angles?complementary angles are two angles that add up to 90 degrees, supplementary angles are two angles that add up to 180 degrees,
vertical angles are opposite angles at an intersection of two straight lines, and adjacent angles are two angles that are next to each other.
According to figure , the sum of ∠1 and ∠2 is 90 degrees and If the sum of the two angles is equal to the measurement of a right angle .
So, the pair of angles ∠1 and ∠2 is said to be complementary angles.
Learn more about angles here:
https://brainly.com/question/25716982
#SPJ1.
Rearrange these numbers from smallest to biggest 1/2, -8, 0, -1/2
Answers
Solution:
To arrange the following numbers in "smallest to biggest" form, look for the smallest number in the group. To see the smallest number in the group, look for a negative number. If there is more than one negative number, the number which is farthest from zero is the smallest. If there are no negative numbers, the closest to 0 will be the smallest number.
In this case, -8 is the smallest number because it is in the negatives and is farthest from 0.The number which is bigger than -8 is -1/2 because it is in the negatives, but it is not farther from 0 than -8.The number which is bigger than -1/2 is 0 because it is neither in the positives nor in the negatives.The biggest number in the group is 1/2 because it is in the positives.We can conclude that the rearranged from (smallest to biggest) is:
-8, -1/2, 0, 1/2Hoped this helped!
What is (x³-8x² + 6x +41) ÷ (x-4)
Answers
Step 1: Write the dividend and divisor:
\(\sf\:\frac{{x^3 - 8x^2 + 6x + 41}}{{x - 4}} \\ \)
Step 2: Divide the first term of the dividend by the first term of the divisor:
\(\sf\:\frac{{x^3}}{{x}} = x^2 \\ \)
Step 3: Multiply the divisor (x - 4) by the result (x^2):
\(\sf\:(x - 4) \cdot (x^2) = x^3 - 4x^2 \\ \)
Step 4: Subtract the result from the original dividend:
\(\sf\:(x^3 - 8x^2 + 6x + 41) - (x^3 - 4x^2) = -4x^2 + 6x + 41 \\ \)
Step 5: Bring down the next term from the dividend:
\(\sf\:\frac{{-4x^2 + 6x + 41}}{{x - 4}} \\ \)
Step 6: Repeat steps 2-5 with the new dividend:
\(\sf\:\frac{{-4x^2}}{{x}} = -4x \\ \)
\(\sf\:(x - 4) \cdot (-4x) = -4x^2 + 16x \\ \)
\(\sf\:(-4x^2 + 6x + 41) - (-4x^2 + 16x) = -10x + 41 \\ \)
Step 7: Bring down the next term from the dividend:
\(\sf\:\frac{{-10x + 41}}{{x - 4}} \\ \)
Step 8: Repeat steps 2-5 with the new dividend:
\(\sf\:\frac{{-10x}}{{x}} = -10 \\ \)
\(\sf\:(x - 4) \cdot (-10) = -10x + 40 \\ \)
\(\sf\:(-10x + 41) - (-10x + 40) = 1 \\ \)
Step 9: There are no more terms to bring down, so the division is complete.
Step 10: Write the final result:
The quotient is \(\sf\:x^2 - 4x - 10\\\) and the remainder is 1.
Therefore, the division of \(\sf\:(x^3 - 8x^2 + 6x + 41) by (x - 4) \\\) is:
\(\sf\:(x^3 - 8x^2 + 6x + 41) ÷ (x - 4) \\ \) \(\sf\:= x^2 - 4x - 10 + \frac{{1}}{{x - 4}} \\ \)