100 percent = Sue's price. 60 percent markup. Retail price is 2 dollars.
Original cost = StartFraction New cost over percent EndFraction. Original cost = StartFraction 2.00 over 1.60 EndFraction. Original cost = StartFraction 2.00 over 1.60 EndFraction times StartFraction 10 over 10 EndFraction
Sue’s Corner Market has a markup of 60% on bottled water. If the market sells a bottle of water for $2, find the original amount that Sue pays.
Sue pays $ for a bottle of water.
Answers
Answer:
1.25
Step-by-step explanation:
did it on edge 2020
Answer:
1.25 i on edge and i got it right
Step-by-step explanation:
Related Questions
help me pliz ill give u full stars i need help quick fast pliz

Answers
Answer:
[-5, 5]
Step-by-step explanation:
what would be the coefficient of determination if the total sum of squares (sst) is 225 and the sum of squares due to error (sse) is 57?
Answers
The coefficient of determination in this scenario is 0.747, which means that approximately 74.7% of the variation in the dependent variable is explained by the independent variable(s). The remaining 25.3% is unexplained and may be due to other factors or errors in the model.
The coefficient of determination, also known as R-squared, is a statistical measure that represents the proportion of the variation in the dependent variable that is explained by the independent variable(s).
It is calculated as 1 - (SSE/SST).
Given that the SST is 225 and SSE is 57, we can calculate the coefficient of determination as follows:
R-squared = 1 - (SSE/SST)
R-squared = 1 - (57/225)
R-squared = 0.747
Therefore, the coefficient of determination in this scenario is 0.747, which means that approximately 74.7% of the variation in the dependent variable is explained by the independent variable(s).
To know more about coefficient of determination refer here:
https://brainly.com/question/31891074
#SPJ11
Find the derivative of the following function:
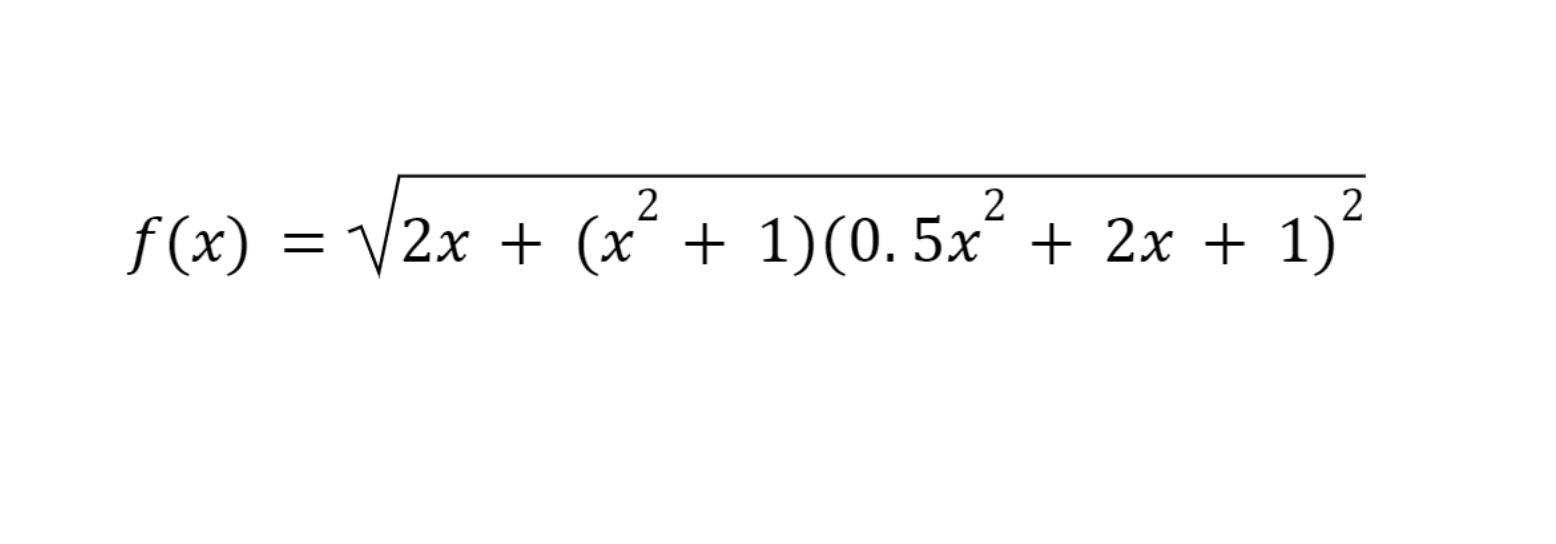
Answers
The derivative of the function \(f(x) = \sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2}\) will be given below.
\(f'(x) = \dfrac{1 + (0.5x^2 + 2x + 1)(1.5x^3 + 4x^2 + 2x + 2)}{\sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2}}\)
What is differentiation?The rate of change of a function with respect to the variable is called differentiation. It can be increasing or decrease.
The equation of the function will be given as,
\(f(x) = \sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2}\)
The derivative of the function will be given as,
\(f'(x) = \dfrac{d}{dx} \ \sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2} \\\\\\f'(x) = \dfrac{2 + 2(x^2 + 1)(0.5x^2 + 2x + 1) \cdot (x + 2) + (0.5x^2 + 2x + 1)^2 \cdot 2x}{2\sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2} }\\\\\\f'(x) = \dfrac{2 + (0.5x^2 + 2x + 1)[2(x^2 + 1)(x + 2) + (0.5x^2 + 2x + 1) \cdot 2x]}{2\sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2} }\\\)
Solve the equation further, then we have
\(f'(x) = \dfrac{1 + (0.5x^2 + 2x + 1)[(x^2 + 1)(x + 2) + (0.5x^2 + 2x + 1) \cdot x}{\sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2}}\\\\\\f'(x) = \dfrac{1 + (0.5x^2 + 2x + 1)(x^3 + 2x^2 + x + 2 + 0.5x^3 + 2x^2 + x)}{\sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2}}\\\\\\f'(x) = \dfrac{1 + (0.5x^2 + 2x + 1)(1.5x^3 + 4x^2 + 2x + 2)}{\sqrt{2x + (x^2 + 1) (0.5x^2 + 2x + 1)^2}}\)
More about the differentiation link is given below.
https://brainly.com/question/24062595
#SPJ1
What is the domain of the function shown on the graph?

Answers
Answer:
Step-by-step explanation:
Looking at the arrows on the graph, it appears that as the graph keep growing UP unbounded, it also keeps growing to the left unbounded (to negative infinity, to be exact). Looking to the right, it appears that as the graph decreases unbounded (the y values keep getting smaller), the graph keeps growing in the x direct to positive infinity. So the domain is
- ∞ < x < ∞
6sin^2 (x) + 6sin (x) + 1 = 0
solve and show steps for the graph ( i already have the graph )
Answers
To solve the equation \(6sin^2(x)\) + 6sin(x) + 1 = 0, we can use algebraic methods and the unit circle to determine the values of x that satisfy the equation.
1. Start by rearranging the equation to a quadratic form: \(6sin^2(x)\) + 6sin(x) + 1 = 0.
2. Notice that the equation resembles a quadratic equation in terms of sin(x). Let's substitute sin(x) with a variable, such as u: \(6u^2\) + 6u + 1 = 0.
3. Solve this quadratic equation for u. You can use the quadratic formula or factorization methods to find the values of u. The solutions are u = (-3 ± √3) / 6.
4. Since sin(x) = u, substitute back the values of u into sin(x) to obtain the values for sin(x): sin(x) = (-3 ± √3) / 6.
5. To find the values of x, we can use the inverse sine function. Take the inverse sine of both sides: x = arcsin[(-3 ± √3) / 6].
6. The arcsin function has a range of [-π/2, π/2], so the values of x lie within that range. Use a calculator to find the approximate values of x based on the values obtained in step 5.
7. Plot the obtained x-values on the graph to show the solutions of the equation \(6sin^2(x)\) + 6sin(x) + 1 = 0. The graph will illustrate the points where the curve intersects the x-axis.
For more such questions on algebraic, click on:
https://brainly.com/question/4344214
#SPJ8
A rectangular prism-shaped display case is 30 1/2 inches wide, 10 1/2 inches long, and 32 1/4 inches tall.
What is the volume of the display case in cubic inches?
Responses
73 1/4 in³
73 and 1 fourth, in³
320 1/4 in³
320 and 1 fourth, in³
10,328 1/16 in³
10328 and 1 sixteenth, in³
28,372 5/8 in
Answers
The volume of the display case is 2161 1/8 cubic inches.To find the volume of a rectangular prism-shaped display case, we multiply its length, width, and height.
Given that the display case is 30 1/2 inches wide, 10 1/2 inches long, and 32 1/4 inches tall, we can calculate the volume as follows:
Volume = Length * Width * Height
Using the given measurements:
Volume = 10 1/2 inches * 30 1/2 inches * 32 1/4 inches
To simplify the calculation, we can convert the mixed numbers to improper fractions:
Volume = (21/2) inches * (61/2) inches * (129/4) inches
Next, we multiply the fractions:
Volume = (21/2) * (61/2) * (129/4) = 17289/8
The resulting fraction, 17289/8, is an improper fraction. To convert it back to mixed number form:
Volume = 2161 1/8 in³.
For such more questions on Rectangular prism-shaped:
https://brainly.com/question/31379597
#SPJ11
Marsha is driving 640 total miles on a trip. She has already driven 2/5 of the distance. How far has Marsha driven?
Answers
Answer:
256 miles
Step-by-step explanation:
Divide 640 into 5 = 128
128 is 1/5 of the distance
128 x 2 = because she has driven 2/5 of the distance already.
128x2= 256 miles
:)
Write an algebraic expression that models the word phrase "the product of 8 and the sum of a number X and 3"
a. 8+3x
b. 3x+8
c. 8x+3
d. 8(x+3)
Answers
The algebraic expression that models the word phrase "the product of 8 and the sum of a number X and 3" is 8(x+3), option d.
Let's break down the phrase into its components and translate it into an algebraic expression:
1. "The product of 8": This means we need to multiply 8 with something.
2. "The sum of a number X and 3": This means we need to add X and 3 together.
To represent the product of 8 and the sum of X and 3 in an algebraic expression, we multiply 8 by the sum of X and 3. This is why the expression is 8(x+3).
Let's consider an example to further illustrate this. If X is equal to 2, we can substitute it into the expression:
8(2+3)
Simplifying the expression within the parentheses:
8(5)
Multiplying 8 by 5:
40
So, if X is 2, the value of the expression 8(x+3) is 40.
Therefore, the correct algebraic expression that models the word phrase "the product of 8 and the sum of a number X and 3" is option d, 8(x+3).
To know more about algebraic expression visit:
https://brainly.com/question/953809
#SPJ11
Which statement about the polygons is true?

Answers
Based on the given polygon, the true statement on the polygons is that Polygon A is similar to Polygon B because Polygon B is a rotation of Polygon A.
How are the polygons related?Polygon A is related to Polygon B because to create Polygon B, Polygon A was dilated.
When a polygon is dilated, it means that it is either made to be larger in size, or smaller in size. In this case, Polygon A was made to be larger in size to create polygon B.
Find out more on dilations at https://brainly.com/question/3457976
#SPJ1
A rectangular portrait has an area of 40 square feet it's perimeter is 26 feet what are the dimensions of the portrait?
Answers
Answer:
Step-by-step explanation:
Portrait is in the shape of a rectangle.
And the area of a rectangle = Length × Width
Let the length of the portrait = l
And width of the portrait = w
Area of the portrait = lw
lw = 40
l = \(\frac{40}{w}\)
Perimeter of a rectangle = 2(Length + width)
Perimeter of the portrait = 2(l + w)
26 = 2(l + w)
l + w = 13 ----------(2)
By substituting the value of 'l' in equation (1) from equation (2)
\(\frac{40}{w}+w=13\)
40 + w² = 13w
w² - 13w + 40 = 0
w² - 8w - 5w + 40 = 0
w(w - 8) - 5(w - 8) = 0
(w - 5)(w - 8) = 0
w = 5, 8 feet
From equation (2)
l = 8, 5 feet
Therefore, dimensions of the portrait are (5 feet by 8 feet) Or (8 feet by 5 feet).
a researcher has participants record how full they feel on a scale from 1 to 7, 1 being not full at all and 7 being very full. what is the type of measure for the dependent variable in this example?
Answers
Type of measure for the dependent variable in this example is self report measure.
What is variable?
A variable in arithmetic is outlined because the graphic symbol that expresses a numerical worth or variety. In algebraical equations, a variable is employed to represent Associate in Nursing unknown amount.
These variables is any alphabets from a to z. most typically, ‘a’,’b’,’c’, ‘x’,’y’ and ‘z’ ar used as variables in equations.
Making pure mathematics calculations with variables like they were categorical numbers permits one to require care of scope of problems during a solitary calculation.
Main body:
As the participants themselves tell how much are they full,so Type of measure for the dependent variable in this example is self report measure.
Hence self report measure is the type .
To know more about variable , visit:
https://brainly.com/question/27894163
#SPJ4
HELPPPPPPPPPP :))))))))))

Answers
Answer:
C
Hope that helps!
Step-by-step explanation:
Find m < E (Image Below)

Answers
Answer:
uiuiuiui86767
Step-by-step explanation:
your answer is e330408384
A 40 gram sample of a substance that's used for drug research has a k-value of 0.1472. N = Noe -kt No = initial mass (at time t = 0) N = mass at time t k = a positive constant that depends on the substance itself and on the units used to measure time t = time, in days
Answers
The image is not downloaded, please send again
are you there?
Pls help me on this if u you don’t know or think you know don’t answer

Answers
Answer:
27:3
Step-by-step explanation:
multiply the 9 by 3 to get the first part
Explanation
To get that value, we multiply every number in the ratio 9:1 by 3
9*3 = 27
1*3 = 3
Therefore, the ratio 9:1 is equivalent to 27:3
) is it possible that ""the sum of two lower triangular matrices be non-lower triangular matrix"" ? explain.
Answers
Yes, it is possible for the sum of two lower triangular matrices to be a non-lower triangular matrix.
To see why, consider the following example:
Suppose we have two lower triangular matrices A and B, where:
A =
[1 0 0]
[2 3 0]
[4 5 6]
B =
[1 0 0]
[1 1 0]
[1 1 1]
The sum of A and B is:
A + B =
[2 0 0]
[3 4 0]
[5 6 7]
This matrix is not lower triangular, as it has non-zero entries above the main diagonal.
Therefore, the sum of two lower triangular matrices can be a non-lower triangular matrix if their corresponding entries above the main diagonal do not cancel out.
To know more about triangular matrix , refer here :
https://brainly.com/question/13385357#
#SPJ11
A teacher recorded unit 4 test scores from her class.
58, 64, 66, 70, 71, 75, 77, 80,
84, 85, 87, 90, 93, 95, 96.
Find the percentile rank of a score of 71 on this street -
Victor is in the 60th percentile, what was his score?
Answers
The percentile score of 71 on test score is 27 and the score of victor is 71.
What is Statistics?Statistics is the science concerned with developing and studying methods for collecting, analyzing, interpreting and presenting empirical data.
Given, the teacher recorded unit 4 test scores from class.
The scores are: 5,4,66,70,71,75,77,80,84,85,87,90,93,95 and 96.
A percentile is a value on a scale of one hundred that indicates the percent of a distribution that is equal to or below it.
The percentile rank of a score of 71 on the test is find by formula
\(R_{100} =\frac{100(N < )+\frac{1}{2} }{N}\)
=100×4+1/2(1)/15
=400+0.5/15
=400.5/15
=26.7=27th
Now we need to find the score of victor who has 60th percentile.
60=Score/15
score=71
Hence, the percentile score of 71 on this test score is 27 and the score of victor is 71.
To learn more on Statistics click:
brainly.com/question/29342780
#SPJ1
Find the cube roots of i. Round all numbers to 3 decimal places. Enter 0s or 1s in the appropriate boxes to answer 0 + i, 1+0i, etc.
Answers
The cube roots of i are:
0.866 + i(0.5)-0.866 + i(0.5)0 - iGiven:
cube root of i
i can be written as cosπ/2 + i sinπ/2
i = cos π/2 + i sinπ/2
general form:
i = cos (2kπ + π/2) + isin(2kπ + π/2) for k=0,1,2
= cos (4kπ+π/2) + i sin(4kπ+π/2)
= cos (4k+1)π/6 + i sin(4k+1) π/6
k = 0: i¹/³ = cos π/6 + i sin π/6
= √3/2 + i 1/2
K = 1, i¹/³ = cos(5π/6) + i sin(5π/6)
= cos(π - π/6) + i sin (π-π/6)
= - cos (π/6) + isin(π/6)
= -√3/2 + i 1/2
k = 2, i¹/³ cos(3π/6) + i sin(3π/6)
= cos (3π/2) + i sin(3π/2)
= cos(π+π/2) + i sin(π+π/2)
= -cos(π/2) - i sin(π/2)
= 0 - i
= -i
Hence we get the required roots.
Learn more about Cube root here:
brainly.com/question/25666481
#SPJ4
we draw a card from an ordinary deck of 52 cards. we define e as the event that the card is a queen and f as the event that the card is a heart. are e and f independent?
Answers
The event that the card is a queen, denoted as e, and the event that the card is a heart, denoted by f, are not independent.
Independent events are events where the occurrence or outcome of one event does not affect or influence the occurrence or outcome of another event. In other words, the outcome of one event has no effect on the probability of the other event happening. For example, flipping a coin and rolling a dice are independent events, because the outcome of one event (e.g., heads on a coin flip) does not affect the outcome of the other event (e.g., rolling a six on a dice).
The event "the card is a queen" reduces the number of cards in the deck, while the event "the card is a heart" also reduces the number of cards in the deck. The intersection of these two events, "the card is a queen of hearts," is a smaller set of cards than either event individually, so these events are dependent.
Learn more about independent events here: https://brainly.com/question/22881926
#SPJ4
Solve the equation.
20 = –d + 16
A. –4
B. –6
C. 4
D. –10
Answers
I hope this helps!!!!
Solve the inequality. 22−2x<38 Which answer represents the solution set?
Answers
Answer:
x >-8
Step-by-step explanation:
22−2x<38
Subtract 22 from each side
22-22−2x<38-22
-2x < 16
Divide each side by -2, remembering to flip the inequality
-2x/-2 > 16/-2
x >-8
A randomly generated list of integers from 0 to 7 is being used to simulate an event, with numbers 1 and 3 representing a success. What is the estimated probability of a success?
Answers
The estimated probability of a success is 3/8.
What is probability?Probability simply means the likelihood that a particular event will take place or occur based on the fact provided.
In this case, randomly generated list of integers from 0 to 7 is being used to simulate an event. Therefore, it means that there are 8 numbers.
Also, the numbers 1 and 3 representing a success.
Therefore, the probability that it's going to be a success will be:
= Number of success / Total number
= 3/8
Learn more about probability on:
brainly.com/question/24756209
#SPJ1
You wake up one morning, and find yourself wearing a toga and scarab ring. Always a logical person, you conclude that you must have become an Egyptian pharoah. You decide to honor yourself with a pyramid of your own design. You decide it should have height h 1020 and a square base with side s = 2400 To impress your Egyptian subjects, find the volume of the pyramid.
Answers
Given:
height, h = 1020 units
side of base, s = 2400 units
Required :
Volume of the Pyramid, V
Solution:
\(V=\frac{1}{3}Bh\)where B is the area of the base and h is the height of the pyramid.
Since the base of the pyramid is a square,
\(B=s^2\)Therefore,
\(\begin{gathered} V=\frac{1}{3}s^2h \\ V=\frac{1}{3}(2400)^2(1020) \\ V=1,958,400,000cubic.units \end{gathered}\)Answer:
The volume of the pyramid is 1,958,400,000 cubic units.
Answer:
To find the volume of the pyramid using integration, we can consider dividing the pyramid into an infinite number of small horizontal slices. Each slice will be a rectangle with width "dx" and length equal to the corresponding side of the square base. Let's denote the variable "x" as the height of each slice, starting from the base of the pyramid. The width of each slice, "dx," will represent a small change in height as we move from the base to the top. The area of each slice can be calculated as the area of a square base, which is "s^2," multiplied by the width "dx." So, the area of each slice is "s^2 * dx." To find the volume of the entire pyramid, we need to sum up the volume of all these small slices. This can be done by integrating the area function from x=0 (base of the pyramid) to x=h (top of the pyramid): Volume = ∫[0 to h] s^2 * dx In this case, s=1340 and h=1280. Integrating the function gives us: Volume = ∫[0 to 1280] 1340^2 * dx Volume = 1340^2 * ∫[0 to 1280] dx Integrating the function "dx" with respect to "x" simply gives us "x": Volume = 1340^2 * [x] [0 to 1280] Now, we can substitute the values of x=1280 and x=0 into the expression: Volume = 1340^2 * [1280 - 0] Volume = 1340^2 * 1280 Volume = 227,072,000 cubic units So, the volume of the pyramid is 227,072,000 cubic units. Note: It's important to understand that this is just one way to approach the problem. There may be alternative methods to find the volume of the pyramid using integration, but this is a commonly used approach.
Nathan usually drinks 36 ounces of water per day. He read that he should drink 64 ounces of water per day. If he starts drinking 64 ounces, what is the percent increase? Round to the nearest percent.
Answers
Answer:
78 percent
Step-by-step explanation:
percent increase = (64-36)/36 ×100
= 28/36 ×100 = 0.78×100= 78
Question 1
If your train travels at 65 miles per
hour for 3.5 hours, how far will it
go?
Answers
If your train travels at 65 miles per hour for 3.5 hours, it go 227.5 miles.
What is Speed ?
Velocity is the pace and direction of an object's movement, whereas speed is the time rate at which an object is travelling along a path. In other words, velocity is a vector, whereas speed is a scalar value.
Given:
Speed S = 65 miles per hour
Time T = 3.5 hours
We know that,
S= D/T Where D is the distance traveled.
Therefore,
65 miles per hour = D/ 3.5 hours
D = 65 x 3.5 miles
=227.5 miles
To learn more about velocity click on the link below:
https://brainly.com/question/21729272
#SPJ1
the sum of the height and radius of a right cylinder is 12 inches. what is the maximum volume of this cylinder
Answers
The maximum volume of this cylinder = 256π cubic inches
What is volume?
In three-dimensional space, volume is the area occupied by an object within its borders. Another name for it is an object's capacity.
Let h be the height and r be the radius.
Then, h+r = 12
=> h=12-r
Volume of the cylinder (V):
V=πr²h
=> h= πr² ( 12-r )
=> h= π ( 12r² - r³ )
Differentiate with respect to r , we get
dv/dr = 24 πr - 3πr²
Find r by taking dv/dr=0
24 πr - 3πr² = 0
3π ( 8r - r² ) = 0
8r = r²
r=8
Again differentiating, we get
d²V/dr² = 24π - 6 π r
When r=8, 24π - 48 π <0
So, V is maximum at r= 8 inches
And h= 12-8= 4 inches
Hence, the required volume of the cylinder = π8²*4 = 256π cubic inches
To learn more about the volume from the given link
https://brainly.com/question/463363
#SPJ4
in a standard television set, the screen height is 0.75 times the screen width. if a television set measures 26 inches along the diagonal, what is the screen width?
Answers
The width of a standard television set is equal tp 16.8 inches.
We will solve this by using the Pythagorean theorem which is below:
Or we can say
w = width
h = height
d = diagonal measure
We know the height is 0.75 times the width so 0.75w. We also know d = 26 , is our diagonal measure.
w = need to find
h = 0.75w
d = 26
\(w^2 + h^2 = d^2\\w^2 + 0.75w^2 = 26 ^2\\1.5625 w^2 = 441\\w^2 = 441/1.5625\\w ^2 = 282.24\)
w =16.8
Therefore width of a standard television set is 16.8 inches.
know more about Pythagorean theorem click here;
brainly.com/question/7721718
#SPJ4
Complete the square
x^2 - 2x - 14 = 0
Answers
To complete the square for the equation x^2 - 2x - 14 = 0, follow these steps:
1. Move the constant term to the right side of the equation: x^2 - 2x = 14
2. Take half of the coefficient of x, square it, and add it to both sides of the equation: x^2 - 2x + 1 = 15
3. Factor the left side of the equation as a perfect square: (x - 1)^2 = 15
4. Take the square root of both sides of the equation, remembering to include both the positive and negative square roots: x - 1 = ±√15
5. Add 1 to both sides of the equation to isolate x: x = 1 ± √15
Therefore, the solutions to the equation x^2 - 2x - 14 = 0 are x = 1 + √15 and x = 1 - √15.
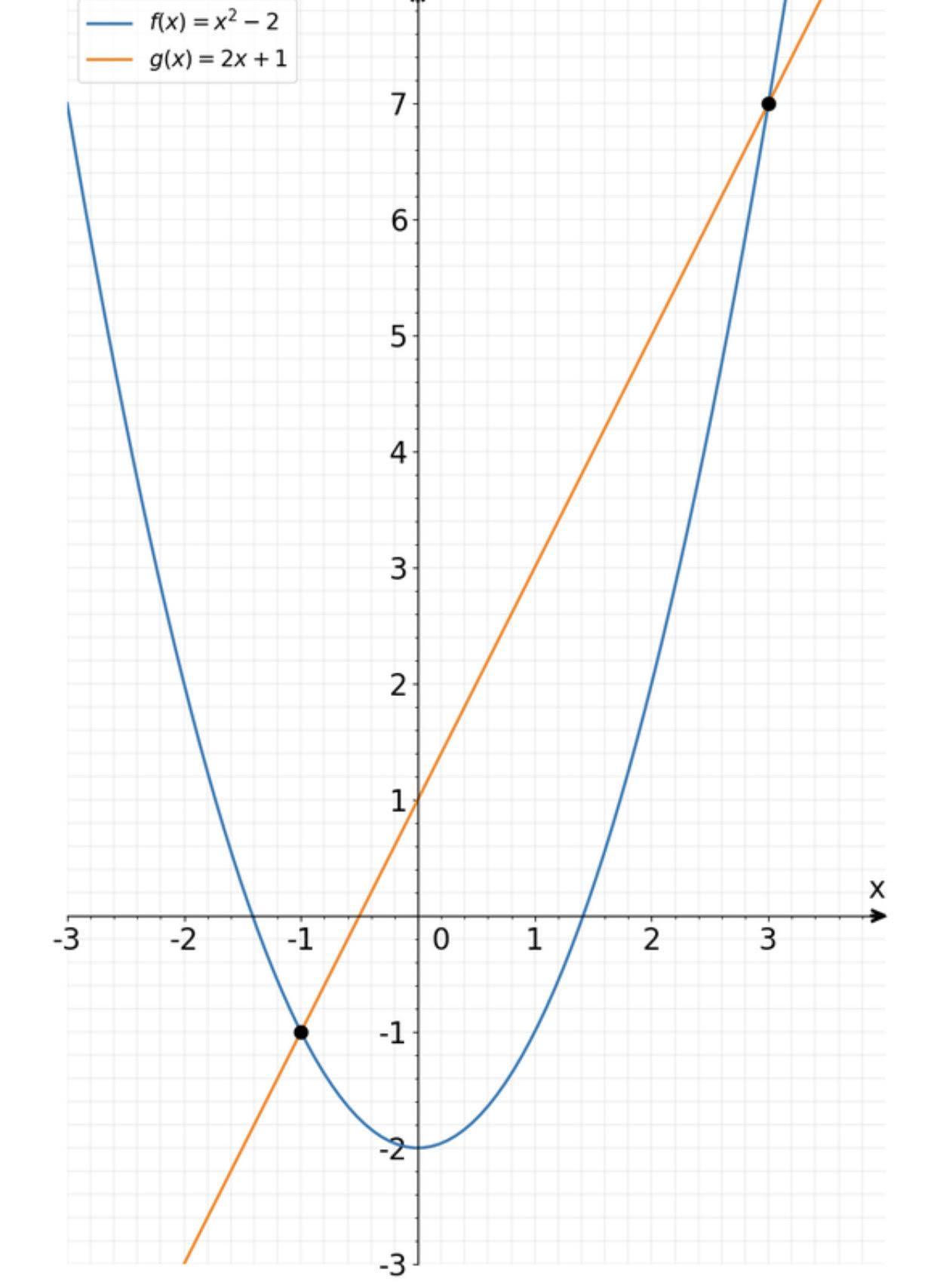
Graph the functions to find The solution.
f(x)=x^2 - 2
g(x)= 2x + 1
Answers
Answer: Look at the picture ⬇️
In creating a new scale for the mercury thermometer, Anders Celsius initially set the boiling point of water at _____.
Answers
Answer:
100°
Step-by-step explanation:
Anders Celsius used centigrade temperature scale along with mercury thermometers with a fixed boiling point of water at zero, he also used a fixed freezing point of water at the mark of 100-degree. This new scale was described in the year 1742 to Swedish Academy of Sciences.
Answer:
100 percent
Step-by-step explanation:
edge 2021