15. According to the US Census Bureau the population in Orange County, CA is growing at a rate of approximately 3.5% every 3 years . If the population of Orange County is approximately 3, 200,000 in 2014 , what will the approximate population be in 2020
Answers
Related Questions
Ian wraps a gift box in the shape of a square pyramid. The figure below shows a netfor the gift box.

Answers
Since we want to know how much wrapping paper he used in square centimeters, we want to figure the total surface area of the gift box.
Since it is a square pyramid, the triangles of the sides are all congruent, and the base is a square.
So, tha area of the square base is the multiplication of its sides, which are equal:
\(A_s=9.3\cdot9.3=86.49\)For the triangles, we can clauclate the area of one of them and multiply by 4 to get the area of all. The area of a triangle is its base multiplyed by its height divided by 2. The height is given and the base is the same as the square sides. So the area of 1 triangle is:
\(A_t=\frac{9.3\cdot9.6}{2}=44.64\)So, the tota area is the area of the square plus 4 times the area of one triangle:
\(A=86.49+4\cdot44.64=86.49+178.56=265.05\)This is already in the proper unit, so the area is 265.05 cm².
Verify that is an orthogonal set, and then find the orthogonal projection of y onto span
Answers
The orthogonal projection of y onto span will be given below.
\(Proj(y) = \begin{bmatrix}6\\3 \\0\end{bmatrix}\)
What is an orthogonal projection?A projection in which the spectrum and zero spaces are perpendicular subspaces is called an orthogonal projection.
If the inversion of a square matrix containing real figures or components equals the inverse matrix, the matrix is said to be orthogonal. Or, to put it another way, an orthogonal matrix is one in which the multiplication of a two - dimensional array and also its transpose yields an identity matrices.
\(y = \begin{bmatrix}6 \\3 \\-2\end{bmatrix} , \ u_1 = \begin{bmatrix} 3\\ 4\\0\end{bmatrix}, \ u_2 = \begin{bmatrix} -4\\ 3\\0\end{bmatrix}\)
Now we have
\(\left ( u_1 \cdot u_2 \right ) = \left (\begin{bmatrix} 3\\ 4\\0\end{bmatrix} \cdot \begin{bmatrix} -4\\ 3\\0\end{bmatrix} \right )\)
(u₁u₂) = -12 + 12 + 0
(u₁u₂) = 0
Thus, u₁ and u₂ are orthogonal.
|u₁|² = 3² + 4² + 0 = 9 + 16 + 0 = 25
|u₂|² = (-4)² + 3² + 0 = 16 + 9 + 0 = 25
\(\left ( y \cdot u_1 \right ) = \left (\begin{bmatrix} 6\\ 3\\-2\end{bmatrix} \cdot \begin{bmatrix} 3\\ 4\\0\end{bmatrix} \right ) = 18 + 12 + 0 = 30\)
and
\(\left ( y \cdot u_2 \right ) = \left (\begin{bmatrix} 6\\ 3\\-2\end{bmatrix} \cdot \begin{bmatrix} -4\\ 3\\0\end{bmatrix} \right ) = -24 + 9 = -15\)
Then the orthogonal projection will be given as
\(Proj(y) = \dfrac{(y,u_1)}{|u_1|^2} + \dfrac{(y,u_2)}{|u_2|^2} \\\\\\Proj(y) = \dfrac{30}{25} \begin{bmatrix} 3\\ 4\\0\end{bmatrix} - \dfrac{15}{25} \begin{bmatrix} -4\\ 3\\0\end{bmatrix}\\\\\\Proj (y) = \dfrac{1}{5} \begin{bmatrix} 18+12\\ 24-9\\0\end{bmatrix}\)
\(Proj(y) = \dfrac{1}{5}\begin{bmatrix} 30\\ 15\\0\end{bmatrix}\\\\\\Proj(y) = \begin{bmatrix}6\\3 \\0\end{bmatrix}\)
More about the orthogonal projection link is given below.
https://brainly.com/question/2292926
#SPJ4
The local middle school has students 2924 The principal wants the students in groups of 24 for an assembly How many groups of students will there be?
Answers
Answer:
1239 it is 1239 I think I have same test
HELP PLEASE SHOW ME HOW TO DO THIS

Answers
Answer:
Step-by-step explanation:
Salary increase in 2 years = Salary in fifth year - salary in 3rd year
= 87,023 - 43,250
= $ 43,773
Salary increase per year= 43773/2 = $ 21,886.50
jane is 3 times as old as kate. in 5 years jane's age will be 2 less than twice kate's. how old are the girls now
Answers
Answer:
Kate is 3 years old, Jane is 9 years old
Step-by-step explanation:
1.) First, assign variables to all of their ages. If we say Kate's age is x, Jane's age is 3 times this, which can be written as j, is 3x.
2.) Jane's age is also 2 less than twice of Kate's in 5 years. This means that her age is also 2(x+5) - 2 = j + 5. With a little simplification, you get that 2x + 8 = j + 5.
3.) Since j, Jane's age, is also 3x, we can substitute 3x in for j in the second equation. If you do this, you get 2x + 8 = 3x + 5.
4.) By moving the x onto one side and the numbers onto another, you get x = 3. X was Kate's age, meaning that Kate is 3 years old.
5.) Finally, since Jane's age is 3 times Kate's age, Jane's age is 3 * 3, which is 9. Jane is 9 years old.
Jane is 9 years old and Kate is 3 years old.To solve the problem, let's first establish variables for Jane and Kate's ages.
Let J represent Jane's age and K represent Kate's age.
According to the student question, Jane is 3 times as old as Kate, which can be represented as:
J = 3K
In 5 years, Jane's age will be 2 less than twice Kate's age, which can be represented as:
J + 5 = 2(K + 5) - 2
Now we can solve the equations step by step:
Substitute the first equation into the second equation to eliminate one of the variables:
3K + 5 = 2(K + 5) - 2
Distribute the 2 on the right side of the equation:
3K + 5 = 2K + 10 - 2
Simplify the equation by combining like terms:
3K + 5 = 2K + 8
Move the 2K term to the left side of the equation:
K = 3
now we know that Kate is currently 3 years old.
Substitute K's value back into the first equation to find Jane's age:
J = 3K
J = 3(3)
Simplify to find Jane's age:
J = 9
So, Jane is currently 9 years old.
Jane is 9 years old and Kate is 3 years old.
for more questions on variables
https://brainly.com/question/9302141
#SPJ11
I need answers to both of the questions Please help

Answers
Answer:
131 minutes, an = 15 + (n - 1)(4)
Step-by-step explanation:
each time she is increasing by 4 minutes
we can write this equation like this since we have the difference and the first part:
an = 15 + (n - 1)(4)
note
"an" (or ace of n)'s n is in subscript and is written just like a superscript but on the bottom
we can plug in this equation to find ace of 30 (a30)
15 + (30 - 1)(4)
15 + 29*4
116 + 15
a30 = 131
Answer:
1.) 192 minutes
2.) If it's the 9th day : 69 minutes
If it's the 10th day : 64 minutes
Step-by-step explanation:
1.) 5th Day - 32 minutes
32*6= 192 minutes on the 30th day.
2.) If it's the 9th day -
3rd Day - 23 minutes
23*3= 69 minutes
2.)If it's the 10th day -
5th day - 32 minutes
32*2= 64 minutes
The least value of the function x^2 + px + q is 3 and this occurs when x = - 2. find the values of p and q
Answers
Answer:
Hello,
p=4
q=7
Step-by-step explanation:
The vertex of the parabola is (-2,3)
Equation of the parabola is k(x+2)²+3=x²+px+q
Let's identify the coefficients:
kx²+4kx+4k+3=x²+px+q
so
k=1
4*1=p
4*1+3=q
The values of the coefficients for the equation of the parabola are p = 4, and q = 7.
Given that,
vertex of the parabola is (-2,3)
The equation of the parabola is given as:
k(x + 2)² + 3 = x² + px + q
Substitute the value of k = 1 into the equation:
(x + 2)² + 3 = x² + px + q
Now, let's expand (x + 2)²:
(x + 2)² = x² + 4x + 4
Substitute this back into the equation:
x² + 4x + 4 + 3 = x² + px + q
Now, simplify the equation:
x² + 4x + 7 = x² + px + q
Now, let's identify the coefficients:
Coefficient of x²:
1 = 1
Coefficient of x:
4 = p
Constant term:
7 = q
Therefore, the values of the coefficients are p = 4, and q = 7.
Learn more about Equation of parabola here:
https://brainly.com/question/11911877
#SPJ7
Please help me ASAP! 5th grade math. Will give brainliest!

Answers
The first question is B
the second one is c
Q1.
7 ÷ 8 = 7/8
1 ÷ 8 = 1/8 Answer
8 ÷ 7 = 8/7
1 ÷ 8 = 1/8
Q2.
5 ÷ 6 = 5/6
6 ÷ 5 = 6/5
1 ÷ 6 = 1/6 Answer
6 ÷ 1 = 6/1 or 6
Hopefully it Help you out :-)
Help plz don’t send the annoying link

Answers
Answer:
\(x^{2} +2x-15\)
Step-by-step explanation:
(x+5)(x-3)
simplifies to:
\(x^{2} +2x-15\)
If 4 is 1/2 , what is the whole?
Answers
Line m passes through point (5,- 8) and has a slope - 3. What is the equation for the line point slope form
Answers
Answer:
\(y + 8 = -3(x-5)\)
Step-by-step explanation:
Point Slope form is:
\(y - y_1 = m(x-x_1)\\\rule{150}{0.5}\\\text{ m - slope}\\(x_1,y_1) \text{ - point}\\\rule{150}{0.5}\)
We are given the point (5, -8) and the slope of -3.
\(y-y_1=m(x-x_1)\rightarrow y - (-8) - -3(x-5)\rightarrow \boxed{y+8=-3(x-5)}\)
Solve for b.
b - 16 = 83
b =
Answers
Answer:
Hello! answer: b = 99
Step-by-step explanation:
To find b we just have to add 83 + 16 so
83 + 16 = 99 and 99 - 16 = 83 So B = 99
Answer:
B=99
Step-by-step explanation:
in our school school we were told that if a number is on the other side of the equals and it is - when you take it to the other side it will be addition so that is what I've just done hope you understand and if it was addition when we take it to the other side it would be subtraction.

a gorcery store sells a smal case of 6 bottles of water $4 and large case of q8 bottles for $10. Is the price per bottle pf water proportional?
Answers
6 bottles for $4 each bottle cost $1.50
Select the correct answer from each drop-down menu.The function f is given by this table of values.х-10Use this table to complete the statements.The parent function of the function represented in the table isIf function fis vertically compressed by a factor of , the-values will beA point in the table for the transformed function is


Answers
As we can note the tables shows a linear behavior. Then, the parent function of the function represented in the table is linear.
If function f is vertically compressed by a factor of 1/4, the f(x) - values will be divided by 4.
excel assume that when adults with smartphones are randomly selected, 56% use them in meetings or in classes. if 25 adult smartphone users are randomly selected, find the probability that exactly 20
Answers
P(exactly 20 out of 25 users) ≈ 0.00806
What is probability?Generally, In mathematics and statistics, probability is a measure of the likelihood that an event will occur. It is expressed as a number between 0 and 1, with 0 indicating that the event is impossible and 1 indicating that the event is certain.
The probability of an event can be calculated by dividing the number of ways that event can occur by the total number of possible outcomes. For example, if a fair coin is flipped, the probability of it landing heads up is 1/2 because there is only one way for it to land heads up out of two possible outcomes (heads or tails).
Probabilities can be expressed in different forms, including fractions, decimals, and percentages. They can also be combined using mathematical operations such as addition, multiplication, and conditional probabilities to calculate more complex probabilities.
Probability theory has applications in many fields, including science, engineering, economics, and social sciences. It is used to make predictions, design experiments, and analyze data.
P(exactly 20 out of 25 users) = (25C20)(0.56^20)(0.44^5)
P(exactly 20 out of 25 users) ≈ 0.00806
Read more about probability
https://brainly.com/question/30034780
#SPJ1
CQ
Excel assume that when adults with smartphones are randomly selected, 56% use them in meetings or in classes. if 25 adult smartphone users are randomly selected, find the probability that exactly 20 of them use their phones in meetings or in classes
The coordinates of the vertices of the preimage of a triangle are (2, 1), (3, 4), and (4, 1). The coordinates of the vertices of the image are (3, 3), (4, 6), and (5, 3). How far and in what direction was the triangle translated?
Answers
pls help asap if you can!!!!

Answers
The statement that proves that angle XWY is equal to angle ZYW is
A. If two parallels are cut by a transverse, then alternate interior angles are congruent
What are alternate interior anglesAlternate interior angles are a pair of angles that are formed on opposite sides of a transversal line when two parallel lines are intersected by the transversal.
When a transversal intersects two parallel lines, it creates eight angles. Among these angles, the alternate interior angles are located on the inside of the parallel lines and on opposite sides of the transversal.
In a parallelogram, the two opposite sides are parallel to each other hence the line crossing them will lead to formation of alternate interior angles
Learn more about alternate interior angles at
https://brainly.com/question/20344743
#SPJ1
A student had taken 7 tests and received scores of 88, 73, 81, 83, 79, 73, and 97.
what is the median, mode, and range of the test scores
Answers
Answer:
median=81
mode=73
range=24
Step-by-step explanation:
median: Middle number from lowest to highest crossing one-off from either side.
(73,73,79,81,88,97)
mode:most popular number.
range: highest number minus lowest number.
Solve |x+2| >5-(x-1)^2 by graphing
Answers
Answer:
x
<
−
1
or
x
>
2
Step-by-step explanation:

Help me on this please 20 POINTS!!!!
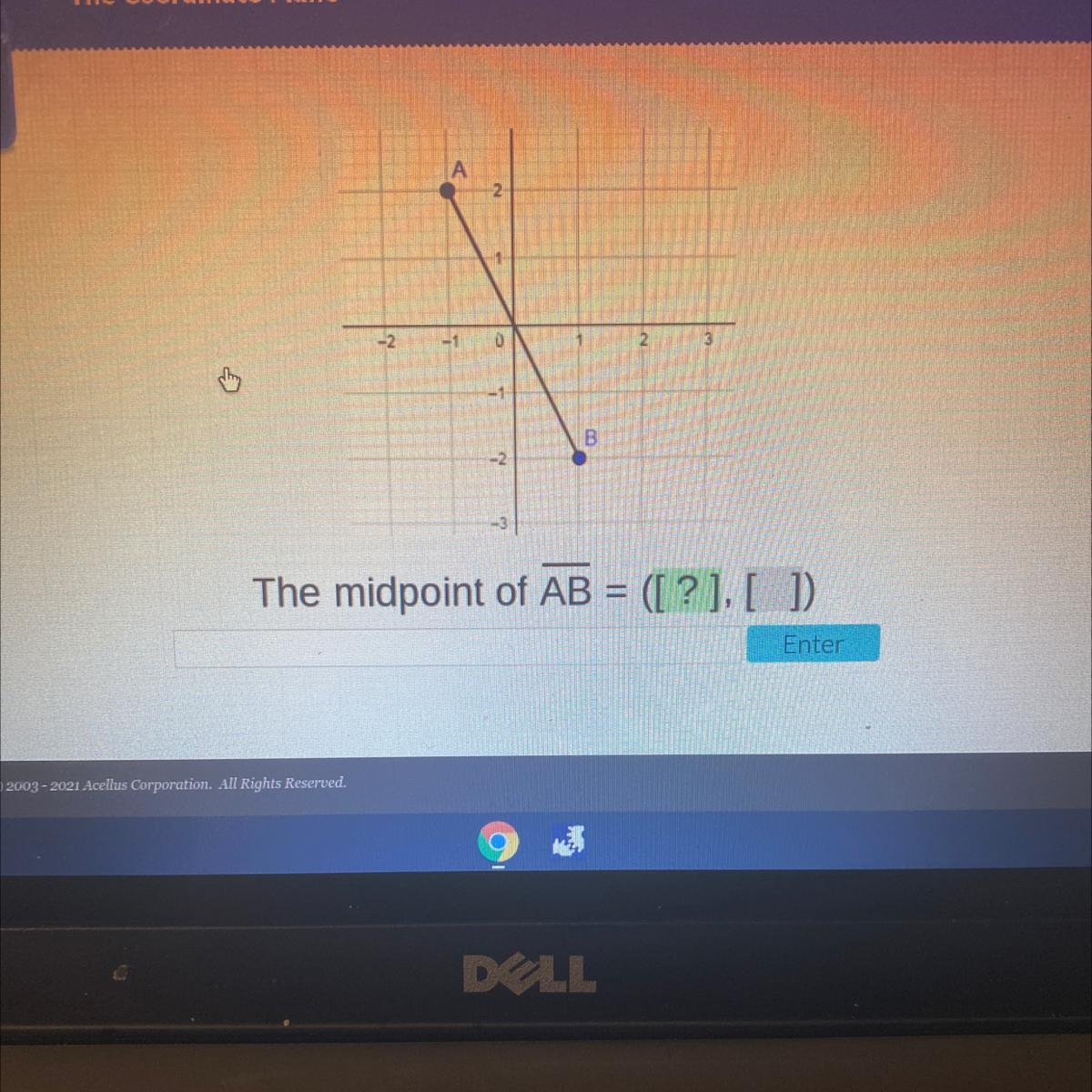
Answers
Answer:
(0,0)
Step-by-step explanation:
2y-2z= -4
- 4y+2z = -2
x+4y-z=-5
Answers
Answer:
(-12,3,5)
x=-12,y=3.z=5
Step-by-step explanation:
Which equations are true for x = –2 and x = 2? Select two options
O x2 – 4 = 0
O x2 = –4
O 3x2 + 12 = 0
O 4x2 = 16
O 2(x – 2)2 = 0
Answers
The only two options with equations that are true for x = –2 and x = 2 are;
Option A: x² - 4 = 0
Option D: 4x² = 16
How to identify the correct domain value?The domain of a function is the set of all input values for which the function is possible.
Now, we are given the domain values as x = –2 and x = 2.
a) x² - 4
f(-2) = (-2)² - 4
= 0
f(2) = (2)² - 2
= 0
b) x² = -4
f(-2) = (-2)²
= 4
f(2) = (2)²
= 4
c) 3x² + 12
f(-2) = 3(-2)² + 12
= 24
f(-2) = 3(2)² + 12
= 24
d) 4x²
f(-2) = 4(-2)²
= 16
f(2) = 4(2)²
= 16
e) 2(x - 2)²
f(-2) = 2(-2 - 2)²
= 32
f(2) = 2(2 - 2)²
= 0
Read more about domain values at; https://brainly.com/question/2264373
#SPJ1
a coin is to be tossed 1000 times. what is the probability that the 785th toss is heads? group of answer choices 0 1/4 1/2 3/4 1
Answers
A coin is to be tossed 1000 times, the probability that the 785th toss is heads is 1/2.
Hence option c is the correct answer.
A coin is to be tossed 1000 times and the probability of the outcome to be Heads oor Tails is recorded accordingly.
Probability of an event refers to the possibility of the event to occur or in other words, how likely is tthe event to occur. Probability ranges from 0 to 1 as number and 0% to 100% as percentage.
Probability can be calculated with the formula as,
Probability of an event to occur = (Nmber of favourable outcomes) / (Total number of outcomes possible)
Here, when tossing a coin we have two outcomes, Heads and Tails
The number of favourable outcome, that is the outcome is Heads is 1.
Thus the probability that the outcome is a Head = 1/2
When a coin is tossed for 785th time the probability that the head will come up = 1/2 (assuming that the coin is unbiased).
The probability remains unchanged for tossing the coin for the first time and for the 785th time since no number of times the coin is tossed the number of favourable outcome is 1 and total outcomes is 2.
To know more about probability here
https://brainly.com/question/5495713
#SPJ4
The volume of this cone is 643,072 cubic inches. What is the radius of this cone?
Use ≈ 3.14 and round your answer to the nearest hundredth.
Answers
The radius of the cone is 783.84/√h
What is volume of a cone?A cone is the surface traced by a moving straight line (the generatrix) that always passes through a fixed point (the vertex).
Volume is defined as the space occupied within the boundaries of an object in three-dimensional space.
The volume of a cone is expressed as;
V = 1/3πr²h
643072 × 3 = 3.14 × r²h
r²h = 614400
r² = 614400/h
r = 783.84/√h
therefore the radius of the cone is 783.84/√h
learn more about volume of cone from
https://brainly.com/question/1082469
#SPJ1
angle is constructed with its base on the x-axis and its upper two vertices on the parabola . what are the dimensions of the rectangle with the maximum area? what is the area?'
Answers
The double integral of sin(xy) over the rectangle r equals 1 when a is approximately equal to 0.986, with 0 ≤ a ≤ π.
The iterated integral to compute the double integral over the rectangle r = {(x,y) : 0 ≤ x ≤ π, 0 ≤ y ≤ a} of sin(xy) is given by:
∫∫r sin(xy) dA = ∫₀^a ∫₀^π sin(xy) dx dy
Integrating with respect to x first, we have:
∫₀^a ∫₀^π sin(xy) dx dy = ∫₀^a [-cos(πy) + cos(0)] dy
= ∫₀^a (1 - (-1)^n) dy
= a - (a/π)sin(πa)
For what values of a is ∫∫r sin(xy) dA equal to 1?
We need to solve the equation:
a - (a/π)sin(πa) = 1
Multiplying both sides by π, we get:
aπ - a sin(πa) = π
Now, let f(a) = aπ - a sin(πa) - π. We need to find the values of a such that f(a) = 0.
Using numerical methods, we can find that there is only one solution in the interval [0,π], which is approximately a = 0.986.
Therefore, the double integral of sin(xy) over the rectangle r equals 1 when a is approximately equal to 0.986, with 0 ≤ a ≤ π.
Click the below link, to learn more about Double integral:
https://brainly.com/question/30217024
#SPJ11
Which quadrant will the figure be located in after a 180 degree rotation?
A) Quadrant 2
B) Quadrant 1
C) Quadrant 3
D) Quadrant 4

Answers
Cuz it’s gonna be on the opposite side of the graph because it’s 180 degrees
-2,-7,-12 ,.... is an arithmetic sequence Find the sum of its first 20 terms ?
Answers
Given sequence:
-2,-7,-12 ,..
Unknown;
Find the sum of the first 20 terms = ?
Solution:
The sequence is -2,-7,-12 ,..
To find he sum of n-terms of a sequence, we use the expression below;
Sₙ = \(\frac{n}{2} (2a + (n-1)d)\)
where n is the number of terms = 20
a is the first term = -2
d is the common difference= -7 - (-)2 = -5
Now input the parameters and solve;
Sₙ = \(\frac{20}{2} (2(-2) + (20 - 1) x -5)\)
Sₙ = 10(-4 + -5(19)) = 10(-99) = -990
The sum of the first 20 terms of the sequence is -990
Can someone help me with B and D, i’ll give branliest! :)

Answers
A = final amount
P= initial principal balance
r = annual interest rate
‡ = time (in years)
A=400(1(0.03)0)
A=400(1(0.03)5)
A=400(1(0.03)10)
A=400(1(0.03)15)
A=400(1(0.03)20)
0=400
5=460
10=520
15=580
20=640
what is 964,019 rounded to the nerest hundred thousand
Answers
Answer:
1,000,000
Step-by-step explanation:
964,019 rounded to the nearest hundred thousand is 1,000,000
The 6 in 964,019 makes the number into 1,000,000
Tina and Annette are planning to go to New York for four days. The travel options are shown below. Which travel option has the best rate?
Option A: $200 per night, $250 flight
B: $300 per night, $200 flight
C: $150 per night, $500 flight
Answers
Answer:
option A
Step-by-step explanation:
Option A is 1,050 in total
option B is 1,400 in total
option C is 1,100 in total
that's my go at it