2/3 of the purses made by the class are blue, 1/8 of the blue purses have red ribbon straps. what fraction of the purses are blue and have red ribbon straps.
Answers
Answer:
1/12
Step-by-step explanation:
2/3 times 1/8 is 1/12.
Answer:
1/12
Step-by-step explanation:
2/3 times 1/8. Which would give 1/12 kinda like what the other answer was so yeah.Have a nice and blessed day.
Related Questions
Will give brainliest for answer

Answers
Answer:
y > -x -3
Step-by-step explanation:
The graph is shaded above the dashed line, indicating y-values in the solution are greater than those on the line, so your inequality will start with ...
y >
The y-intercept of the line is (0, -3), so the "b" value in ...
y > mx +b
will be -3.
The line has a rise of -3 for a run of 3 (between the marked points), so the slope is ...
m = rise/run = -3/3 = -1
Then the inequality you want is ...
y > -x -3

You are choosing between two health clubs. Club A offers membership for a fee of $12 plus a monthly fee of $28. Club B offers membership for a fee of $20 plus a
monthly fee of $26. After how many months will the total cost of each health club be the same? What will be the total cost for each club?
In __ months the total cost of each health club will be the same.
Answers
A has fixed one time fee of $12 and if you go to it say "m" months you pay $28 for each month, so your total cost at A is really 12 + 28m.
B has a fixed one time fee of $20 and if you go to it "m" months you pay $26 for each month, so you total cost at B is 20 + 26m.
how many months for the cost to be the same?
\(\stackrel{A}{12+28m}=\stackrel{B}{20+26m}\implies 12+2m=20\implies 2m=8\implies m=\cfrac{8}{2}\implies m=4\)
well, since the cost for both is the same, we can just get A's, knowing that B is the same
\(12+28(4)\implies 12+112\implies 124\)
For what value of X must ABCD be a parallelogram?
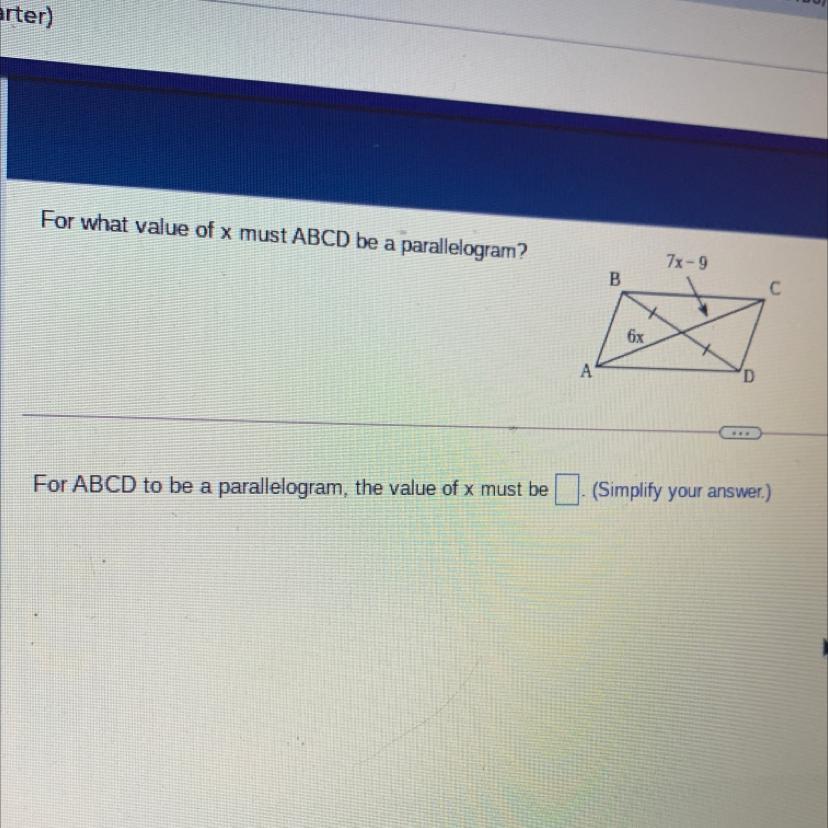
Answers
Answer:
x = 9
Step-by-step explanation:
If ABCD is a parallelogram, then 6x = 7x - 9
as the point of intersection of the 2 diagonals is the midpoint of AC
6x = 7x - 9
Subtract 6x from both sides:
⇒ 0 = x - 9
Add 9 to both sides:
⇒ 9 = x
Solution:
We know that:
\(\frac{AC}{2} = 6x\)This means that:
\(AC = (6x)(2) = 12x\)\(AC = 12x = 6x + 7x - 9\)Step-by step calculations:
Simplify the RHS:
\(12x = 6x + 7x - 9\)=> \(12x = 13x - 9\)Subtract 13x both sides:
=> \(-13x + 12x = 13x -13x - 9\)=> \(-x = -9\)Divide -1 both sides:
=> \(-x = -9\)=> \(\frac{-x}{-1} = \frac{-9}{-1}\)=> \(x = 9\)What is the product of 3/4 and -6/7?
Answers
Answer:
-9/14
Step-by-step explanation:
Hey there!
Guide:
• Difference means subtract/subtraction
• Product means multiply/multiplication
• Sum means add/addition
• Quotient means divide/division
• Now that we know what “product” means… we can make the question/equation easier to solve.
3/4 × -6/7
= 3(-6) / 4(7)
= -18 / 28
= -18 ÷ 2 / 28 ÷ 2
= -9 / 14
Therefore, your answer is: -9/14
Good luck on assignment & enjoy your day!
~Amphitrite1040:)
A mechanical device is used to launch a potato vertically into the air. The potato is launched from a platform 20 feet above the ground, with an initial vertical velocity of 92 feet per second
The function h(t) = -16t^2+ 92t + 20 models the height of the potato over the ground, in feet, t seconds after launch,
Here is the graph representing the function.
Part A
1. What is the height of the potato 1 second after launch?
2, 8 seconds after launch, will the potato still be in the air ?
3, Will the potato reach 120 feet? If so, when will it happen ?
4. When will the potato hit the ground?

Answers
1) The potato reaches a height of 96 feet at a second after launch.
2) The potato cannot be in the air at t = 8 seconds.
3) The potato will reach a height of 120 feet at t = 4.295 seconds and t = 1.455 seconds.
4) The potato will hit the ground at t = 5.960 seconds.
How to analyze and interpret the height of a potato being launched
In this question we find the case of a potato being thrown to the air, the height of the potato as a function of time is a quadratic equation. The first part consists in determining the height of the potato a second after its launch:
h(1) = - 16 · 1² + 92 · 1 + 20
h(1) = - 16 + 92 + 20
h(1) = 96
In the second part we must determine if the potato is still in the air after 8 seconds:
h(8) = - 16 · 8² + 92 · 8 + 20
h(8) = - 268
Since h(8) < 0, then the potato cannot be in the air when t = 8 seconds.
The third part asks us if the potato reaches a height of 120 feet and, if possible, when it will happen:
120 = - 16 · t² + 92 · t + 20
- 16 · t² + 92 · t - 100 = 0
16 · t² - 92 · t + 100 = 0
16 · [t² - (23 / 4) · t + 25 / 4] = 0
t₁ = 4.295 s or t₂ = 1.455 s.
Finally, we determine when the potato will hit the ground:
- 16 · t² + 92 · t + 20 = 0
t₁ = 5.960 s or t₂ = - 0.210 s
The potato will hit the ground at t = 5.960 seconds.
To learn more on quadratic equations: https://brainly.com/question/1863222
#SPJ1
15% of the seats on an airplane are first class if there are 24 class seats how many seats are on the plane
Answers
Answer:
160
Step-by-step explanation:
there are 24 first class seats. 15% of the total number is 24. that would mean 5% of the total number is 8. 20 times 8 is 160.
How do you write 5.44 in words?
Answers
Answer:
five and forty-four hundredths
Step-by-step explanation:
Answer:
five point four four
Step-by-step explanation:
28453 dg a t
porfa ayudenme
pipipipi
Answers
Answer:
488
Step-by-step explanation:
You divide 28453 by 58.3053278689 and get 488.
Identify the interquartile range from the box and whisker plot above

Answers
Answer:
interquartile range = 6
Step-by-step explanation:
the interquartile range is the difference between the upper quartile Q₃ and the lower quartile Q₁
Q₃ is the value at the right side of the box, that is 86
Q₁ is the value at the left side of the box, that is 80
interquartile range = Q₃ - Q₁ = 86 - 80 = 6
Which graph has the parent function 1/x?
Answers
Answer:
The graph of parent function \(f(x)=\frac{1}{x}\) is a hyperbola.
Step-by-step explanation:
A rational function is described as the fraction of polynomials, where the denominator has degree of at least 1 .
Or it can be said that there must be a variable in the denominator.
The general form of a rational function is:
\(\text{Rational Function}= f(x)=\frac{p(x)}{q(x)}\)
In this case the parent function provided is: \(f(x)=\frac{1}{x}\).
The function is rational.
The graph of parent function \(f(x)=\frac{1}{x}\) is a hyperbola.
The graph is attached below.

Please help. Solve the following system of linear equations by substitution. Show all work.
Y= -2
4x -3y=18
Answers
Answer:
The solution (3, -2).
Step-by-step explanation:
The easiest way to find this solution is inputting these two equations into Desmos Graphing Calculator!
Answer:
x = 3,y = -2
Step-by-step explanation:
Given data:
Y= -2
4x -3y=18
Solve:
Substitute the given y-value into 4x−3y=18 and solve for x
Since, y = -2,
\(4x-3(-2)=18\)
\(4x+6=18\)
\(4x+6-6=18-6\)
\(4x=12\)
\(\frac{4x}{4}=\frac{12}{4}\)
\(x = 3\)
The solution to the system of equations is x = 3, y = -2
~Learn with Lenvy~
Which is enough information to prove that line s is parallel to line t
Answers
Answer:
line s and t would not meet even if you extend them and also they have the same slope and gradient
1. Out of 318 seventh and eighth grade CAVA students there were 48 students that chose Music as their elective
course and the rest chose World Language. There were a total of 124 eighth grade students and 108 of them
chose World Language as their elective.
Use this information to complete the two-way table completely.
Enter your answer by filling in the boxes to complete the table (5 pts)
Answer:
8th Grade
7th Grade
Totals
Music
48
World Language
108
Totals
124
318

Answers
The students in 8th class who choose music are 16 in number,
The students in 7th class who choose music are 32 in number
The total who choose world language is 270
The totals who are in 7th grade be 194
The number of students who choose world language in 7th class are 162
Let x be the students in 8th class who choose music
x+108=124
x=124-108
x=16 students
Now y be the students in 7th class who choose music
16+y=48
y=48-16
y=32
Let z be the total who choose world language
48+z=318
z=318-48
z=270
Now the totals who are in 7th grade be A
124+A=318
A=318-124
A=194
B be the number of students who choose world language in 7th class
Now 32+B=194
B=194-32
B=162
To learn more on Equation:
https://brainly.com/question/10413253
#SPJ1
not a school question but how do i get more points lol
Answers
PLEASE HELP
Graph the following equation:
y=1/4x-2
Answers
Answer:
see explanation
Step-by-step explanation:
-2 is your y-intercept so one point on your plot is (0,-2)
your slope is 1/4, rise/run
so from the y-int go up 1 and right 4, so another point is (-1,4)
and you can keep going up 1 and right 4 for as many points as you need.
On a temperature versus time graph, how does the temperature at the beginning of a change of state compare with the temperature at the end of the change? always lower always the same usually lower usually higher
Answers
Answer:
Always the same
Step-by-step explanation:
The temperature at the beginning of a change of state is always the same as the temperature at the end.
This is because phase change is an isothermal process. it means that all the energy absorbed during the phase change process is utilized in the breaking of the bonds in the compound as it changes from one state of matter to another.
As a result, no increase in the temperature of the material will be detected by the thermometer.
The range of this function is {2, 4, 5, 6}
2
4
5
6
8
13
O True
O False
Answers
Answer:
True l True-----------------^~^
Pls help!!!!!!!!!!!!!!!!!!

Answers
Answer:
d
Step-by-step explanation:
the figure below represent 7/9 of a full circle.What is the central angle
Answers
Answer:
Step-by-step explanation:
there is no figure Shown for
Your question
Find the area of a circle that has a radius of 12
Answers
Answer:
Step-by-step explanation:
452.39 is the area
How would I solve this using derivative rules? I know the answer is 5/2x^2 but what I don’t understand is how you get that. Because with the power rule I thought you had to take the derivative of both separately, and the derivative of 5/2 is 0

Answers
Answer:
The constant factor remains a multiplier.
Step-by-step explanation:
The derivative of "a·f(x)" is a·f'(x). That is, the constant multiplier remains a constant multiplier, unchanged.
For ax^-1, the derivative is a(-x^-2) per the power rule. For your value of a=-5/2, this is ...
\(\dfrac{d}{dx}\left(-\dfrac{5}{2x}\right)=\left(-\dfrac{5}{2}\right)\left(\dfrac{-1}{x^2}\right)=\dfrac{5}{2x^2}\)
on January 21, the high temperature in palm springs, California, was 89°f, and the high temperature in Whitefield, New Hampshire was-31°f. what was the difference between the temperature in palm springs and the temperature in Whitefield?
Answers
Palm springs temperature: 89° F
Whitefield temperature: 31°F
Subtract the tempreatures to obtain the difference:
89 -31 = 58°
Answer: 120 degrees
Step-by-step explanation:
89-(-31)
= 89+31
= 120 degrees
Eugene and Jessica each improved their yards by planting hostas and geraniums. They bought
their supplies from the same store. Eugene spent $150 on 18 hostas and 6 geraniums. Jessica
spent $113 on 7 hostas and 16 geraniums. Find the cost of one hosta and the cost of one
geranium.
Answers
The cost of one hosta is approximately $7 and the cost of one geranium is approximately $4.
To find the cost of one hosta and one geranium, we can set up a system of equations based on the given information.
Let's assume the cost of one hosta is represented by 'h' and the cost of one geranium is represented by 'g'.
From the information given, we can set up the following equations:
Eugene's spending:
18h + 6g = $150
Jessica's spending:
7h + 16g = $113
We can now solve this system of equations to find the values of 'h' and 'g'.
Multiplying the first equation by 2 and the second equation by 3 to eliminate 'g', we get:
36h + 12g = $300
21h + 48g = $339
Now, we can subtract the second equation from the first to eliminate 'h':
(36h + 12g) - (21h + 48g) = $300 - $339
36h - 21h + 12g - 48g = -$39
15h - 36g = -$39
Simplifying further, we have:
15h - 36g = -$39
Now we can solve this equation for 'h' and substitute the value back into any of the original equations to find 'g'.
Let's solve for 'h':
15h = 36g - $39
h = (36g - $39) / 15
Substituting this value of 'h' into Eugene's equation:
18[(36g - $39) / 15] + 6g = $150
(648g - $702) / 15 + 6g = $150
648g - $702 + 90g = $150 * 15
738g - $702 = $2250
738g = $2250 + $702
738g = $2952
g = $2952 / 738
g ≈ $4
Now, substituting the value of 'g' back into Eugene's equation:
18h + 6($4) = $150
18h + $24 = $150
18h = $150 - $24
18h = $126
h = $126 / 18
h ≈ $7
Therefore, the cost of one hosta is approximately $7 and the cost of one geranium is approximately $4.
For such more questions on Cost of Hosta & Geraniums
https://brainly.com/question/14324591
#SPJ8
From Monday - Tuesday, Adam earns $122 dollars. If he continues to earn the same amount for the rest of the week, how much will he have at the end?
Answers
Answer:
$437
Step-by-step explanation:
There are 7 days in a week.
Let's stop at the last even number, the 6th day.
Tuesday is four days away from Sunday, so multiply 122 by 3.
\(\mathrm{122}\) × \(3\) = \(366\)
Finally, we are at the 7th day. There is no 8th day in a week, so divide 122 by 2.
\(122\) ÷ \(2\) \(=61\)
Now, let's add 61 to 366. We get $437, and that is our answer.
Hope this helps!
Select all ratios equivalent to
12:9
Answers
A ratio equivalent to 12:9 is 4:3.
From the given choices, the correct option is B.
What is the ratio?A ratio is a mathematical relationship that demonstrates how frequently one number contains or is contained inside another. Two numbers are compared using it.
Given:
A ratio,
12:9.
To find the equivalent ratio of the given ratio:
Simplifying the given ratio,
12:9,
= 12/9
Factors of 12 are 1, 2, 3, 4, 6, and 12.
Factors of 9 are 1, 3, and 9.
The common factors of 12 and 9 are 1 and 3.
So, the equivalent ratios are;
12/9 = 4/3.
Therefore, the equivalent ratio is 4:3.
To learn more about the ratio;
https://brainly.com/question/13419413
#SPJ9
The complete question:
Select all ratios equivalent to
12:9
A. 12: 7
B. 4:3
C. 12:11
D: 12: 4
1. Write a rule in words and as an expression. Input Output 1 25 2 50 3 75 4 100
Answers
Output values are 25 times the input values, and the expression can be given as y = 25x.
What is an algebraic expression?An algebraic expression is consists of variables, numbers with various mathematical operations.
The given input values are,
1, 2, 3, and 4
The output values are,
25, 50, 75, and 100.
Let x represents the input values, and y represents the output values,
The expression for the input and output values can be give by,
y = k x (1)
Substitute x =1 , and y = 25
25 = k (1)
k = 25
To find the required expression, substitute k = 25 in equation (1),
y = 25x
y = 25x is the expression, in which y is 25 times the value of x.
To know more about Algebraic expression on:
https://brainly.com/question/19245500
#SPJ1
(4p^4)^2 without exponents
Answers
Answer:
(4p x 4) x 2 = 16p x 2 = 32p
Step-by-step explanation:
The required simplification is (4p⁴)² = 256p⁸.
What are the rules of exponents?Some of the rules of exponents are as follows,
The product of two exponents having the same power is equal to the power of their base multiplied.
The product of two exponents having the same base is equal to the sum of the powers of different exponents to the same base.
The given expression is as below,
(4p⁴)²
Without using the exponents the given expression can be simplified as follows,
The power to any number simply means that the number will be multiplied to itself as many number of times. this basic rule is used to obtain result as,
(4p⁴)²
= (4p × 4p × 4p × 4p)²
= (16p⁴)²
= 16p⁴ × 16p⁴
= 256 × (p × p × p × p × p × p × p × p)
= 256p⁸
Hence, the given expression can be simplified as 256p⁸.
To know more about exponents click on,
https://brainly.com/question/5497425
#SPJ2
Is -2 rational or irrational
Answers
Answer:
Rational.
Step-by-step explanation:
A rational number doesn't have a repeating decimal. -2 is a rational number.
Answer: irrational
Step-by-step explanation:
In other words, a rational number is negative, if its numerator and denominator are of the opposite signs. Each of the rational numbers -1/6, 2/-7, -30/11, 13/-19, -15/23 are negative rationals, but -11/-18, 2/5, -3/-5, 1/3 are not negative rationals. 1. ... Since both the numerator and denominator are of the same sign.
Juan is running a race. He ran for 3 hours at a speed of 7.5 miles per hour. For how many miles does he run
Answers
d = v x t
d = 7.5 miles/h x 3 = 22.5 miles
Answer:
22.5 miles
Step-by-step explanation:
3x7.5=22.5
Which of the following is the slope of the line y - 3x =0
Answers
Answer:
-3
Step-by-step explanation:
Answer: The slope whould be 3
Step-by-step explanation:
Graph the line using the slope and y-intercept, or two points.
Slope: 3
y-intercept:
(0,0)
x|y
----
0|0
1|3
