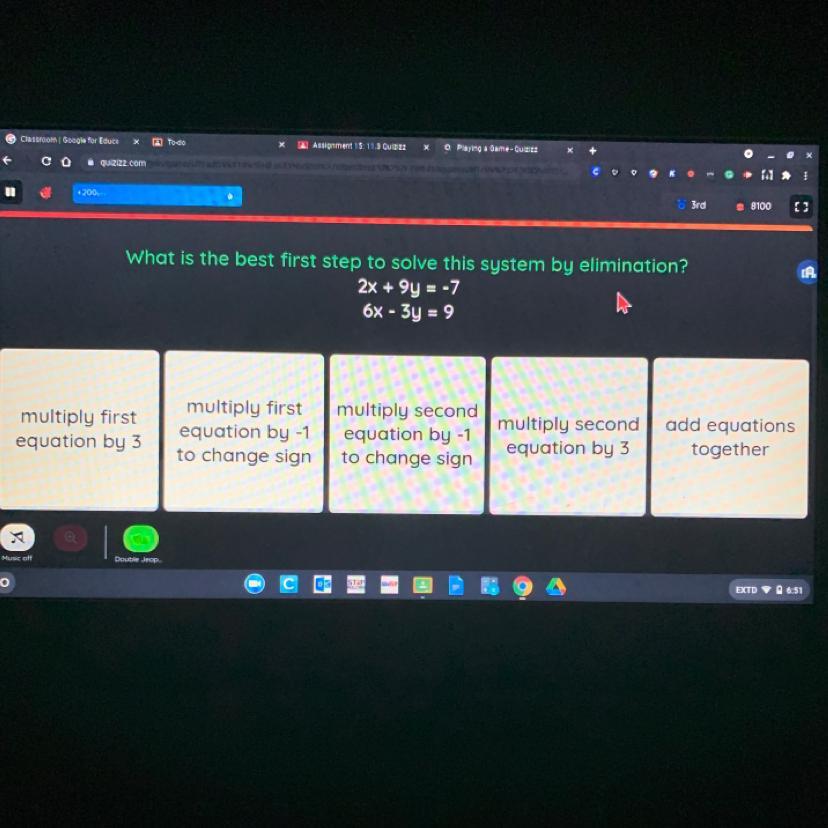
Answers
Answer:
Add the equations together
Step-by-step explanation:
The reason why I know this is because they are all like terms so that would be the smartest choice to do first.
If this answer helped you then please mark it as brainliest so I can get to the next rank
Related Questions
Being in series means that for the system to operate, both components a and b must work. assume the two components are independent. the probability a works is 0.90 and the probability b functions is also 0.90. what is the probability the system works under these conditions
Answers
The probability of a work is 0.90 and the probability of b functions is also 0.90. the probability the system works under these conditions is 0.81 (0.90 x 0.90 = 0.81).
This is because the system works if both components a and b are functioning, so we need to calculate the probability of both of them working, which is the probability of component a working (0.90) multiplied by the probability of component b working (0.90).
To get this answer, we can use the concept of joint probability which is the probability of two or more independent events happening at the same time. We can calculate the joint probability by multiplying the probabilities of each of the individual events.
In this case, the probability of the system working is the product of the probability of component a working (0.90) and the probability of component b working (0.90). This gives us a joint probability of 0.81, which is the probability the system works under these conditions.
To know more about joint probability refer to the link brainly.com/question/27098956
#SPJ4
What is 24/240 as a decimal
Answers
Answer:
0.1
Step-by-step explanation:
Just take 24/24 which is 1 and move the decimal one place to the left because there is an extra 0 on the denominator
What type of angle is an 11° angle?
Answers
Answer:
\(\large\boxed{\textsf{Acute Angle.}}\)
Step-by-step explanation:
\(\textsf{We are asked what type of angle is an 11}^{\circ} \textsf{angle.}\)
\(\textsf{There are a few types of angles. Let's review them.}\)
\(\large\underline{\textsf{Types of Angles:}}\)
\(\textsf{Acute Angles are angles less than 90}^{\circ}.\)
\(\textsf{Right Angles are angles equal to 90}^{\circ}.\)
\(\textsf{Obtuse Angles are angles less than 180}^{\circ}, \ \textsf{but greater than 90}^{\circ}.\)
\(\textsf{Straight Angles are angles equal to 180}^{\circ}.\)
\(\textsf{Reflex Angles are angles greater than 180}^{\circ}, \ \textsf{but less than 360}^{\circ}.\)
\(\textsf{Because our angle is less than 90}^{\circ}, \ \textsf{this angle is Acute.}\)
The ratio of the number of cakes Ann had to the number of cakes Rose had was 5 : 2. After Ann sold 28cakes, the ratio became 3 : 4. How many cakes did each girl have at first?
Answers
The ratio of the number of cakes at first of 5 : 2 and the new ratio after Ann sold 28 cakes which is 3 : 4, indicates that Ann had 40 cakes while Rose had 16 cakes
What is a ratio in mathematics?A ratio between two quantities, indicates the number of times one quantity is contained in the other quantity.
The ratio of the number of cakes Ann had to the number of cakes Rose had = 5:2The number of cakes Ann sold that changed the ratio = 28 cakesThe new ratio of the number of cakes = 3 : 4Let X represent the initial number of cakes Ann had, and let Y represent the initial number of cakes Rose had, we get;
\(\dfrac{X}{Y} =\dfrac{5}{2}\)...(1)
\(\dfrac{X-28}{Y} =\dfrac{3}{4}\)...(2)
The expression in equation (1) indicates;
2 × X = 5 × Y
X = 5 × Y ÷ 2 = 2.5·Y
Therefore;
\(\dfrac{X-28}{Y} =\dfrac{3}{4}\)
We get;
\(\dfrac{2.5\cdot Y-28}{Y} =\dfrac{3}{4}\)
4 × (2.5·Y - 28) = 3 × Y
10·Y - 4 × 28 = 3·Y
10·Y - 3·Y = 4 × 28 = 112
7·Y = 112
Y = 112/7 = 16
Y = 16
The number of cakes Rose had first, Y = 16 cakesX = 2.5·Y
Therefore; X= 2.5 × 16 = 40
X = 40
The number of cakes Ann had first, X = 40 cakesLearn more about ratios here:
https://brainly.com/question/28392725
#SPJ1
a rectangle is inscribed with its base on the x-axis and its upper corners on the parabola 6-x^2 . what are the dimensions of such a rectangle with the greatest possible area?
Answers
The dimensions of the rectangle with the greatest possible area are 2\(\sqrt{(2)}\) by 4.
Let's denote the dimensions of the rectangle as length (L) and width (W). Since the base of the rectangle is on the x-axis, the width of the rectangle is equal to the x-coordinate of the upper corners of the rectangle.
Let's assume that the x-coordinate of the upper corners of the rectangle is x. Then, the width of the rectangle is W = 2x, and the length of the rectangle is L = 6 -\(x^2\).
The area of the rectangle is A = LW = \(2x(6 - x^2) = 12x - 2x^3.\)
To find the maximum area, we need to take the derivative of A with respect to x and set it equal to zero:
\(dA/dx = 12 - 6x^2 = 0\)
Solving for x, we get:
x = ±sqrt(2)
Since we are interested in the dimensions of the rectangle on the parabola, we take the positive value of x.
Therefore, the width of the rectangle is:
W = 2x = 2sqrt(2)
And the length of the rectangle is:
L = 6 - \(x^2\)= 6 - 2 = 4
So the dimensions of the rectangle with the greatest possible area are 2sqrt(2) by 4.
To learn more about dimensions visit: https://brainly.com/question/28688567
#SPJ11
3. Which explicit formula is equivalent to a₁ = 1, an = -6an-1?
○ an = -6(1)n-1
O an = 1 + (n − 1)6
O an = −6+ (n − 1)
O an = 1(-6)n-1
Answers
Answer:
an = -6(1)n-1.
Step-by-step explanation:
since a = 1
what is e * 6 - 4 * 1
Answers
Answer:
14 is the answer if you mean 3 by that e
Which ordered pair is a solution to the system of equations?
y=3x
Y= 2x +3

Answers
Answer:
(3, 9 )
Step-by-step explanation:
y = 3x → (1)
y = 2x + 3 → (2)
Substitute y = 3x into (2)
3x = 2x + 3 ( subtract 2x from both sides )
x = 3
Substitute x = 3 into (1)
y = 3 × 3 = 9
solution is (3, 9 )
Bookwork code: T89
Tomas travelled to his friend's house and his journey is shown on the distance-
time graph below.
He travelled the final 3 hours of his journey at a constant speed of 80 km/h.
Work out the value of d.
Answers
As he travelled the final 3 hours of his journey at a constant speed of 80 km/h, the value of d is 240 km.
What is the value of d in Tomas's journey?From the graph, Tomas traveled the final 3 hours of his journey at a constant speed of 80 km/h. We mut find the distance he traveled during this time period.
Since speed is defined as distance divided by time, we will use: Distance = speed × time.
Given data: Tomas traveled for 3 hours at a constant speed of 80 km/h,
Distance = Speed × Time
Distance = 80 km/h × 3 h
Distance = 240 km.
Read more about Distance
brainly.com/question/26550516
#SPJ1
PLEASE HELP LIKE RN, thank you so much

Answers
Answer:
Facts.
Step-by-step explanation:
what is the equation for the idealized regression line? label each part of the equation. what is the equation for the least-squares regression line? label each part of the equation. what are the four assumptions and conditions that must be met in order to perform inference for regression? explain how to check each one. what three aspects of the scatterplot affect the standard error of the regression slope? what is the formula for the standard error for the slope? what is the formula for the sampling distribution for regression slopes? show and explain formulas. if there is no association between two variables, what should be the value of the slope of the regression line?
Answers
The idealized regression line is y = β₀ + β₁x + ε, and the least-squares regression line is Y = b₀ + b₁x. Four assumptions for regression inference are linearity, independence, homoscedasticity, and normality. The standard error of the regression slope is affected by the spread, sample size, and relationship strength. The formula for the standard error of the slope is SEb₁ = √[ Σ(\(y_i - Y_i\))² / (n-2) ] / √[ Σ(\(x_i\) - X)² ]. If there is no association between two variables, the slope of the regression line should be zero.
There are two equations commonly used in regression analysis: the idealized regression line and the least-squares regression line.
The equation for the idealized regression line is
y = β₀ + β₁x + ε
where
y is the dependent variable
x is the independent variable
β₀ is the intercept
β₁ is the slope
ε is the error term
The equation for the least-squares regression line is
Y = b₀ + b₁x
where
Y is the predicted value of y
x is the independent variable
b₀ is the intercept
b₁ is the slope
There are four assumptions and conditions that must be met in order to perform inference for regression
The relationship between the independent variable and dependent variables is called Linearity . To check linearity, plot the dependent variable against the independent variable and look for a straight-line pattern.
Independence, The observations are independent of each other. To check independence, ensure that there is no relationship between the residuals (the difference between the observed value and the predicted value) and any other variables.
The constant variance of errors across independent variable of all level is called homoscedasticity. To check homoscedasticity, plot the residuals against the predicted values and look for a consistent spread of points around zero.
Normality, The errors are normally distributed. To check normality, plot a histogram of the residuals and look for a roughly bell-shaped distribution.
The formula for the standard error for the slope is
SEb₁ = √[ Σ(\(y_i\)- \(Y_i\))² / (n-2) ] / √[ Σ(\(x_i\) - X)² ]
where
\(y_i\), observed value of variable which is dependent for the ith observation
\(Y_i\) is the predicted value of the dependent variable for the ith observation
\(x_i\), the observed value of variable which is independent for the ith observation
X is the mean of the independent variable
n is the sample size
The formula for the sampling distribution for regression slopes is
b₁ ~ N(β₁, σ² / Σ(\(x_i\) - X)²)
where
b₁, least-squares regression line's slope
β₁ is the true population slope
σ² is the variance of the errors
If there is no association between two variables, the slope of the regression line should be zero. it depicts that the variable which is independent has no effect on the variable which is dependent.
To know more about regression line:
https://brainly.com/question/7656407
#SPJ4
Suppose △A′B′C′ is scalene. Which line segment in △A′B′C′ is the same length as line segment

Answers
Answer:
B
Step-by-step explanation:
A'C'
Find 3 consecutive odd integers with a sum of 63. PLEASE HELP!!!
Answers
Answer:
the numbers are 20, 21 and 22
Step-by-step explanation:
x+(x+1) + (x+2) = 63
3x +3 = 63
3x = 60
x = 20
doing this for ponits
Answer:
the numbers are 20, 21 and 22
Step-by-step explanation:
x+(x+1) + (x+2) = 63
3x +3 = 63
3x = 60
x = 20
Fill-in the missing pieces for the equation of the line that is perpendicular to y = 6x - 1 and passes through (-6, 4).
Answers
Therefore , the solution of the given problem of equation comes out to be y = (-1/6)x + 3.
Describe equation.A recovery model built on linearity is based on the equation y=mx+b. The inclination is B, and the y-intercept is m. The aforementioned statement is often referred to as "Bivariate linear formulas have two components," even though y and y are distinct parts. There are no simple answers when it arrives at using linear functions. and. The formula is Y=mx+b, where m represents slopes and b represents the y-intercept.
Here,
specified that the specified line has a slope of 6, the perpendicular line's slope is:
=> m = -1/6
We can now determine the equation of the vertical line using the point-slope form of the equation of a line:
=> y - y1 = m(x - x1)
where m is the slope we just discovered and (x1, y1) is the provided point on the line (-6, 4):
=> y - 4 = (-1/6)(x - (-6))
Simplifying:
=> y - 4 = (-1/6)(x + 6)
=> y - 4 = (-1/6)x - 1
=> y = (-1/6)x + 3
The line that passes through (-6, 4) and is orthogonal to y = 6x - 1 has the equation:
=> y = (-1/6)x + 3.
To know more about equation visit:
https://brainly.com/question/11897796
#SPJ1
A rectangular garden has a walkway around it. The area of the garden is 2(5.5x + 3.5). The combined area of the garden and the walkway is 2.5(8x + 6). Find the area of the walkway around the garden as the sum of two terms.
Answers
The total of the two terms that represent the length of the garden's walkway: 26x + 28
Explain the features of term rectangle?An enclosed 2-D shape called a rectangle has four sides, four corners, and four right angles (90°). A rectangle has equal and parallel opposite sides. A rectangle has two dimensions—length and width—because it is a two-dimensional form. The rectangle's longer side is its length, while its shorter side is its breadth.A walkway around a rectangular garden.
The garden's size is 4(4.5 x + 1.5) square meters.The garden's and the walkway's combined area is 5.5(8 x + 4).Simplifying each equation.
Area of the garden : 4(4.5 x + 1.5) = 18x + 6
Combined area: 5.5(8 x + 4) = 44x + 22
Thus,
Area of walkway = Combined area - Area of the garden
= 44x + 22 - 18x + 6
= 26x + 28
Thus, the total of the two terms that represent the length of the garden's walkway: 26x + 28
To know more about the rectangle, here
https://brainly.com/question/25292087
#SPJ1
suppose f : rn → rm is a linear map. what is the derivative of f ?
Answers
If f: rn → rm is a linear map, then its derivative is simply the map itself. This is because a linear map is a function that preserves vector addition and scalar multiplication.
In other words, if we take two vectors in the domain and add them together, and then apply the linear map, it is the same as applying the linear map to each vector separately and then adding the results. Similarly, if we multiply a vector in the domain by a scalar and then apply the linear map, it is the same as multiplying the result of applying the linear map to the original vector by the same scalar.
Formally, we can express this idea using the concept of a Jacobian matrix. The Jacobian matrix of a function describes the rate at which the function changes near a particular point. For a linear map, the Jacobian matrix is simply the matrix that represents the map. This means that the derivative of f is the matrix A such that f(x) = Ax for all x in rn.
To see why this makes sense, consider the simplest case of a linear map from R1 to R1, given by f(x) = ax, where a is a constant. The derivative of this function is f'(x) = a, which is just the constant coefficient of the linear map. More generally, the derivative of a linear map f: rn → rm is the matrix A such that f(x) = Ax for all x in rn.
To learn more about linear map, refer:-
https://brainly.com/question/29129296
#SPJ11
Chris deposited $1,000 into an account that earned 8% compound interest over 48 months. What is the account value earned after 48 months?
Answers
Answer:
She would have $1080 dollars in 48 months.
Step-by-step explanation:
So she earned 8% interest in 48 months.
So you find 8% of 1000 and that would be 80 and add 80 to 1000 because she gained 80 dollars in interest over 48 months so in 48 months she has $1080 dollars.
a is an nn matrix. determine whether the statement below is true or false. justify the answer. if a for some scalar , then is an eigenvector of a.
Answers
The statement is false. The existence of a scalar α such that αv is an eigenvector of a does not imply that v itself is an eigenvector of a.
What is matrix?A group of numbers built up in a rectangular array with rows and columns. The elements, or entries, of the matrix are the integers. In addition to numerous mathematical disciplines, matrices find extensive use in the fields of engineering, physics, economics, and statistics.
The statement is false. An eigenvector is a non-zero vector that, when multiplied by a matrix, produces a scalar multiple of itself. In other words, if v is an eigenvector of a matrix A, then Av = λv, where λ is the corresponding eigenvalue.
The statement suggests that if a is an nn matrix (presumably an n x n matrix), and a scalar α exists such that αv is an eigenvector of a, then v must also be an eigenvector of a. However, this is not necessarily true.
Let's consider a counterexample to demonstrate this. Suppose we have the 2x2 identity matrix I:
I = [[1, 0],
[0, 1]]
In this case, any non-zero vector v will satisfy the condition αv = v for α = 1. However, not all non-zero vectors v are eigenvectors of I. In fact, the only eigenvectors of I are [1, 0] and [0, 1] with corresponding eigenvalues of 1.
Therefore, the statement is false. The existence of a scalar α such that αv is an eigenvector of a does not imply that v itself is an eigenvector of a.
Learn more about matrix on:
https://brainly.com/question/30715889
#SPJ4
Identify which of the following statements are true or false:
Statement A: Hitting the bull's eye is reliability.
Statement B: Hitting the same spot again and again is validity.
Answers
Please help me with this homework with the correct answer

Answers
Answer:
You can do this! Everyone believes you can do it!
Step-by-step explanation:
When an alternating current of frequency f and peak current I_0 passes through a resistance R, then the power delivered to the resistance at time t seconds is P = I^2_0 R sin^2 2 pi ft. Write an expression for the power in terms of csc^2 2 pi ft. P = I^2_0 R/(csc^2 2 pi ft) P = I^2_0 R (csc^2 2 pi ft) P = I^2_0/(1 - csc^2 2 pi ft) P = I^2_0 R(1 - csc^2 2 pi ft)
Answers
The expression for the power delivered to a resistance in terms of csc^2 2 pi ft is P = I^2_0 R (csc^2 2 pi ft).
According to the given information, the power delivered to a resistance R when an alternating current of frequency f and peak current I_0 passes through it is represented by the equation P = I^2_0 R sin^2 2 pi ft.
To express this equation in terms of csc^2 2 pi ft, we can use the trigonometric identity csc^2 x = 1/sin^2 x. Substituting this identity into the equation, we get P = I^2_0 R (1/sin^2 2 pi ft).
Since csc^2 x is the reciprocal of sin^2 x, we can rewrite the equation as P = I^2_0 R (csc^2 2 pi ft). This expression represents the power delivered to the resistance in terms of csc^2 2 pi ft.
Therefore, the correct expression for the power delivered to the resistance in terms of csc^2 2 pi ft is P = I^2_0 R (csc^2 2 pi ft).
Learn more about resistance here:
https://brainly.com/question/33728800
#SPJ11
How do you solve the equation above using substitution? Please show work :)

Answers
Answer:
the solution is (21/2, 19/2)
Step-by-step explanation:
The first equation, x = y + 1, has already been solved for x. We can eliminate x in the second equatin by substituting y + 1 for x:
y = -(1/3)(y + 1) + 6
Let's eliminate fractions by multiplying all three terms by 3:
3y = y + 1 + 18
Combining like terms results in:
2y = 19, so that y = 19/2.
Substituting this 19/2 for y in the first equation, we get:
19/2 + 2/2 = x, or
x = 21/2
Thus the solution is (21/2, 19/2)
a gym knows that each member, on average, spends 70 minutes at the gym per week, with a standard deviation of 20 minutes. assume the amount of time each customer spends at the gym is normally distributed. a. what is the probability that a randomly selected customer spends less than 65 minutes at the gym? b. suppose the gym surveys a random sample of 49 members about the amount of time they spend at the gym each week. what are the expected value and standard deviation (standard error) of the sample mean of the time spent at the gym?
Answers
(a) The probability that a randomly selected customer spends less than 65 minutes at the gym is 0.4013. (b) The expected value and standard deviation (standard error) of the sample mean of the time spent at the gym is 2.857 minutes
a) The probability that a randomly selected customer spends less than 65 minutes at the gym is calculated using the standard normal distribution formula.
z = (x - μ) / σ
where,μ = 70 minutes, σ = 20 minutes, x = 65 minutes
Substituting the given values, we get
z = (65 - 70) / 20
z = -0.25
Using a standard normal table or calculator, the probability that a randomly selected customer spends less than 65 minutes at the gym is 0.4013.
b) The standard deviation (standard error) of the sample mean can be calculated using the formula:
SE = σ/√n
where,σ = 20 minutes, n = 49
Substituting the given values, we get
SE = 20/√49
SE = 2.857 minutes
Therefore, the expected value and standard deviation (standard error) of the sample mean of the time spent at the gym is 2.857 minutes, respectively.
Know more about Probability here :
https://brainly.com/question/24756209
#SPJ11
On Thursday it snowed 3 ½ inches in 4 hours. On Friday it snowed 8 inches in 9 hours. On which day was it snowing faster?
Answers
Answer:
Friday
Step-by-step explanation:
Thursday 7/2 ÷ 4 = 7/8 inches an hour (.875)
Friday 8 ÷ 9 = 8/9 inches an hour (.888)
Friday it was snowing faster
Answer:
Friday
if Thursday snowed 3½ inch 4 hours
then for Friday
9 ÷ 8 = 1.125 inches for one hour
1.125 × 4 = 4.5 inches for 4 hours
1.25 inches more than Thursday. So, the answer is Friday ^^
d)about 6.8 because 36<45.5<49
help pls imma give lots of pionts

Answers
Answer:
have a great day:)))))
Step-by-step explanation:
what is the probability that a randomly selected person would be a protestant and at the same time bea democrat or a republican?
Answers
The probability of picking a red ball is 2/3.
As given in the student question,
The probability that a randomly selected person would be a protestant and at the same time be a democrat or a republican is not given in the problem statement.
Hence, it cannot be determined without further information.
Probability is the branch of mathematics that deals with the study of uncertainty.
It provides a framework for analyzing random events that occur in the real world.
Probability helps us to estimate the likelihood of a particular event occurring. It helps us to make informed decisions when faced with uncertain outcomes.
It plays a critical role in many fields such as science, engineering, economics, and finance.
Probability formula:
The probability of an event is calculated by dividing the number of favorable outcomes by the total number of outcomes.
The formula for probability is given by:
P (A) = Number of favorable outcomes/ Total number of outcomes
where P (A) represents the probability of event A.
Example:
Suppose we have a bag containing 10 red balls and 5 blue balls.
The total number of outcomes = 10 + 5 = 15
The number of favorable outcomes = 10P (Red ball) = 10/15 = 2/3
Therefore, the probability of picking a red ball is 2/3.
For similar question on probability:
https://brainly.com/question/30034780
#SPJ11
What is the area of the irregular polygon shown below?

Answers
Answer:
86 sq units
Step-by-step explanation:
it is the because:
18*4=72
3.5*4/2=7
7+7+72=86 sq units
show that the equation x^3-15x+c=0 has at most one root in the interval parentheses -2, 2.
Answers
Therefore, the equation x^3 - 15x + c = 0 has at most one root in the interval (-2, 2).
To show that the equation x^3 - 15x + c = 0 has at most one root in the interval (-2, 2), we can use the concept of the Intermediate Value Theorem and Rolle's Theorem.
Let's assume that the equation has two distinct roots, denoted as a and b, in the interval (-2, 2). Without loss of generality, we assume a < b.
Since the function is continuous on the closed interval [-2, 2] and differentiable on the open interval (-2, 2), we can apply Rolle's Theorem. According to Rolle's Theorem, there exists a point c in the open interval (a, b) such that the derivative of the function at c is zero.
Consider the derivative of the function f(x) = x^3 - 15x + c:
f'(x) = 3x^2 - 15
Setting f'(c) = 0, we have:
3c^2 - 15 = 0
c^2 - 5 = 0
c^2 = 5
Taking the square root of both sides, we get:
c = ±√5
Now, let's consider the function values at the endpoints of the interval (-2, 2):
f(-2) = (-2)^3 - 15(-2) + c = -8 + 30 + c = 22 + c
f(2) = (2)^3 - 15(2) + c = 8 - 30 + c = -22 + c
If c = √5, then f(-2) = 22 + √5 and f(2) = -22 + √5.
If c = -√5, then f(-2) = 22 - √5 and f(2) = -22 - √5.
In either case, the function values at the endpoints have different signs. This implies that there exists at least one value, say k, in the interval (-2, 2) such that f(k) = 0, according to the Intermediate Value Theorem.
However, we assumed at the beginning that there are two distinct roots in the interval (-2, 2), denoted as a and b. This contradicts our finding that there is at most one root in the interval. Hence, our assumption of having two distinct roots is false.
To know more about equation,
https://brainly.com/question/27695523
#SPJ11
In the diagram below of AACD, DB is a median to AC, and AB = DB.
D
A
B
c
If mZDAB = 32°, what is mZBDC?
(1) 320
(3) 58°
(2) 520
(4) 64°

Answers
Answer:
it will be (3)
Step-by-step explanation:
since DB is a median
then DB= half AC , AB=BC
then AB=BD
then the two triangles are isosceles
The required m∠BDC is 32° .
In geometry, a median of a triangle is a line segment joining a vertex to the midpoint of the opposite side, thus bisecting that side. Every triangle has exactly three medians, one from each vertex, and they all intersect each other at the triangle's centroid.
According to the given question ;
DB is a median;
By the symmetry ;
Then DB = half AC ,
= AB = BC
Then= AB = BD
In a triangle, a line that connects one corner (or vertices ) to the middle point of the opposite side is called a median. A property of isosceles triangles, which is simple to prove using triangle congruence, is that in an isosceles triangle the median to the base is perpendicular to the base.
Then the two triangles are isosceles.
Means m∠DAB = m∠BDC = 32°
For the more information about the properties of isosceles triangle click the link given below.
https://brainly.in/question/13106654
NO LINKS!! Please help me with this exercise.

Answers
Answer:
20 ml of corn syrup and 180 ml of water make 10% solution-------------------------------
GivenThe volume of solution is 200 ml,Syrup content of solution is 10%.SolutionLet's assume the volume of syrup is x, then we set up equation:
x = 10% of 200x = 10*200 / 100x = 20 ml