3.) solve for h,
V= 1/3 bh
Answers
Answer:
\(h = \frac{3V}{b}\)
Step-by-step explanation:
Given the formula, \(V = \frac{1}{3}bh\):
Start with isolating b from the right-hand side of the equation by using the multiplicative inverse of b, which is \(\frac{1}{b}\). Multiply both sides of the equation by \((\frac{1}{b})\) :
\(V (\frac{1}{b}) = \frac{1}{3}bh (\frac{1}{b})\)
\(\frac{V}{b} = \frac{1}{3}h\)
Next, isolate h by using the multiplicative inverse of \(\frac{1}{3}\), which is \(\frac{3}{1}\). Multiply both sides of the equation by \((\frac{3}{1})\) :
\(\frac{V}{b} (\frac{3}{1}) = (\frac{3}{1}) \frac{1}{3}h\)
\(h = \frac{3V}{b}\)
Hope my explanation helps :)
Answer:
Step-by-step explanation:
To solve this equation for h, multiply both sides by 3. We get:
3V = bh.
Dividing this result by b results in
3V
h = ----------
b
Related Questions
what is the slope of the line that passes through the points (_5,0) and (-3,1)?
Answers
Step 2 : Define parameters
\(\begin{gathered} y_1=0 \\ y_2=1 \\ x_1=-5 \\ x_2=\text{ -3} \end{gathered}\)Step 3: Substitute the parameters into the equation
\(\begin{gathered} \text{slope}=\text{ }\frac{y_2-y_1}{x_2-x_1} \\ \text{slope}=\frac{1-0}{-3-(-5)} \\ \text{slope = }\frac{1}{-3+5}=\frac{1}{2} \\ \text{slope}=\text{ }\frac{1}{2}\text{ or 0.5} \end{gathered}\)what is equivalent to 4 - z - z - z - z
Answers
Answer: 4-4z
Step-by-step explanation: Coming the like terms in order to simplify the equation. There are four z's and they combine as they are like terms. Since we don't know the value of z, thats the most simplified form.
The area of a circle is 9л cm². What is the circumference, in centimeters?
Express your answer in terms of pi.
Answers
Answer: 6π cm
Step-by-step explanation:
The formula for the area of a circle is:
A = πr²
where A is the area and r is the radius.
Given that the area of the circle is 9π cm², we can solve for the radius as follows:
9π = πr²
Dividing both sides by π, we get:
r² = 9
Taking the square root of both sides, we get:
r = 3
Therefore, the radius of the circle is 3 cm.
The formula for the circumference of a circle is:
C = 2πr
Substituting the value of r, we get:
C = 2π(3) = 6π
Therefore, the circumference of the circle is 6π cm.
A coordinate plane. Use the coordinate plane to plot the points (6, 0) and (0, 5). Which statement is true? (0, 5) is located on the x-axis. (0, 5) is located at the origin. (6, 0) is located on the x-axis. (6, 0) is located at the origin.
Answers
Answer:
The correct option is (6, 0) is located on the x-axis.
Step-by-step explanation:
Given that we plot (6, 0) and (0, 5) on the xy–plane. We know that in (6, 0) 6 is the x-coordinate and 0 is the y-coordinate while in (0, 5) 0 is the x-coordinate and 5 is the y-coordinate.
Therefore, (6, 0) can be found on the x–axis not on the origin because it has shifted from 0 to 6 and (0, 5) can be found on the y-axis not on the origin because it has shifted from 0 to 5.
Hence, the correct option is (6, 0) is located on the x-axis.
Answer:
x-axis
Step-by-step explanation:
in a train yard there are tank cars, boxcars, and flatcars. how many ways can a train be made up consisting of tank cars, boxcars, and flatcars? (in this case, order is not important.)
Answers
There are 55 ways to form a train consisting of tank cars, boxcars, and flatcars by using concept of combinations.
If there are t tank cars, b boxcars, and f flatcars in the train yard, then the number of ways to form a train by selecting some of these cars is the same as the number of ways to distribute n = t + b + f identical objects into three distinct boxes, such that each box may receive any number of objects (including zero). The solution is given by the combination formula:
C(n + k - 1, k - 1)
where k is the number of boxes (in this case, k = 3). Therefore, the number of ways to form a train from t tank cars, b boxcars, and f flatcars is:
C(t + b + f + 3 - 1, 3 - 1) = C(t + b + f + 2, 2) = (t + b + f + 2)! / ((t + b + f)! * 2!)
There are 4 tank cars, 3 boxcars, and 2 flatcars in the train yard, then the number of ways to form a train is:
C(4 + 3 + 2 + 2, 2) = C(11, 2) = 55
Therefore, there are 55 ways to form a train consisting of tank cars, boxcars, and flatcars
To know more about concept of combinations.
https://brainly.com/question/23760736
#SPJ4
A coin is tossed 3 times. Find the probability of getting exactly two head
Answers
In this problem, we have a binomial probability distribution
so
n=3
p=0.50
q=0.50
Find out P(X=2)
\(P(X=2)=\frac{3!}{2!(3-2)!}*0.50^2*0.50^{(3-2)}\)P(X=2)=0.375Which is the value of this expression when a = 5 and k=-2?
*
32a-2
75
9
* 25
25
9
75
Answers
Answer: it’s A
Step-by-step explanation: I got it right, hope it helps ✨
The value of the expression \((\frac{3^{2}a^{-2} }{3a^{-1} } )^{k}\) when a = 5 and k = -2 is \(\frac{25}{9}\)
How to substitute and get the value of an expression?The expression is as follows:
\((\frac{3^{2}a^{-2} }{3a^{-1} } )^{k}\)
using law of indices
Hence,
when a = 5, k = -2
\((\frac{3^{2}a^{-2} }{3a^{-1} } )^{k} = (\frac{9(5^{-2} )}{3(5^{-1} )})^{-2}\)
Then,
\((\frac{9(5^{-2} )}{3(5^{-1} )})^{-2}=(\frac{\frac{9}{25} }{\frac{3}{5} } )^{-2}\)
\((\frac{\frac{9}{25} }{\frac{3}{5} } )^{-2}=(\frac{9}{25} (\frac{5}{3}))^{-2}\)
\((\frac{9}{25} (\frac{5}{3}))^{-2}=(\frac{3}{5})^{-2}\)
\((\frac{3}{5})^{-2}=\frac{25}{9}\)
Therefore, the value of the expression is 25 / 9
learn more on expression here:https://brainly.com/question/12879207
#SPJ5

can anyone answer these two questions? please :)


Answers
The one with color is 1,2,6
What is the unit rate if a student reads 81 pages in 3 hours?
Answers
Answer:
27 pages per hour
Step-by-step explanation:
81 pages divide by 3 hours. think of it as a fraction and divided 3 on both bottom and top then it gives you 27pages per hour as a fraction
Solve each matrix equation.
[-3 2 5 -1 ] = [4 5 -1 3] - (1/2)X
Answers
The solution matrix of the matrix equation \(\left[\begin{array}{ccc}-3&2\\5&-1\end{array}\right]\) = \(\left[\begin{array}{ccc}4&5\\-1&3\end{array}\right]\) - (1/2) X is given by X = \(\left[\begin{array}{ccc}14&6\\-12&8\end{array}\right]\).
Matrix is an array or arrangement of numbers.
Addition or subtraction of two matrices is given by the addition and subtraction of corresponding numeric value respectively.
Given the matrix equation is,
\(\left[\begin{array}{ccc}-3&2\\5&-1\end{array}\right]\) = \(\left[\begin{array}{ccc}4&5\\-1&3\end{array}\right]\) - (1/2) X
We have to find the matrix X.
Now,
(1/2) X = \(\left[\begin{array}{ccc}4&5\\-1&3\end{array}\right] -\left[\begin{array}{ccc}-3&2\\5&-1\end{array}\right]\) , arranging the matrices of the given equation in one side
(1/2) X = \(\left[\begin{array}{ccc}4-(-3)&5-2\\-1-5&3-(-1)\end{array}\right]\)
(1/2) X = \(\left[\begin{array}{ccc}4+3&3\\-6&3+1\end{array}\right]\)
(1/2) X = \(\left[\begin{array}{ccc}7&3\\-6&4\end{array}\right]\)
X = \(2\left[\begin{array}{ccc}7&3\\-6&4\end{array}\right]\) , multiplying 2 with both sides of the equation
X = \(\left[\begin{array}{ccc}14&6\\-12&8\end{array}\right]\)
Hence the solution matrix is given by X = \(\left[\begin{array}{ccc}14&6\\-12&8\end{array}\right]\).
To know more about matrix equation here
https://brainly.com/question/29132693
#SPJ4
In a class of 40 students, 10 are math majors. The teacher selects three students at random from this class. By assuming the independence, the probability that all three selected students are math majors is approximately
Answers
The approximate probability that all three selected students are math majors is 1/64.
To calculate the probability that all three selected students are math majors, we can use the concept of independent events.
Given that there are 10 math majors out of 40 students in total, the probability of selecting a math major on the first draw is 10/40 = 1/4. Since the events are assumed to be independent, the probability of selecting a math major on the second draw is also 1/4, and the same applies to the third draw.
To find the probability of all three events happening (i.e., all three selected students being math majors), we multiply the probabilities together:
P(all three selected students are math majors) = (1/4) * (1/4) * (1/4) = 1/64.
Therefore, the approximate probability that all three selected students are math majors is 1/64.
To know more about "Probability" refer here:
brainly.com/question/30034780#
#SPJ4
find m
how would i solve these? do i use the exterior angle theorem? pls help

Answers
Um maybe try it out then ask for help from the teacher
What property is shown by this number sentence?
52 + 0 = 52
Answers
Answer:
Addition Identity Property
Step-by-step explanation:
Addition Identity Property because a number + 0 = that number is Addition Identity Property.
What is the equation of the line that passes through the point (-4, 5) and has an
undefined slope?
Answers
Answer:
y = 5 or y = 0x + 5 (they're both the same)
Step-by-step explanation:
y = mx + b
y = 0x + b
5 = 0(-4) + b
5 = 0 + b
b = 5
y = 5 or y = 0x + 5 (they're both the same)
Step-by-step explanation:
an undefined slope is y/0.
not 0/x.
remember, the slope is the ratio (y coordinate change / x coordinate change) when going from one point on the line to another.
so, if x never changes, we get the undefined slope.
that means it is a vertical line through a point x on the x-axis, and it represents all points for which x = that constant value.
now, we look at our given point, its x-coordinate is -4.
so, the equation of the line is
x = -4
A small town has 35003500 inhabitants. At 8AM,2808AM,280 people have heard a rumor. By noon half the town has heard it. At what time will 9090% of the population have heard the rumor?(Do not round kk in your calculation. Round your final answer to one decimal place.)
Answers
It will take approximately 3.9 hours from noon for 90% of the population to hear the rumor, which means they will have heard it by approximately 3:00 PM.
By noon, half of the town's population, which is 3500/2 = 1750, has heard the rumor. This means that 1750 - 280 = 1470 people heard the rumor between 8AM and noon. Therefore, the number of people who have yet to hear the rumor is 3500 - 1750 = 1750.
To find out when 90% of the population will have heard the rumor, we can use the formula:
(people who have heard the rumor) / (total population) = 0.9
Solving for the number of people who need to hear the rumor, we get:
(0.9)(3500) = 3150
To find out how many people need to hear the rumor from noon onwards, we subtract the number of people who have already heard it:
3150 - 1750 = 1400
To find out how long it will take for 1400 more people to hear the rumor, we can use the ratio:
(number of people who hear the rumor per hour) / (total population) = (1400 / 1750)
Solving for the number of people who hear the rumor per hour, we get:
(number of people who hear the rumor per hour) = (1400 / 1750) * (1 / k)
where k is the number of hours it takes for 90% of the population to hear the rumor. Solving for k, we get:
k = (1400 / 1750) * (1 / (number of people who hear the rumor per hour))
Plugging in the value of 280 for the number of people who heard the rumor from 8AM to noon, we get:
\(k = (1400 / 1750) * (1 / ((1470 + 280) / 4))\)
k = 3.86
Therefore, it will take approximately 3.9 hours from noon for 90% of the population to hear the rumor, which means they will have heard it by approximately 3:00 PM.
For more questions like Percentage visit the link below:
https://brainly.com/question/30077132
#SPJ11
Which values are solutions to the inequality below? Check all that apply.
√x <10
A. 100
B. 25
C. -100
D. 105
E. 36
F. 9
Answers
Answer:
The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²vvvThe diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²The diagram shows measures of some of the line segments in APQR. 4.5 /62.58° Q Length of QS P Area of APQR S ㅏ Use the given information to find the measures of the unknown angles and segment shown below. Round your answers to the nearest whole number. Length of PS units 6.4 -5.0- units R units²v
Step-by-step explanation:
its E and A
Answer:
The solutions to the inequality are B, E, and F: 25, 36, and 9.
Step-by-step explanation:
The solutions to the inequality √x < 10 are the values of x that make the inequality true when plugged in. To find these values, we can square both sides of the inequality:
√x < 10
x < 10^2 = 100
So, the values of x that make the inequality true are those that are less than 100. The options that satisfy this condition are:
A. 100 (not a solution)
B. 25 (solution)
C. -100 (not a solution)
D. 105 (not a solution)
E. 36 (solution)
F. 9 (solution)
you randomly choose one shape from the bag. find the number of ways the event can occur. find the favorable outcomes of the event

Answers
(a) The number of ways that the event can occur is 6.
(b) Probabilities are :
1) 1/2, 2) 1/6 and 3) 1/3.
(a) Given a bag of different shapes.
Total number of shapes = 6
So, if we select one shape from random,
total number of ways that the event can occur = 6
(b) Number of squares in the bag = 3
Probability of choosing a square = 3/6 = 1/2
Number of circles in the bag = 1
Probability of choosing a circle = 1/6
Number of stars in the bag = 2
Probability of choosing a star = 2/6 = 1/3
Hence the required probabilities are found.
Learn more about Probability here :
https://brainly.com/question/14210034
#SPJ1
Find the two solutions to the following equation:
x(x + 10) = 0
Answers
If (x + 10) = 0, then we can rearrange the equation to solve for x: x = -10. Thus, x = -10 is also a solution to the equation.
Therefore, the two solutions to the equation x(x + 10) = 0 are x = 0 and x = -10.
Answer:
The correct answer is (0) and (-10)
Step-by-step explanation:
The standard form of this equation is (x-a)(x-b)=0
so, here given equation is : x(x+10)=0
For solving the equation, equate both the LHS parts equals to RHS i.e. :
x(x+10)=0
x=0 & (x+10)=0 or x=(-10)
Can someone tell me the slope ?

Answers
Answer:
slope = 4
Step-by-step explanation:
We can find the slope of a line when knowing 2 points by using the slope formula, \(\frac{y_2 - y_1}{x_2 - x_1}\). \(x_1\) and \(y_1\) represent the x and y values of the first point, respectively. \(x_2\) and \(y_2\) represent the x and y values of the second point, respectively.
The two points we have are (2,8) and (0,0). So, in this case, \(x_1\) = 2, \(y_1\) = 8, \(x_2\) = 0, and \(y_2\) = 0. Let's substitute those values into the formula and solve to get the slope:
\(m =\) \(\frac{0-8}{0-2}\)
\(\frac{-8}{-2}\)
\(4\)
So, the slope is 4.
A pizza parlor offers 15 different specialty pizzas. If the Almeida family wants to order 3 specialty pizzas from the menu, which method could be used to calculate the number of possibilities? 15!
3!
15!
12!
15!
12!3!
15!
Answers
To calculate the number of possibilities for the Almeida family ordering 3 specialty pizzas from the menu of 15 different options, the appropriate method to use is the combination formula.
The combination formula calculates the number of ways to choose a subset of items from a larger set without considering the order in which they are chosen. In this case, the Almeida family wants to order 3 pizzas out of 15 options, and the order in which they choose the pizzas does not matter.
The formula for combinations is given by:
C(n, r) = n! / (r! * (n - r)!)
where n is the total number of options, and r is the number of choices.
Therefore, the calculation for the number of possibilities for the Almeida family can be done using the combination formula as:
=C(15, 3) = 15! / (3! * (15 - 3)!)
= (15 * 14 * 13 * 12!) / (3! * 12!)
= (15 * 14 * 13) / (3 * 2 * 1)
= 455
the number of possibilities for the Almeida family is 455.
Learn more about possibilities here:
https://brainly.com/question/29086517
#SPJ11
What is the a total area of the figure

Answers
Answer:
125cm
Step-by-step explanation:
To find out the width (right side) you do the area ÷ length. 100÷100= 1
To find out how much is sticking out the rectangle, you do 150-100=50. And for area of a triangle you do the length (50) x width (1) ÷ 2 = 25
100+25=125
PLEASE HELP!!!!!! In which week was the rainfall in Portland the highest and how much fell?
weekly rainfall bar graph
Week 3, 800 cm
Week 4, 1400 cm
Week 4, 1200 cm
Week 3 900 cm

Answers
Answer:
Week 4, 1400
Brainliest plz
Step-by-step explanation:
Answer:
Week 3 and 900 cm
Step-by-step explanation:
Brainliest Please!
What is p(8 or less than 7) write answer as a whole number
Answers
The expression "p(8 or less than 7)" suggests that we are looking for the probability of an event that satisfies one of two conditions: either the outcome is 8 or the outcome is less than 7. However, we need to know the specific probability distribution or situation in which this function p is being used to calculate a meaningful answer.
For example, if p represents the probability of rolling a certain number on a fair six-sided die, then p(8 or less than 7) would be 0, because 8 is not a possible outcome and all outcomes less than 7 are equally likely. However, if p represents the probability of a certain event occurring in a particular situation, then we would need more information about the nature of the event to calculate the probability.
Find out more about the probability distribution
brainly.com/question/14210034
#SPJ4
Flyer Co. buys back 5102 shares of their $3 par value common stock for $17 per share. The amount debited to the treasury stock account to record the purchase would be $ ___. Enter zero if the treasury stock account should be credited. Show whole numbers only, no commas, decimals, etc. Mark for Review What's This?
Answers
If Flyer Co. buys back 5102 shares of their $3 par value common stock for $17 per share. The amount debited to the treasury stock account to record the purchase would be $ 86,834.
To calculate the amount debited to the treasury stock account, we need to multiply the number of shares bought back by the price per share.
In this case, Flyer Co. buys back 5102 shares at $17 per share.
The amount debited to the treasury stock account is calculated as follows:
Amount = Number of shares bought back * Price per share
Amount = 5102 * $17
Amount = $86,834
Therefore, the amount debited to the treasury stock account to record the purchase would be $86,834.
To learn more about stock click on,
https://brainly.com/question/15712198
#SPJ4
Compare the dimensions of the prisms. How many times greater is the surface area of the red prism than the surface area of the blue prism?

Answers
Answer:
9
Step-by-step explanation:
12 m / 4 m = 3
6 m / 2 m = 3
9 m / 3 m = 3
The scale factor from the small prism to the large prism is 3.
The ratio of the areas is the square of the scale factor.
3² = 9
Answer: 9
Answer: 9
Explanation: The surface area of the blue prism is 52, and the red prism is 468, if we divide 468 by 52, we get 9, so the red prism is 9 times greater than the blue prism's surface area.
(c) Given the set T:= {(x, y) = R²: |ry| ≤ 1}. Is T a compact set? Show your working. If you say it is not compact, then find the smallest compact set containing T. (d) Given a metric spaceX, p, and two compact subsets S, TE X. Prove that SUT is compact.
Answers
(c) To determine if the set T := {(x, y) ∈ R²: |ry| ≤ 1} is compact, we need to analyze its properties.
A set is considered compact if it is closed and bounded.
Boundedness: Let's consider the set T. We observe that for any point (x, y) in T, the absolute value of ry is less than or equal to 1. This means that |ry| ≤ 1. Since |ry| is bounded by 1 for all points in T, T is bounded.
Closedness: To check if T is closed, we need to examine its complement. The complement of T, denoted as T^c, consists of all points in R² that are not in T. In this case, T^c is the set of points (x, y) ∈ R² for which |ry| > 1.
Now, let's consider the point (x, y) ∈ T^c such that |ry| > 1. We can construct an open ball centered at (x, y) with radius r = |ry| - 1. Any point within this ball will also have |ry| > 1, and therefore, will not belong to T. This implies that T^c contains all its limit points, making it closed.
Since T is bounded and its complement T^c is closed, we can conclude that T is a compact set.
The set T := {(x, y) ∈ R²: |ry| ≤ 1} is a compact set.
(d) To prove that the union of two compact sets S and T, i.e., S ∪ T, is also compact, we need to show that it is closed and bounded.
Boundedness: Since S and T are compact, they are both bounded sets. Let's consider the union of S and T, denoted as S ∪ T. Any point in S ∪ T will belong to either S or T. Since both S and T are bounded, their union S ∪ T is also bounded.
Closedness: Let's consider the complement of S ∪ T, denoted as (S ∪ T)^c. We know that S and T are both compact, which implies that their complements S^c and T^c are closed sets. Since the complement of a union is the intersection of complements, we have:
(S ∪ T)^c = S^c ∩ T^c
Since both S^c and T^c are closed sets, their intersection S^c ∩ T^c is also closed. Therefore, the complement (S ∪ T)^c is closed, which implies that S ∪ T is a compact set.
Conclusion: The union of two compact sets S and T, i.e., S ∪ T, is a compact set.
To know more about open ball centered, visit
https://brainly.com/question/32645201
#SPJ11
PLEASE HELP THIS IS THE LAST QUESTION I HAVE TO DO!!!!!! YOU WILL GET 25 POINTS AND FIRST TO ANSWER WILL GET BRAINLIEST!!!
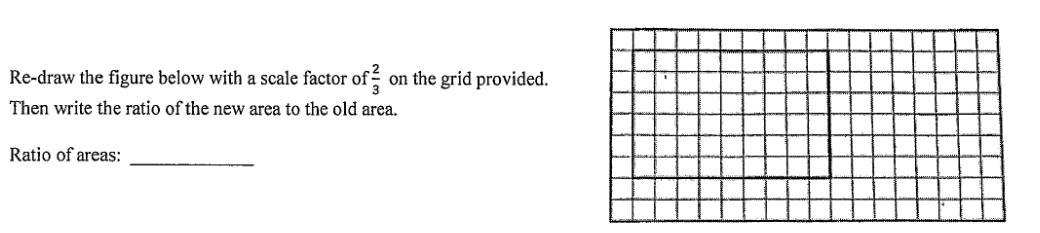
Answers
Answer:
can you give a little better of an explanation
Step-by-step explanation:
Find the quotient. 514÷15 A. 720 B. 7834 C. 267 D. 314
Answers
Answer:314
Step-by-step explanation:
2-2 webassign: problems and exercisesFind the absolute maximum and absolute minimum values of f on the given interval.f(x) = 6x^3 − 9x^2 − 216x + 9, [−4, 5]absolute minimum absolute maximum
Answers
The absolute maximum value is 412 and the absolute minimum value is -617.
A function's absolute maximum point is the location at which it can reach its highest value. A similar absolute lowest point is where the function's maximum value is obtained.
The absolute maximum and minimum values of f(x) = 6x^3 − 9x^2 − 216x + 9 on the interval [-4, 5] can be found using the First and Second Derivative Tests.
First, find the critical points of f(x) by setting its first derivative equal to zero and solving for x:
f'(x) = 18x^2 - 18x - 216 = 0
Using the quadratic formula, we get x = 4,-3.
Next, use the second derivative test to determine if the critical points are maxima or minima:
f''(x) = 36x - 18
At x = 4, f''(x) > 0, so x = 6 is a local minimum.
At x = -3, f''(x) < 0, so x = -9 is a local maximum.
Finally, check the endpoints of the interval to see if they contain the absolute maximum or minimum:
At x = -4, f(-4) = 6(-4)^3 - 9(-4)^2 - 216(-4) + 9 = 345
At x = 5, f(5) = 6(5)^3 - 9(5)^2 - 216(5) + 9 = -546
At x= 4, f(4) = -617
At x= -3, f(-3) = 412
Therefore, the absolute maximum value is 412 and the absolute minimum value is -617.
To know more about absolute maximum refer to:
brainly.com/question/29030328
#SPJ4
Your school organizes a clothing drive as a fundraiser for your class trip. The school earns $100 for every 400 pounds of donation clothing. How much money does your school earn for donating 2200 pounds of clothing?
Answers
Answer:
$550
Step-by-step:
2,200 minus 400 = 1,800. $100
1,800 minus 400 = 1,400. $100
1,400 minus 400 = 1,000. $100
1,000 minus 400 = 600. $100
600 minus 400 = 200. $100
Now here's the simple but tricky part.
400 hundred doesn't go into 200 right? Now every 400 equals $100 so you're gonna divide both numbers by 2.
400 divided by 2 = 200
100 divided by 2 = 50.
Now add up:
100+
100+
100+
100+
100+
50+
= 550.
Still have trouble understanding, I'll try and put it out simpler.
Hope that helps.
Your school earns $550 for donating 2200 pounds of clothing.
What is the unitary method?The unitary method is a method for solving a problem by the first value of a single unit and then finding the value by multiplying the single value.
Given that the school earns $100 for every 400 pounds of donated clothing.
2,200 - 400 = 1,800.
1,800 - 400 = 1,400.
1,400 - 400 = 1,000.
1,000 - 400 = 600.
600 - 400 = 200.
Now we know that every 400 equals $100 so we are gonna divide both numbers by 2.
400 divided by 2 = 200
100 divided by 2 = 50.
Now add up:
100+ 100+100+100+100+ 50 = 550.
Thus, Your school earns $550 for donating 2200 pounds of clothing.
To learn more about the unitary method, please visit the link given below;
https://brainly.com/question/23423168
#SPJ2