Answers
Answer:
\(10\frac{11}{20}\)
Step-by-step explanation:
\(5\frac{1}{2} + 3\frac{4}{5} + 1\frac{1}{4}\)
1.Find the LCM(Least Common Multiple).
That would be 20.
2.Multiply so all denominators are equal.
\(5\frac{10}{20} + 3\frac{16}{20} + 1\frac{5}{20}\)
3. Add
\(9\frac{31}{20}\)
4. Simplify
\(10\frac{11}{20}\)
Related Questions
factor the trinomial below. x^2+13x+42
Answers
Answer:
(x + 6)(x + 7)
Step-by-step explanation:
To factor the trinomial x^2 + 13x + 42, we need to find two numbers that multiply to 42 and add up to 13.
One way to do this is to list all the pairs of factors of 42 and see which pair adds up to 13:
1, 42 -> 1 + 42 = 43
2, 21 -> 2 + 21 = 23
3, 14 -> 3 + 14 = 17
6, 7 -> 6 + 7 = 13
So the pair of factors that we want is 6 and 7. We can use these numbers to rewrite the middle term of the trinomial:
x^2 + 13x + 42 = x^2 + 6x + 7x + 42
Next, we can group the first two terms and the last two terms:
x^2 + 6x + 7x + 42 = (x^2 + 6x) + (7x + 42)
Now, we can factor out the greatest common factor from each group:
x(x + 6) + 7(x + 6)
Notice that we have a common factor of (x + 6) in both terms. We can factor this out:
(x + 6)(x + 7)
40 POINTS I cant find the missing side

Answers
Answer:
Below
Step-by-step explanation:
See image below....do you see how you can use the Pythagorean Theorem to find the length of the missing side?
(missing side)^2 = 32^2 + 24^2
then you can add all of the sides to find the perimeter....yah?

if p(x)=3x^2+5x-8 then find p(-2)
Answers
P(x) = P(-2)
P(-2) = 3(-2)^2 + 5(-2)-8
= 3(4) + (-10) - 8
= 12 + (-10 -8)
= 12 + (-18)
= 12 - 18
= - 6
P(-2) = -6
(divided on both sides by -2)
Answer:
P = 3
(btw i am not sure if this is the answer you are looking for. Please clarify ths objective of this equation. What are we looking for? Are we solving for "P" ?)
I need help with this question please ASAP before tomorrow!
Thank you :)

Answers
Answer:
23 females are right handed and 9 are left handed
Step-by-step explanation:
So to get this answer :
18 OF THE 41 RIGHT HANDED EMPLOYEES ARE MALE.
The statement means that there are 41 right handed employees and 18 of those are male thus the remaining are FEMALE.
To find the number of right handed employees who are female just subtracted 18 from 41 ( becaus etotal number of right handed males is 18 and the total number of right handed employees is 41.)
SO 23 FEMALES IN THE COMPANY ARE RIGHT HANDED.
Because 23 females are right handed that means to get the number of left handed females you subtract the total number of right handed females from the total number of females in the company THUS:
32 - 23 = 9 LEFT HANDED FEMALES
HOPE IT HELPED
A rectangular carpet measures 10 m by 6 m
Part of the carpet is covered by a square rug of length 2m
What fraction of the carpet is
covered by the rug?
Give your answer in its simplest form.
Optional working
Answer:
Answers
Answer:
Step-by-step explanation:
A rectangular carpet measures 8 m by 6 mPart of the carpet is covered by a square rug of length 2 m
Please help i need this soon

Answers
Answer
180mm2
Step-by-step explanation:
Area of ceb=(15*6)/2=45
Area of aecd=15*9=135
Adding both
Area of shaded =45+135=180
No explanation needed
a random sample of 22 people, the mean commute time to work was 34.2 minutes and the standard deviation was 7.1 minutes. assume the population is normally distributed and use a t-distribution to construct a 95% confidence interval for the population mean . what is the margin of error of y? interpret the results.
Answers
We're 95% confident that the true population mean is between 31.361 and 37.039 minutes, with a margin of error of 2.839 minutes.
To find the t-value from the t-distribution table, we need to know the degrees of freedom. Since we have a sample size of 22, the degrees of freedom are 22-1=21. Looking up the t-value with 21 degrees of freedom and a 95% confidence level, we get 2.080.
Plugging in this value, we get:
CI = 34.2 ± 2.080*(7.1/√22)
CI = 34.2 ± 2.839
Therefore, the 95% confidence interval for the population mean commute time to work is (31.361, 37.039). This means that if we were to take many random samples of size 22 from the population and construct a 95% confidence interval for each sample, 95% of those intervals would contain the true population mean.
The margin of error of y is the amount added and subtracted from the sample mean to get the upper and lower bounds of the confidence interval. In this case, the margin of error is 2.839 minutes.
This means that we're 95% confident that the true population mean commute time to work is between 31.361 and 37.039 minutes, with a margin of error of 2.839 minutes.
To know more about confidence interval here
https://brainly.com/question/24131141
#SPJ4
2. What is the variable in this expression? 4y-5
Answers
Answer:
y
Step-by-step explanation:
A variable is a letter that holds the place of a number. y in this expression matches that description, so it is the variable.
How do you find the equation of a line with a slope passing through a point?
Answers
The equation of a straight line passing through the given point and slope is y - y1 = m(x - x1).
What is the simple definition of slope?
The slope of a line is a measure of its steepness. Mathematically, slope is calculated as "rise over run" (change in y divided by change in x).
Let the given point be (x1,y1).
slope be m.
we know that,
Line equation passing through point is:
y - y1 = m(x - x1)
where m = slope and (x1,y1) = given point.
Therefore The equation of a straight line passing through the given point and slope is y - y1 = m(x - x1).
Learn more about the equation here:
brainly.com/question/10724260
#SPJ4
f(x) = 4x2 + 5x - 3
g(x) = 4x2 – 3x2 + 5
Find (f +g)(x).
Answers
Answer:
5x + 12
Step-by-step explanation:
simplify f(x) and g(x)
g(x) = 4*2 -3*2 +5
g(x) = 8 -6 + 5
g(x) = 7
and
f(x) = 4*2 + 5x -3
f(x) = 8 + 5x - 3
f(x) = 5x + 5
So (f + g)(x) = f(x) + g(x)
(f + g)(x) = (5x + 5) + (7)
(f + g)(x) = 5x + 12
A school cafeteria is considering new menu options. The manager puts a comment box in the cafeteria where students can anonymously submit their choices. Is this a representative sample? Why or why not?
Answers
Answer:
A representative sample is a subset of a population that seeks to accurately reflect the characteristics of the larger group that is unbiased. So yes this is a representative sample since it is being submitted anonymously
Step-by-step explanation:
A gardener wants to divide a square piece of lawn in half diagonally. What is the length of the diagonal if the side of the square is ? Leave your answer in simplest radical form.Viruses
a. 16√8
b. 2√8
c. 8√8
d. 4
Answers
The correct response is c. 8√2 . The length of the diagonal if the side of the square is 8√2.
A polygon's opposed vertices (or corners) are connected by a line segment known as a diagonal. Or to put it another way, a diagonal is a line segment joining two polygonal vertices that are not neighbouring. With the exception of the figure's edges, it connects a polygon's vertices. For instance, a diagonal line or movement runs slopingly from one corner of a square to the other corner. a type of straight line called a diagonal. Straight up, down, or across are not possible on a diagonal line. It is a line that joins the corners of a form at two points. Instead of running straight up or across, a diagonal is formed by a straight line that is positioned at an angle.
Right isosceles triangles with both legs 8 feet long would make up each half.
The hypotenuse that both of those triangles would share is the diagonal.
We must determine the hypotenuse's length, d, in feet.
According to the Pythagorean theorem, the lengths of the legs' squares sum up to the length of the hypotenuse's square, so
\(8^{2} +8^{2} =8\sqrt{2}\)
To know more about diagonal here
https://brainly.com/question/25740584
#SPJ4
A gardener wants to divide a square piece of lawn in half diagonally. What is the length of the diagonal if the side of the square is 8ft? Leave your answer in simplest radical form.
a. 16√8
b. 2√8
c. 8√2
d. 4
$22.00+$14.00=
answer this
Answers
Answer:
$36
Step-by-step explanation:
22+14=36
a line passes through both points (-2, 2) and (8, 9). what is the angle between the line and the x axis?
Answers
Step-by-step explanation:
To find the angle between a line and the x-axis, we need to calculate the slope of the line first. The slope of a line passing through two points (x₁, y₁) and (x₂, y₂) is given by the formula:
m = (y₂ - y₁) / (x₂ - x₁)
Using the points (-2, 2) and (8, 9), we can substitute the values into the formula:
m = (9 - 2) / (8 - (-2))
= 7 / 10
= 0.7
The slope of the line is 0.7. The angle between the line and the x-axis can be found using the inverse tangent (arctan) function. The tangent of an angle is equal to the slope of the line, so we can write:
tan(θ) = 0.7
Taking the inverse tangent of both sides, we find:
θ = arctan(0.7)
Using a calculator, we can evaluate this to be approximately:
θ ≈ 35.87 degrees
Therefore, the angle between the line and the x-axis is approximately 35.87 degrees.
What shape is figure a?
Figure A is a cone and figure B is a cylinder.
cylinder
sphere
cone
pyramid
Mark this and return
Answers
In the given figure, shape A is cone and shape B is cylinder. Therefore, option C is the correct answer.
A cone is a three-dimensional shape with a flat base (typically circular) that gradually narrows to a point at the top, called the apex or vertex. It is formed by a set of lines or half-lines extending from the apex to all points lying on a plane that does not contain the apex.
A cylinder is a three-dimensional figure that is made up of two parallel flat surfaces connected by a curved side. The two bases are typically circular, but can also be any other shape, such as an ellipse. The two bases are usually at different heights and the curved surface is made up of straight side walls connecting the two bases. Cylinders can be thought of as a circular tube that has been cut in half and then the cut edges pushed together.
Therefore, option C is the correct answer.
To learn more about the volume of a cone visit:
https://brainly.com/question/1984638.
#SPJ1

2-3. The triangles below are drawn to scale and congruent sides and angles are indicated with tick marks.
Are the triangles congruent? If so, write a congruence statement and
explain how you know they are congruent.
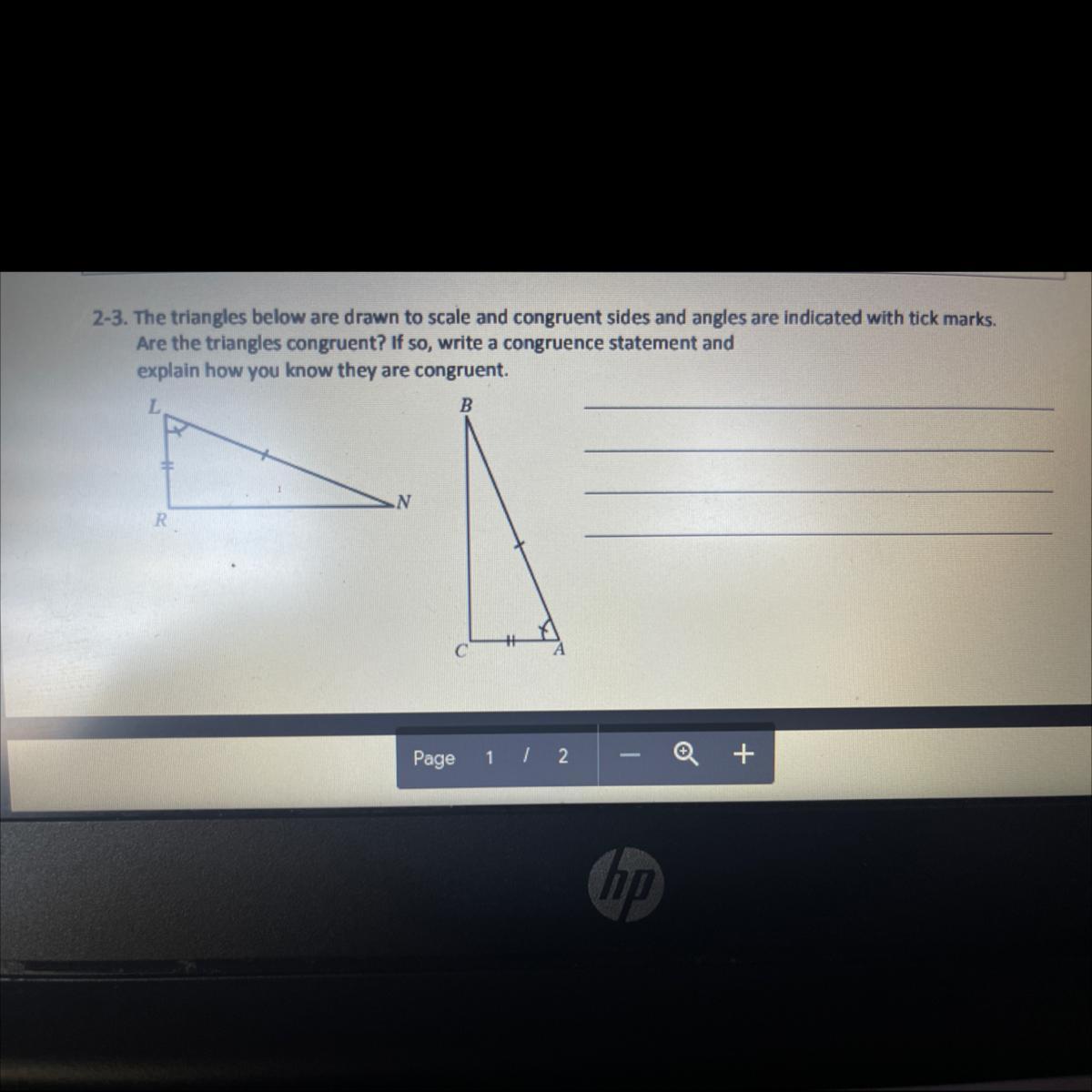
Answers
Answer: The triangles are congruent by SAS(side-angle-side)
Step-by-step explanation:
The triangles are congruent because 2 corresponding sides and one corresponding angle are given as congruent in an order respectable as a congruency statement(the SAS part).
the administration team compiled test results for those who had been tested for strep throat in a random sample of 400 sick patients who had been tested. the following relative frequency table shows the data. positive negative total has the flu 54% 6% 60% does not have the flu 8% 32% 40% total 62% 38% 100% based on the data, what is the ratio of false positives to true positives? 6 over 32 8 over 6 8 over 54
Answers
The ratio of false positives to true positives is 8 over 54.
According to the Question
Results of a strep throat test performed on a sample of 400 ill people are displayed in the table's data. People who have the flu and those who don't are separated into two categories. The patients are then separated into groups based on whether they tested positive or negative for strep throat within each of these categories.
We must first define what a true positive and a false positive are in order to calculate the ratio of false positives to true positives. A patient who tests positive for strep throat but does not actually have the illness is said to have a false positive. The 8% of patients in this instance who tested positive for strep throat but did not have the flu would fall under that category. A patient who tests positive for strep throat and truly has the illness is said to have a true positive. That would be the 54% of patients who tested positive for both the flu and strep throat in this instance.
We divide the percentage of false positives (8%) by the percentage of true positives (54%), which gives us the ratio of false positives to true positives.
As a result, the ratio becomes 8% / 54%, or 8/54.
It's critical to remember that this ratio is dependent on the sample and test performed and may not be the same for different populations or testing methodologies.
To know more about proportion and ratio on brainly :
brainly.com/question/26974513
#SPJ4
A container holds 4800 cubic inches of waste. The dimensions of the container are tripled. How many cubic feet
of waste does the new container hold?
Answers
This is a 3-D container and the dimensional factor is F=3. The volume increases by a factor of 33 = 27.
The answer is 4608 x 27 / 123 = 72
suppose the family wise error rate is 0.05, if there are ten levels of one factor but only 7 comparisons are of importance, what would the individual error rate need to be for each of those seven comparisons? group of answer choices 7 0.070 0.007 .7
Answers
The individual error rate for each of the 7 comparisons should be approximately 0.007 to maintain a family-wise error rate of 0.05. Option C is correct.
If there are 10 levels of one factor but only 7 comparisons are of importance, the total number of pairwise comparisons is given by the formula:
nC2 = (10 choose 2) = 45
Since only 7 of these comparisons are of importance, we need to adjust the individual error rate for multiple comparisons to maintain the overall family-wise error rate at 0.05.
Let α be the individual error rate for each of the 7 comparisons. Then, using the Bonferroni correction, the adjusted individual error rate for each comparison would be:
α' = α / m
where m is the number of comparisons of interest, which in this case is 7.
We want the family-wise error rate to be 0.05, so we have:
1 - (1 - α')^m = 0.05
Substituting α' = α / m, we get:
1 - (1 - α/m)^7 = 0.05
Solving for α, we get:
α ≈ 0.007
Option C is correct.
To know more about error, here
https://brainly.com/question/29417975
#SPJ4
we wish to construct a rectangular auditorium with a stage shaped as a semicircle of radius $r$, as shown in the diagram below (white is the stage and green is the seating area). for safety reasons, light strips must be placed on the perimeter of the seating area. if we have $45\pi 60$ meters of light strips, what should $r$ be so that the seating area is maximized?
Answers
To maximize the seating area while using 45π + 60 meters of light strips, the radius of the semicircular stage should be approximately 29π/3 - 5 meters.
To maximize the seating area, we need to determine the dimensions of the rectangular auditorium that will give us the largest possible area while using the given length of light strips.
Let the length of the rectangular auditorium be L, and its width be W.
The seating area consists of the rectangular portion minus the semicircular stage. So, the seating area's length is L - 2r (subtracting the semicircle's diameter) and the seating area's width is W - 2r.
The perimeter of the seating area is the sum of the lengths of its four sides, excluding the semicircular stage. The perimeter is given as 45π + 60 meters.
Perimeter = 2(L - 2r) + 2(W - 2r) + πr = 45π + 60
Simplifying: 2L + 2W - 8r + πr = 45π + 60
Rearranging: 2L + 2W = 8r + 44π + 60
The area of the seating area is given by A = (L - 2r)(W - 2r).
We want to maximize A, so we need to express it in terms of a single variable. Since we have an equation with two variables (L and W), we can rewrite one of the variables in terms of the other.
Rearranging the perimeter equation: 2L + 2W = 8r + 44π + 60
Solving for L: L = (8r + 44π + 60 - 2W) / 2
Substituting L in terms of W into the area equation: A = [(8r + 44π + 60 - 2W) / 2 - 2r] (W - 2r)
Simplifying: A = (4r + 22π + 30 - W) (W - 2r)
Now we have the area equation in terms of a single variable, W. To maximize A, we can take the derivative of A with respect to W, set it equal to zero, and solve for W.
dA/dW = 2(4r + 22π + 30 - W) - (W - 2r) = 0
Solving for W: 8r + 44π + 60 - W = W - 2r
Simplifying: 10r + 44π + 60 = 2W
W = 5r + 22π + 30
Now that we have W in terms of r, we can substitute this expression back into the area equation to get the area in terms of r only.
A = (4r + 22π + 30 - (5r + 22π + 30)) ((5r + 22π + 30) - 2r)
Simplifying: A = (r - 22π) (3r + 22π + 30)
Expanding: A = 3r² + 8rπ + 30r - 66πr - 660π
Now, to find the maximum area, we can take the derivative of A with respect to r, set it equal to zero, and solve for r.
dA/dr = 6r + 8π + 30 - 66π = 0
Simplifying: 6r - 58π + 30 = 0
6r = 58π - 30
r = (58π - 30) / 6
r ≈ 29π/3 - 5
Therefore, to maximize the seating area while using 45π + 60 meters of light strips, the radius of the semicircular stage should be approximately 29π/3 - 5 meters.
Learn more about maximum area click;
https://brainly.com/question/29635164
#SPJ12
two eggs and two pieces of bacon have a total of 22 grams of fat. two eggs and four pieces of bacon have a total of 32 grams of fat. how many grams of fat are in each?
Answers
We can solve the given system of equations to find how many grams of fat are in each when two eggs and two pieces of bacon have a total of 22 grams of fat and two eggs and four pieces of bacon have a total of 32 grams of fat.
Step-by-step explanation:
Let's consider that each piece of bacon has "x" grams of fat and each egg has "y" grams of fat.
From the given information, we can form two equations as follows:
Equation 1: 2x + 2y = 22 (when two eggs and two pieces of bacon have a total of 22 grams of fat)
Equation 2: 4x + 2y = 32 (when two eggs and four pieces of bacon have a total of 32 grams of fat)
To solve for "x" and "y", let's perform elimination by multiplying Equation 1 by (-2) and adding it to Equation 2.
-4x - 4y = -44 (multiply Equation 1 by -2)
+ 4x + 2y = 32 (Equation 2)
-2y = -12
y = 6
Substitute the value of "y" in Equation 1 or 2 to solve for "x":
2x + 2y = 22
2x + 2(6) = 22
2x = 10
x = 5
Therefore, each piece of bacon has 5 grams of fat and each egg has 6 grams of fat.
To learn more about “system of equations” refer to the https://brainly.com/question/13729904
#SPJ11
A recipe calls for 1 1/2 cups of mayonnaise and 3/8 cups of pecans. How much mayonnaise should be used if 1 cup of pecans is used?
Answers
Answer:
4 cups of mayonnaise.
Step-by-step explanation:
The ratio of the amount of pecans comparing reality and the recipe is:
1 : 3/8
= 1/(3/8)
= 8/3
Hence, to find the amount of mayonnaise that should be added:
8/3 * 3/2
= 8/2
= 4 cups
Hope this helped!
the circle contains (-2, 16) and x intercepts -2 and -32, write the equation of the circle described
Answers
The equation of the circle described by the given information is \((x + 2)^2 + (y - 16)^2 = 225.\)
To write the equation of a circle, we need the coordinates of the center and the radius. In this case, we are given the coordinates of a point on the circle and the x-intercepts.
Given:
Point on the circle: (-2, 16)
X-intercepts: -2 and -32
The x-intercepts represent the points where the circle intersects the x-axis. The distance between these points is equal to the diameter of the circle, which is twice the radius.
Radius = (Distance between x-intercepts) /\(2 = (-32 - (-2)) / 2 = -30 / 2 = -15\)
Now, we can use the coordinates of the center and the radius to write the equation of the circle in the standard form: \((x - h)^2 + (y - k)^2 = r^2\)
Center: (-2, 16)
Radius: -15
Substituting the values into the equation, we get:
\((x - (-2))^2 + (y - 16)^2 = (-15)^2\)
Simplifying further:
\((x + 2)^2 + (y - 16)^2 = 225\)
for more such questions on equation
https://brainly.com/question/22688504
#SPJ8
Which table is y a function of x?

Answers
Answer:
The first chart
Step-by-step explanation:
jennifer has 64 fair coins. she tosses all the coins. any coin that lands on tails is tossed again. coins that land on tails on the second toss are tossed a third time. what is the expected number of coins that are heads at the end?
Answers
The expected number of coins that are heads at the end after Jennifer has tossed 64 fair coins and those that landed on tails were tossed again is 32. the expected number of coins that are heads at the end is 48.
Here is a detailed explanation:Jennifer tosses 64 coins, and each coin has an equal chance of landing on either heads or tails. Thus, the probability that any one coin lands on heads is 0.5, and the probability that it lands on tails is also 0.5.When Jennifer tosses the 64 coins, some of them will land on tails.
Those coins will be tossed again. However, the probability of getting heads or tails for these coins is still 0.5 since the coins are fair.Therefore, the probability that a coin that has already been tossed and landed on tails to land on heads the second time is also 0.5.
The same applies to the third toss. The probability that a coin will land on heads or tails is 0.5.The total number of coins that land on tails during the first toss is given by
64*0.5 = 32.
Since these coins are tossed again, the expected number of coins that land on tails for the second toss is given by
32*0.5 = 16.
The remaining coins that landed on heads during the first toss are not affected. Thus, the expected number of coins that landed on heads during the first toss is
64 - 32 = 32.
The total number of coins that landed on heads during the first toss is given by 32.
Adding the expected number of coins that land on heads during the second toss (16),
the expected number of coins that land on heads is
32 + 16 = 48.
for more such question in probability
https://brainly.com/question/13604758
#SPJ11
grouping symbols exponents multiply and divide add and subtract
Answers
Grouping symbols exponents multiply and divide add and subtract represent the order of mathematical operations/calculations.
In mathematics, grouping symbols, exponents, multiplication and division, and addition and subtraction are the order of operations used to simplify mathematical expressions. The order of operations is a set of rules that dictate the order in which different operations are performed when evaluating an expression. The acronym PEMDAS is often used to help remember the order of operations:
P - Parentheses: Expressions inside parentheses should be evaluated first
E - Exponents: Exponents (such as "2^3" or "5^2") should be evaluated next
MD - Multiplication and Division: Multiplication and division should be done next, working from left to right
AS - Addition and Subtraction: Finally, addition and subtraction should be done, working from left to right.
It is important to follow the order of operations to ensure that mathematical expressions are evaluated correctly.
You can learn more about PEMDAS at
https://brainly.com/question/396257
#SPJ4
Ellen wants to put a down payment on a house in six years. She must accumulate $50,000 for the 10% down payment. Ellen puts X dollars in the bank now, X dollars after one year and X dollars after two years. How much should X be if the bank pays 5% interest, compounded annually? (b) [5 marks] After four years, the bank raises the interest it pays to 6% compounded annually. At the 6 year mark, Ellen takes $50,000 and uses it for the down payment and the rest is donated to a charity. How much is donated?
Answers
To calculate the value of X that Ellen should deposit in the bank, we need to determine the present value of the future payments that will accumulate to $50,000 in six years.
Using the formula for compound interest, the present value can be calculated as follows:
PV = X/(1 + r)^1 + X/(1 + r)^2 + X/(1 + r)^3,
where r is the annual interest rate (5%) expressed as a decimal.
To find the value of X, we set the present value equal to $50,000 and solve for X:
50,000 = X/(1 + 0.05)^1 + X/(1 + 0.05)^2 + X/(1 + 0.05)^3.
Once we determine the value of X, we can proceed to the next step.
For the second part of the question, after four years, the bank raises the interest rate to 6%.
From year four to year six, Ellen's money will continue to accumulate interest.
To find the amount donated, we calculate the future value of the remaining amount after deducting the down payment of $50,000:
Remaining amount = X/(1 + 0.06)^2 + X/(1 + 0.06)^3 + X/(1 + 0.06)^4.
The donated amount is then the difference between the remaining amount and the total accumulated after six years.
By evaluating these expressions, we can determine the value of X and the amount donated by Ellen.
Learn more about Compound Interest here:
brainly.com/question/12982348
#SPJ11
PLEASE HELP!!!
A circle with a radius of 17 centimeters is being dilated by a scale factor of 5. What is the length of the radius after the dilation?
Answers
The new radius of the dilated circle is 3.4 centimetres.
How to find the length of the radius of a dilated circle?The circle has a radius of 17 centimetres and is being dilated by a scale factor of 5. Therefore, the length of the radius after dilation can be calculated as follows:
A dilation is a transformation that produces an image that is the same shape as the original, but is a different size.
The circle was dilated by a scale factor of 5.
Hence,
New length of the radius = 17 / 5
New length of the radius = 3.4 centimetres
learn more on scale factor here: https://brainly.com/question/2910388
#SPJ1
Please help, I have been struggling for a whole week (40pts)


Answers
Answer:
3. Last choice: Domain: (-3, 0) U (0, ∞)
4. Last choice: Domain: (-∞, -3) U (-3, ∞), Range: (-∞, 0) U (0, ∞)
Step-by-step explanation:
3.
\((\frac{f}{g})(x) = \frac{1}{x}/ \sqrt{x+3}\)
=
\((\frac{f}{g})(x) = \frac{1}{x\sqrt{x+3}}\)
This function will be undefined at x = 0 and x = -3 because the denominator would be equal to 0. This means that the function has vertical asymptotes at x = 0 and x = -3, therefore:
Domain: (-3, 0) U (0, ∞)
*** Remember, x < -3 is not included in the domain because a square root of a negative number does not exist.***
4.
\(f(x) = \frac{1}{2x} - 3\)
Use "y" instead of f(x), and swap positions of x and y:
\(x = \frac{1}{2y} - 3\)
Simplify to isolate for "y":
\(x+3 = \frac{1}{2y}\)
\(2y(x+3) = 1\)
\(2y = \frac{1}{x+3}\)
\(y = \frac{1}{2(x+3)}\)
Based on the denominator, the function has a vertical asymptote at x = -3, therefore:
Domain: (-∞, -3) U (-3, ∞)
There is also an EBA asymptote of y = 0 since the denominator has a higher degree than the numerator, so:
Range: (-∞, 0) U (0, ∞)
prove 38^9-38^8 is divisible by 37
pls help
Answers
Answer:
see below
Step-by-step explanation:
38^9-38^8
Factor out 38 ^8
38^8 ( 38-1)
38^8 * (37)
This shows that it is divisible by 37 since one of the factors is 37
