A 3-meter ladder stands against the wall. The foot of the ladder moves outward at a constant speed of 0.1 m/s. (a) When the foot is 1 m from the wall, how fast is the top of the ladder falling? (b) What is the value of this speed when the foot is 2 m from the wall?
Answers
a) When the foot is 1 m from the wall (x = 1), we can substitute the values into the equation and solve for dh/dt. (b) we can apply the same concept of related rates. We'll calculate the rate of change of the height at that specific point.
To solve both parts, we can use the Pythagorean theorem to relate the sides of the right triangle formed by the ladder. Let h be the height of the triangle and x be the distance of the foot from the wall. Then, h^2 + x^2 = 3^2.
By differentiating the equation with respect to time, we can find the relationship between the rates of change. Using the chain rule, we obtain 2h(dh/dt) + 2x(dx/dt) = 0. Since the foot is moving outward at a constant speed of 0.1 m/s, dx/dt = 0.1 m/s.
In part a, when the foot is 1 m from the wall (x = 1), we can substitute the values into the equation and solve for dh/dt, which represents the rate at which the height is changing.
In part b, when the foot is 2 m from the wall (x = 2), we can substitute the values into the equation and solve for dh/dt again to find the speed at that point.
To learn more about Pythagorean theorem click here, brainly.com/question/14930619
#SPJ11
Related Questions
Help asap, Algebra 1 easy question

Answers
Answer:
x^2+9x+18
Step-by-step explanation:
x-y=0
x=y
in this case we have the roots which are the x values so:
x=-6
x+6=0
and
x=-3
x+3=0
(x+3)(x+6)=0
x^2+6x+3x+18=0
x^2+9x+18=0
Tickets to the school musical cost $6.25. The amount received from ticket sales is $706.25. How many tickets were sold?
Answers
Answer:
Step-by-step explanation:
if we received 706.25 dollars and a ticket is 6.25
706.25/6.25=113 tickets sold
Answer:
113
Step-by-step explanation:
You have to divided 706.25 by 6.25 and then you get 113. 113 is your anwser.
Hope this helped!
Find the distance between -23 and 17 on a number line.
d =
Answers
Answer:
Distance between -23 & 17 = 40
Step-by-step explanation:
what does the dats suggest

Answers
Answer:
the bigger the handspan the more jolly ranchers they can pick up with one hand
Step-by-step explanation:
ind the limit, if it exists. limx→0+ (e^2x+x)^1/x a.1 b.2 c.[infinity] d.3 e.e^2
Answers
The limit of the expression as x approaches 0 from the positive side is e^2. Therefore, the limit of the expression is (1/x) * ln(e^(2x) + x) = (1/x) * 0 = 0.
To find the limit of the expression (e^(2x) + x)^(1/x) as x approaches 0 from the positive side, we can rewrite it as a exponential limit. Taking the natural logarithm of both sides, we have:
ln[(e^(2x) + x)^(1/x)].
Using the logarithmic property ln(a^b) = b * ln(a), we can rewrite the expression as:
(1/x) * ln(e^(2x) + x).
Now, we can evaluate the limit as x approaches 0 from the positive side. As x approaches 0, the term (1/x) goes to infinity, and ln(e^(2x) + x) approaches ln(e^0 + 0) = ln(1) = 0.
Therefore, the limit of the expression is (1/x) * ln(e^(2x) + x) = (1/x) * 0 = 0.
Taking the exponential of both sides, we have:
e^0 = 1.
Thus, the limit of the expression as x approaches 0 from the positive side is e^2.
Learn more about logarithmic property here:
https://brainly.com/question/12049968
#SPJ11
I just need help pleaseee
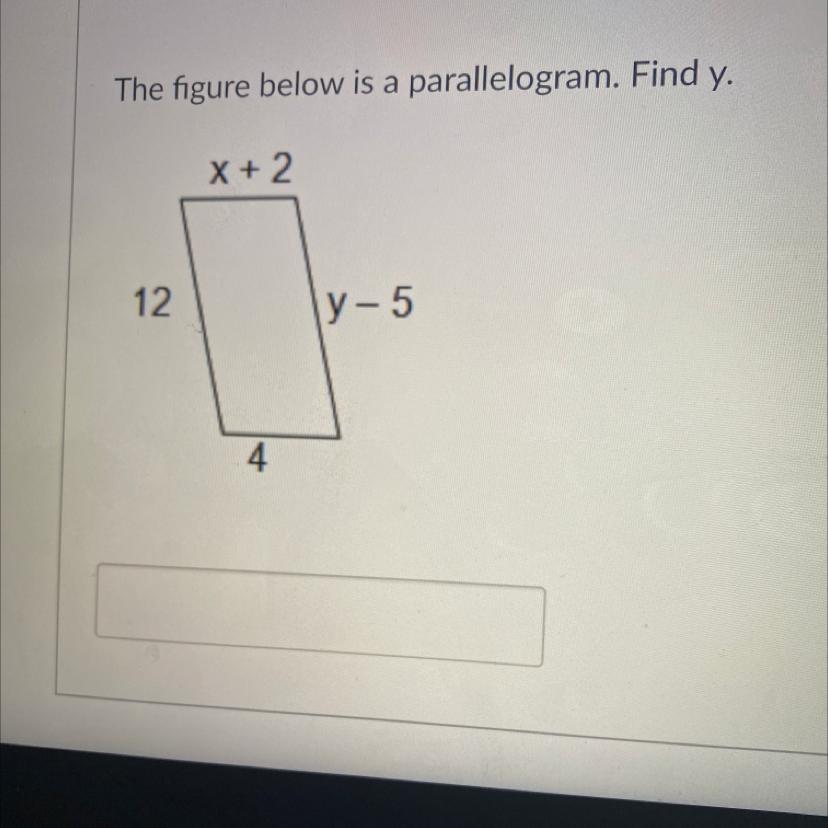
Answers
Answer:
y=17
Step-by-step explanation:
opposite sides of parallelogram are congruent.
so , 12= y-5
or, y=17
Forum - Radicals (Moderated)
View Full Description
Which do you prefer: leaving √5 in radical form, or converting it to a decimal? Why? When would it be useful to use each?
Answers
I would prefer leaving the answer as a radical number as √5 since it is a shorter form and exact form of the answer.
How to express radical numbers?My reasons for leaving it as a radical form or a decimal approximation are;
1) If we are trying to find an exact answer, then we should leave it as √5. For example, if we are told a square has an area of 5 square units, then it means that the exact length of the side is √5 units.
2) If √5 is the length of an object that has to be made to that specific length, then we would require an approximation. For example, if you are asked to make a square out of cardboard whose area is 5 square feet, then we will definitely need an approximation of √5 ft = 2.24 ft, which would help us make each side of the cardboard square in the length of 2.24 ft.
Read more about Number radicals at; https://brainly.com/question/28519153
#SPJ1
how do do the work for 12c+6c=36
Answers

Answer:
c = 2
Step-by-step explanation:
To solve this equation, you need to combine like terms and then divide by the coefficient of the variable on both sides of the equation.
To start, add 12c and 6c because they are like terms.
12c + 6c = 18c
Then, divide by 18 on both sides of the equation to solve for c.
18c = 36
---- ----
18 18
c = 2
An electric monorail transport is slowing down at a rate of 2t + 25 ft per second per second, where t is the time in seconds. by how many feet per second does the monorail slow down between t = 1 and t = 5 seconds?
124 ft/sec
125 ft/sec
126 ft/sec
127 ft/sec
Answers
The electric monorail slows down by 124 ft between t = 1 and t = 5 seconds. To find the change in the rate of slowing down for the electric monorail transport between t = 1 and t = 5 seconds, we need to calculate the difference in its deceleration at these two points in time.
Given the deceleration formula: 2t + 25 ft/s², let's find the deceleration rates at t = 1 and t = 5:
Deceleration at t = 1:
2(1) + 25 = 2 + 25 = 27 ft/s²
Deceleration at t = 5:
2(5) + 25 = 10 + 25 = 35 ft/s²
Now, we will find the difference in deceleration between these two points in time:
Difference = Deceleration at t = 5 - Deceleration at t = 1
Difference = 35 ft/s² - 27 ft/s²
Difference = 8 ft/s²
The electric monorail slows down by 8 ft/s² between t = 1 and t = 5 seconds. However, this is not listed as one of your options. If you meant to ask how many feet it slows down in total between t = 1 and t = 5 seconds, we can calculate the integral of the deceleration formula:
∫(2t + 25)dt from t = 1 to t = 5
The integral of (2t + 25) is t² + 25t + C. Now, we can evaluate it:
(5² + 25*5) - (1² + 25*1) = (25 + 125) - (1 + 25) = 150 - 26 = 124 ft
So, the electric monorail slows down by 124 ft between t = 1 and t = 5 seconds. Your answer: 124 ft/sec.
learn more about deceleration here: brainly.com/question/75351
#SPJ11
how many positive integers of 3 digits may be made from the digit 1,2,3,4,5, each digit may be used just once
Answers
60 positive integers of 3 digits may be made from the digit 1,2,3,4 and 5 if the digits are not repeated.
According to the question,
We have the following information:
3 digits integers are to be made from 1,2,3,4 and 5
So, we will use permutation to find the possible number of digits that can be made if we apply all the given conditions.
Now, we have:
Total number of digits = 5
Number of digits to be made = 3
So, we have:
\(^{5} P_{3}\)
Solving this expression:
5*4*3
60
Hence, 60 positive integers of 3 digits may be made from the digit 1,2,3,4 and 5 if the digits are not repeated.
To know more about digits here
https://brainly.com/question/28062468
#SPJ4
An alloy contains 13. 5 gms of copper and 4. 5 gms of zinc. Find the ratio by mass of copper to zinc in the alloy
Answers
The ratio by mass of copper to zinc in the alloy is 3:1.
To find the ratio by mass of copper to zinc in the alloy, we need to first calculate the total mass of the alloy. We can do this by adding the mass of copper and zinc:
Total mass of alloy = 13.5 g + 4.5 g = 18 g
Now we can find the ratio of copper to zinc by dividing the mass of copper by the mass of zinc:
Ratio of copper to zinc = 13.5 g / 4.5 g = 3:1
Therefore, the ratio by mass of copper to zinc in the alloy is 3:1.
Learn more about mass here
https://brainly.com/question/86444
#SPJ11
what is the probability that a random integer from 92 to 734 is divisible by 15? (all integers in the given range are equally likely to be chosen).
Answers
There are 43 integers in the range from 92 to 734 that are divisible by 15. The probability that a random integer from 92 to 734 is divisible by 15 is approximately 0.067.
To find the probability that a random integer from 92 to 734 is divisible by 15, we need to first determine the total number of integers in this range. The difference between 734 and 92 is 642, but since we want to include both endpoints, we need to add 1 to this difference. So there are a total of 643 integers in the range from 92 to 734. Next, we need to determine how many of these integers are divisible by 15. To do this, we need to find the smallest multiple of 15 that is greater than or equal to 92, and the largest multiple of 15 that is less than or equal to 734. The smallest multiple of 15 that is greater than or equal to 92 is 105 (which is 7 times 15), and the largest multiple of 15 that is less than or equal to 734 is 720 (which is 48 times 15).
So the integers from 105 to 720 (inclusive) are all divisible by 15. To count the number of integers in this range, we can divide the difference between 720 and 105 by 15, and add 1 to account for the first multiple of 15:
(720 - 105) / 15 + 1 = 43
Therefore, there are 43 integers in the range from 92 to 734 that are divisible by 15.
To find the probability that a random integer from this range is divisible by 15, we can divide the number of integers that are divisible by 15 (43) by the total number of integers in the range (643):
43 / 643 ≈ 0.067
So the probability that a random integer from 92 to 734 is divisible by 15 is approximately 0.067.
Learn more about probability here: brainly.com/question/30034780
#SPJ11
What is the average rate of change for this quadratic function for the interval from x = 0 to x = 2?
A. –2
B. 2
C. 4
D. –4
Answers
Someone plz help me :(

Answers
Answer:
10 cars
Step-by-step explanation:
50*20/100
20/2=10
Answer:
Hello!!
If Mandy has 50 toy cars and 20% of the cars are silver. 10 cars are silver.
Step-by-step explanation:
\(50\) ×\(0.20=10\)
Hope this helps!!
3) Five times the second of three consecutive odd integers is
thirteen less than three times the sum of the first and third
integers. Find the largest odd integer..
Answers
9514 1404 393
Answer:
15
Step-by-step explanation:
Let x represent the middle odd integer.
5x = 3((x-2)+(x+2)) -13
5x = 6x -13
x = 13 . . . . the middle of 3 consecutive odd integers
The largest odd integer is 15.
Which expression represents a difference of squares ?

Answers
Answer:
first and last options
Step-by-step explanation:
Difference of squares are in the form a² - b². The only choices that satisfy this are 25x² - 36 and 1 - 16x².
Answer:
1 and 4
Step-by-step explanation:
Which event is certain?
The sun will rise
You will become president
A student will be late for class
It will snow tomorrow
Answers
Answer:
will rise it is most likely
Step-by-step explanation:
Marjane wants to create a set of data with 6 values. She wants the mode to be as good as the median to represent the data set. Which set of data best represents what Marjane could create?
24, 24, 25, 29, 29, 29
24, 25, 26, 27, 30, 30
24, 25, 25, 25, 26, 26
24, 24, 25, 26, 26, 27
Answers
As per the median, the set of data that fulfilling Marjane's requirement is 24, 25, 25, 25, 26, 26 (option c).
In statistics, data is a collection of numbers or values that represent a particular phenomenon. One way to measure central tendency, or the typical or representative value of the data, is through the median and the mode.
The median is the middle value when the data is arranged in numerical order, and the mode is the value that appears most frequently.
The third set of data is 24, 25, 25, 25, 26, 26.
The median is the middle value, which is also (25+25)/2 = 25.
The mode is the value that appears most frequently, which is 25.
Therefore, the mode and median are the same, fulfilling Marjane's requirement.
Therefore, the correct option is (c).
To know more about median here
https://brainly.com/question/30891252
#SPJ4
Pls help, question on picture, will do brainliest if right
no links!!!!!

Answers
Answer:
\(tan(\theta) = \frac{3}{4}\)
Step-by-step explanation:
Remember the trigonometric ratios, keep in mind that the way the side is referred to is based on its position relative to the angle getting referenced. The side next to the referenced angle (\(\theta\)) is called the adjacent side, the one opposite the angle is called the opposite side, and the side opposite the right angle of the triangle is called the hypotenuse.
\(sin (sine) = \frac{opposite}{hypotenuse}\\\\cos (cosine) = \frac{adjacent}{hypotenuse}\\\\tan(tangent) = \frac{opposite}{adjacent}\)
In this problem, one is asked to find the value of (\(\theta\)) with regards to a trigonometric function. One is given the opposite and adjacent sides, it would make the most sense to use the trigonometric function (tangent). Substitute the sides into their respective place, and simplify to find the value of the trigonometric function.
\(tan(\theta) =\frac{15}{20}\\\\\\tan(\theta)=\frac{3}{4}\)
a rectangular table can be detrimental by emphasizing status differences between learners. group of answer choices true false
Answers
The statement "a rectangular table can be detrimental by emphasizing status differences between learners" is true.
Rectangular tables, unlike round or oval tables, can make it difficult for all learners to be equal since rectangular tables often have a head or primary position.
Therefore, the person who is sitting in the primary position can quickly become the central focus of the group, causing other members to feel inferior. This is particularly important for learners in a classroom environment, where creating a sense of equality and encouraging everyone to participate and share ideas is crucial.
Learners might feel isolated or overlooked when seated around a rectangular table, particularly if they are at the end of the table or farther from the primary position. This can have an impact on their self-confidence and participation in group activities, as well as their capacity to learn.
Learn more about detrimental:
https://brainly.com/question/31572115
#SPJ11
In ΔWXY, \overline{WY} WY is extended through point Y to point Z, \text{m}\angle YWX = (3x+17)^{\circ}m∠YWX=(3x+17) ∘ , \text{m}\angle XYZ = (10x-5)^{\circ}m∠XYZ=(10x−5) ∘ , and \text{m}\angle WXY = (3x+2)^{\circ}m∠WXY=(3x+2) ∘ . Find \text{m}\angle WXY.m∠WXY
Answers
The value of ∠WXY = 20.
What is Exterior angle theorem?
The exterior angle theorem describes the connection between the two remote angles in a triangle and the external angle created by an extended side outside the triangle.
Given: Measure of angle YWX = (3x + 17) °
Measure of angle WXY = (3x + 2) °
Measure of angle XYZ = (10x − 5) °
Therefore, m∠XYZ = m∠YWX + m∠WXY (exterior angle theorem)
⇒ (10x − 5) ° = (3x + 17) ° + (3x + 2) °
Solve for x,
⇒ 10x - 5 = 3x + 17 + 3x + 2
⇒ 10x - 6x = 17 + 7
⇒ 4x = 24
⇒ x = 6
∴ ∠WXY = (3x + 2) = 18 + 2 = 20
Hence, value of ∠WXY = 20.
To learn more about exterior angle theorem from the given link:
https://brainly.com/question/11356657
#SPJ1
group of 25 pennies is arranged into three piles such that each pile contains a different prime number of pennies. what is the greatest number of pennies possible in any of the three piles?
Answers
The greatest number of pennies possible in any of the three piles is 23.
Given that the group of 25 pennies is arranged into three piles such that each pile contains a different prime number of pennies.
We need to find the greatest number of pennies possible in any of the three piles, we need to consider prime numbers less than or equal to 25. Let's examine the prime numbers less than 25:
2, 3, 5, 7, 11, 13, 17, 19, 23
Since we have 25 pennies in total, we can't have a pile with a prime number greater than 25.
Therefore, the greatest number of pennies possible in any of the three piles is 23.
To learn more on Number system click:
https://brainly.com/question/22046046
#SPJ4
The napikin fing picfurod in the following figare is to be resivared. How many scuare mall meters must be covered? The area to be allvered is mm 2
. (Type an exact answer in terms of π.)
Answers
The mall meters must be covered (0.09π) square meters.
The number of square centimeters that must be covered.
Given figure: We know that the napkin is in the shape of a circle, therefore we will use the formula for the area of a circle:
Area of the circle = πr²
Here,r = radius of the circle.
To find the radius of the circle, we need to find the diameter first.
Now, we can see that the diameter is equal to the side of the square that surrounds the circle.
Therefore, diameter = 6cmSo, radius = diameter/2= 6/2= 3cm
Area of the circle = πr²= π(3)²= 9π square cm
Also, given that the area to be covered is mm².
But, we know that 1 cm = 10 mm.
So, 1 cm² = (10 mm)²= 100 mm²
Therefore, 9π square cm= 9π × (100 mm²)= 900π square mm= 900π/10000 square m= 9π/100 square m= (0.09π) square m
So, the area that must be covered is (0.09π) square m.
Therefore The mall meters must be covered (0.09π) square meters.
Learn more About meters from the link
https://brainly.com/question/1578784
#SPJ11
Given points A(-1,4) and B(x,7), determine the value(s) of x if AB=5cm
Answers
The value of x is either 3 or -5 based on the distance formula.
What is a co-ordinate system?
In pure mathematics, a coordinate system could be a system that uses one or additional numbers, or coordinates, to uniquely confirm the position of the points or different geometric components on a manifold like euclidean space.
Main body:
according to question
Given points A(-1,4) and B(x,7)
Also AB = 5 cm
Formula of distance = \(\sqrt{(y1-y2)^{2}+(x1 -x2)^{2} }\)
here by using points ,
5 = \(\sqrt{(x+1)^{2} +(7-4)^{2} }\)
taking square on both side ,'
25 = \((x+1)^{2} +3^{2}\)
25-9 = (x+1)²
16 = (x+1)²
taking square root on both sides,
x+1= ±4
x = 4-1 = 3 or x = -4-1 = -5
Hence value of x is either 3 or -5.
To know more about point , visit:
https://brainly.com/question/26310043
#SPJ1
Let X be distributed over the set N of non-negative integers, with pmf a P(X = i) = 21 for some fixed α E R. Find EX For Y X mod 3, find ·P(Y= 1) .ELY
Answers
Let's analyze the given probability mass function (pmf) for the random variable X. We know that P(X = i) = 21 for some fixed α in the set of real numbers, R. However, it seems there is an error in the given pmf value. The probability of any specific value in a discrete probability distribution should be between 0 and 1. Therefore, it is not possible for P(X = i) to equal 21.
To proceed with finding EX, we need a valid pmf. Without further information or clarification, it is not possible to determine the expected value of X.
Moving on to the second part of the question, we introduce a new random variable Y = X mod 3. The modulus operator (mod) finds the remainder when dividing X by 3. In other words, Y represents the numbers in X that leave a remainder of 1 when divided by 3.
To find P(Y = 1), we need to calculate the probability that Y takes the value 1. Since Y represents the remainder when dividing X by 3, Y can only take the values 0, 1, or 2.
To calculate P(Y = 1), we sum up the probabilities of all the values in X that leave a remainder of 1 when divided by 3. Mathematically, we can express this as:
P(Y = 1) = P(X = 1) + P(X = 4) + P(X = 7) + ...
However, since the pmf values were given incorrectly, it is not possible to compute P(Y = 1) without a valid pmf. Therefore, we cannot provide a specific numerical answer for P(Y = 1) in this case.
In summary, without a valid pmf for X, it is not possible to determine the expected value of X (EX) or calculate the probability P(Y = 1) for the random variable Y = X mod 3.
Learn more about Probability Mass Function :
https://brainly.com/question/30765833
#SPJ11
what is the slope of the line between (0,8) and (2,20)
Answers
Answer:-1/6
Step-by-step explanation:
Answer:
slope is 6
Step-by-step explanation:
Write the equation of a parabola whose directrix is x=4 and has a focus at (-6,-5).
Write the equation of a parabola whose directrix is y=2 and has a focus at (3,10).
Find the equation for the parabola that has its focus at (-3,2) and has directrix y=6.
Find the equation for the parabola that has its vertex at the origin and has directrix at x=-1/43.
Find an equation for the parabola that has its vertex at the origin and has its focus at the point (0,-6.4).
Answers
The equations of the parabolas are: (a) (x + 5)^2 = 8(y + 6) (b) (y - 6)^2 = 4(x - 0)
(c) (y - 0)^2 = 16(x + 1/43) (d) (y + 6.4)^2 = 4y
(a) To find the equation of a parabola with directrix x = 4 and focus at (-6, -5), we can use the formula: (x - h)^2 = 4p(y - k), where (h, k) is the vertex and p is the distance between the vertex and the focus. In this case, the vertex is (-6, -5), and p is the distance from (-6, -5) to the directrix x = 4, which is 10 units. Plugging in the values, we get (x + 6)^2 = 8(y + 5).
(b) For a parabola with directrix y = 2 and focus at (3, 10), we use the formula: (y - k)^2 = 4p(x - h). The vertex is (3, 10), and the distance between the vertex and the directrix y = 2 is 8 units. Plugging in the values, we get (y - 10)^2 = 32(x - 3).
(c) To find the equation for a parabola with focus at (-3, 2) and directrix y = 6, we can use the formula (y - k)^2 = 4p(x - h). The vertex is the midpoint between the focus and the directrix, which is (-3, 4). The distance between the vertex and the focus (or directrix) is the value of p, which is 2 units. Plugging in the values, we get (y - 4)^2 = 16(x + 1/43).
(d) For a parabola with vertex at the origin and focus at (0, -6.4), we can use the formula (x - h)^2 = 4p(y - k). The vertex is (0, 0), and the distance between the vertex and the focus (or directrix) is the value of p, which is 6.4 units. Plugging in the values, we get (y - 0)^2 = 4(6.4)y, which simplifies to y^2 = 4(6.4)y.
Learn more about Parabola here: brainly.com/question/11911877
#SPJ11
Hello I need help with this math problem

Answers
Answer:
75 degrees. You have to add 45+60 and you get 105. Then you do 180-105 and you get 75.
In network high availability measurement, what is the meaning of "five nines"? (Choose one answer)
A. Means the network should have 99.999 percent uptime (no more than 5 hours downtime per year)
B. Means the network should have 99.999 percent uptime (no more than 1 hour of downtime per year)
C. Five out of every nine network administrators are always available to take care of network problems
D. Means the network should have 99.999 percent uptime (no more than 5 minutes downtime per year)
Answers
The meaning of "five nines" in network high availability measurement is that the network should have 99.999 percent uptime, which translates to no more than 5 minutes of downtime per year.
In network high availability measurement, "five nines" refers to a standard for uptime reliability. It represents a target of 99.999 percent uptime, which is an extremely high level of availability.
This means that the network should be operational and accessible to users for 99.999 percent of the time, allowing for a maximum of 5 minutes of downtime in a year.
Achieving such a high level of uptime requires implementing robust redundancy, failover mechanisms, and fault-tolerant systems. It involves ensuring that network components, such as routers, switches, servers, and connectivity,
are highly reliable and resilient to failures. Redundancy in critical infrastructure, backup power systems, and proactive maintenance practices are typically employed to minimize downtime and provide continuous network availability.
The "five nines" standard is commonly used in industries where uninterrupted network connectivity is crucial, such as telecommunications, data centers, financial institutions, and healthcare.
It signifies a commitment to providing a highly reliable and stable network infrastructure to meet the demanding needs of users and ensure minimal disruption to operations.
To know more about measurement click here
brainly.com/question/26591615
#SPJ11
to investigate this claim, a random sample of 150 students is selected. what are the appropriate hypotheses?h0: the distribution of lunch preferences is 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza : the distribution of lunch preferences is not 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza place.h0: the distribution of lunch preferences is not 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza : the distribution of lunch preferences is 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza place.h0: in the sample of 150 students, 105 will prefer the cafeteria, 15 will prefer the hut, 15 will prefer the taco wagon, and 15 will prefer pizza : in the sample of 150 students, the distribution will not be that 105 will prefer the cafeteria, 15 will prefer the hut, 15 will prefer the taco wagon, and 15 will prefer the pizza place.h0: in the sample of 150 students, the distribution will not be that 105 will prefer the cafeteria, 15 will prefer the hut, 15 will prefer the taco wagon, and 15 will prefer the pizza : in the sample of 150 students, 105 will prefer the cafeteria, 15 will prefer the hut, 15 will prefer the taco wagon, and 15 will prefer the pizza place.
Answers
H0: The distribution of lunch preferences is 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza.
Ha: The distribution of lunch preferences is not 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza place.
In hypothesis testing, we have a null hypothesis (H0) and an alternative hypothesis (Ha). The null hypothesis represents the claim or assumption we want to test, while the alternative hypothesis represents the opposite or alternative claim.
In this case, the null hypothesis (H0) states that the distribution of lunch preferences is 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza place. The alternative hypothesis (Ha) states that the distribution of lunch preferences is not 70% cafeteria, 10% hut, 10% taco wagon, and 10% pizza place.
To test these hypotheses, a random sample of 150 students is selected, and their lunch preferences are recorded. The goal is to determine if the observed distribution of lunch preferences in the sample provides enough evidence to reject the null hypothesis in favor of the alternative hypothesis.
Learn more about sample here:
https://brainly.com/question/27860316
#SPJ11