a 38-ft ladder is leaning against a building the bottom of the ladder is 7 ft from the base of the building to the nearest foot how high it does the ladder reach the building?
Answers
Answer:
37 ft.
Step-by-step explanation:
Use the Pythagorean theorem to solve.
The hypotenuse = 38 and a leg is 7.
Let d = distance from ground to top of the ladder
\(38^{2} = x^{2} + 7^{2}\)
1444 = \(x^{2} + 49\)
\(x^{2} = 1395\\x = \sqrt{1395} = 37.3496...\\37 ft.\)
Related Questions
which of these expression is equivalent to 2×+XY

Answers
Find the probability if two dice are rolled. (Enter the value of probability in decimals. Round the answer to three decimal places.)
Answers
The probability of getting a total of 8 when two dice are rolled is 0.139 when the answer is rounded to three decimal places.
What is meant by Probability?Numerous uses of mathematics' probability formula may be found. Probability can be used, for instance, to determine the likelihood that a certain marketing would really be successful in generating fresh customers. Calculating the likelihood of something happening is another use of probability.
Let's examine what probability is, how to compute the probabilities of single and many random occurrences, and how the terms probability and odds vary from one another.
The likelihood that an event like rolling two dice will occur is determined by probability:
P(A) = f / N
Odds and probability are connected, but the probability determines the odds. Probability is required before calculating the likelihood of an occurrence.
The probability is P(getting a total of 8 from both dices)=5/36= 0.139.
To learn more about Probability, visit:
https://brainly.com/question/23554593
#SPJ4
What is the mathematical expression for modified Reynolds Analogy, also known as Chilton-Colburn analogy?
Answers
The modified Reynolds analogy, also known as the Chilton-Colburn analogy, is expressed mathematically as Nu = f * Re^m * Pr^n. It relates the convective heat transfer coefficient (h) to the skin friction coefficient (Cf) in fluid flow. This equation is widely used in heat transfer analysis and design applications involving forced convection.
The modified Reynolds analogy is a useful tool in heat transfer analysis, especially for situations involving forced convection. It provides a correlation between the heat transfer and fluid flow characteristics. The Nusselt number (Nu) represents the ratio of convective heat transfer to conductive heat transfer, while the Reynolds number (Re) characterizes the flow regime. The Prandtl number (Pr) relates the momentum diffusivity to the thermal diffusivity of the fluid.
The equation incorporates the friction factor (f) to account for the energy dissipation due to fluid flow. The values of the constants m and n depend on the flow conditions and geometry, and they are determined experimentally or by empirical correlations. The modified Reynolds analogy is widely used in engineering calculations and design of heat exchangers, cooling systems, and other applications involving heat transfer in fluid flow.
Learn more about Prandtl number here:
https://brainly.com/question/32353670
#SPJ11
if f(x)= x²+x(2a+2b)+4ab-x. f'(x)=....
DON'T COPY PASTE!!! please use the methods and steps
Answers
\(f(x) = x^2 +x (2a+2b) +4ab-x\\\\f'(x) = 2x+x \cdot 0 + 2a+2b + 0 -1\\\\~~~~~~~~=2x+0+2a+2b-1\\\\~~~~~~~~=2x+2a+2b-1\)
From the definition of the derivative,
\(f'(x) = \displaystyle \lim_{h\to0} \frac{f(x+h) - f(x)}h\)
We have
\(f(x) = x^2 + x(2a+2b) + 4ab - x = x^2 + (2a+2b-1)x + 4ab\)
and so
\(\displaystyle f'(x) = \lim_{h\to0} \frac{\left((x+h)^2 + (2a+2b-1)(x+h) + 4ab\right) - \left(x^2 + (2a+2b-1)x + 4ab\right)}h\)
\(\displaystyle f'(x) = \lim_{h\to0} \frac{x^2 + 2xh + h^2 + (2a+2b-1)x + (2a+2b-1)h + 4ab - x^2 - (2a+2b-1)x - 4ab}h\)
\(\displaystyle f'(x) = \lim_{h\to0} \frac{2xh + h^2 + (2a+2b-1)h}h\)
\(\displaystyle f'(x) = \lim_{h\to0} (2x + h + 2a+2b-1)\)
\(\displaystyle f'(x) = \boxed{2x + 2a + 2b - 1}\)
Some friends played a board game. During the game, one unlucky player had to move back 8 spaces 5 turns in a row. Find a number to represent that player's movements for those 5 turns.
NEXT
A football team loses 8 yards on each of 4 consecutive plays. Find the total change in yards from where the team started.
The total change is
nothing yards. plsssssssssssssssssssssssssssss help. their i raised the points'
Answers
Answer:
I think that it would be -40
Step-by-step explanation:
I think this because they had to go back 40 spaces because 8 x 5 = 40.
The required number is 40 which represents that player's movements for those 5 turns.
Given that,
Some friends played a board game. During the game, one unlucky player had to move back 8 spaces 5 turns in a row.
To determine the number to represent that player's movements for those 5 turns.
In mathematics, it deals with numbers of operations according to the statements. There are four major arithmetic operators, addition, subtraction, multiplication and division,
Here,
For every turn, he has back 8 spaces
To the number of spaces back in 5 turns,
Total spaces back = 5 * 8
= 40
Thus, the required number is 40 which represents that player's movements for those 5 turns.
Learn more about arithmetic here:
brainly.com/question/14753192
#SPJ2
if x+y=10 and x-y=6 , what is the value of x³-y³
Answers
Answer:
\(\huge\boxed{x^3-y^3=508}\)
Step-by-step explanation:
\(\underline{+\left\{\begin{array}{ccc}x+y=10\\x-y=6\end{array}\right}\qquad|\text{add the sides of the equations}\\.\qquad2x=16\qquad|\text{divide both sides by 2}\\.\qquad\boxed{x=8}\\\\\text{Substitute it to the first equation}\\8+y=10\qquad|\text{subtract 8 from both sides}\\\boxed{y=2}\\\\x^3-y^3=8^3-2^3=512-8=504\)
Solving For x.
\(2x + 5 = 1\)
Step By Step Explanation, Please.
Answers
![Solving For x. [tex]2x + 5 = 1[/tex]Step By Step Explanation, Please.](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/nMAN0iAqOIxwM3FaH5yn96pLBvaIcXPC.png)
![Solving For x. [tex]2x + 5 = 1[/tex]Step By Step Explanation, Please.](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/TshMY7e4e2H0EOKQInMyjdqgiF36owkF.png)
The area of a triangle garden can
be no more than 120 square
feet. The base of a triangle
is 16 feet in
lenght What
inequalitie describes the
possible heights, h, of the
triangular garden?
Answers
Answer:
0< h ≤ 15
Step-by-step explanation:
A = 1/2 * base * height = 1/2*16*h = 8h
A no more is ≤.
A ≤ 120
8h ≤ 120
h ≤ 15
0< h ≤ 15
Height should be more than 0 feet and no more than 15 feet.
the ninth phase of the training was probably the most difficult. astronaut #6 did the best he only had to start over once every 30 seconds. astronaut #8 on the other hand perform very poorly. he had to start over once every sixth seconds. How many seconds did it take the two of them to start over 50 times?
Answers
Answer: 10
Step-by-step explanation:
The time taken by both of the astronaut is 1500 sec to start over 50 times.
What is time and work?Work is defined as anything that affects or results in something else; frequently the one wanted or anticipated. The basic idea of proportionality, which applies to all arithmetic subjects, is the same to time and work.
When the amount of work completed remains constant, efficiency is inversely proportional to time spent.
Given time to start over for astronaut #6 = 30 sec
time to start over for astronaut #8 = 6 sec
common time for both astronaut to start is
taking LCM of 30 and 6
2 x 3 x 5 = 30 sec
total time for two of them to start over 50 times is 30 x 50 = 1500 sec
Hence 1500 sec is taken by two of them to start over 50 times.
Learn more about time & work;
https://brainly.com/question/3854047
#SPJ2
ANSWER QUICKlY ASAP!!!!

Answers
Answer:
\( \sqrt{9 } = 3 \)
in an after-school program for juniors and seniors there is a debate team with an equal number of students from each class on the team. among the 28 students on the program, 25% of the juniors and 10% of the seniors are on the debate team. how many juniors are in the program?
Answers
There are 8 juniors in the program, Let J be the number of juniors in the program and S be the number of seniors. We are given that J + S = 28 and 0.25J = 0.1S.
Solving the first equation for S, we get S = 28 - J. Substituting this into the second equation, we get:
0.25J = 0.1(28 - J)
Simplifying the equation, we get J = 8.
Therefore, there are 8 juniors in the program.
Let J be the number of juniors in the program and S be the number of seniors.
We are given that J + S = 28 and 0.25J = 0.1S.
Solving the first equation for S, we get S = 28 - J. Substituting this into the second equation, we get:
Code snippet
0.25J = 0.1(28 - J)
Simplifying the equation, we get J = 8.
Therefore, there are 8 juniors in the program.
The answer can also be found by using a Venn diagram. The Venn diagram shows that there are 4 juniors on the debate team and 4 seniors on the debate team.
Since the number of students on the debate team is equal to the number of juniors, there must be 8 juniors in the program.
To know more about equation click here
brainly.com/question/649785
#SPJ11
Let R be the region in the first quadrant bounded by x2 − y2 = 16, the horizontal line y = 0, and the vertical line x = 5. What is the volume of the solid formed by revolving R about the x-axis?
Answers
Check the picture below.
so the region is the one bounded by a hyperbola and two straight lines, one of which is the x-axis, or namely y = 0, since the hyperbola has a vertex at x = 4, then our bounds are from 4 to 5.
\(x^2-y^2=16\implies x^2-16=y^2\implies \pm\sqrt{x^2-16}=y \\\\\\ \stackrel{I~Quadrant}{+\sqrt{x^2-16}=y}\qquad \qquad \implies \stackrel{\textit{using the disk method}~\hfill }{\displaystyle\int_{4}^{5}~\pi \left( \sqrt{x^2-16} \right)^2\cdot dx\implies \pi \int_{4}^{5}(x^2-16)dx} \\\\\\ \pi \left( \left. \cfrac{x^3}{3} \right]_{4}^{5} ~~ - ~~\left. 16x\cfrac{}{} \right]_{4}^{5}\right)\implies \pi \left[\cfrac{61}{3}~~ - ~~16 \right]\implies \cfrac{13\pi }{3}\)

The point p(x, y) lies on the terminal side of an angle theta = startfraction 3 pi over 4 endfraction in standard position. what are the signs of the values of x and y? both x and y are positive. both x and y are negative. x is positive, and y is negative. x is negative, and y is positive.
Answers
The 'x' coordinate is negative and 'y' coordinate is positive.
What is Fraction ?A fraction is a part of a whole. In arithmetic, the number is expressed as a quotient, in which the numerator is divided by the denominator. In a simple fraction, both are integers. A complex fraction has a fraction in the numerator or denominator. In a proper fraction, the numerator is less than the denominator.
We know that :
π = 180
Therefore,
3π/4 = (3×180)/4 = 3 × 45
3π/4 = 135
In second quadrant, we have negative value if x coordinate and positive value of y coordinate.
Hence, the angle lies in the second quadrant. Second quadrant is enclosed by the negative x axis and positive y axis.
To learn more about Fraction visit :
https://brainly.com/question/16536310
#SPJ4
Answer:
x is negative, and y is positive.
Step-by-step explanation:
The answer above is correct.
More help plsssssssss

Answers
3
x = 3
hope this helped
Answer:
8) x = 3
9) x = 10
Step-by-step explanation:
8) 45° ≅ 15x, therefore, the equation would look like: \(45 = 15x\)
☆Let's solve it!
\( \frac{45}{15} = \frac{15x}{15} \\ 3 = x\)
9) 12x - 10 is supplementary to 8x - 10. So the equation would be: \(12 x- 10 + 8x - 10 = 180\)
☆Let's solve it!
\(12x - 10 + 8x - 10 = 180 \\ 20x - 20 = 180 \\ \frac{ \: \: \: \: \: \: \: \: \: \: + 20 = + 20}{ \frac{20x}{20} = \frac{200}{20} } \\ x = 10\)
Use the relationship between the angles in the figure to answer the question.
Which equation can be used to find the value of x?
Drag and drop the equation into the box.
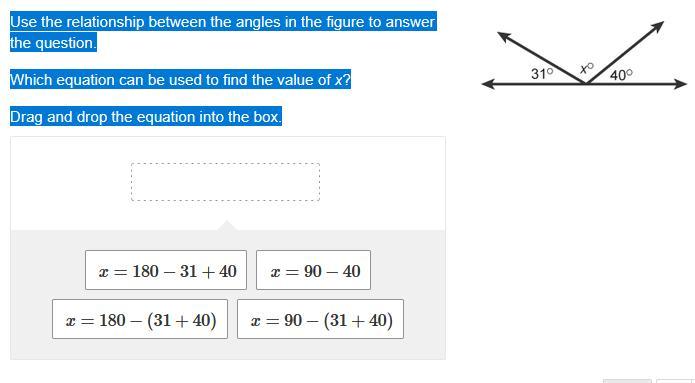
Answers
Answer:
x= 180 - 31 + 40
Step-by-step explanation:
Answer:
Its the first one X = 180 - 31 + 40 :))
Please help me with the question please ASAP ASAP

Answers
Answer:
It's a rectangle
so, m∠2= 90-62=28°
Step-by-step explanation:
Answer: All im gonna say is you can take out 28 and 118 because there is nothing you can do correctly to get those as your answer.
Evaluate a + 4 when a=7
Answers
Because:
(7) + 4 = 11
please help will give brainliest

Answers
Answer:
First option: 37°, 11°, 132°
Step-by-step explanation:
The sum of the three interior angles of a triangle must add up to 180°
Only the first option 37°, 11° and 132° add up to 180°
37 + 11 + 132 = 48 + 132 = 180°
You don't need to add up all the other sets of angles completely. Just look at the units place
Option 2, units sum = 4 + 6 + 1 =11; does not end in a 0, ends in 1
Option 3: units sum = 1 + 0 + 2; does not end in a 0, ends in 3
Option 4: units sum = 0 + 1 + 5 ends in a 6
I NEED HELP FAST PLEASE HELP ME
Select the correct answer.
Which stem and leaf plot represents the given data set?
57,28,15,20,21,13,36
42,14,52,15,29,29,13,57

Answers
The Fibonacci sequence 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, … is formed by summing two consecutive numbers to get the next number. The number after 55 is 34 + 55 = 89. A Fibonacci game cube has a different Fibonacci number on each face selected from the set {1, 2, 3, 5, 8, 13}. One red and one blue Fibonacci game cube are tossed together. How many of the 36 possible outcomes show a pair of numbers on the tops of the cubes whose sum is also a Fibonacci number?
Answers
===================================================
Explanation:
The possible Fibonacci sums are:
1+1 = 21+2 = 32+3 = 53+5 = 85+8 = 138+13 = 21Look at the chart below and highlight when those sums occur.
I've highlighted them in green. A fairly interesting pattern forms. There are two instances of each sum in the set {3,5,8,13,21}. That set has 5 items. The sum "2" only shows up once.
So we have 2*5+1 = 11 Fibonacci sums overall.

a box is constructed out of two different types of metal the mtal for the top and bottom which are both squares costs 1/ft2 and the metal for the sides costs 2/ft2 find the dimensions that minimize cost if the box has a volume of 20
Answers
There are no dimensions that minimize the cost of the box while maintaining a volume of 20.
To find the dimensions that minimize cost, we need to consider the relationship between the volume of the box and the cost of the materials used.
Let's start by determining the dimensions of the box. We know that the box has a volume of 20. Since the box is constructed out of two different types of metal, we can divide the box into two parts: the top and bottom, which are both squares, and the sides.
Let's assume the length of each side of the top and bottom squares is x. Therefore, the area of each square is x * x = x^2.
The height of the box can be represented by h.
Since the box has a volume of 20, we can set up an equation:
x^2 * h = 20
Now, let's determine the cost of the materials used.
The metal for the top and bottom squares costs 1/ft^2, so the cost for each square is x^2 * (1/ft^2) = x^2/ft^2.
The metal for the sides costs 2/ft^2, so the cost for the sides is 4 * (x * h) * (2/ft^2) = 8xh/ft^2.
The total cost of the materials is the sum of the cost for the top and bottom squares and the cost for the sides:
Cost = (x^2/ft^2) + (8xh/ft^2)
To minimize the cost, we can differentiate the cost function with respect to x and h, and then set the derivatives equal to zero:
dCost/dx = 2x/ft^2 + 8h/ft^2 = 0 (equation 1)
dCost/dh = 8x/ft^2 = 0 (equation 2)
From equation 2, we can see that x = 0 is not a valid solution since it represents a box with zero dimensions. Therefore, x ≠ 0.
From equation 1, we can solve for h:
2x/ft^2 + 8h/ft^2 = 0
8h/ft^2 = -2x/ft^2
h = -2x/8
Since h represents the height of the box, it cannot be negative. Therefore, h ≠ -2x/8.
To find the valid values of x and h, we can substitute the value of h into the equation for the volume:
x^2 * (-2x/8) = 20
Simplifying this equation gives:
-2x^3/8 = 20
-2x^3 = 160
x^3 = -80
Since we're looking for dimensions, x cannot be negative. Therefore, there are no valid values of x and h that satisfy the equation for the volume.
In conclusion, there are no dimensions that minimize the cost of the box while maintaining a volume of 20.
To know more about volume refer here:
https://brainly.com/question/32714484
#SPJ11
Compare the triangles and determine whether they can be proven congruent by SSS, SAS, ASA, AAS, or HL. If not, type "NONE".

Answers
Answer: HL
Step-by-step explanation:
The hypotenuse is actually a shared side of the two triangles. (The line segment that crosses the middle of the rectangle) The hypotenuse is the side of a triangle opposite the 90 degree angle. We can use this for hypotenuse congruency. The diagram also shows the shorter legs of the two triangles are congruent. (The legs refer to the sides that make the 90 degree angle)
So we have hypotenuse congruency and leg congruency.
=HL
Write the equation in slope intercept steps by steps
-5x+y=2
Answers
The equation -5x + y = 2 in slope-intercept form is y = 5x + 2.
What is slope intercept?The line with m as the slope, m and c as the y-intercept is the graph of the linear equation y = mx + c. The values of m and c are real integers in the slope-intercept form of the linear equation.
To write these equations in slope-intercept form, we need to isolate y on one side of the equation.
-5x + y = 2
Adding 5x to both sides, we get:
y = 5x + 2
Therefore, the equation -5x + y = 2 in slope-intercept form is y = 5x + 2.
Learn more about slope intercept on:
brainly.com/question/9317111
#SPJ9
Consider an experiment where a coin is tossed repeatedly until the first time a head is observed. a) What is the sample space for this experiment? What is the probability that the coin turns up heads after i tosses? b) Let E be the event that the first time a head turns up is after an even number of tosses. What set of outcomes belong to this event? What is the probability that E occurs?
Answers
a) The sample space for this experiment is {H, TH, TTH, ... so on} . The probability that the coin turns up heads after i tosses is (1/2)^i for i = 1, 2, 3, and so on.
b) Let E be the event that the first time a head turns up is after an even number of tosses, the set of outcomes belong to this event is {TH, TTTH, TTTTTH, ..... so on}. the probability that event E occurs is 1/3.
a) The sample space for this experiment consists of all possible sequences of tosses that end in a head. This includes the sequence "H" (which is the outcome when the first toss is a head), the sequence "TH" (which is the outcome when the first toss is a tail followed by a head), the sequence "TTH" (which is the outcome when the first two tosses are tails followed by a head), and so on.
The sample space is infinite because there is no limit to the number of tosses that could occur before a head is observed.
The probability that the coin turns up heads after i tosses is (1/2)^i for i = 1, 2, 3, and so on. This is because the probability of getting a head on any given toss is 1/2, and the tosses are independent of each other.
Therefore, the probability of getting a head on the first toss is 1/2, the probability of getting a head on the second toss is (1/2)(1/2) = 1/4, the probability of getting a head on the third toss is (1/2)(1/2)*(1/2) = 1/8, and so on.
b) The set of outcomes that belong to the event E consists of all sequences of tosses that end in a head and where the number of tosses before the first head is even. This includes the sequence "TH" (which has one even-numbered toss before the head), the sequence "TTTH" (which has three even-numbered tosses before the head), and so on.
The probability that event E occurs is given by the sum of the probabilities of all the sequences in E. To calculate this, we can use the formula for an infinite geometric series, which is:
sum = a/(1-r)
where a is the first term of the series, r is the common ratio, and sum is the sum of the series. In this case, a = (1/2)^2 = 1/4 (since the first even-numbered toss occurs on the second toss), and r = (1/2)^2 = 1/4 (since each subsequent even-numbered toss has probability (1/2)^2 of occurring).
Therefore, the sum of the probabilities of all the sequences in E is:
sum = (1/4)/(1-1/4) = (1/4)/(3/4) = 1/3
So the probability that event E occurs is 1/3.
To learn more about probability click on,
https://brainly.com/question/15290040
#SPJ4
A retailer paid $33.00 for a lamp. What price should she sell the lamp for if she wants to make a 35% profit?
Answers
Answer: $44.55
Step-by-step explanation:
She should sell if for $33.00 x 1.35 = $44.55.
in a sample of n=23, the critical value of the correlation coefficient for a two-tailed test at alpha =.05 is
A. Plus/minus .497
B. Plus/minus .500
C. Plus/minus .524
D. Plus/minus .412
Answers
The critical value of the correlation coefficient for a two-tailed test at alpha = 0.05 with a sample size of n = 23 is approximately plus/minus 0.497.
To understand why this is the case, we need to consider the distribution of the correlation coefficient, which follows a t-distribution. In a two-tailed test, we divide the significance level (alpha) equally between the two tails of the distribution. Since alpha = 0.05, we allocate 0.025 to each tail.
With a sample size of n = 23, we need to find the critical t-value that corresponds to a cumulative probability of 0.025 in both tails. Using a t-distribution table or statistical software, we find that the critical t-value is approximately 2.069.
Since the correlation coefficient is a standardized measure, we divide the critical t-value by the square root of the degrees of freedom, which is n - 2. In this case, n - 2 = 23 - 2 = 21.
Hence, the critical value of the correlation coefficient is approximately 2.069 / √21 ≈ 0.497.
Therefore, the correct answer is A. Plus/minus 0.497.
Learn more about correlation coefficient here:
brainly.com/question/29978658
#SPJ11
A paper company needs to ship paper to a large printing business. The paper will be
shipped in small boxes and large boxes. The volume of each small box is 6 cubic feet
and the volume of each large box is 13 cubic feet. A total of 19 boxes of paper were
shipped with a combined volume of 156 cubic feet. Determine the number of small
boxes shipped and the number of large boxes shipped.
There were
Submit Answer
small boxes shipped and
large boxes shipped.

Answers
There were 13 small boxes shipped and 6 large boxes shipped.
Let's denote the number of small boxes as 's' and the number of large boxes as 'l'.
According to the given information, the volume of each small box is 6 cubic feet, so the total volume of all small boxes can be calculated as 6s cubic feet.
Similarly, the volume of each large box is 13 cubic feet, so the total volume of all large boxes can be calculated as 13l cubic feet.
We are also given that the combined volume of all the boxes is 156 cubic feet, so we can write the equation:
6s + 13l = 156 ...(1)
Additionally, we know that a total of 19 boxes were shipped, so we can write another equation:
s + l = 19 ...(2)
Now, we have a system of equations (equation 1 and equation 2) that we can solve simultaneously to find the values of 's' and 'l'.
To solve this system of equations, we can use substitution or elimination method. Let's use the substitution method here.
From equation 2, we can rewrite it as s = 19 - l.
Substituting this value of s into equation 1, we get:
6(19 - l) + 13l = 156
Simplifying the equation:
114 - 6l + 13l = 156
Combining like terms:
7l = 42
Dividing both sides by 7:
l = 6
Now, we can substitute this value of l back into equation 2 to find the value of s:
s + 6 = 19
s = 13
The number of small boxes shipped is 13, and the number of large boxes shipped is 6.
There were 13 small boxes shipped and 6 large boxes shipped.
For more questions on shipped
https://brainly.com/question/29352184
#SPJ8
These salsas are made for a competition.
On Fire!
2 tablespoons
peppers
5 tablespoons tomatoes
Blazin'
4 tablespoons peppers
12 tablespoons tomatoes
Kickin'
6 tablespoons peppers
15 tablespoons tomatoes
Feel the Burn
3 tablespoons peppers
6 tablespoons tomatoes
If peppers and tomatoes are the only ingredients in the salsas
and the names are based on taste, which two salsas should have the same name?
Answers
Answer:
Step-by-step explanation:
On Fire! and Kickin' should have the same name. Both salsas have 2:5 ratio of peppers to tomatoes. Blazin' has a ratio of 1:3 and Feel the Burn has a ratio of 1:2.
9,369 divided 53 help please

Answers
Answer:
the answer is
\(176 \times \frac{41}{53} \)
hope this helps
between 1/4 aand 9/2 which fraction is greater
Answers
Answer: the answer is 9/2