A box is 20 cm long. Which of these is closest to the length of this box in feet?
Answers
Answer: 0.66 Feet
Step-by-step explanation: There are 2.54 centimeters in an inch and there are 12 inches in a foot.
Please mark me as brainliest!
Related Questions
There is an ice cream cake in the freezer. On Monday, the family eats 1/2 of
the cake. On Wednesday, they eat 1/4 of the cake. How much did the family
eat altogether?
Answers
Answer: 2/4
Step-by-step explanation: Easy 2 + 2 = 4
A car can travel 64 mlles in two hours, 128 miles in four hours, and 192 mlles In six hours. What is the constant rate of change?
Answers
Answer:
1 hour/ 32 miles
Step-by-step explanation:
Given the absolute error for each observation, calculate MSE. Observation Absolute Error 2.00 1 2. 5.80 3 2.22 4 6.00 5 7.40 6 2.66 Correct! 23.40 6,60 33.64 4.35
Answers
The Mean Squared Error is approximately 22.734. It is the average squared difference between the predicted values and the actual values in a dataset.
Mean Squared Error (MSE) is a common metric used to measure the accuracy of a statistical model or predictor.
To calculate the Mean Squared Error (MSE), we first need to square the absolute errors and then take the average of the squared errors.
The squared errors for each observation are:
\(1^2 = 1\\5.8^2 = 33.64\\2.22^2 = 4.9284\\6^2 = 36\\7.4^2 = 54.76\\2.66^2 = 7.0756\)
To find the MSE, we add up the squared errors and divide by the number of observations:
MSE = (1 + 33.64 + 4.9284 + 36 + 54.76 + 7.0756) / 6
= 136.404 / 6
= 22.734
Therefore, the Mean Squared Error is approximately 22.734.
To learn more about Mean Squared Error visit;
https://brainly.com/question/29662026
#SPJ4
Could someone explain the answer in step by step
\((5^2*2^4)^2(5^{-2}*2^5)\\Answer: 2^{13} * 5^4\)
Answers
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️
\(( { {5}^{2} \times {2}^{4} })^{2} ( {5}^{ - 2} \times {2}^{5} ) = \)
\( {5}^{2 \times 2} \times {2}^{4 \times 2} \times {5}^{ - 2} \times {2}^{5} = \)
\( {5}^{4} \times {2}^{8} \times {5}^{ - 2} \times {2}^{5} = \)
\( {5}^{4} \times {5}^{ - 2} \times {2}^{8} \times {2}^{5} = \)
\( {5}^{4 + ( - 2)} \times {2}^{8 + 5} = \)
\( {5}^{4 - 2} \times {2}^{13} = \)
\( {5}^{2} \times {2}^{13} = \)
\( {2}^{13} \times {5}^{2} \)
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️
Your backpacker's guide contains a grid map of London, with each unit on the grid representing 0.125 kilometers. If Buckingham Palace is located at (2, -3) and Westminster Abbey is located at (-1, 4), what is the direct distance (not walking distance, which would have to account for bridges and roadways) between the two landmarks in kilometers? Round your answer to two decimal places, if necessary.
Answers
Answer:
~ .95 km
Step-by-step explanation:
Use distance formula to find the distance between the two points then multiply by .125 km
d^2 = ( 2- -1)^2 + ( -3 -4)^2
d^2 = 9 + 49
d^2 = 58
d = sqrt 58
sqrt 58 * .125 km = .952 km ~ .95 km
If the area of each of the triangular regions is as shown, what is the area of the polygon?
18
17
16

Answers
Answer:
18
Step-by-step explanation:
The city planning commission has been given a budget of 300,000 if the density of concrete is 150 lbs/ft3 determine if the cpc can afford to buy the bridge. The cost of concrete is 0.80/lb
Answers
Answer:
Check Explanation
Step-by-step explanation:
The volume of concrete required to build the bridge is missing and should be provided.
In its absence, let us assign a letter to it.
Let the volume of concrete required to build the bridge be V ft³.
And we further assume that the concrete is the only major material needed to build the bridge for the sake of our question.
Density = (mass/volume)
Density = 150 lb/ft³
Mass = ?
Volume = V ft³
Mass = (Density) × (Volume)
Mass of concrete required = 150 × V = (150V) lb
Cost of concrete is 0.80 per lb
1 lb of concrete costs 0.80
150V of concrete will cost 150V × 0.80 = (120V) currency amount.
Now, depending on the value of V, we can now determine if the city planning commission (cpc) can afford the bridge.
If 120V > 300,000 budget, the cpc cannot afford the bridge, but if 300,000 budget > 120V, then the cpc can afford the bridge.
Hope this Helps!!!
1. Find the scale factor from the large figure to the small figure.
2. Find the scale factor from the small figure to the large figure.

Answers
Answer:
sorry I can see the whole answer on the image
State where in the ty-plane the hypotheses of the Existence and Uniqueness Theorem are satisfied for the equation y'=(ycot(2t))/(t^2+y^2+1)
Answers
We can conclude that the hypotheses of the Existence and Uniqueness Theorem are satisfied in any rectangular region in the ty-plane that does not contain the curve t² + y² = -1.
Where in the ty-plane the hypotheses of the existence and uniqueness theorem are satisfiedThe Existence and Uniqueness Theorem for first-order ordinary differential equations states that if a differential equation of the form y' = f(t, y) satisfies the following conditions in some rectangular region in the ty-plane:
1. f(t, y) is continuous in the region.
2. f(t, y) satisfies a Lipschitz condition in y in the region, i.e., there exists a constant L > 0 such that |f(t, y₁) - f(t, y₂)| ≤ L|y₁ - y₂| for all t and y₁, y₂ in the region.
then there exists a unique solution to the differential equation that passes through any point in the region.
In the case of the differential equation y' = (y cot(2t)) / (t² + y² + 1), we have:
f(t, y) = (y cot(2t)) / (t² + y² + 1)
This function is continuous everywhere except at the points where t² + y² + 1 = 0, which is the curve t² + y² = -1 in the ty-plane. Since this curve is not included in any rectangular region, we can say that f(t, y) is continuous in any rectangular region in the ty-plane.
To check if f(t, y) satisfies a Lipschitz condition in y, we can take the partial derivative of f with respect to y and check if it is bounded in any rectangular region. We have:
∂f/∂y = cot(2t) / (t² + y² + 1) - (2y² cot(2t)) / (t² + y² + 1)²
Taking the absolute value and simplifying, we get:
|∂f/∂y| = |cot(2t) / (t² + y² + 1) - (2y² cot(2t)) / (t² + y² + 1)²|
= |cot(2t) / (t² + y² + 1)| * |1 - (2y² / (t² + y² + 1)))|
Since 0 ≤ (2y² / (t² + y² + 1)) ≤ 1 for all t and y, we have:
1/2 ≤ |1 - (2y² / (t² + y² + 1)))| ≤ 1
Also, cot(2t) is bounded in any rectangular region that does not contain the points where cot(2t) is undefined (i.e., where t = (k + 1/2)π for some integer k). Therefore, we can find a constant L > 0 such that |∂f/∂y| ≤ L for all t and y in any rectangular region that does not contain the curve t² + y² = -1.
Learn more on differential equation here;
https://brainly.com/question/1164377
#SPJ1
Is the data Jamaya collected a random sample if the population is books from her entire household? Why or why not? *Remember that Jamaya picked 15 books from her book shelf in her bedroom.
Answers
Using sampling concepts, it is found that it is not a random sample, as it considers only the books in her bedroom, hence books from other people in the household are likely to not be considered.
What is sampling?It is a common statistics practice, when we want to study something from a population, we find a sample of this population, which has to involve all groups in the population.
In this problem, a random sample should involve the books of all people in the household, not only Jamaya's. Hence, it is not a random sample, as it considers only the books in her bedroom, hence books from other people in the household are likely to not be considered.
More can be learned about sampling concepts at https://brainly.com/question/25122507
45 percent of consumers prefer to purchase electronics online. You randomly select 8 consumers. Find the probability that the number of consumers who prefer to purchase electronics online is (a) exactly five, (b) more than five, and (c) at most five.
Answers
a) The probability that exactly five consumers prefer to purchase electronics online is: 0.2604.
b) The probability that more than five consumers prefer to purchase electronics online is: 0.3624.
c) The probability that at most five consumers prefer to purchase electronics online is: 0.6376.
How to solve Binomial Probability Distribution Problems?(a) The binomial probability formula is expressed as:
P(X = k) = (\(.^{n} C_{k}\)) * \(p^{k}\) * \((1 - p)^{n - k}\)
where:
n = number of trials
k = number of successes
p = probability of success
We are given:
n = 8
k = 5
p = 0.45
Thus,
P(X = 5) = (8C5) * (0.45)⁵ * (1 - 0.45)⁽⁸⁻⁵⁾
P(X = 5) ≈ 0.2604
(b) To find the probability that more than five consumers prefer to purchase electronics online is:
P(X > 5) = P(X = 6) + P(X = 7) + P(X = 8)
P(X > 5) = [(⁸C₆) * (0.45)⁶ * (0.55)²] + [(⁸C₇) * (0.45)⁷ * (0.55)¹] + [(⁸C₈) * (0.45)⁸ * (0.55)⁰]
P(X > 5) ≈ 0.3624
(c) To find the probability that at most five consumers prefer to purchase electronics online is:
P(X ≤ 5) = 1 - P(X > 5)
P(X ≤ 5) ≈ 1 - 0.3624
P(X ≤ 5) ≈ 0.6376
Read more about Binomial Probability Distribution at: https://brainly.com/question/15278907
#SPJ1
Solve for x: 3 < x + 3 < 6
6 < x < 9
6 > x > 9
0 < x < 3
0 > x > 3
Answers
Answer:
Subtract - 3 in each term
3 - 3 < x+3 - 3 < 6- 3
0 < x < 3
Find the length of a side of a square whose diago- nal is 16 cm long. Round your answer to the nearest tenth.
Answers
Answer:
11.3 cm
Step-by-step explanation:
(see attached for reference)
using the Pythagorean theorem
hypotenuse ² = length ² + length ²
16² = L² + L²
16² = 2L² (express 2 = (√2)²
16² = (√2)²L²
16² = (√2L)²
16 = √2L
L = 16 /√2
L = 11.3 cm

11.3
use Pythagoras theorem give each side is "a"
a^2+a^2=16^2
2*a^2=256
a^2=256/2=128
a=sqrt(128)=11.3 sqrt=square root
Can anyone help me plz?

Answers
Answer:
\( y = \frac{1}{4}x + 1 \)
Step-by-step explanation:
Line is passing through the points (0, 1) & (4, 2)
Slope of line = (2 - 1)/(4 - 0) = 1/4
y-intercept (b) = 1
Equation of line in slope-intercept form is given as:
\(y = mx + b \\ \\ y = \frac{1}{4}x + 1 \)
Answer:
\(y = \frac{1}{4}x + 1\)
Step-by-step explanation:
Slope intercept form allows one to write an equation for a line from only the y-intercept and the slope, so we need to identify those two things.
The slope is simply \(\frac{y_2 - y_1}{x_2-x_1}\)=>\(\frac{2-1}{4-0}\)=> 1/4
The slope intercept is the y value when x = 0, and since we have the point (0,1), the y-intercept must be 1
The equation is: y = mx + b where m is the slope and b is the intercept so the final equation is:
\(y = \frac{1}{4}x + 1\)
What’s the answer please

Answers
Answer:
40
Step-by-step explanation:
plugging in a and b will get us:
\(2^{2} + 6^{2} = c^{2}\)
4 + 36 = \(c^{2}\)
40 = \(c^{2}\)
c= \(\sqrt{40\\\)
(7y + 5) - 2(4y - 3)
Answers
Answer:
-y+11
Step-by-step explanation:
Answer:-1y + 11
Step-by-step explanation:
7y + 5 - 8y + 6
-simplify
7y - 8y = -1y
5+6=11
= -1y + 11
write and solve an equation to find the value of x

Answers
Answer:
x=5
Step-by-step explanation:
30 = (12*x)/2
60 = 12*x
60/12 = (12*x)/12
5=x
Please help me ASAP!

Answers
Answer:
y = 6.5
Step-by-step explanation:
When triangles are similar, we can set up a proportion to compare the sides. I will have the "left" side on the bottom of the fractions, and the side I am solving for on the top.
\(\frac{y}{6} =\frac{13}{12}\)
[Cross multiply]
12y = 78
y = 6.5
Have a nice day!
I hope this is what you are looking for, but if not - comment! I will edit and update my answer accordingly.
- Heather
help!! i’ve been stuck on this problem!
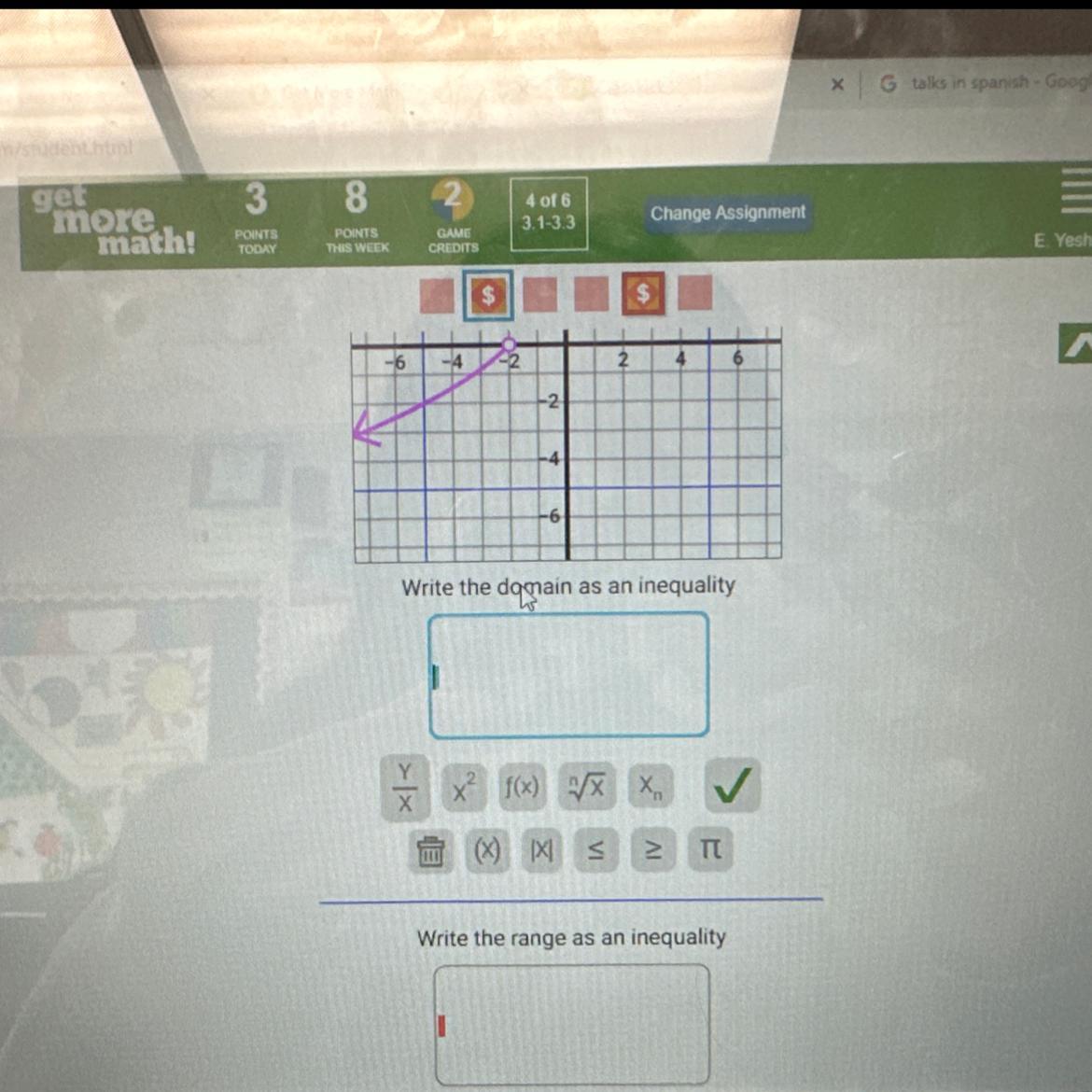
Answers
Answer:
x less than or equal to -2
Step-by-step explanation:
I believe that's the answer
Answer:
x < -2
Step-by-step explanation:
You want the domain of a function graphed as a curve with an open circle at x=-2, which is the right end of the curve.
DomainThe domain is the horizontal extent of the graph. It is the set of values for which the relation is defined.
ApplicationThe open circle at (x, y) = (-2, 0) means that point is not part of the graph. All x-values to the left of that point have corresponding y-values, so the extent of the graph can be said to be ...
x < -2
The domain of the relation is x < -2.
please help.........

Answers
y-intercept = 2
50 Points! Multiple choice algebra question. Photo attached. Thank you!

Answers
Using the formula of margin of error, the margin of error on this data is 6.7%
What is the margin of error?The formula for the margin of error is calculated by multiplying a critical factor (for a certain confidence level) with the population standard deviation. Then the result is divided by the square root of the number of observations in the sample.
The formula is given as;
\(MOE = Z*\sqrt{\frac{p(1 - p)}{n} }\)
where:
Z is the Z-score for the desired confidence levelp is the percentage of students who will vote for Candidate Bn is the sample sizeIn this case, the Z-score for a 95% confidence level is 1.96, p is 0.38, and n is 220.
Let substitute the values and solve;
\(MOE = 1.96*\sqrt{\frac{0.38(1 - 0.38)}{220} } \\MOE = 0.067\)
Converting this into percentage;
The margin of error is 6.7%
Learn more on margin of error here;
https://brainly.com/question/29849722
#SPJ1
Can you guys help me on this question 4 + 5y - 6y + y
Answers
Answer:
4
Step-by-step explanation:
You combine like terms first:
5y-6y+1y=0
Then you also have a 4.
4+0=4
Hope it helps!:)
I need help, I know it gave me the answer but I don’t understand how it got (-3) someone explain please

Answers
Answer: h=14
Step-by-step explanation:
If x=-18, then
h=17+(-18)/6
h=17+(-3)
h=17-3
h=14
Answer:
Step-by-step explanation:
so, the calculation was : h = 17 + \(\frac{-18}{6\\}\)
To subtract "\(\frac{-18}{6}\) " we need to make it the same denominator as "17"
(it's not written but technically 17 = \(\frac{17}{1}\) )
Yet, we see that \(\frac{-18}{6} =\frac{-3* 6}{1*6}\)
We just need to remove both the 6 that are not useful here and :
\(\frac{-3*6}{1*6} =\frac{-3}{1}= -3\)
We obtain h = 17 - 3 = 14
hope it helped :)
Mr. Silvano drove 128.6 km each day for 8 days. Then, he drove 44.3 km each day for 12 days. What was the total distance Mr. Silvano drove?
Answers
Answer:
Ans: 1559.6km
Step-by-step explanation:
(128.6x8)+(44.3x12)=1559.6km
Answer:1559.6km
Step-by-step explanation:
128.6*8=1,028.8
44.3*12=531.6
1,028+531.6=1559.6km
A person with a height of 2 meters stand near a tree. The tree casts a shadow that is 30 meters long and the person has a shadow that is 3
meters long. What is the height of tree

Answers
9514 1404 393
Answer:
20 m
Step-by-step explanation:
The 30 m shadow of the tree is 10 times the 3 m length of the person's shadow. We expect the tree to be 10 times as tall as the person:
10(2 m) = 20 m . . . height of the tree
For the statement, find the constant of variation and the variation equation.
y varies directly as the cube of x; y = 36 when x = 6
Answers
The constant of variation is 1/6 and variation equation is y=(1/6)*\(x^{3}\) .
This is a question of direct variation .
⇒ y is directly proportional to x .
Since y varies directly as the cube of x .
Let k be the constant of variation .
y = k\(x^{3}\) eq1
Substituting values of y and x in the above quadratic equation
36 = k\(6^{3}\)
k = 36/216
k= 1/6
Hence , constant of variation = 1/6 .
Variation Equation is made by substituting value of Constant of Variation in above equation .
Variation equation is
y=(1/6)*\(x^{3}\)
Therefore , constant of variation = 1/6 and variation equation is
y=(1/6)*\(x^{3}\) .
To learn more about variation equation visit the link :
https://brainly.com/question/13998680
#SPJ9
A couple plans to purchase a house. The bank requires a 20% down payment on the $240,000 house. The couple will finance the rest of the cost with a fixed- rate mortgage at 8.5% annual interest with monthly payments over 30 years.
Complete the parts below. Do not round any intermediate computations. Round your final answers to the nearest cent if necessary. If necessary, refer to the list of financial formulas.
(a) Find the required down payment.
(b) Find the amount of the mortgage.
(c) Find the monthly payment.

Answers
(A) The required down payment is $48,000.
(B) The amount of the mortgage is $192,000.
(C) Monthly payment = $192,000 * (0.085/12) * (1 + (0.085/12))^(3012) / (((1 + (0.085/12))^(3012)) - 1)
(a) To find the required down payment, we need to calculate 20% of the house price.
Down payment = 20% of $240,000
Down payment = 0.2 * $240,000
Down payment = $48,000
The required down payment is $48,000.
(b) The amount of the mortgage is equal to the total cost of the house minus the down payment.
Mortgage amount = Total cost of the house - Down payment
Mortgage amount = $240,000 - $48,000
Mortgage amount = $192,000
The amount of the mortgage is $192,000.
(c) To find the monthly payment for the mortgage, we can use the formula for the monthly payment on a fixed-rate mortgage:
Monthly payment = P * r * (1 + r)^n / ((1 + r)^n - 1)
Where:
P = Principal amount (mortgage amount)
r = Monthly interest rate (8.5% annual interest divided by 12 months and converted to a decimal)
n = Total number of monthly payments (30 years multiplied by 12 months)
Monthly payment = $192,000 * (0.085/12) * (1 + (0.085/12))^(3012) / (((1 + (0.085/12))^(3012)) - 1)
Using this formula and performing the calculation will give you the monthly payment amount.
for more questions on down payment
https://brainly.com/question/1698287
#SPJ8
The second of two numbers is five more than twice the first. There sum is 80 . Find the numbers.
Answers
The first number = x
The second number = 5 + 2x
80 = x + 5 + 2x
Let's combine like terms.
80 = 3x + 5
Subtract 5 from both sides.
75 = 3x
Divide both sides by 3
25 = x
The first number = 25
Let's plug 25 into x for our second number's equation.
The second number = 5 + 2(25) = 55
Answer:
x = first number
y = second number
1. y = 2x +5
2. x = x
3. x + 2x + 5 = 80
4. 3x +5 = 80
5. 3x = 75
x = 25
sub back in to steps 1 and 2:
x = 25
y = 2(25) + 5
y = 55
the first number is 25, and the second number is 55.
CHECK:
80 - 25 = 55
Step-by-step explanation:
1. define your variables
2. use the question to find what each number equals
3. set them equal to 80
4. combine like terms, divide, etc. (SOLVE)
5. sub back into defined variables.
6. check it by subtracting and adding
Select the correct answer. A company manufactures computers. Function N represents the number of components that a new employee can assemble per day. Function E represents the number of components that an experienced employee can assemble per day. In both functions, t represents the number of hours worked in one day. Which function describes the difference of the number of components assembled per day by the experienced and new employees? A. B. C. D.
Answers
The function which describes the difference of the number of computer components assembled per day by the experienced and new is D(t)=10t(2t+13)/[(t+4)(t+3)].
What is a Function?A function assigns the value of each element of one set to the other specific element of another set.
Function N represents the number of components that a new employee can assemble per day.
N(t)=(50t)/(t+4)
Function E represents the number of components that an experienced employee can assemble per day.
E(t)=(70t)/(t+3)
In both functions, t represents the number of hours worked in one day. The function which represent the difference of the number of components assembled per day by the experienced and new employees is,
\(D(t)=\dfrac{70t}{t+3}-\dfrac{50t}{t+4}\\D(t)=\dfrac{70t(t+2)-50t(t+3)}{(t+4)(t+3)}\\D(t)=\dfrac{10t(7(t+4)-5(t+3))}{(t+4)(t+3)}\\D(t)=\dfrac{10t(7t+28-5t-15)}{(t+4)(t+3)}\\D(t)=\dfrac{10t(2t+13)}{(t+4)(t+3)}\\\)
Thus, the function which describes the difference of the number of computer components assembled per day by the experienced and new is D(t)=10t(2t+13)/[(t+4)(t+3)].
Learn more about Function here:
https://brainly.com/question/5245372
#SPJ1
Answer:
D(t)= 10t(2t+13)/ (t+4)(t+3)
Step-by-step explanation:
Ramon knows there are 60 seconds in 1 minute. He writes the equation 60s=m to find the number of minutes, m, in any number of seconds, s.
Use the drop-down menus to complete the statements about Ramon's equation.
Answers
Answer:
60 seconds is 1 minute, let's say for example you wanna find how many seconds is 3 minutes. So you gotta take 60(3) = 180m. :)
Step-by-step explanation: