A circular table top has a radius of 90 cm. A force of 810 N is applied to the whole table top. Calculate the pressure in N/m2 to 4sf.
Answers
The pressure of the circular table top has a radius of 90 cm. A force of 810 N is applied to the whole table top = 287.23 N/m².
The force defined as:A force seems to be an influence that could also cause an object's motion to change. A force can cause a mass item's velocity or acceleration to alter. The force can be expressed intuitively as a push or perhaps a pull. Because it has both magnitude and direction a force is a vector quantity.
What exactly do you mean by pressure?The force applied against the surface of an item per unit area across which that force is spread is referred to as pressure (symbol: p or P).
According to the given data:Force = 810N
Radius of the circular table = 90cm.
Pressures = force /area
Finding the area of circular table = πr²
= π x 90 x 10⁻² (1 cm = 10⁻²)
= 2.82 m²
substituting the value in the formula.
Pressures = force /area
= 810/2.82
= 287.23 N/m²
The pressure of the circular table top has a radius of 90 cm. A force of 810 N is applied to the whole table top = 287.23 N/m²
To know more about force visit:
https://brainly.com/question/13191643
#SPJ9
Related Questions
please help first will be brainliest

Answers
Answer:
Just multiply or divide the numbers they give you try both
Step-by-step explanation:
Factor each completely.
5n^2 + 19n + 12
Answers
Answer:
Factor by grouping, (5n+4)(n+3), or alternatively, (n+3)(5n+4)
Answer:
(n +3)(5n + 4)
Step-by-step explanation:
5n^2 + 19n + 12
= 5n^2 + 15n + 4n + 12
= 5n(n + 3) + 4(n + 3)
= (n +3)(5n + 4)
Hence Factorized.
Unit:transformations homework 5 identifying transformations
Answers
The transformation given as A(-8,7) → A'(-8,-7) represents a reflection over x-axis .
In the reflection of the point (x,y) over x-axis transformation ,the x-coordinate of each point remains the same, but the y-coordinate is negated (changes sign).
Let's consider the coordinates of point A that is (-8, 7).
The transformation takes this point to A' (-8, -7).
We can see that the x-coordinate of the point remains the same which is -8. but , the y-coordinate changes from 7 to -7.
We know that in a reflection over the x-axis, the x-coordinate remains the same while the y-coordinate changes sign.
Therefore , the transformation that takes A to A' is a reflection over the x-axis.
Learn more about Transformations here
https://brainly.com/question/29208647
#SPJ4
The given question is incomplete , the complete question is
Identify the Transformation given as A(-8,7) → A'(-8,-7)?
The graph of line l is shown

Answers
Answer:
The general equation of a straight line is y = mx + c, where m is the gradient, and y = c is the value where the line cuts the y-axis. This number c is called the intercept on the y-axis. The equation of a straight line with gradient m and intercept c on the y-axis is y = mx + c.
Step-by-step explanation:
your welcome
Complete the explanation of what happens when you round 6.992 to the nearest tenth.
Since there is a _______-
in the hundredths place, the number in the tenths place will
________
. Since 9 + 1 = 10, a
____
will go in the tenths place and the
______
will be added to the 6 in the ones place making the answer _______
Please answer quickly!
Answers
Answer:
1. 9
2. increase
3. 1
4. 1
5. 7
There are 2 gyms. One, Buff Billy’s, has a joining cost of $80 and charges $25 a month. The other, Shredded Eddie’s, has no joining cost but charges $35 a month. At what month are both gyms the same total cost?
Answers
Answer:
One, Buff Billy's, has a joining cost of $80 and charges $25 a month. The other, Shredded Eddie's, has no joining cost but charges $35 a ...
Step-by-step explanation:
12,10, 70
From least to greatest, the numbers are
, and
8.5
9
9.5
10
105
11.5
12
70
72
10
?
Answers
Answer:
Im not sure if the numbers in the title were included, but the answer would be: 8.5, 9, 9.5, 10, 10, 10, 11.5, 12, 12, 70, 70, 72, 105.
Step-by-step explanation:
please help me with this one

Answers
Answer: Choice D
k < 5Graph has an open hole at 5 and shading to the left============================================================
Explanation:
We solve this as if it were an equation. In other words, we follow the same sort of steps.
To solve k - 3 = 2, we add 3 to both sides getting k = 5
So solving k - 3 < 2 leads to k < 5. The inequality sign does not flip.
To graph k < 5, we will plot an open hole at 5 and shade to the left
The open hole means we do not include 5 as part of the solution set. The graph visually represents all values smaller than 5.
This all leads to choice D being the final answer.
The Call First cell phone company charges $45 per month and an additional $0.24 for each text message sent during the month. Another cell phone company, Cellular Plus, charges $65 per month and an additional $0.23 for each text message sent during the month.
Answers
Answer:
2000
Step-by-step explanation:
Let \(x\) be the total number of text messages sent per month in order for the plans to cost the same each month.
The first cell phone company, The Call, charges $45 per month and $0.24 per text message for the month.
So, the total cost as per the plan of The Call, for x text messages during the month, is
\(C_1= 45+0.24x\cdots(i)\)
Another cell phone company, Cellular Plus, charges $65 per month and $0.23 per text message for the month.
So, the total cost as per the plan of Cellular Plus, for x text messages during the month, is
\(C_2= 65+0.23x\cdots(ii)\)
As the cost for both the companies are the same, so, compute the number of text messages, x, during the month by equation \(C_1\) and \(C_2\) from equation (i) and (ii), we have
\(C_1=C_2\)
\(\Rightarrow 45+0.24x=65+0.23x\)
\(\Rightarrow 0.24x - 0.23x=65-45\)
\(\Rightarrow 0.01 x = 20\)
\(\Rightarrow x = 20/0.01\)
\(\Rightarrow x= 2000\)
Hence, there must be 2000 text message to be sent during the month in order to have the same cost from both the companies.
Part of the population of 7,000 elk at a wildlife preserve is infected with a parasite. A random sample of 50 elk shows that 7 of them are infected. How many elk are likely to be infected?
Answers
Answer:
620
Explanation:
When the sample is given, the number of elk are likely to be infected is to be considered as the 620.
Calculation of the number of elk:
Since the population is 7,750.
The random sample is 50.
So here be like
= 620
hence, When the sample is given, the number of elk are likely to be infected is to be considered as the 620.
Consider the line represented by y+4=2/5 (x−9). Write an equation (in point-slope form) representing a different line with the same slope that passes through the point (3,6).
Answers
The equation of the line, in point-slope form, is: y - 6 = 2/5(x - 3).
What is an Equation of a Line in Point-Slope Form?The point-slope form that an equation of a line can be written in is given as y - b = m(x - a), here:
The coordinate of a point on the line is represented as a and b.
The slope of the line = m.
Given an equation of a line, in point-slope form as y + 4 = 2/5(x - 9), the slope (m) of the line is m = 2/5.
To write the equation of the line that has the same slope (m) and passes through the point (3, 6), substitute m = 2/5 , a = 3, and b = 6 into y - b = m(x - a):
y - 6 = 2/5(x - 3)
Learn more about point-slope equation on:
https://brainly.com/question/24907633
#SPJ1
y = 2/5 x + 24/5 is the equation representing the different line
How to write the equation of a line in point-slope form
Given a line y+4 = 2/5 (x−9)
First, make y the subject:
y+4 = 2/5 (x−9)
y = 2/5 x − 18/5 + 4
y = 2/5 x - 2/5 The slope (m) of this line is 2/5
Note: The general form of the equation of a line is y = mx + c.
Where m is the slope and c is the intercept
For the other line:
Since the other has the same slope with y = 2/5 x - 2/5 and it passes through the point (3,6).
The slope is also 2/5. Since it passes through the point (3,6). That means x = 3 and y = 6.
So put x = 3, y = 6 and m = 2/5 into y = mx + c:
6 = 2/5 x 3 + c
6 = 6/5 + c
c = 6 - 6/5
c = 24/5
Put m = 2/5 and c = 24/5 into y = mx + c:
y = 2/5 x + 24/5
Therefore, the equation representing the different line is y = 2/5 x + 24/5.
Learn more equation of a line on:
https://brainly.com/question/28722124
#SPJ1
Find the area of the sector of a circle that has a central angle of 30 degrees and a radius of 20cm. Round your answer to the nearest hundredth.The area is Answercm^2

Answers
To solve this problem, we will use the following formula for determining the area of a sector:
\(A=\frac{\theta}{360}\times\pi r^2,\)where r is the radius of the circle, and θ is the central angle in degrees.
Substituting r= 20 cm, and θ=30 in the above formula, we get:
\(A=\frac{30}{360}\times\pi(20cm)^2.\)Simplifying, we get:
\(A\approx104.72cm^2.\)Answer:
\(104.72cm^2.\)Find the savings plan balance after 18 months with an apr of 6% and monthly payments of $800. assume an ordinary annuity. a. $15,028.63 c. $15, 360.28 b. $15,280.36 d. $15, 306.82
Answers
The savings plan balance after 18 months will be 15,028.63 dollars.
So the answer is a) $15,028.63
What is the annuity problem?
An annuity is a series of equal payments made at regular intervals.
APR (Annual Percentage Rate) = 6%
Monthly payments = $800
Number of months = 18
We can use the formula for the future value of an annuity (FV) to find the savings plan balance after 18 months. The formula for the future value of an ordinary annuity is:
FV = PMT x (((1 + r)^n - 1) / r)
Where:
FV = future value of the annuity
PMT = the regular payment made
r = the interest rate per period (in this case, the monthly interest rate)
n = the number of payments or periods
The interest rate is given as an annual percentage rate (APR), so we need to divide it by the number of periods per year to find the interest rate per period. In this case, 6% / 12 months/year = 0.5% per month.
FV = 800 x (((1 + 0.005)^18 - 1) / 0.005)
FV = $15,028.63
Hence, the savings plan balance after 18 months will be 15,028.63 dollars.
So the answer is a) $15,028.63
To learn more about the annuity problem, visit:
https://brainly.com/question/25792915
#SPJ4
Answer:
A
Step-by-step explanation:
help plz its due at 12!!
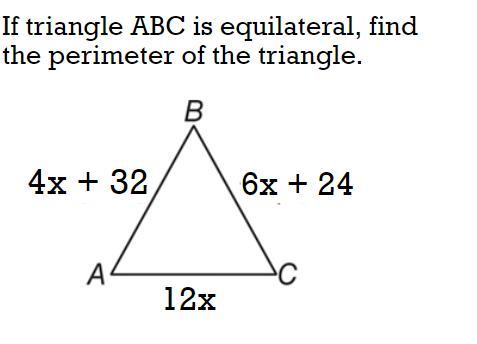
Answers
Answer:
Send this to a Tutor. They will help, and also when asking a tutor make sure you ask them for a backup explanation because they may be wrong
Answer:
144
Step-by-step explanation:
Since the triangle is equilateral all the sides are equivalent, meaning you can make any 2 sides equal to eachother
4x + 32 = 12x
32 = 8x
x=4
Replace the x of one of the sides
12(4) = 48
and since we are finding the perimeter multiply 48 by 3 since there are 3 sides
48(3)
144
Find the distance traveled by a particle with position ( x, y ) as t varies in the given time interval. Compare with the length of the curve.
x=sin^2(theta) , y=cos^2(theta) 0
Answers
The distance traveled is equal to sin^2(θ), as seen before.
To find the distance traveled by a particle with position (x, y) as t varies in the given time interval, we need to first find the parametric equations for x and y in terms of t, and then compute the arc length of the curve.
Given x = sin^2(t) and y = cos^2(t), we first find the derivatives of x and y with respect to t:
dx/dt = 2 * sin(t) * cos(t)
dy/dt = -2 * sin(t) * cos(t)
Next, we compute the square root of the sum of the squares of the derivatives:
sqrt((dx/dt)^2 + (dy/dt)^2) = sqrt((2 * sin(t) * cos(t))^2 + (-2 * sin(t) * cos(t))^2) = sqrt(4 * sin^2(t) * cos^2(t) + 4 * sin^2(t) * cos^2(t)) = 2 * sin(t) * cos(t)
Now, we can find the distance traveled by integrating the above expression with respect to t over the given time interval (0, θ):
Distance traveled = ∫(2 * sin(t) * cos(t) dt) from 0 to θ
Using the substitution u = sin(t), du = cos(t) dt, we get:
Distance traveled = ∫(2 * u du) from 0 to sin(θ)
Now, integrating with respect to u, we get:
Distance traveled = u^2 | from 0 to sin(θ) = (sin^2(θ)) - (0^2) = sin^2(θ)
The length of the curve can be computed as the arc length:
Length of the curve = ∫(2 * sin(t) * cos(t) dt) from 0 to θ
As we computed earlier, the distance traveled is equal to sin^2(θ). Therefore, the distance traveled by the particle is the same as the length of the curve.
To know more about distance, visit:
https://brainly.com/question/29055485#
#SPJ11
Part A: The table shows the number of new stores a smoothie chain opened over the past 10 years. The data models an exponential function.
1. Use technology or hand calculations to determine the equation for the exponential function modeled by the data in the table. Show an image of your final answer. (10 points)
2. Using the equation found in question 1, predict the approximate number of stores the retail chain will open in year 13. (8 points)

Answers
To determine the equation for the exponential function modeled by the data, you need to identify the pattern and use regression analysis or logarithms. Please provide the data in the table, and I will help you find the equation.
Once we have the equation, we can use it to predict the approximate number of stores the retail chain will open in year 13. This is done by substituting the value of 13 into the equation and solving for the unknown variable.
To determine the equation for the exponential function modeled by the data, find the common ratio and use it to write the equation. To predict the number of stores in year 13, substitute the value into the equation.
Explanation:To determine the equation for the exponential function modeled by the data in the table, we need to find the common ratio. The common ratio is the ratio of a term to its previous term. We can calculate the common ratio by dividing any term by the previous term. Once we find the common ratio, we can use it to write the equation of the exponential function. To predict the approximate number of stores the retail chain will open in year 13, we can substitute the value of 13 into the equation we found in question 1.
Learn more about Exponential functions here:https://brainly.com/question/37289664
#SPJ11Final answer:
To determine the equation for the exponential function modeled by the data, find the common ratio and use it to write the equation. To predict the number of stores in year 13, substitute the value into the equation.
Explanation:To determine the equation for the exponential function modeled by the data in the table, we need to find the common ratio. The common ratio is the ratio of a term to its previous term. We can calculate the common ratio by dividing any term by the previous term. Once we find the common ratio, we can use it to write the equation of the exponential function. To predict the approximate number of stores the retail chain will open in year 13, we can substitute the value of 13 into the equation we found in question 1.
Learn more about Exponential functions here:https://brainly.com/question/37289664
#SPJ11
what is the estimated number of cell phone subscribers in 2018? algebra 1
Answers
Long answer: According to the International Telecommunication Union (ITU), there were an estimated 5 billion unique mobile phone users in the world as of January 2018. However, it is important to note that this number does not necessarily equate to the number of individual subscribers, as some people may have more than one mobile phone or SIM card. Additionally, the number of cell phone subscribers can vary by country and region. For example, according to Statista, the number of mobile phone users in the United States was approximately 266 million in 2018. Overall, while there is not a definitive global estimate for the number of cell phone subscribers in 2018, it is clear that mobile technology continues to have a significant impact on the way people communicate and access information worldwide.
PLEASE HELP ME!!
The QUADRATIC curve-of-best-fit for the data in the table is given by the equation
A) y = 0.8x2 + 1.7x - 1.4.
B) y = 1.1x2 + 2.2x - 1.2.
C) y = 1.4x2 - 2.9x + 1.0.
D) y = 2.3x2 - 2.4x + 3.5

Answers
Equation D , y =2.3x²-2.4x+3.5 gives the Quadratic curve-of-best-fit for the data in the table.
What is a Quadratic Equation ?A Quadratic equation is an equation that can be represented as
y = ax²+bx+c
Here in the question the data points and the quadratic equations are given ,
We have to find the best fit
From the graph attached the best fit can be determined.
Equation D , y =2.3x²-2.4x+3.5 gives the Quadratic curve-of-best-fit for the data in the table.
To know more about Quadratic Equation
https://brainly.com/question/2263981
#SPJ1
what is 0.34 round to the nearest tenth
Answers
Answer:
0.3
Step-by-step explanation:
We use the following rules to round 0.34 to the nearest tenth:
A) If the last digit in the fractional part of 0.34 is less than 5, then simply remove the last the digit of fractional part.
B) If the last digit in the fractional part of 0.34 is 5 or more and the first digit in the fractional part is less than 9, then add 1 to the first digit of the fractional part and remove the second digit.
C) If the last digit in the fractional part of 0.34 is 5 or more and the first digit in the fractional part is 9 then add 1 to the Integer part and make the fractional part 0.
With 0.34, rule A applies and the answer is: 0.3
Answer:
0.3
Step-by-step explanation:
round down because its 4
Write an expression that tells you how fast height is changing, with respect to time, after a seconds have passed.
Answers
A(n) _______ design is used when the researcher wants to test a relationship between existing variables, and a(n) _______ design is used when a research wants to test a causal link between variables.
Answers
A(n) "correlational" design is used when the researcher wants to test a relationship between existing variables. This design aims to determine the degree to which two variables are related or associated with each other. In a correlational design, the researcher measures the variables of interest and examines the statistical relationship between them, typically using correlation coefficients.
On the other hand, a(n) "experimental" design is used when a researcher wants to test a causal link between variables. In an experimental design, the researcher manipulates one or more independent variables and measures the effect on a dependent variable. By controlling for confounding variables and using random assignment, an experimental design allows for causal inferences to be made.
It's important to note that while correlational designs can provide valuable insights into the relationships between variables, they do not establish causality. Experimental designs, on the other hand, provide stronger evidence for causal relationships by systematically manipulating variables.
A(n) \(\textbf{correlational}\) design is used when the researcher wants to test a relationship between existing variables.
A(n) \(\textbf{experimental}\) design is used when a researcher wants to test a causal link between variables.
To know more about Evidence visit-
brainly.com/question/30780436
#SPJ11
hey! please help i’ll give brainliest

Answers
Answer:
either c or b
Step-by-step explanation:
im so sorry im also stuck but im more leaning towards near future but im not sure! i hope that the process of elimination helped a bit at least
Answer:
In the near future.
Step-by-step explanation:
In the past it would have said was etc..
Right now, it would say "it just started"
In the far future it would have said in a few days or so.
3. At an exhibition, the ratio of the number of men to the number of women was 10:3. Halfway through the exhibition, 110 men left and the number of men was 5/12 of the total number of people who remained behind. How many women were there at the exhibition?
Answers
Answer:
Step-by-step explanation:
Let's assume the initial number of men at the exhibition is 10x, and the initial number of women is 3x.
After 110 men left, the number of men remaining is 10x - 110.
The total number of people remaining is (10x - 110) + (3x) = 13x - 110.
According to the given information, the number of men remaining (10x - 110) is 5/12 of the total number of people remaining (13x - 110). We can write this as an equation:
10x - 110 = (5/12)(13x - 110)
To solve this equation, we can start by simplifying both sides:
10x - 110 = (65/12)x - (55/6)
To get rid of the fractions, we can multiply both sides of the equation by 12:
12(10x - 110) = 65x - 110(2)
120x - 1320 = 65x - 220
Next, we can bring the x terms to one side and the constant terms to the other side:
120x - 65x = 1320 - 220
55x = 1100
Dividing both sides by 55, we find:
x = 20
Now, we can substitute the value of x back into the initial expressions to find the number of men and women:
Number of men = 10x = 10(20) = 200
Number of women = 3x = 3(20) = 60
Therefore, there were 60 women at the exhibition
Hope this answer your question
Please rate the answer and
mark me ask Brainliest it helps a lot
How much is 2% of 3.50
Answers
Answer:
.07
Step-by-step explanation:
3.50 * 2% = .07
or
3.50 * .02 = .07
two fair dice are thrown. given that the first shows 3, what is the probability that the total exceeds 6?
Answers
The probability that the total of the number on the dice exceeds 6 is 1/12.
When the two fair dice are thrown the total possible outcome are,
= 6 x 6
= 36
So, there are a total of 36 possible outcomes.
If one of the dice shows 3, then the total favorable outcomes that the sum of the numbers on the dice exceeds 6 is,
(3+4)(3+5)(3+6)
Hence, the total possible favorable outcomes are 3.
Probability of an event = Total favorable outcomes/total possible outcomes.
Probability that the sum exceeds 6 = 3/36
Probability that the sum exceeds 6 = 1/12
So, Probability that the sum exceeds 6 is 1/12.
To know more about probability, visit,
https://brainly.com/question/13604758
#SPJ4
pleaase someone help me !
How much would you have to repay on a credit card debt of 3500$ after 2-years if the interest rate is 19.5% compounded half-yearly .
Answers
Answer:
$ 4,865
Step-by-step explanation:
First find the simple intere
Answer:
P = A (1 + i)^n where P is the payment, A the original amount and i the interest rate for the payment period.
Assuming .195 is the annual interest rate then
i = .195 / 2 = .0975 the rate that will be compounded every 6 mos.
P = 3500 * (1 + .0975)^4 = $5078
show that acos(wt)+bsin(wt) can be written in the form
Answers
This form of the equation is useful because it shows that any periodic function of time can be represented as a sum of sine and cosine functions with different amplitudes and phases.
We can show that acos(wt) + bsin(wt) can be written in the form Rsin(wt + φ) where R and φ are constants and R is the amplitude of the wave.
Using the trigonometric identity sin(a+b) = sin(a)cos(b) + cos(a)sin(b), we can write:
acos(wt) + bsin(wt) = R(sin(wt+φ)),
where R = √(a^2+b^2) and φ = tan⁻¹(b/a).
To see how this works, we can use the values of a = 3 and b = 4 as an example:
acos(wt) + bsin(wt) = 3cos(wt) + 4sin(wt)
R = √(a^2+b^2) = √(3^2 + 4^2) = 5
φ = tan⁻¹(b/a) = tan⁻¹(4/3)
So we can rewrite the equation as:
acos(wt) + bsin(wt) = 5sin(wt + tan⁻¹(4/3))
This form of the equation is useful because it shows that any periodic function of time can be represented as a sum of sine and cosine functions with different amplitudes and phases. It is also helpful in analyzing the properties of waves, such as their amplitude and frequency.
Learn more about periodic function here
https://brainly.com/question/29120892
#SPJ11
A trapezoid has bases measuring 5cm and 9cm, and an area of 42cm. Find the height
Answers
The height of the trapezoid is 6 cm
How to find the height?The given parameters are:
Bases = 5cm and 9cm
Area = 42 square cm
The height is calculated using
Area = 0.5 * (Sum of bases) * Height
So, we have:
42 = 0.5 * (5 + 9)* Height
This gives
42 = 7* Height
Divide through by 7
Height= 6
Hence, the height of the trapezoid is 6 cm
Read more about area at:
https://brainly.com/question/24487155
#SPJ1
M. A rectangular swimming pool is 5.4 m long and
3.5 m wide. It has a
path 0.5 m wide
Path
around it. What is
the area of the path?
Swimming pool
NEED HELPPPPP
Answers
Answer:
i need help please with my maths
Step-by-step explanation:
What percent of Mr. Reyes total compensation was withheld for federal taxes?

Answers
The percentage of Mr. Reyes total compensation that was withheld for federal taxes is given as follows:
14.2%.
How to calculate the percentage?From the table, we have that the parameters for this problem are given as follows:
Mr. Reyes total compensation: $61,987.50.Amount withheld for federal taxes purposes: $8,802.26.Hence the proportion of Mr. Reyes total compensation that was withheld for federal taxes is given as follows:
8802.26/61987.5 = 0.142.
Then the percentage is given as follows:
0.142 x 100% = 14.2%.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ1