a merchant asked to sell 350 short on 6% comminsion and to invest the balance in purchasing sportsware thr agent charged 9% commidion on the purchased earned rs 10122 on the two trancatioms at what price was each shirt sold
Answers
Answer:
The answer is in the picture. Hope this will help you.
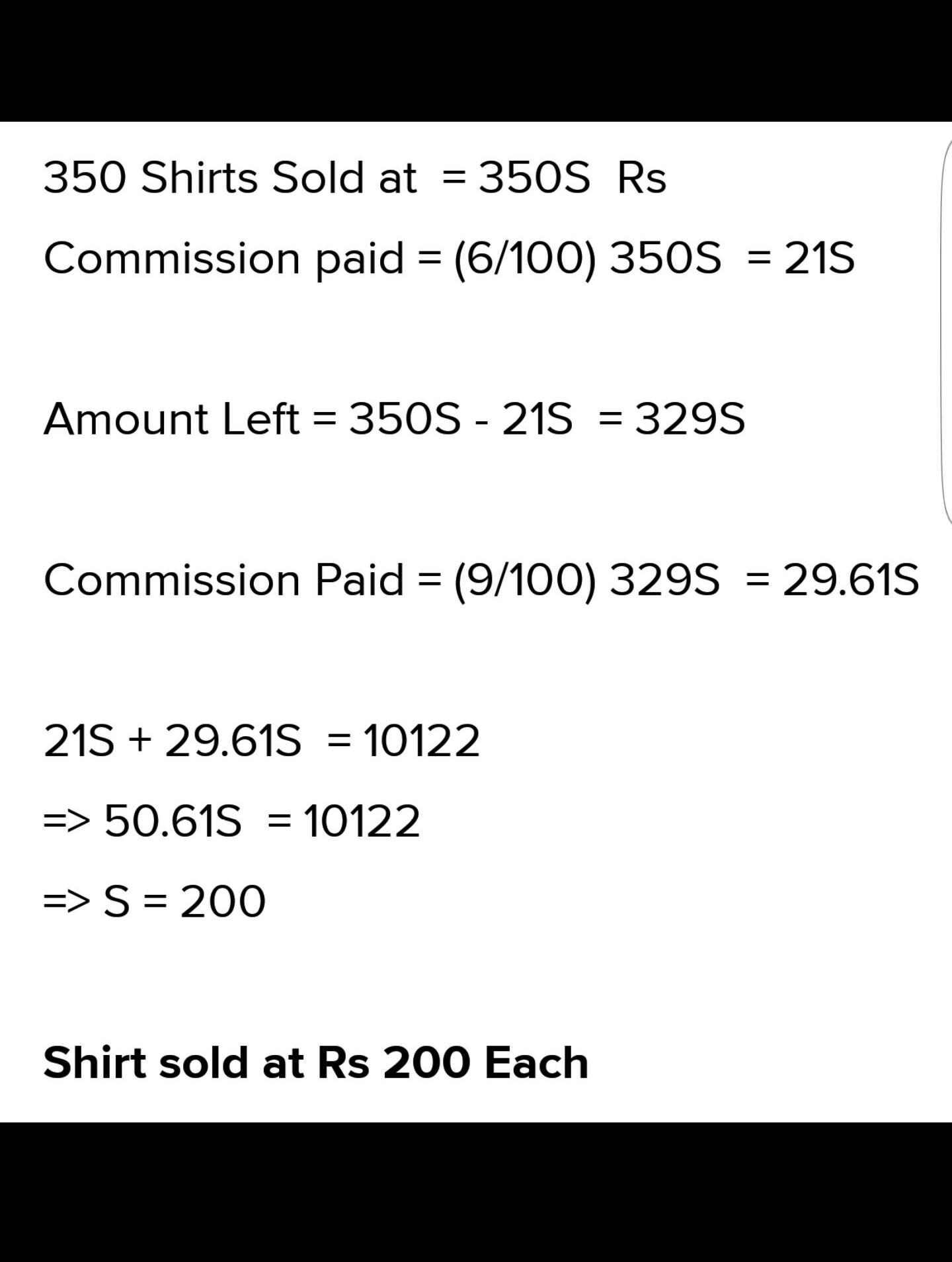
Answer:
28.92 shirt each
Step-by-step explanation:
10122 / 0.09 = 910.98 agent commission then 10122 * 0.06= 607.32=6% profit 910.98 + 607.32 = 1518.30 earns between merchant and agent he reinvested all or part? into shirts reinvested all sold quick = 10122/ 350 =28.92 shirt each
Related Questions
solve for the value of the variable -2(8x+6)=44
Answers
the equation is:
\(-2(8x+6)=44\)the we can divede for minus 2 in each side of the equation so:
\(\begin{gathered} \frac{-2(8x+6)}{-2}=\frac{44}{-2} \\ 8x+6=-22 \end{gathered}\)now we substract 6 units so:
\(\begin{gathered} 8x+6-6=-22-6 \\ 8x=-28 \end{gathered}\)finally we divide for 8:
\(\begin{gathered} \frac{8x}{8}=-\frac{28}{8} \\ x=3.5 \end{gathered}\)The table shows the cost for hamburgers at a restaurant. What is the cost of 10 hamburgers at the restaurant?
Number of Hamburgers
Cost
2
$7.00
4
$14.00
6
$21.00
8
$28.00
10
:: $28.00
# $30.00
- $35.00
$40.00
- $55.00
$70.00

Answers
Question is in the picture below

Answers
it is probably the one that us not visible beacause the ones that are visible look to be wrong so the 4th on on the page
Answer:
The first map
Hope you could understand.
If you have any query, feel free to ask.

What is the solution to the equation x^3=64?
X=
Answers
Answer:
These are two imaginary solutions.
x = − 4 , 2 ± 2 √ 3 i
Step-by-step explanation:
f(x)=-1/2x+3. G is a quadratic function with the following properties: g has lesser y-intercept than f; the maximum value of g and y-intercept are not equal; first g increases and the it decreases. Graph function g
Answers
The equation is \(g(x) = -\frac{1}{16} (x - 1)^2 + 2\) Graphing this equation below, we get a parabola that opens downwards and intersects the x-axis at x = -2 and x = 4, with a vertex at (1, 2).
Define the term graph?To demonstrate the connection between the two variables, a line or curve is drawn between the plotted points.
To graph function g, we need to find its equation that satisfies the given properties. We know that the y-intercept of g is less than that of f, so we can choose it to be 1. Also, the parabola of g opens downwards and its vertex is between the two x-intercepts, which are at x = -2 and x = 4. We can use these points to find the equation of the parabola in the standard form: g(x) = a(x - h)² + k. Using the fact that g(0) = 1 and the x-intercepts, we can solve for a, h, and k. The resulting equation is:
\(g(x) = -\frac{1}{16} (x - 1)^2 + 2\)
Graphing this equation, we get a parabola that opens downwards and intersects the x-axis at x = -2 and x = 4, with a vertex at (1, 2).
To know more about graph, visit:
https://brainly.com/question/11803331
#SPJ1

the boots was 50% of the sale price was $29.49 what is the price before
Answers
Answer:
$58.98
Explanation:
Let the original price be x.
We can go ahead and find x as follows;
\(\begin{gathered} \frac{50}{100}\times x=29.49 \\ 0.5x=29.49 \\ x=\frac{29.49}{0.5} \\ \therefore x=58.98 \end{gathered}\)6x - 2y = 18
3x + 4y= -6
Which of the following ordered pairs (x, y) is the
solution to the system of equations shown above?
A. (-3,2)
B. (-2,3)
C. (2, -3)
D. (3,-2)
Answers
Answer:
C. (2, -3)
Step-by-step explanation:
Easiest and fastest way to get the solution set is to graph the systems of equations and analyze where the graphs intersect.

Find an autonomous differential equation with all of the following properties:
equilibrium solutions at y=0 and y=3,
y' > 0 for 0
y' < 0 for -inf < y < 0 and 3 < y < inf
dy/dx = ______
Answers
dy/dx = (y-3)(y+3) is the autonomous differential equation that satisfies all of the given properties.
The autonomous differential equation that satisfies all of the given properties is dy/dx = (y-3)(y+3). This equation has two equilibrium solutions at y = 0 and y = 3, and is positive for -inf < y < 0, and negative for 0 < y < 3, and positive for 3 < y < inf.
To demonstrate this, let's consider the equation at y=-3. Since y=-3 is less than 0, the equation can be simplified to dy/dx = 6. Since 6 is positive, y' is also positive, meaning that y is increasing. Similarly, if y=3, dy/dx = 0 which is neither positive nor negative, so y remains constant. Finally, for y>3, dy/dx = -6, which is negative, so y is decreasing.
Therefore, dy/dx = (y-3)(y+3) is the autonomous differential equation that satisfies all of the given properties.
Learn more about differential equation here:
https://brainly.com/question/14620493
#SPJ4
Find u+v-4u.
u=(5,-2) and v= (-5,7)
Answers
By placing the given value in equation , we get u + v - 4u = (-20, 13)
How to evaluate vector?A physical quantity that has both directions and magnitude is referred to as a vector quantity.
A lowercase letter with a "hat" circumflex, such as "û," is used to denote a vector with a magnitude equal to one. This type of vector is known as a unit vector.
To evaluate u + v - 4u, we first need to find the vector 4u, which is obtained by multiplying the vector u by the scalar 4:
4u = 4(5,-2) = (20,-8)
Now, we can add u and v, and subtract 4u from the result:
u + v - 4u = (5,-2) + (-5,7) - (20,-8)
= (5 - 5 - 20, -2 + 7 + 8)
= (-20, 13)
Therefore, u + v - 4u = (-20, 13).
To know more about vector visit:
https://brainly.com/question/29740341
#SPJ1
Bean Seed Growth Temp 25 20 15 10 Days S 7 9 If the trend continues, how many days will it take at 10 degrees?

Answers
The needed number of days at 10 degrees is given as follows:
11 days.
How to define a linear function?The slope-intercept equation for a linear function is presented as follows:
y = mx + b
In which:
m is the slope.b is the intercept.For this problem, we have that when x decreases by 5, y increases by 2, hence the slope m is given as follows:
m = -2/5
m = -0.4.
Hence the time needed for a temperature of 10 degrees is given as follows:
9 + 2 = 11 days.
More can be learned about linear functions at https://brainly.com/question/15602982
#SPJ1
Round 3 487 to the nearest thousands place.
Answers
Answer:
3,000
Step-by-step explanation:
Answer:
3,000
Step-by-step explanation:
Since 4 is less than 5, the digit must not change. Meaning its 3,000
hoped i helped and hopefully i didnt sound confusing
At a local play, student tickets cost $6 each and adult tickets cost $11 each. If ticket sales were $3,000 for 400 tickets, how many students attended the play?
A 120
B 180
C 220
D 280
Answers
There are 280 students attending the play.
What are linear equations?Each term in a linear equation has an exponent of 1, and when this algebraic equation is graphed, it always produces a straight line. It is called a "linear equation" for this reason.
Both linear equations with one variable and those with two variables exist.
Given student tickets cost $6 each and adult tickets cost $11 each,
let a number of students be x and the number of tickets be y
total tickets sold = 400
x + y = 400
and total amount made = $3000
so 6x + 11y = 3000
from equation 1,
y = 400 - x
substitute in the equation,
6x + 11y = 3000
6x + 11(400 - x) = 3000
6x + 4400 - 11x = 3000
-5x = 3000 - 4400
-5x = -1400
x = 1400/5
x = 280
and y = 400 - 280
y = 120
Hence 280 students attended the play.
Learn more about linear equations;
https://brainly.com/question/11897796
#SPJ1
Determine which integers in the set S: {−2, −3, −4, −5} will make the inequality 4p − 7 ≥ 9p + 8 true.
PLS HELP ME
Answers
The integers in the set s: {-2,-3,-4,-5} will make the inequality 4p-7 \(\geq\) 9p+8 true are : -3, -4, -5
Let's solve the inequality first
4p -7 \(\geq\) 9p +8
Taking p's on the same side we will get :
-7 - 8 \(\geq\) 9p - 4p
-15 \(\geq\) 5p
Divide by 5 into both sides
-3 \(\geq\) p
i.e. p \(\leq\) -3
Therefore p must be less than or equal to -3
From the set, we have the numbers -3,-4,-5 which are less than or equal to -3
Hence the integers -3,-4,-5 will make the inequality 4p-7 \(\geq\) 9p+8 true
To learn more about inequality:
https://brainly.com/question/30238989
If a cylinder has a height of 12 feet and a radius of 4 feet, what is its surface area (in square feet)? What is its volume (in cubic feet)? (Round your answers to two decimal places.)
Answers
The volume of the cylinder is 602.88 ft³.
The surface area of the cylinder is 301.44 ft²
How to find the volume and surface area of a cylinder?volume of a cylinder = πr²h
volume of a cylinder = 3.14 × 4² × 12
volume of a cylinder = 3.14 × 16 × 12
volume of a cylinder = 602.88 ft³
Surface are of a cylinder = 2πr(r + h)
Surface are of a cylinder = 2 × 3.14 × 4(4 + 8)
Surface are of a cylinder = 25.12(12)
Surface are of a cylinder = 301.44 ft²
learn more on cylinder here: https://brainly.com/question/27851775
#SPJ1
Give a linear function for the cost see from the electrician to come to your house and wait for three hours he charges 95 flight for you to come to your house and 35 per hour he is there to work

Answers
SOLUTION TO QUESTION 1
Step1:Define the parameters
\(\begin{gathered} \text{let cost be c} \\ \text{and } \\ \text{the time taken be t} \end{gathered}\)Step2: Write the given information
\(\begin{gathered} \text{ \$95 for flight } \\ \text{and } \\ \text{ \$35 per hour of work } \end{gathered}\)Then, the total cost will be
\(\text{ C=price for the flight+35}\times\text{ number of hours he work}\)Step3: Write out the linear function in terms of t
\(\begin{gathered} C(t)=95+35(t) \\ C(t)=95+35t \end{gathered}\)Therefore the linear function for the cost for the electrician to come and work will be
C(t)=95+35t
EMILY COOKIE RECIPE CALLS FOR 3 CUPS OF FLOUR AND 1 CUP OF SUGAR WHAT IS THE RATIO OF FLOUR TO SUGAR
Answers
There are 26 boys and 20 girls in a class.
The boys and the girls have some counters.
The mean number of counters that the boys have is 28.
The mean number of counters that the girls have is 19.
Work out the mean number of counters the 46 children have.
Answers
Computing the total number of counters in the class as 1,108, the mean number of counters that the 46 children have is 24.
What is the mean?The mean refers to the average value.
The average is the quotient of the total value divided by the number of items in the data set.
The number of boys in the class = 26
The number of girls in the class = 20
The total number of boys and girls in the class = 46
The mean number of counters that the boys have = 28
The total number of counters that the boys have = 728 (28 x 26)
The mean number of counters that the girls have =19
The total number of counters that the girls have = 380 (19 x 20)
The total number of counters that the class has = 1,108 (728 + 380)
The average or mean number of counters in the class = 24 (1,108 ÷ 46)
Learn more about the average at https://brainly.com/question/130657.
#SPJ1
Solve 3x + 9 = 24.
A. x = 5
B. x = 11
C. x = 12
D. x = 18
Answers
Answer:
A. x = 5
Step-by-step explanation:
3x + 9 = 24
→ Minus 9 from both sides
3x = 15
→ Divide both sides by 3
x = 5
A conveyor belt at a recycling center is 294 feet long. The belt moves 7 feet in 6 seconds. A sorter calculates that it
takes 343 seconds for an object to travel the length of the belt. He places his plastic water bottle at the start of the belt so he can tie
his shoe. He walks to the end of the belt before 343 seconds pass. His bottle is already in the shredder. How many seconds does it
take the water bottle to travel the length of the belt? What is the sorter's error?
Answers
The sorter's error is 91 seconds. This means that the sorter miscalculated the time it would take for the water bottle to travel the length of the belt by 91 seconds.
What lengths are examples of?When anything is referred to as being "long," it refers to its overall length. In other words, it is the larger of the third or upper two dimensions of a geometric object. An example of a shape with two dimensions is a rectangle.
To find out how many seconds it takes for the water bottle to travel the length of the belt, we can use the equation:
distance = rate x time
We know the distance (294 feet) and the rate (7 feet per 6 seconds). Let's solve for time:
294 feet = (7 feet/6 seconds) x time
294 feet x 6 seconds = 7 feet x time
1764 seconds = 7 feet x time
time = 1764 seconds / 7 feet
time = 252 seconds
So it takes 252 seconds for the water bottle to travel the length of the belt.
Now let's calculate the sorter's error:
343 seconds - 252 seconds = 91 seconds
The sorter's error is 91 seconds. This means that the sorter miscalculated the time it would take for the water bottle to travel the length of the belt by 91 seconds.
To know more about length visit:
brainly.com/question/29868754
#SPJ1
60% discount on $500 sweater
Answers
The discount price of the sweater will be, the original price minus the percentage of discount of the original price.
First, express the percentage of discount as a decimal:
60% = 60/100 = 0.6
so:
\(\begin{gathered} 500-0.6\cdot500 \\ 500-300=200 \end{gathered}\)The discount price of the sweater is $200
an influencer had 400 subscribers in January. The number of subscribers have grown by a factor of 1.5 every month since then. write a sequence that represents the number of subscribers for the three months that followed (january through april)
Answers
Answer:
y=400(1.5)^4
Step-by-step explanation:
Which of these rotations will map an equilateral triangle to itself?(1 point)
45°
60°
90°
120°
Answers
Answer:
120°
Step-by-step explanation:
The equilateral triangle is the triangle that has three sides equal also they are congruent to each other and each side has an angle of 60 degrees
Now for rotation it would be a map to itself at 120 degrees counterclockwise
So the same is to be considered
Hence, the last option is correct
And, the other options are incorrect
The accompanying technology output was obtained by using the paired data consisting of foot lengths (cm) and heights (cm) of a sample of 40 people. Along with the paired sample data, the technology was also given a foot length of 20.4cm to be used for predicting height. The technology found that there is a linear correlation between height and foot length. If someone has a foot length of 20.4 cm, what is the single value that is the best-predicted height for that person?
Answers
The best-predicted height for someone with a foot length of 20.4 cm is approximately 65.83 cm.
To find the best-predicted height for someone with a foot length of 20.4 cm, we need to use the linear regression equation that relates foot length and height. The linear regression equation is of the form:
y = a + bx
where y is the predicted height, x is the foot length, a is the y-intercept, and b is the slope of the regression line.
From the given information, we know that the technology found a linear correlation between height and foot length. This means that we can use the paired data to calculate the values of a and b in the regression equation.
Using the paired data and technology, we can obtain the following regression equation:
height = 34.774 + 1.4966 (foot length)
Now we can substitute the given foot length of 20.4 cm into the equation to obtain the predicted height:
height = 34.774 + 1.4966 (20.4)
height = 65.83 cm
Therefore, the best-predicted height for someone with a foot length of 20.4 cm is approximately 65.83 cm.
Know more about linear regression here:
https://brainly.com/question/29665935
#SPJ11
Write the slope-intercept form of the equation that passes through the point (-2,2) and is parallel to the line y = 3x - 5
Answers
Parallel lines have equivalent slopes, so as the slope of the given line is 3, the slope of the line we want to find is also 3.
Substituting into point-slope form,
\(y-2=3(x+2)\\\\y-2=3x+6\\\\\boxed{y=3x+8}\)

Giving a test to a group of students, the grades and gender are summarized below
A B C Total
Male 20 13 7 40
Female 9 2 8 19
Total 29 15 15 59
If one student is chosen at random,
Find the probability that the student got an A.
20
29
29
59
20
59
Answers
The grades and gender is 29/59
What is probability?It is a branch of mathematics that deals with the occurrence of a random event.
Given:
A B C Total
Male 20 13 7 40
Female 9 2 8 19
Total 29 15 15 59
Total candidates who have A grade = 20+9 = 29
So, the probability that the student got an A
= 29/59
Learn more about probability here:
https://brainly.com/question/16970489
#SPJ1
A large truck, traveling 30 miles per hour, carries a letter from the central post office to the local post office. The letter is then loaded onto a local vehicle, which travels at an average speed of 10 miles per hour, until the letter reaches its destination. The large truck carried the letter for a minutes and the local vehicle carried the letter for b minutes. If the total distance that the letter travelled from the central post office to its destination was 24 miles, which of the following equations correctly relates a and b?
Answers
The linear equation that will relate a and b is 30a+10b=24.
What is linear equation?
A linear equation is an equation in which the highest power of the variable is always 1. It is also known as a one-degree equation. The standard form of a linear equation in one variable is of the form Ax + B = 0. Here, x is a variable, A is a coefficient and B is constant. The standard form of a linear equation in two variables is of the form Ax + By = C. Here, x and y are variables, A and B are coefficients and C is a constant.
Now,
As given
speed and time it carried letter of large truck=30m/h and a
speed and time it carried letter of local vehicle=10m/h and b
Total distance travelled=24miles
As speed=distance/time
distance=speed*time
Hence,
24=30a+10b
i.e. 30a+10b=24
To know more about linear equations visit the link
https://brainly.com/question/29739212?referrer=searchResults
#SPJ1
A sample of 15 buttons is randomly selected and the following diameters are measured in inches. Give a point estimate for the population standard deviation. Round your answer to three decimal places.
1.33,1.25,1.22,1.27,1.39,1.34,1.33,1.32,1.26,1.20,1.28,1.20,1.24,1.38,1.31
Answers
Answer:
The point estimate for the population standard deviation is of 0.061.
Step-by-step explanation:
The point estimate for the population standard deviation is the sample standard deviation.
Sample mean:
\(\overline{x} = \frac{1.33+1.25+1.22+1.27+1.39+1.34+1.33+1.32+1.26+1.20+1.28+1.20+1.24+1.38+1.31 }{15} = 1.288\)
Sample standard deviation:
Square root of the sum of the difference squared of all these values (1.33, 1.25, 1.22,...) and the mean of 1.288, divided by 15 - 1 = 14.
Using a calculator, it is of:
\(s = 0.061\)
The point estimate for the population standard deviation is of 0.061.
Write this English phrase as an algebraic expression. Let the variable x represent the number.
five more than a number
translated into an algebraic expression:
Answers
Answer:
5+x
Step-by-step explanation:
the variable x represents the number
five more than the number, meaning we have to add 5 to the number
5+x
Answer: x+5
Step-by-step explanation:
Convert the fraction below into a decimal 23/40?
Answers
Answer:
Step-by-step explanation:
23/40 = 0.575
Solve the system by the addition method. x + 3y = 6 3x + 4y = −2
Answers
The solution to the system is x = -6 and y = 4.
To solve the system by the addition method, we want to add the equations together in a way that will eliminate one of the variables.
Let's start by multiplying the first equation by -3 to get -3x - 9y = -18, and then add the second equation to it:
-3x - 9y = -18
+ 3x + 4y = -2
-------------
-5y = -20
Now we can solve for y by dividing both sides by -5:
y = 4
We can substitute y=4 into one of the original equations, say x+3y=6, to solve for x:
x + 3(4) = 6
x + 12 = 6
x = -6
So the solution to the system is x = -6 and y = 4.
for such more question on variables.
https://brainly.com/question/19803308
#SPJ11