Answers
Answer:
I'll do 15 through 19
Step-by-step explanation:
15.
4m +2 =18
subtract 2 from both side
4m=16
divide by 4 to solve for m
4/4m=16/4
m=4
17. 3t=2t +5
-2t from both sides
1t=5
t=5
19. -5x =3x- 24
subtract 3x from both sides
-8x= -24
divide by -8 to get x alone( Remember a negative divided by a negative is a positive)
x=3
Related Questions
prove that sum of angles of a convex plygon of n sides is (n-2)pi, where n>3
Answers
The IH states that the sum of the angles in convex polygon C(which, by the IH, is (n - 2)·π) and the sum of the angles in triangle B (180°) is the same as the sum of the angles in A.
Using induction all convex polygons with n vertices have angles that add to (n - 2)·π, therefore let P(n) be that. We will demonstrate that P(n), where n≥3, holds for every n ∈ N. We demonstrate P(3), which states that any convex polygon with three vertices has an angle total of 180 degrees. Any triangle formed by such a polygon has angles that add up to 180°.
Assume that P(n) holds for some n ≥ 3 and that all convex polygons with n vertices have angles that add to (n-2) 180° for the inductive step. We demonstrate P(n+1), which states that each convex polygon with n+1 vertices has an angle sum of (n-1)·π. Let A represent any n+1-vertex convex polygon.
The IH states that the sum of the angles in convex polygon C (which, by the IH, is (n - 2)·π) and the sum of the angles in triangle B (180°) is the same as the sum of the angles in A. As a result, the total angle in A is (n-1) 180°. Thus, the induction is complete and P(n + 1) holds.
To learn more about angles in convex polygon link is here
brainly.com/question/13585442
#SPJ4
What are the tests for parallel and perpendicular lines?
Answers
To determine if a line is parallel or perpendicular, we can place each line on a sloped intersection shape (y = mx + b) and observe the slope m of each line. Equal slopes result in parallel lines. If the slope is multiplied by -1, then the line is vertical.
Parallel Lines:
Two or more lines that lie in the same plane and do not intersect are called parallel lines. They are equidistant from each other and have the same slope. A straight line equation is usually written in the form of a slope intercept represented by the equation y = mx + b. where "m" is the slope and "b" is the y-intercept. The "m" value defines the slope and tells how steep the line is.
Perpendicular Lines:
A vertical line or perpendicular line is two separate lines that intersect at an angle of 90° to each other. These are straight lines known as perpendiculars that meet each other at certain angles (right angles).
We have already seen what a vertical line looks like. If a shape has an "L" shape, its vertex angles are right angles. Vertical lines always intersect each other, but not all intersecting lines are always perpendicular to each other.
Two main properties of vertical lines:
1. Perpendicular lines always intersect or intersect each other.
2. The angle between two vertical lines is always 90°.
Learn more about Perpendicular:
https://brainly.com/question/29268451
#SPJ4
Dose anyone know how to solve this?

Answers
Strictly increasing functions:
Let a function f(a) for x = a, then,
f(a + h), for x = a + h,
If f(a + h) > f(a), we say this a strictly increasing function, as with increase in x, f(x) also increases.
In the given graph, there are straight lines so you can say increasing interval would have +ve slope.
For x = -5 to -4, it increases = [-5, -4]
For x = 1 to 3, it increases = [1, 3]
Answer: [-5, -4] , [1, 3]
Mr John leased two thousand fifty meter square of land from the city administration. He gave one eighth of his land to his brother. How many meters square did he have left?
Answers
Answer:
1793.75 m²
Step-by-step explanation:
Given that :
Total amount of land leased = 2050 m²
Fraction of land given to brother = 1/8
Amount of land given to brother :
1/8 * 2050 = 256.25 m²
Amount of land left :
2050 m² - 256.25 m²
= 1793.75 m²
Find the weighted average of these values.
Value Weight
7.00 75.0%
8.00 15.0%
9.00 10.0%
Answers
By using the concept of weighted averages, the weighted average of the given values is equal to 7.35.
How to determine weighted averages?
Weighted average is a kind of average in which each element has an specific weight in contrast with uniform average, where each element has the same weight. In this problem we have three values associated to three distinct weights, whose weighted average is:
x = 0.75 · 7 + 0.15 · 8 + 0.10 · 9
x = 7.35
By using the concept of weighted averages, the weighted average of the given values is equal to 7.35.
To learn more on weighted averages: https://brainly.com/question/18554478
#SPJ1
The perimeter of a rectangular pool i 110 feet. If the pool i 17 feet wide, find the length of the pool
Answers
It will be 38 feet long
What is perimeter?The perimeter is the space surrounding a shape's edge.
The sum of the lengths of all the sides in a rectangular shape is its perimeter. P = 2( L+B)
Given that a rectangle's width is 17 feet and its perimeter is 110 feet, the length will be P = 2 (L + B)
110 = 2 (L + 17)
55 - 17 = L.
The pool is therefore 38 feet long.
To know more about perimeter of a rectangular check the link:
brainly.com/question/16445166
#SPJ4
I need help answering this question for trig equations.
Sec^2x+4secx=-4
Answers
Answer:
x = 120°
Step-by-step explanation:
We are given;
sec²x + 4secx = -4
Rearranging gives;
sec²x + 4secx + 4 = 0
Factorizing the left hand side gives;
(sec x + 2)(sec x + 2) = 0
This means that;
(sec x + 2) = 0
From trigonometry, we know that sec x = 1/cos x
Thus;
(1/cos x) + 2 = 0
1/cos x = -2
cos x = -1/2
cos x = -0.5
x = cos^(-1) -0.5
x = 120°
daniel bought 6 shirts for $28.93 if he paid the same price for each shirt how much would he spend if he bought 15 shirts?
Answers
Answer:
i dont know do it yourself chump
Step-by-step explanation:
PLEASE HELP ME!!! Graph the line with a slope of 2/3 that contains
the point (-3,-5).
Answers
Answer:
y=2/3x-3
Step-by-step explanation:
first we fill in the values for y=2/3x to find y intercept
-5=2/3(-3)
-5=-6/3
-5=-2
We know in order to make this equation true we have to subtract 3 so we write the new equation like this
y=2/3x-3
And we can test it
-5=2/3(-3)-3
-5=-6/3-3
-5=-2-3
-5=-5
Hopes this helps please mark brainliest
What is halfway between pie over 2 and pie
Answers
Answer:
3π/2 is halfway between π and 2π.
Step-by-step explanation:
3π/2 is halfway between π and 2π.
Answer:
3π/2 is halfway between π and 2π
Hope this helps u
Hi, may someone help me with this question? Thank you!:)
“If f(x) = x^2 + 7, find f(x+2)”
Answers
Answer:
=x^2 +4x+11
Step-by-step explanation:
f(x) = x^2 + 7,
Replace x with x+2
f(x+2) = (x+2)^2 + 7
= (x+2)(x+2) +7
FOIL
= x^2 +2x+2x+4 +7
Combine like terms
=x^2 +4x+11
Answer:
\(f(x+2)=x^2+4x+11\)
Step-by-step explanation:
In \(f(x)=x^2+7\), for all values of \(x\), we substitute \(x\) (what is in the parentheses) into \(x^2+7\) to output a \(y\) value.
In \(f(x+2)\), the term \((x+2)\) is in the parentheses. Therefore, substitute \((x+2)\) for \(x\) in \(x^2+7\) to find \(f(x+2)\):
\(f(x+2)=(x+2)^2+7\)
Expand using \((a+b)^2=a^2+2ab+b^2\),
\(f(x+2)=x^2+4x+4+7\)
Combine like terms:
\(\boxed{f(x+2)=x^2+4x+11}\)
The first drop box option are exactly, at least, less thanDrop box 2 option are interval, domain, rate of change, rangeThe third drop box is the same as the secondThe last drop box option are a, x, f(x), b

Answers
Solution
A function is a relation where each input value is assigned to AT LEAST one output value
The DOMAIN of a function is the set of all input values, or x-values, for which the function is defined.
The RANGE of a function is the set of all output values, or y-values, for which the function is defined.
To write the equation y = ax + b in function notation, substitute f(x) for y.
17. Write a rational function in expanded form that satisfies the
following criteria:
• V.A. x=2
• SI.A y =5x-1
• Zero of function (-1,0)
pls help thank you<3
Answers
Answer:
\(f(x) = \frac{5x^2 + 4x - 1}{x - 2}\)
Step-by-step explanation:
Given
VA; \(x = 2\)
SLA: \(y = 5x - 1\)
Zero of function: \((-1,0)\)
Required
Determine the rational function in expanded form
Analyzing the vertical asymptote
The vertical asymptote is given as:
\(x = 2\)
Subtract 2 from both sides
\(x - 2 = 2 - 2\)
\(x - 2 = 0\)
This means that the denominator must be \(x - 2\)
Analyzing the zero of the function
The zero of the function is given as: \((-1,0)\)
This means that \(x = -1\), when \(y = 0\)
Equate \(x = -1\) to 0 by add 1 to both sides
\(x + 1 =- 1 + 1\)
\(x + 1 =0\)
This means that one of the numerators must be \(x + 1\)
Analyzing the slant asymptote:
\(y = 5x - 1\)
This means that one of the numerators must be \(5x - 1\)
Hence, the function is:
\(f(x) = \frac{(5x - 1)(x+1)}{x - 2}\)
Expand the numerator
\(f(x) = \frac{5x^2 + 5x - x - 1}{x - 2}\)
\(f(x) = \frac{5x^2 + 4x - 1}{x - 2}\)
Ayo can y’all help? No rush but it’s 25 points with brainliest

Answers
You know the answer is the third choice (C) because 7^2 is 49 and 8^2 is 56, the two closest squared numbers to 54.
49 < 54 < 56
The square root of 54 is roughly 7.4
I hope that helps :)
WILL GIVE BRAINLIEST

Answers
Step-by-step explanation: First, we create a table using the equation provided: "y = -2/3x + 1". If x is 0, then y = 1. If x = 3, y = -1. Thus, we plot the points (0, 1) and (3, -1). Then, we draw a line through them.
Please help me I dont understand this! its due soon by the end on class omg!

Answers
The number of people who would prefer Choco chocolate chip is 120.
How many people would prefer Choco chocolate chip?
Ratio expresses the relationship between two or more numbers. It shows the frequency of the number of times that one value is contained within other value(s).
In order to determine the number of people who would prefer Choco chocolate chip , multiply the given ratio of people who prefer Choco chocolate chip by the total number of people in the group.
The number of people who would prefer Choco chocolate chip = (ratio of people who prefer choco / sum of ratios) x number of people
(3/5) x 200 = 120
To learn more about ratios, please check: https://brainly.com/question/25927869
#SPJ1
A pendant hanging on a necklace is in the shape of a rhombus. The front of the pendant is covered with gold leaf.
A rhombus where the left side of the horizontal diagonal has length 7.5 millimeters and the right side of the horizontal diagonal has length 7.5 millimeters. The top of the vertical diagonal has length 11 millimeters and the bottom of the vertical diagonal has length 11 millimeters.
How much gold leaf is needed to cover the pendant?
18.5 mm2
82.5 mm2
165 mm2
330 mm2
Answers
please give brainliest
Answer:
To find the amount of gold leaf needed to cover the pendant, we can calculate the area of the rhombus. The area of a rhombus is given by the formula: area = (diagonal 1 * diagonal 2) / 2.
In this case, the diagonals are given as follows:
Left side of the horizontal diagonal: 7.5 mm
Right side of the horizontal diagonal: 7.5 mm
Top of the vertical diagonal: 11 mm
Bottom of the vertical diagonal: 11 mm
Now, we can calculate the area:
Area = (7.5 mm * 11 mm) / 2
Area = 82.5 mm²
Therefore, the amount of gold leaf needed to cover the pendant is approximately 82.5 mm².
The function d(s) = 0.0056s squared + 0.14s models the stopping distance
of a car, d(s), in metres, and the speed, s, in kilometres per hour. What
is the speed when the stopping distance is 7 m? Use a graph to solve.
Answers
Answer:
The car must have a speed of 25 kilometres per hour to stop after moving 7 metres.
Step-by-step explanation:
Let be \(d(s) = 0.0056\cdot s^{2} + 0.14\cdot s\), where \(d\) is the stopping distance measured in metres and \(s\) is the speed measured in kilometres per hour. The second-order polynomial is drawn with the help of a graphing tool and whose outcome is presented below as attachment.
The procedure to find the speed related to the given stopping distance is described below:
1) Construct the graph of \(d(s)\).
2) Add the function \(d = 7\,m\).
3) The point of intersection between both curves contains the speed related to given stopping distance.
In consequence, the car must have a speed of 25 kilometres per hour to stop after moving 7 metres.

If Joseph has 12 pineapples and sells them at a farmers market for $4.75 dollars each, how much money does he make if he sells 7 of them?
Answers
Answer:
$57
Step-by-step explanation:
12*4.75=57
If the company had $4000 worth of office supplies at the beginning of the period. What is the entry required if we find that at the end of the period we have $3900 of supplies remaining.
Answers
The entry required to account for the change in office supplies would depend on the accounting method used. Assuming the company follows the periodic inventory system, where office supplies are expensed as they are used, the entry would be as follows:
At the beginning of the period:
Debit: Office Supplies Expense - $4,000
Credit: Office Supplies - $4,000
At the end of the period:
Debit: Office Supplies - $3,900
Credit: Office Supplies Expense - $3,900
Explanation:
1. At the beginning of the period, the company records the office supplies as an asset (Office Supplies) and recognizes an expense (Office Supplies Expense) for the same amount. This reduces the value of the asset and reflects the cost of supplies used during the period.
2. At the end of the period, when it is determined that $3,900 worth of supplies remains, the company adjusts the office supplies account by reducing it by the remaining amount. This adjustment is necessary to reflect the correct value of supplies on hand at the end of the period.
The entry ensures that the net effect of the transactions is an expense of $100 ($4,000 - $3,900), which represents the cost of supplies consumed during the period.
Mandy has $2.25 credit on her mobile phone. It costs $0.10 to send a text message. What is the largest amount of text messages she can possibly send to her friends with $2.25?
Answers
Answer:
22.5
Step-by-step explanation:
since 2.25 divided by 0.10 is 22.5 so she can message 22.5 friends
Which has greater kinetic energy, a 9 kg mass moving at 4 m/s or a 16 kg mass moving at 3 m/s?
Answers
Answer:assuming this is strictly proportional, 16/3>9/4 so I’d assume the 16kg object has greater kinetic energy
Step-by-step explanation:
When interpreting Cohen's d, it's important to remember that:A. Cohen's suggestions for small, medium, and large effects are only general rules of thumb.B. what counts as a small, medium, or large effect varies quite a bit by field.C. all of the above.D. it is probably best to consider an effect size relative to other similar findings in the field.
Answers
The correct answer to when interpreting Cohen's d, it's important to remember that, is: (C) : all of the above.
When interpreting Cohen's d, it's important to remember that Cohen's suggestions for small, medium, and large effects are only general rules of thumb, and what counts as a small, medium, or large effect varies quite a bit by field.
Therefore, it is probably best to consider an effect size relative to other similar findings in the field. In summary, all the options listed are important considerations when interpreting Cohen's d, and none of them should be overlooked. Hence, the correct answer is option (C).
To know more about Cohen's d refer here:
https://brainly.com/question/9393085#
#SPJ11
Students in a gaming club have 10 games
to choose from. Each time the club meets, they randomly choose a game
to play. What are 2 different tools they could use to simulate choosing a game?
Answers
Two different tools that the students can use to simulate choosing a game include:
Random number generatorPhysical game spinnerHow can the students choose a game ?The gaming club could use a random number generator to simulate the selection of a game. They could assign a unique number to each of the 10 games (for example, 1-10), and then use a random number generator to generate a number between 1 and 10. The game that corresponds to the generated number would be the game that is selected for play.
The gaming club could also create a physical game spinner with 10 sections, each labeled with the name of a game. They could spin the spinner to select the game for play at each meeting.
Find out more on tools at https://brainly.com/question/30862586
#SPJ1
I need help, Half of the stuff on this test we haven’t learned
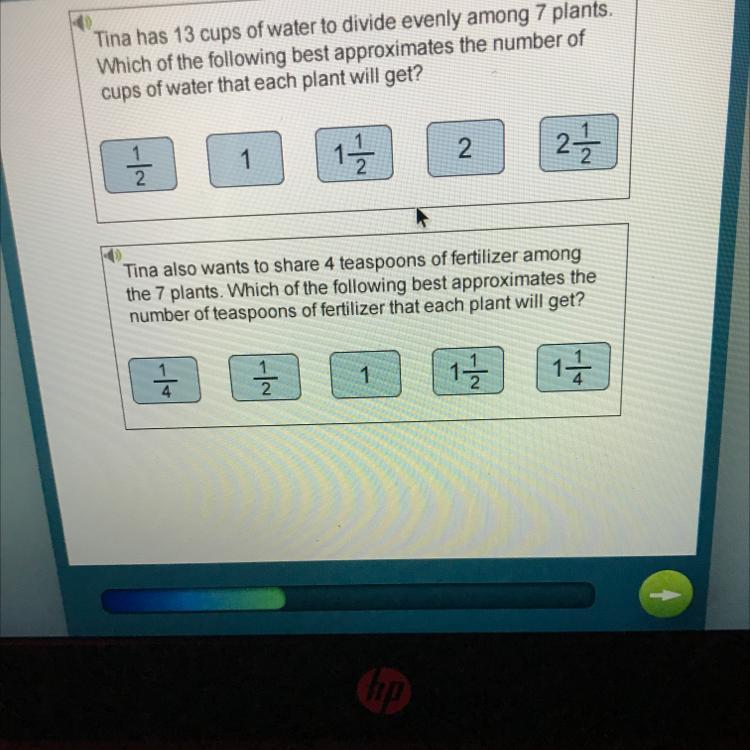
Answers
Answers:
1.) 1 and 1/2
2.) 1/2
Consider the following function.
f(x) = 4 ? x2/3
Find
f(?8) and f(8).
f(?8) = f(8) = Find all values c in (?8, 8) such that
f?'(c) = 0.
(Enter your answers as a comma-separated list. If an answer does not exist, enter DNE.)
c =
Based off of this information, what conclusions can be made about Rolle's Theorem?
This contradicts Rolle's Theorem, since f is differentiable, f(?8) = f(8), and f?'(c) = 0 exists, but c is not in (?8, 8).This does not contradict Rolle's Theorem, since f?'(0) = 0, and 0 is in the interval (?8, 8). This contradicts Rolle's Theorem, since f(?8) = f(8), there should exist a number c in (?8, 8) such that f?'(c) = 0.This does not contradict Rolle's Theorem, since f?'(0) does not exist, and so f is not differentiable on (?8, 8).Nothing can be concluded.
Answers
Based off of the information given , we can concluded this contradicts Rolle's Theorem, since f(?8) = f(8), there should exist a number c in (?8, 8) such that f?'(c) = 0. The correct answer is c.
We have:
f(x) = 4 - x^(2/3)
f(-8) = 4 - (-8)^(2/3) = 4 + 4 = 8
f(8) = 4 - 8^(2/3)
To find all values c in (-8, 8) such that f'(c) = 0, we first find the derivative of f(x):
f'(x) = -(2/3)x^(-1/3)
Setting f'(c) = 0, we get:
-(2/3)c^(-1/3) = 0
c^(-1/3) = 0
This has no solutions since c cannot be raised to a negative power and equal zero.
Therefore, the correct answer is:
This contradicts Rolle's Theorem, since f(?8) = f(8), there should exist a number c in (?8, 8) such that f?'(c) = 0. The correct answer is c.
Learn more about rolle's theorem at https://brainly.com/question/12279222
#SPJ11
the greatest solution of x in the equation x^2+8x−30=18
Answers
Answer:
The two possible outcomes are 4 and -12, so 4
Step-by-step explanation:
The greatest value of x is 4.
What is factorization?
Factorization is when you break a number down into smaller numbers that, multiplied together, give you that original number. When you split a number into its factors or divisors, that's factorization.
Given equation
⇒ \(x^{2} +8x-30 = 18\)
⇒ \(x^{2} +8x-30-18=0\)
⇒ \(x^{2} +8x-48=0\)
⇒ \(x^{2} +12x-4x-48=0\)
⇒ \(x(x+12)-4(x+12)=0\)
⇒ \((x+12)(x-4)=0\)
x = -12 and x = 4
Hence, the greatest value of x is 4.
Find out more information about factorization here
https://brainly.com/question/1863222
#SPJ2
a trader claims that the proportion of stocks that offer dividends is different from 0.14. if the trader wants to conduct a hypothesis test, should they use a left-, right-, or two-tailed hypothesis test to analyze whether the proportion of stocks that offer dividends is different from 0.14?
Answers
To conduct a hypothesis test to analyze whether the proportion of stocks that offer dividends is different from 0.14, a two-tailed hypothesis test should be used.
To analyze whether the proportion of stocks that offer dividends is different from 0.14, the trader should use a two-tailed hypothesis test.
In a two-tailed hypothesis test, the null hypothesis states that the proportion of stocks offering dividends is equal to 0.14. The alternative hypothesis, on the other hand, is that the proportion is different from 0.14, indicating a two-sided test.
The trader wants to test whether the proportion is different, without specifying whether it is greater or smaller than 0.14. By using a two-tailed test, the trader can assess whether the proportion significantly deviates from 0.14 in either direction.
Know more about hypothesis test here;
https://brainly.com/question/17099835
#SPJ11
what is the answer111111111111111111

Answers
Answer:
honestly sorry what subject that
Answer:
a=11 and b=12
Step-by-step explanation:
1) 106=9b-2 (Angles B and D are congruent)
2)b=12 (Find answer)
3)180-106=74 (Angle C + Angle D= 180)
4)7a-3=74 (Find Angle C)
5) a=11 (Find Answer)
As the project progresses, the actual finish times (AFs) of completed activities will determine
the earliest start and earliest finish times for the remaining activities in the network diagram, as well as the total slack.
Answers
As the project progresses, the actual finish times (AFs) of completed activities play a crucial role in determining various aspects of the project schedule.
The AFs are used to calculate the earliest start and earliest finish times for the remaining activities in the network diagram. These calculations are based on the dependencies between activities and the actual durations of completed activities.
By considering the AFs, the project team can determine the earliest possible start and finish times for the remaining activities, taking into account the sequence of activities and any imposed constraints. This information helps in managing the project schedule and identifying any potential delays or critical paths.
Know more about actual finish times here:
https://brainly.com/question/31925763
#SPJ11
