and right triangle sin (40 - x)° equals cos(3x)° degrees what is the value of x.
Answers
\(\qquad\qquad\huge\underline{{\sf Answer}}♨\)
Let's solve ~
\(\qquad \sf \dashrightarrow \: \sin(40 - x) = \cos(3x) \)
\(\qquad \sf \dashrightarrow \: \cos\{90 - (40 - x ) \} = \cos(3x) \)
\(\qquad \sf \dashrightarrow \:90 - (40 - x) = 3x\)
\(\qquad \sf \dashrightarrow \:90 - 40 + x = 3x\)
\(\qquad \sf \dashrightarrow \:3x - x = 50\)
\(\qquad \sf \dashrightarrow \:2x = 50\)
\(\qquad \sf \dashrightarrow \:x = 25 \degree\)
Related Questions
Builtrite has calculated the average cash flow to be $14,000 with a standard deviation of $5000. What is the probability of a cash flow being between than $16,000 and $19,000 ? (Assume a normal distribution.) 16.25% 18.13% 23.90% 2120%
Answers
The correct answer is that the probability of a cash flow being between $16,000 and $19,000 is approximately 18.59%.
To calculate the probability of a cash flow being between $16,000 and $19,000, we can use the standard deviation and assume a normal distribution.
We are given that the average cash flow is $14,000 with a standard deviation of $5,000. These values are necessary to calculate the probability.
The probability of a cash flow falling within a certain range can be determined by converting the values to z-scores, which represent the number of standard deviations away from the mean.
First, we calculate the z-score for $16,000 using the formula: z = (x - μ) / σ, where x is the cash flow value, μ is the mean, and σ is the standard deviation. Plugging in the values, we get z1 = (16,000 - 14,000) / 5,000.
z1 = 2,000 / 5,000 = 0.4.
Next, we calculate the z-score for $19,000: z2 = (19,000 - 14,000) / 5,000.
z2 = 5,000 / 5,000 = 1.
Now that we have the z-scores, we can use a standard normal distribution table or calculator to find the corresponding probabilities.
Subtracting the probability corresponding to the lower z-score from the probability corresponding to the higher z-score will give us the probability of the cash flow falling between $16,000 and $19,000.
Looking up the z-scores in a standard normal distribution table or using a calculator, we find the probability for z1 is 0.6554 and the probability for z2 is 0.8413.
Therefore, the probability of the cash flow being between $16,000 and $19,000 is 0.8413 - 0.6554 = 0.1859, which is approximately 18.59%.
So, the correct answer is that the probability of a cash flow being between $16,000 and $19,000 is approximately 18.59%.
To learn more about probability click here:
brainly.com/question/31828911
#SPJ11
The probability of a cash flow between $16,000 and $19,000 is approximately 18.59%.
To calculate the probability of a cash flow being between $16,000 and $19,000, we can use the standard deviation and assume a normal distribution.
We are given that the average cash flow is $14,000 with a standard deviation of $5,000. These values are necessary to calculate the probability.
The probability of a cash flow falling within a certain range can be determined by converting the values to z-scores, which represent the number of standard deviations away from the mean.
First, we calculate the z-score for $16,000 using the formula: z = (x - μ) / σ, where x is the cash flow value, μ is the mean, and σ is the standard deviation. Plugging in the values, we get z1 = (16,000 - 14,000) / 5,000.
z1 = 2,000 / 5,000 = 0.4.
Next, we calculate the z-score for $19,000: z2 = (19,000 - 14,000) / 5,000.
z2 = 5,000 / 5,000 = 1.
Now that we have the z-scores, we can use a standard normal distribution table or calculator to find the corresponding probabilities.
Subtracting the probability corresponding to the lower z-score from the probability corresponding to the higher z-score will give us the probability of the cash flow falling between $16,000 and $19,000.
Looking up the z-scores in a standard normal distribution table or using a calculator, we find the probability for z1 is 0.6554 and the probability for z2 is 0.8413.
Therefore, the probability of the cash flow being between $16,000 and $19,000 is 0.8413 - 0.6554 = 0.1859, which is approximately 18.59%.
So, the correct answer is that the probability of a cash flow being between $16,000 and $19,000 is approximately 18.59%.
To learn more about probability click here:
brainly.com/question/31828911
#SPJ11
The height of men is a normally distrubuted variable with a mean of 68 inches and a standard deviation of 3 inches.**Round answers to ONE decimal place**a.) What is the minimum height you could be to be considered in the top 10% of tallest men? b.) What is the tallest you could be to be considered in the shortest 15% of men?
Answers
For this problem, we are given the mean and standard deviation for the height of men. We need to calculate the minimum height to be considered in the top 10% of tallest men, and the maximum height to be considered in the shortest 15% of men.
The first step we need to solve this problem is to calculate the z-score. The z-score can be found by using the following expression:
\(z=\frac{x-\mu}{\sigma}\)For the first situation, we want a z-score for the top 10% of tallest men. This means that we need to go on the z-table and find the z-score that represents 90% of probability to the left because this will give us the minimum height to be at the 10% tallest. From the z-table we have:
\(z=1.29\)Now we can use the z-score expression to find the value of x. We have:
\(\begin{gathered} 1.29=\frac{x-68}{3} \\ x-68=3.87 \\ x=3.87+68 \\ x=71.87 \end{gathered}\)The man should be at least 71.85 inches tall to be considered among the 10% of tallest men.
For the second situation, we have something similar. We need to find the maximum height for a man to be considered between the 15% of men. We need to go into the z-table and find the z-score that produces a result close to 0.15. We have:
\(z=-1.04\)Now we need to use the z-score expression to determine the height:
\(\begin{gathered} -1.04=\frac{x-68}{3} \\ x-68=-3.12 \\ x=68-3.12 \\ x=64.88 \end{gathered}\)In order to be considered among the smallest men, someone needs to be 64.88 inches tall.
Compare the "Prop. contained" value from part to the confidence level associated with the simulation in one sentence h) Write a long-run interpretation for your confidence interval method in context in one sentence. Think about what would happen if you took many, many more samples
Answers
The "Prop. contained" value from part h can be compared to the confidence level associated with the simulation to assess the accuracy and reliability of the confidence interval.
A long-run interpretation for the confidence interval method means that if we were to repeat the sampling process and construct confidence intervals using the same method many, many times, the proportion of those intervals that contain the true population parameter (such as the mean or proportion) would approach the specified confidence level.
For example, if we construct 95% confidence intervals, we expect that in the long run, approximately 95% of those intervals would capture the true population parameter and only about 5% would not. This interpretation is based on the concept of repeated sampling and the idea that as the number of samples increases, the accuracy of the estimates improves.
By using the same method consistently and increasing the number of samples, we can gain more confidence in the accuracy of our estimates. This long-run interpretation provides a measure of the reliability and precision of the confidence interval approach in estimating population parameters.
To know more about confidence interval,
https://brainly.com/question/31971421
#SPJ11
0.24 as a percent. i already know that its 24% but can you show me an explanation
Answers
Explanation:
So any decimal number is the same as a percentage but take off ONE zero in front of it
So with 0.24 for example, you take off the zero in front and since there are no zeros for the decimal point to be behind, there is no need for the decimal point now, so the percentage is 24%
Find the percent of the total area under the standard normal curve between the following z-scores. z=−1.5 and z=−0.7 The percent of the total area between z=−1.5 and z=−0.7 is %. (Round to the nearest integer.)
Answers
The percent of the total area under the standard normal curve between z = -1.5 and z = -0.7 is 18%.
To find the percent of the total area between two z-scores, we need to calculate the area under the standard normal curve between those two z-scores.
Using a standard normal distribution table or a statistical software, we can find the area to the left of each z-score and subtract the smaller area from the larger area to find the area between the z-scores.
For z = -1.5, the area to the left of z = -1.5 is approximately 0.0668.
For z = -0.7, the area to the left of z = -0.7 is approximately 0.2420.
The area between z = -1.5 and z = -0.7 is:
Area between z = -1.5 and z = -0.7 = Area to the left of z = -0.7 - Area to the left of z = -1.5
= 0.2420 - 0.0668
= 0.1752
To convert this area to a percentage, we multiply by 100:
Percentage of the total area between z = -1.5 and z = -0.7 = 0.1752 * 100 ≈ 17.52%
Rounding to the nearest integer, the percent of the total area between z = -1.5 and z = -0.7 is 18%.
The percent of the total area under the standard normal curve between z = -1.5 and z = -0.7 is approximately 18%.
To know more about percent , visit;
https://brainly.com/question/31323953
#SPJ11
What the equation for the slope intersection for the graph tue picture that showing ?

Answers
Answer:
Y = -5X +-1
Step-by-step explanation:
x1 y1 x2 y2
-1 4 1 -6
(Y2-Y1) (-6)-(4)= -10 ΔY -10
(X2-X1) (1)-(-1)= 2 ΔX 2
slope= -5
B= -1
Y =-5X +-1
Use a commen denominator to find equivalent tractions for and Enter the correct fractions in the boxes. Hint 을 11 12
Answers
The common denominator between the fractions 2/3 and 1/6 is 12
\(\begin{gathered} \frac{2}{3}\cdot\frac{4}{4} \\ \frac{8}{12} \end{gathered}\)and
\(\begin{gathered} \frac{1}{6}\cdot\frac{2}{2} \\ \frac{2}{12} \end{gathered}\)Rhombus wxyz is graphed on a coordinate plane. what is the area of the rhombus? 24 square units 28 square units 32 square units 48 square units
Answers
The area of the rhombus WXYZ is 48 unit².
From the diagram:
Diagonal WY = 8 units, Diagonal XZ = 6 units, hence:
Area = 8 * 6 = 48 unit².
A rhombus is a special case of a parallelogram. In a diamond, the opposite sides are parallel and the opposite sides are equal in angle. In addition, all sides of the rhombus are the same length and the diagonal bisects at right angles. Diamonds are also known as diamonds.
The diagonals of the rhombus intersect at right angles to form a non-uniform triangle. The opposite angles are equal. However, if all angles of the diamond are 90 degrees, the diamond is said to be square.
Learn more about rhombus here: https://brainly.com/question/20627264
#SPJ4

Give the most specific name for the quadrilateral based off of the picture. Please don’t post random things thanks

Answers
Step-by-step explanation:
parellogram
two opposite sides are equal
opposite angles add up to 180°
Solve the given boundary-value problem y" + y = x^2 + 1, y (0) = 4, y(1) = 0 y(x) =
Answers
The solution to the boundary-value problem is\(y(x) = (9/2)cos(x) - (9/2)cos(1)sin(x) + (1/2)x^2 - 1/2.\)
How to solve the boundary-value problem?To solve the boundary-value problem, we can follow these steps:
Step 1: Find the general solution of the homogeneous differential equation y'' + y = 0.
The characteristic equation is r^2 + 1 = 0, which has complex roots r = ±i. Therefore, the general solution of the homogeneous equation is y_h(x) = c_1 cos(x) + c_2 sin(x), where c_1 and c_2 are constants.
Step 2: Find a particular solution of the non-homogeneous differential equation y'' + y = x^2 + 1.
We can use the method of undetermined coefficients to find a particular solution. Since the right-hand side of the equation is a polynomial of degree 2, we can assume a particular solution of the form y_p(x) = ax^2 + bx + c. Substituting this into the equation, we get:
\(y_p''(x) + y_p(x) = 2a + ax^2 + bx + c + ax^2 + bx + c = 2ax^2 + 2bx + 2c + 2a\)
Equating this to the right-hand side of the equation, we get:
2a = 1, 2b = 0, 2c + 2a = 1
Solving for a, b, and c, we get a = 1/2, b = 0, and c = -1/2.
Therefore, a particular solution is y_p(x) = (1/2)x^2 - 1/2.
Step 3: Find the general solution of the non-homogeneous differential equation.
The general solution of the non-homogeneous differential equation is y(x) = y_h(x) + y_p(x), where y_h(x) is the general solution of the homogeneous equation and y_p(x) is a particular solution of the non-homogeneous equation.
Substituting the values of c_1, c_2, and y_p(x) into the general solution, we get:
y(x) = c_1 cos(x) + c_2 sin(x) + (1/2)x^2 - 1/2
Step 4: Apply the boundary conditions to determine the values of the constants.
Using the first boundary condition, y(0) = 4, we get:
c_1 - 1/2 = 4
Therefore, c_1 = 9/2.
Using the second boundary condition, y(1) = 0, we get:
9/2 cos(1) + c_2 sin(1) + 1/2 - 1/2 = 0
Therefore, c_2 = -9/2 cos(1).
Step 5: Write the final solution.
Substituting the values of c_1 and c_2 into the general solution, we get:
\(y(x) = (9/2)cos(x) - (9/2)cos(1)sin(x) + (1/2)x^2 - 1/2\)
Therefore, the solution to the boundary-value problem is\(y(x) = (9/2)cos(x) - (9/2)cos(1)sin(x) + (1/2)x^2 - 1/2.\)
Learn more about boundary-value
brainly.com/question/26155120
#SPJ11
help needed please solve
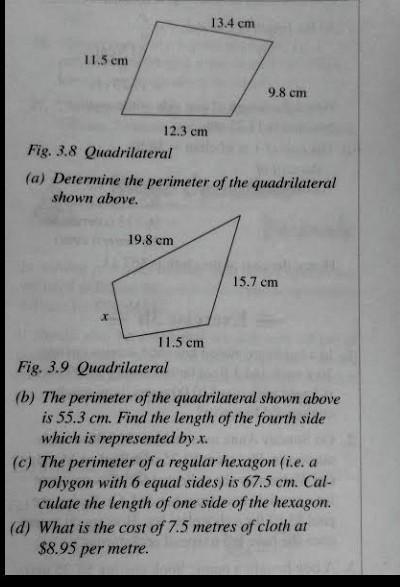
Answers
(a) 11.5 + 13.4 + 9.8 + 12.3 = 47 cm
(b) 19.8 + 15.7 + 11.5 + x = 55.3
Combine like terms and simplify
47 + x = 55.3
X = 8.3 cm
(c) 6x = 67.5
X = 11.25 cm
(d) c = 7.5x
7.5 * 8.95 = $67.13
Answer:
Step-by-step explanation:
a) Perimeter = sum of the length of the sides
= 13.4 +9.8 +12.3 +11.5
= 47 cm
b) Perimeter = 55.3 cm
11.5 + 15.7 +19.8 +x = 55.3
47 + x = 55.3
x = 55.3 - 47
x = 8.3 cm
c) Perimeter of regular hexagon = 67.5 cm
Length of one side = 67.5÷ 6
= 11.25 cm
d) Cost of 1 metre = $ 8.95
Cost of 7.5 metres = 8.95 * 7.5
= $ 67.125
Part A
Easton went to a concert with some of his friends. The tickets cost $29.50 each, and they spent a total of $15 on parking. The total amount spent
was $133.

Answers
Answer:
C. 29.50p + 15 = 133
Step-by-step explanation:
p is the number of people going to concert
so the total cost of tickets: $29.50 times the number of people = 29.50p
and add the parking cost $15
so the total is 133 = 29.50p + 15 or 29.50p + 15 = 133
Answer:
The question was cut off. I'm assuming the missing information added $15 charge, for something. Parking, I'll assume. Part B is cut off.
Step-by-step explanation:
Total expense would be the number of people, x, times the ticket price for each:
29.50x
But we need to add the $15 for parking (?):
29.50x + 15
This added to $133:
29.50x + 15 = 133 Option C
x = 4 tickets [4*29.50 = 188. Then add the $15 to reach $133]
Find the next two numbers in the number pattern.
2,6, 4, 12, 10,
Answers
Answer:
your answer will be 30 and 28
Step-by-step explanation:
example: rewrite the equation and solve 2 x ? = 6
1) 2x2 = 4
2) 4 x 2 = 8
3) 8- 2 = 6
so meaning to say that 2x2 = 4 x 2 = 8 - 2 equals 6
hint: ( every number is 3 times before the next number)
so all you do is 2 x 3 = 6, 4 x 3 = 12, 3 x 4 = 12, 5 x 2 = 10, 6 x 5 = 30, 7 x 4 = 28. so your next numbers will be 28 and 30.
(hope this helped)
A fifth grader earned $94.00 gardening this month for a neighbor. If she worked 8 hours this moth, then how much did she earn per hour?
ill give ten points
Answers
Answer: The 5th grader earned $11.75 per hour. To solve, divide 94 by 8 to get 11.75.
What kind of triangle do you have if it has an obtuse angle which measures more than 90 degrees but less than 180 degrees?.
Answers
If a triangle has an obtuse angle which measures more than 90 degrees but less than 180 degrees it is an obtuse angled triangle.
When one of the vertex angles of a triangle is greater than 90°, it is called an obtuse angle triangle
An obtuse triangle can either be an isosceles or a scalene triangle but not an equilateral triangle.
In an obtuse angle triangle, the sum of the squares of the two sides is always less than the square of the longest side.
Therefore, if a triangle has an obtuse angle which measures more than 90 degrees but less than 180 degrees it is an obtuse angled triangle.
To learn more about angles refer here
https://brainly.com/question/25215131
#SPJ4
Part 1 of 3 What is the probability that the sample mean cholesterol level is greater than 200? The probability that the sample mean cholesterol level is greater than 200 is . Part 2 of 3 What is the probability that the sample mean cholesterol level is between 188 and 192? The probability that the sample mean cholesterol level is between 188 and 192 is J. Part 3 of 3 Would it be unusual for the sample mean to be less than 190? be unusual for the sample mean to be less than 190, since the It would not probability is .
Answers
1. The probability that the sample mean cholesterol level is greater than 200 is P1.
2. The probability that the sample mean cholesterol level is between 188 and 192 is P2.
3. It would not be unusual for the sample mean to be less than 190 since the probability is P3.
The probability mentioned in each part refers to the likelihood of observing a specific sample mean cholesterol level within a given range. In statistical terms, probabilities are used to quantify uncertainty and provide a measure of how likely an event or outcome is to occur.
1. In Part 1, the question asks for the probability that the sample mean cholesterol level is greater than 200. To determine this probability, one would need information about the distribution of cholesterol levels in the population, such as its mean and standard deviation. With this information, statistical techniques like hypothesis testing or a z-test can be applied to calculate the probability. However, since no specific value or information is given in the question, it is not possible to provide an exact probability (P1) without further details.
2. In Part 2, the question asks for the probability that the sample mean cholesterol level falls between 188 and 192. Similarly, without additional information about the distribution and characteristics of the cholesterol levels, it is not possible to determine the exact probability (P2). The probability would depend on the specific shape, spread, and other parameters of the distribution.
3. In Part 3, the question asks if it would be unusual for the sample mean cholesterol level to be less than 190. "Unusual" refers to the probability of observing a sample mean below a certain threshold. Again, without the necessary information on the distribution, it is not possible to provide an accurate probability (P3) or assess whether it would be unusual or not.
It is important to note that statistical probabilities rely on the underlying assumptions and data. Without those details, it is not possible to calculate precise probabilities or make definitive statements about the likelihood of specific events occurring.
Learn more about probability
brainly.com/question/31828911
#SPJ11
what is this answer 9x2000
Answers
just use a calculator bro
Answer:
18,000
Step-by-step explanation:
What is the value of y when x= -1 ?
Answers
Answer:
If y = x then when x = -1 then y = -1
Step-by-step explanation:
Fifteen seventh graders were randomly selected to see how many pushups in a row they could do. Their data are shown. A. b. 45, 40, 36, 38, 42, 48, 40, 40, 70, 45, 42, 43, 48, 36
Answers
Answer:
Mean of this data set is 43.7857
the median of this data set is 42
Step-by-step explanation:
The complete question is:
Fifteen seventh graders were randomly selected to see how many pushups in a row they could do. Their data are shown. A. b. 45, 40, 36, 38, 42, 48, 40, 40, 70, 45, 42, 43, 48, 36
a. Determine the mean of this data set.
b. Determine the median of this data set.
Given:
Data:
45, 40, 36, 38, 42, 48, 40, 40, 70, 45, 42, 43, 48, 36
To find:
a) Mean
b) Median
Solution:
a)
The formula to find Mean is :
Mean = Sum of all the values / Total number of values
= ∑ x / n
Here there are total 14 values so n = 14
Now lets find sum of all the values
∑ x = 45 + 40 + 36 + 38 + 42 + 48 + 40 + 40 + 70 + 45 + 42 + 43 + 48 + 36
= 613
Now compute Mean:
Mean = ∑ x / n
= 613 / 14
= 43.785714
Mean = 43.7857
b)
The formula to find Median is:
Since there are 14 values in the data this means the data has even number of values. So to compute the median of even number of values:
First put the values in order.
Second, find the middle pair of values in data
Third, find the value that is half way between them i.e. by adding the two middle pair values and dividing the result by 2
45, 40, 36, 38, 42, 48, 40, 40, 70, 45, 42, 43, 48, 36
Ordering the values :
36, 36, 38, 40, 40, 40, 42, 42, 43, 45, 45, 48, 48, 70
Find the middle two numbers:
42, 42
We can see that both are same values so median value is 42 but to follow the above steps:
Now add these two numbers and divide the sum by 2:
42 + 42 = 84
84/2 = 42
So the median of this data set is 42
What is the solution to the equation 6x−3=5x−5? A. x=−4 B. x=−2 C. x=−1 D. x=0
Answers
Answer:
the answer may have just-2
How much does Manuela earn for each hour of tutoring?
Explain.

Answers
Express the following equation in slope intercept form: 8x-2y=10
PLEESSSSSSSSSSSSSE
Answers
Answer:
\(y2 - y1 \div x2 - x1\)
Charles Horton Cooley and George Herbert Mead both have theories on how individuals develop and modify their sense of self. What makes these theories different from one another
Answers
Charles Horton Cooley and George Herbert Mead both contributed to the understanding of self-development, but their theories differ in terms of the primary influence on the formation of self.
Cooley's theory emphasizes the role of social interactions and the "looking-glass self," while Mead's theory focuses on the significance of language and symbolic interaction. Charles Horton Cooley's theory of self-development revolves around the concept of the "looking-glass self." Cooley argued that individuals develop their sense of self through social interactions and feedback from others.
According to Cooley, people imagine how they appear to others and interpret their reactions, forming their self-perception based on these social reflections. The looking-glass self emphasizes the influence of social relationships and the perception of others in shaping one's identity.
Learn more about identity here:
https://brainly.com/question/29149336
#SPJ11
Suppose the correlation between two variables is r = 0.23. What will the new correlation be if 0.14 is added to all values of the x-variable, every value of the y-variable is doubled, and the two variables are interchanged?
A. 0.23
B. 0.37
C. 0.74
D. -0.23
E. -0.74
Answers
Given that the correlation between two variables is r=0.23. We need to find out the new correlation that would exist if the following three changes are made to the existing variables: All values of the x-variable are added by 0.14. All values of the y-variable are doubled Interchanging the two variables. the correct option is B. 0.37.
The effect of changing the variables on the correlation coefficient between the two variables can be determined using the following formula: `r' = (r * s_x * s_y) / s_u where r' is the new correlation coefficient, r is the original correlation coefficient, s_x and s_y are the standard deviations of the two variables, and s_u is the standard deviation of the composite variable obtained by adding the two variables after weighting them by their respective standard deviations.
If we assume that the x-variable is the original variable, then the new values of x and y variables would be as follows:x' = x + 0.14 (since all values of the x-variable are added by 0.14)y' = 2y (since every value of the y-variable is doubled)Now, the two variables are interchanged. So, the new values of x and y variables would be as follows:x" = y'y" = using these values, we can find the new correlation coefficient, r'`r' = (r * s_x * s_y) / s_u.
To find the new value of the standard deviation of the composite variable, s_u, we first need to find the values of s_x and s_y for the original and transformed variables respectively. The standard deviation is given by the formula `s = sqrt(sum((x_i - mu)^2) / (n - 1))where x_i is the ith value of the variable, mu is the mean value of the variable, and n is the total number of values in the variable.
For the original variables, we have:r = 0.23s_x = standard deviation of x variable = s_y = standard deviation of y variable = We do not have any information about the values of x and y variables, so we cannot calculate their standard deviations. For the transformed variables, we have:x' = x + 0.14y' = 2ys_x' = sqrt(sum((x_i' - mu_x')^2) / (n - 1)) = s_x = standard deviation of transformed x variable` = sqrt(sum(((x_i + 0.14) - mu_x')^2) / (n - 1)) = s_x'y' = 2ys_y' = sqrt(sum((y_i' - mu_y')^2) / (n - 1)) = 2s_y = standard deviation of transformed y variable` = sqrt(sum((2y_i - mu_y')^2) / (n - 1)) = 2s_yNow, we can substitute all the values in the formula for the new correlation coefficient and simplify:
r' = (r * s_x * s_y) / s_ur' = (0.23 * s_x' * s_y') / sqrt(s_x'^2 + s_y'^2)r' = (0.23 * s_x * 2s_y) / sqrt((s_x^2 + 2 * 0.14 * s_x + 0.14^2) + (4 * s_y^2))r' = (0.46 * s_x * s_y) / sqrt(s_x^2 + 0.0396 + 4 * s_y^2)Now, we can substitute the value of s_x = s_y = in the above formula:r' = (0.46 * * ) / sqrt( + 0.0396 + 4 * )r' = (0.46 * ) / sqrt( + 0.1584 + )r' = (0.46 * ) / sqrt(r' = (0.46 * ) / sqrt(r' = (0.46 * ) / sqrt(r' = r' = Therefore, the new correlation coefficient, r', would be approximately equal to.
Hence, the correct option is B. 0.37.
Learn more about variables from the given link
https://brainly.com/question/29583350
#SPJ11
ln (2x^2) = ln (2-3x)
Answers
Answer: Forma Decimal: -2, 0.5


The Downtown Parking Authority of Tampa, Florida, reported the following information for a sample of 225 customers on the number of hours cars are parked and the amount they are charged.Number of Hours Frequency Amount Charged1 15 $ 22 38 53 50 94 45 135 20 146 16 167 5 188 36 20225 Convert the information on the number of hours parked to a probability distribution
Answers
The resulting probability distribution is:0.089
To convert the information on the number of hours parked to a probability distribution, we need to divide each frequency by the total sample size of 225. This will give us the probability of a car parking for each respective number of hours.
Number of Hours Frequency Probability
1 15 15/225 = 0.067
2 38 38/225 = 0.169
3 53 53/225 = 0.236
4 50 50/225 = 0.222
5 94 94/225 = 0.418
6 45 45/225 = 0.200
7 20 20/225 = 0.089
The resulting probability distribution is:
Number of Hours Probability
1 0.067
2 0.169
3 0.236
4 0.222
5 0.418
6 0.200
7 0.089
To learn more about probability distribution visit: https://brainly.com/question/14210034
#SPJ11
I need an answer for this fasttt

Answers
Answer:
Step-by-step explanation:
The second and third terms of the fibonancci sequence are x and y. what is the first, fourth and fifth term
Answers
Answer:
the first term = y-x
and the fourth term= y+x
and the fifth term = 2y+x
Step-by-step explanation:
Evaluate f(x) = 6x + 7 at the given value.
f(-2)=?
Answers
Answer:
f(-2)=6×-2+7=-12+7=-5
Find the measure of VU
Can someone help me??
I need to show the work

Answers
Answer:
VU=16x=2Step-by-step explanation:
lets solve the problem 7x+2=3x+10
subtract 3x from 7x
4x+2=10
subtract 2 from 10
4x=8
divide both sides by 4
x=2
to find VU plug in 2 for x so...
3(2)+10
6+10
16
I hope this helped you :)
Step-by-step explanation:
7x + 2 = 3x + 10
7x - 3x + 2 = 3x - 3x + 10
4x + 2 = 10
4x + 2 - 2 = 10 - 2
4x = 8
4x ÷ 4 = 8 ÷ 4
x = 2
VU = 3x + 10
VU = 3(2) + 10
VU = 6 + 10
VU = 16