Andre is paid $12 per hour at his caddying job and $9.50 per hour working at a local fast food restaurant. He would like to keep both jobs and earn at least $275.00 a week, but he can work no more than 25 hours. If x represents the number of hours Andre works at his caddying job and y represents the number of hours he works at the restaurant, which system models this scenario?
Answers
The equation that can be used to model this scenario is 12x + 9.5y ≥ 275 and x + y ≤ 25
Let x represents the number of hours Andre works at his caddying job and y represents the number of hours he works at the restaurant.
Since he earn at least $275.00 a week, hence:
12x + 9.5y ≥ 275 (1)
Also, he can work no more than 25 hours, hence:
x + y ≤ 25 (2)
Therefore the equation that can be used to model this scenario is 12x + 9.5y ≥ 275 and x + y ≤ 25
Find out more at: https://brainly.com/question/25285332
Related Questions
A random number generator is used to select a number from 1 to 100. What is the probability of selecting an even number?
0.95
0.005
0.50
0.25
Answers
The probability of selecting an even number from 1 to 100 is C) 0.5, or 50%.
There are 100 numbers to choose from, and half of them (50) are even numbers (2, 4, 6, 8, ... 98, 100), while the other half are odd numbers (1, 3, 5, 7, ... 97, 99). Since each number has an equal chance of being selected, the probability of selecting an even number is equal to the ratio of the number of even numbers to the total number of possible choices, which is 50/100 or 0.5. This means that in the long run, if we were to select numbers randomly from 1 to 100 using the same method, we would expect to get an even number about half of the time.
For more questions like Probability visit the link below:
https://brainly.com/question/26944192
#SPJ11
2.
Solve: 4!
24
1
6
16
Answers
Answer:
24
Step-by-step explanation:
4! means 4 'factorial' which means to start multiplying backwards from 4 until you reach 1
4 x 3 x 2 x 1 = 24
Xavier is buying a new pair of snowshoes. They are regularly $85, but they are on sale for $68. What percent is the markdown?
(PLEASE PUT HOW YOU GOT THE ANSWER ASWELL, I’LL MAKE YOU BRAINLEST!!)
Answers
Answer:
The percent of the markdown price is 20%.
Step-by-step explanation:
$85 x 20% = $17
you subtract $17 from $85 and get $68 !
U welcome :) have a great day !
The markdown percent is 20%.
What is percentage?A relative value indicating hundredth parts of any quantity.
Given that, the original price of a pair of shoes is $85 but at sale it was $68,
Markdown percent = \(\frac{original price - current price}{original price} *100\)
= (85-68)/85*100
= 17/85*100
= 20%
Hence, The markdown percent is 20%.
For more references on percentage, click;
https://brainly.com/question/29306119
#SPJ2
HELP URGENT!! the data set for a random sample of 10 soccer players heights compared to their shoe sizes is summarized in the table.
questions included in the photo.

Answers
Part A: The equation of least squares regression line is
ŷ = 0.4684X + 64.69888
Part B: The residual that we have here is - 1.382
Part C: Residual refers to the difference between the observed value and the predicted value
How to solve for the residualSum of X = 102.5
Sum of Y = 695
Mean X = 10.25
Mean Y = 69.5
Sum of squares (SSX) = 33.625
Sum of products (SP) = 15.75
Regression Equation = ŷ = bX + a
b = SP/SSX = 15.75/33.63 = 0.4684
a = MY - bMX = 69.5 - (0.47*10.25) = 64.69888
ŷ = 0.4684X + 64.69888
Residual = observed - predicted
The observed = 68
The predicted = 0.4684 x 10 + 64.69888
= 4.684 + 64.69888
= 69.382
residual = 68 - 69.382
= -1.38
Read more on random sample here :
https://brainly.com/question/17831271
#SPJ1
Use the functions and the scenario to solve the problem.
f(x)=1/2x+6
g(x)=x−3
Shiloh and Leanne are asked to evaluate f(x)−g(x).
Shiloh suggests f(x)−g(x)=(x−3)−(1/2x+6)=1/2x−9.
Leanne suggests f(x)−g(x)=(12x+6)−(x−3)=−12x+9.
Evaluate each student's answer.
Select two answers. Select the correct evaluation of Shiloh's work and the correct evaluation of Leanne's work.
Answers
The function f(x) - g(x) is an illustration of a composite function
Leanne's end result of f(x) - g(x) = -1/2x + 9 is correct
How to estimate the student's answer?The functions are given as:
f(x) = 1/2x + 6
g(x) = x - 3
The function f(x) - g(x) is calculated using:
f(x) - g(x) = 1/2x + 6 - x + 3
Collect like terms
f(x) - g(x) = 1/2x - x + 6 + 3
Evaluate the like terms
f(x) - g(x) = -1/2x + 9
By comparing the above solution to the students' response, we can see that:
Leanne's end result of f(x) - g(x) = -1/2x + 9 is correct
Read more about composite functions at:
https://brainly.com/question/13502804
Find the area of this kite.
3 m
5 m
6 m
5 m
Answers
Answer:
450m
Step-by-step explanation:
Create another line perpendicular to AB passing through C on AB. Measure the angle at the intersection to verify your result. Take a screenshot of your work, and paste it below.
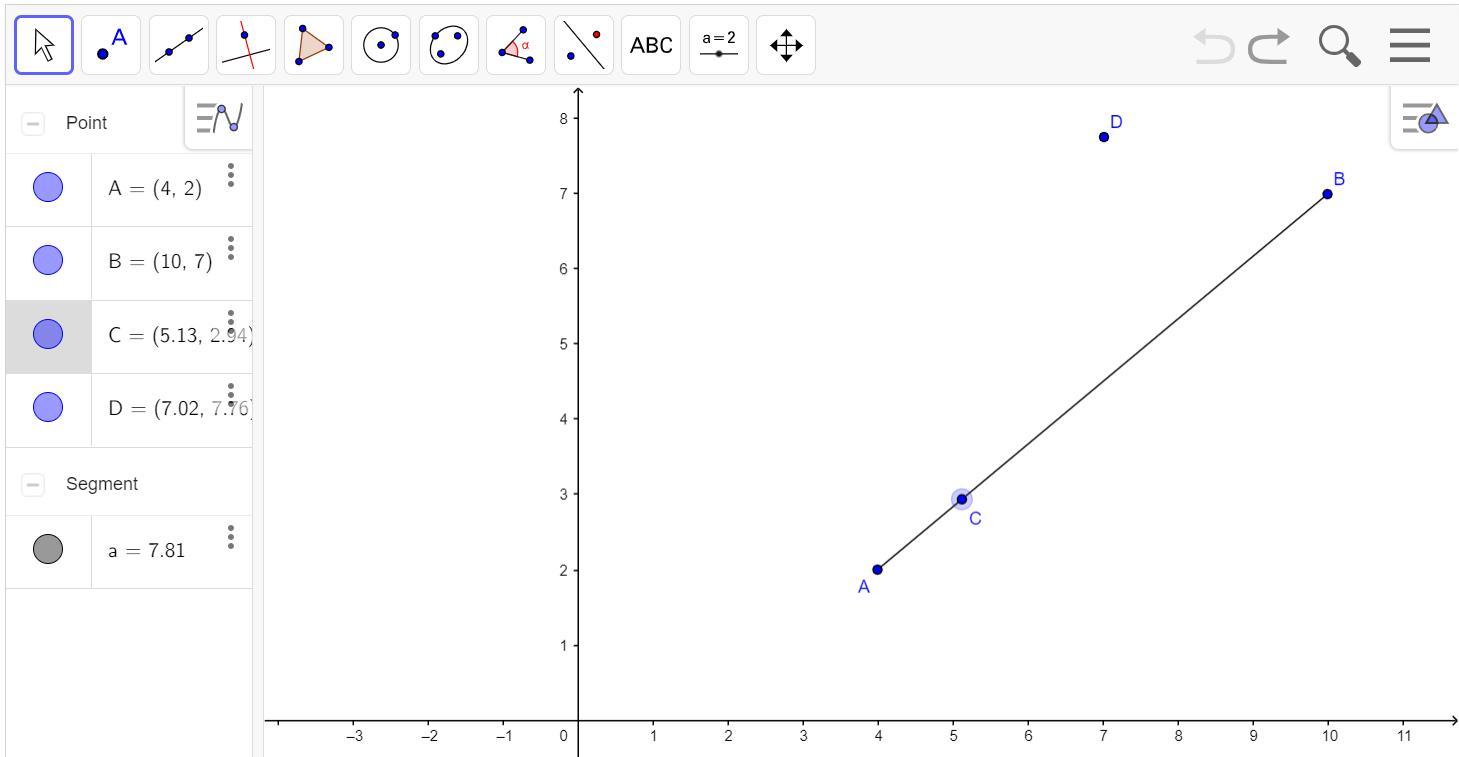
Answers
Line passing through AB is perpendicular. A pair of angles that are linear have an angle sum of 180 degrees. The lines are therefore shown to be perpendicular to one another because the angles are 90 degrees in size.
Define perpendicular.Two lines intersect to generate perpendicular lines, and the angle formed between the two lines must be 90 degrees (right angle).
Given,
Create another line perpendicular to AB passing through C on AB..
Line passing through AB is perpendicular.
Two lines are perpendicular if they cross at a point where a pair of linear congruent angles results. In a plane, a transversal is perpendicular to the other line if it is parallel to one of the two parallel lines.
A pair of angles that are linear have an angle sum of 180 degrees. The lines are therefore shown to be perpendicular to one another because the angles are 90 degrees in size. The linear pair perpendicular theorem is demonstrated by this.
To learn more about perpendicular, visit:
https://brainly.com/question/11707949
#SPJ1

what do all the captain codes of ethnics have in common ?A.they all identify four or ethnic or principles of accounting B.they all spend hundreds of pages covering confidentiality C.they all go into great detail on the responsibilities of accountants D. they all separate accounting principles into three categories (just answer)

Answers
An accountant’s ethical code of conduct represents the moral values, principles, and rules that accountants should have.
The ethical codes of conduct of AICPA and IFAC are the two main codes most countries adopt to guide their members on how to deal with accounting information from an ethical perspective.
The fundamental principles within the Code – integrity, objectivity, professional competence and due care, confidentiality, and professional behavior – establish the standard of behavior expected of a professional accountant (PA) and it reflects the profession’s recognition of its public interest responsibility.
The common thing in all accounting codes of conduct is that "they all go into great detail on the responsibilities of accountants."
Therefore, the correct option is OPTION
a $4\times 4\times 4$ open cubical box contains $64$ identical small cubes that exactly fill the box. how many of these small cubes touch the bottom or one of the four lateral sides of the box?
Answers
Total number of identical cubes that touch he bottom or one of the four lateral sides of the box is 52
4 x 4 x 4 open cubical box contains 64 identical small cubes that exactly fill the box
The 2 ends = 16 each small identical cubes touch the end sides
2*16 = 32
The 2 sides=8 each small identical cubes touch the lateral sides
2*8=16
On The bottom there are four small identical cubes
Total number of identical cubes that touch he bottom or one of the four lateral sides of the box will be
32 + 16 + 4 = 52
Thwerefore, Total number of identical cubes that touch he bottom or one of the four lateral sides of the box is 52
To learn more about squares refer here
https://brainly.com/question/22744289
#SPJ4
A triangle has side lengths of 10 inches, 11 inches, and 12 inches. What kind of triangle is it?
Answers
Answer:
Acute scalene triangle.
More Information:
Sides: a = 10 b = 11 c = 12
Area: T = 51.521
Perimeter: p = 33
Semiperimeter: s = 16.5
Angle ∠ A = α = 51.318° = 51°19'4″ = 0.896 rad
Angle ∠ B = β = 59.17° = 59°10'10″ = 1.033 rad
Angle ∠ C = γ = 69.513° = 69°30'46″ = 1.213 rad
Height: ha = 10.304
Height: hb = 9.367
Height: hc = 8.587
Median: ma = 10.368
Median: mb = 9.579
Median: mc = 8.631
Inradius: r = 3.122
Circumradius: R = 6.405
Vertex coordinates: A[12; 0] B[0; 0] C[5.125; 8.587]
Centroid: CG[5.708; 2.862]
Coordinates of the circumscribed circle: U[6; 2.242]
Coordinates of the inscribed circle: I[5.5; 3.122]
Exterior (or external, outer) angles of the triangle:
∠ A' = α' = 128.682° = 128°40'56″ = 0.896 rad
∠ B' = β' = 120.83° = 120°49'50″ = 1.033 rad
∠ C' = γ' = 110.487° = 110°29'14″ = 1.213 rad
How to Solve:
The calculation of the triangle has two phases. The first phase calculates all three sides of the triangle from the input parameters. The first phase is different for the different triangles query entered. The second phase calculates other triangle characteristics, such as angles, area, perimeter, heights, the center of gravity, circle radii, etc. Some input data also results in two to three correct triangle solutions (e.g., if the specified triangle area and two sides - typically resulting in both acute and obtuse) triangle).
1. Input data entered: sides a, b, and c.
a=10
b=11
c=12
We know the lengths of all three sides of the triangle, so the triangle is uniquely specified. Next, we calculate another of its characteristics - the same procedure for calculating the triangle from the known three sides SSS.
a=10
b=11
c=12
2. The triangle perimeter is the sum of the lengths of its three sides
3. Semiperimeter of the triangle
The semiperimeter of the triangle is half its perimeter. The semiperimeter frequently appears in formulas for triangles to be given a separate name. By the triangle inequality, the longest side length of a triangle is less than the semiperimeter.
4. The triangle area using Heron's formula
Heron's formula gives the area of a triangle when the length of all three sides is known. There is no need to calculate angles or other distances in the triangle first. Heron's formula works equally well in all cases and types of triangles.
5. Calculate the heights of the triangle from its area.
There are many ways to find the height of the triangle. The easiest way is from the area and base length. The triangle area is half of the product of the base's length and height. Every side of the triangle can be a base; there are three bases and three heights (altitudes). Triangle height is the perpendicular line segment from a vertex to a line containing the base.
6. Calculation of the inner angles of the triangle using a Law of Cosines
The Law of Cosines is useful for finding a triangle's angles when we know all three sides. The cosine rule, also known as the Law of Cosines, relates all three sides of a triangle with an angle of a triangle. The Law of Cosines extrapolates the Pythagorean theorem for any triangle. Pythagorean theorem works only in a right triangle. Pythagorean theorem is a special case of the Law of Cosines and can be derived from it because the cosine of 90° is 0. It is best to find the angle opposite the longest side first. With the Law of Cosines, there is also no problem with obtuse angles as with the Law of Sines because the cosine function is negative for obtuse angles, zero for right, and positive for acute angles. We also use inverse cosine called arccosine to determine the angle from the cosine value.
7. Inradius
An incircle of a triangle is a tangent circle to each side. An incircle center is called an incenter and has a radius named inradius. All triangles have an incenter, and it always lies inside the triangle. The incenter is the intersection of the three-angle bisectors. The product of the inradius and semiperimeter (half the perimeter) of a triangle is its area.
8. Circumradius
The circumcircle of a triangle is a circle that passes through all of the triangle's vertices, and the circumradius of a triangle is the radius of the triangle's circumcircle. The circumcenter (center of the circumcircle) is the point where the perpendicular bisectors of a triangle intersect.
9. Calculation of medians
A median of a triangle is a line segment joining a vertex to the opposite side's midpoint. Every triangle has three medians, and they all intersect each other at the triangle's centroid. The centroid divides each median into parts in the ratio of 2:1, with the centroid being twice as close to the midpoint of a side as it is to the opposite vertex. We use Apollonius's theorem to calculate the length of a median from the lengths of its side.
Learn More: https://brainly.com/question/28937541

For the set of triangle vertices, find the coordinates of the vertices of the image after a dilation by the given scale factor and center of dilation. Thern graph the preimage and image on a separate sheet of paper. J(-8, 0), K(-4, 4), L(-2, 0), k = 0.5. centered at the origin
Answers
The coordinates of the vertices of the image triangle are J'(-4, 0), K'(-2, 2), and L'(-1, 0).
To find the coordinates of the vertices of the image after dilation by a scale factor of 0.5 centered at the origin, we multiply each coordinate by the scale factor.
Let's apply the dilation to each vertex:
J' = (-8 * 0.5, 0 * 0.5) = (-4, 0)
K' = (-4 * 0.5, 4 * 0.5) = (-2, 2)
L' = (-2 * 0.5, 0 * 0.5) = (-1, 0)
Therefore, the coordinates of the vertices of the image triangle are J'(-4, 0), K'(-2, 2), and L'(-1, 0).
To graph the preimage and image, you can plot the points J(-8, 0), K(-4, 4), and L(-2, 0) on one sheet of paper to represent the preimage triangle. Then, on a separate sheet of paper, plot the points J'(-4, 0), K'(-2, 2), and L'(-1, 0) to represent the image triangle. Make sure to label each vertex accordingly.
Learn more about scale factor visit:
brainly.com/question/29464385
#SPJ11
Consider the complex number ^w = , where z = x+iy and I = square root of -1. a) If w = I, determine z in the form z = r cos theta. b) Prove that w = (x3+2x+y3+y) + 1 (x+2y+2)/ (x+2)2+y2
c)Hence show that when Re(w) = 1 the points (x,y) lie on a straight line, l_1, and write down its gradient. d)Given arg(z)= arg(w) = pie/4,
Answers
a) If w = I, then we can write w = 0 + I. This means that x = 0 and y = 1. Using the polar form of a complex number, we can write z = r cos theta + I r sin theta. Since x = 0 and y = 1, we can write z = r cos theta + I r sin theta = 0 + I. This means that r cos theta = 0 and r sin theta = 1. Since cos theta = 0, theta = pi/2. Therefore, z = r cos (pi/2) = I.
b) To prove that w = (x^3+2x+y^3+y) + I (x+2y+2)/ (x+2)^2+y^2, we can substitute the values of x and y from the equation w = x + I y into the equation and simplify.
w = (x^3+2x+y^3+y) + I (x+2y+2)/ (x+2)^2+y^2
= (x^3+2x+y^3+y) + I (x+2y+2)/ (x^2+4x+4+y^2)
= (x^3+2x+y^3+y) + I (x+2y+2)/ (x^2+4x+4+y^2)
= (x^3+2x+y^3+y) + I (x+2y+2)/ (x^2+4x+4+y^2)
= w
Therefore, the equation is true.
c) When Re(w) = 1, this means that the real part of w is equal to 1. Therefore, x^3+2x+y^3+y = 1. We can rearrange this equation to get y^3+y = 1-x^3-2x. Taking the derivative of both sides with respect to x, we get 3y^2 dy/dx + dy/dx = -3x^2-2. Solving for dy/dx, we get dy/dx = (-3x^2-2)/(3y^2+1). This is the gradient of the line l_1.
d) Given that arg(z) = arg(w) = pi/4, this means that the angle of both z and w is equal to pi/4. Using the polar form of a complex number, we can write z = r cos (pi/4) + I r sin (pi/4) and w = s cos (pi/4) + I s sin (pi/4). Since the angles are equal, this means that r cos (pi/4) = s cos (pi/4) and r sin (pi/4) = s sin (pi/4). Therefore, r = s and z = w.
Learn more bout complex number
brainly.com/question/20566728
#SPJ11
The area of the shaded sector is shown.

Answers
Answer:
3.99
Step-by-step explanation:
The total sum of central angle of circle is 360 which mean the area of the circle = (12.36 x 360)/89
A=πr^2
=> (12.36 x 360)/89 = 3.14(r^2)
r^2 = 15.92
r = 3.99
For one Midwest city, meteorologists believe the distribution of four-week summer rainfall is given as follows: 39% 32% 16% 13%
Answers
The expected value of the four-week summer rainfall in the Midwest city is 1.39 units. This value can be used to predict the rainfall for the city in the future.
In this case, we can calculate the expected rainfall using the formula. Expected value = (1 * probability of occurrence) + (2 * probability of occurrence) + (3 * probability of occurrence) + (4 * probability of occurrence). Meteorologists believe the distribution of four-week summer rainfall for one Midwest city is given as follows: 39% 32% 16% 13%.
Here, the expected value is given as:Expected value = (1 * 0.39) + (2 * 0.32) + (3 * 0.16) + (4 * 0.13).
Expected value = 1.39, which means the expected value of the four-week summer rainfall in the Midwest city is 1.39 units. This value can be used to predict the rainfall for the city in the future.
The expected value is not necessarily the actual value that will be observed, but it is the average value that can be expected over a long period of time.
To know more about Probability of occurrence visit-
brainly.com/question/24173730
#SPJ11
What is the symbol used to represent the population mean?
Answers
The population mean, or average score for the population on a certain variable, is symbolised as follows:μ = ( Σ Xi ) / N.
What is mean?The mean of a data set is the sum of all values divided by the total number of values, often called the arithmetic mean (as opposed to the geometric mean). Often referred to as the "mean", it is the most commonly used measure of central tendency. This result is obtained by dividing the total number of values in the data set by the sum of all those values. Both raw data and data combined into frequency tables can be used in calculations. Mean refers to the average of a number. The calculation is simple: divide by the number of numbers when you added all the numbers. the total divided by the number.
The population mean or population mean score for a given variable is symbolized as follows:μ = ( Σ Xi ) / N. The symbol 'μ' represents the population mean.
To know more about mean visit:
brainly.com/question/30094057
#SPJ4
Find the slope and y-intercept for the given linear inequalities;then graphs the given linear inequalities. 1. 6>2x+y m=? b=? 2. x<3y-6 m=? b=?
Answers
Answer:
6 > 2x + y
To find the slope and y-intercept for this linear inequality, we need to write it in slope-intercept form, which is y = mx + b, where m is the slope and b is the y-intercept.
Rearranging the given inequality, we get:
y > -2x + 6
Comparing this with y = mx + b, we can see that the slope is -2 and the y-intercept is 6.
So, the slope is m = -2 and the y-intercept is b = 6.
To graph this linear inequality, we can draw the line y = -2x + 6 (which has a slope of -2 and y-intercept of 6) as a dashed line (since y > -2x + 6, not y = -2x + 6). Then we shade the region above the line, since all the points in that region satisfy the inequality y > -2x + 6.
x < 3y - 6
Again, we need to write this inequality in slope-intercept form to find the slope and y-intercept. Rearranging, we get:
3y > x + 6
Dividing both sides by 3, we get:
y > (1/3)x + 2
So, the slope is m = 1/3 and the y-intercept is b = 2.
To graph this linear inequality, we draw the line y = (1/3)x + 2 (which has a slope of 1/3 and y-intercept of 2) as a dashed line (since x < 3y - 6, not x = 3y - 6). Then we shade the region below the line, since all the points in that region satisfy the inequality x < 3y - 6.
Your friend is celebrating her 25 th birthday today and wants to start saving for her anticipated retirement at age 65 . She wants to be able to withdraw $250,000 from her saving account on each birthday for 20 years following her retirement; the first withdrawal will be on her 66th birthday. Your friend intends to invest her money in a retirement account, which earns 8 percent return per year. She wants to make an equal annual deposit on each birthday into the account for her retirement fund. Assume that the annual return on the retirement account is 8 percent before retirement and 5 percent after retirement. If she starts making these deposits on her 26 th birthday and continue to make deposits until she is 65 (the last deposit will be on her 65 th birthday and the total number of annual deposits is 40), what amount must she deposit annually to be able to make the desired withdrawals at retirement? (Hint: One way to solve for this problem is to first find the value on your friend's 65 th birthday of the $250,000 withdrawal per year for 20 years after her retirement using the annual return after retirement and then find the equal annual deposit that she needs to make from her 26th birthday to 65 th birthday using the annual return before retirement.) Ignore taxes and transaction costs for the problem.
Answers
The correct answer is your friend needs to deposit approximately $13,334.45 annually from her 26th birthday to her 65th birthday to be able to make the desired withdrawals at retirement.
To determine the annual deposit your friend needs to make for her retirement fund, we'll calculate the present value of the desired withdrawals during retirement and then solve for the equal annual deposit.
Step 1: Calculate the present value of the withdrawals during retirement
Using the formula for the present value of an annuity, we'll calculate the present value of the $250,000 withdrawals per year for 20 years after retirement.
\(PV = CF * [1 - (1 + r)^(-n)] / r\)
Where:
PV = Present value
CF = Cash flow per period ($250,000)
r = Rate of return after retirement (5%)
n = Number of periods (20)
Plugging in the values, we get:
PV = $250,000 * \([1 - (1 + 0.05)^(-20)] / 0.05\)
PV ≈ $2,791,209.96
Step 2: Calculate the equal annual deposit before retirement
Using the formula for the future value of an ordinary annuity, we'll calculate the equal annual deposit your friend needs to make from her 26th birthday to her 65th birthday.
\(FV = P * [(1 + r)^n - 1] / r\)
Where:
FV = Future value (PV calculated in Step 1)
P = Payment (annual deposit)
r = Rate of return before retirement (8%)
n = Number of periods (40)
Plugging in the values, we get:
$2,791,209.96 = \(P * [(1 + 0.08)^40 - 1] / 0.08\)
Now, we solve for P:P ≈ $13,334.45
Therefore, your friend needs to deposit approximately $13,334.45 annually from her 26th birthday to her 65th birthday to be able to make the desired withdrawals at retirement.
Learn more about compound interest here:
https://brainly.com/question/24274034
#SPJ11
Ummm i know the answer isn't 4 pls help

Answers
Answer:
11
4m-28 = 16
4m = 44
m=11
Step-by-step explanation:
Bags of limes = 4
Total limes in each bag = m
Total limes = 4m
ATQ
4m - 7(4)= 16
4m = 16+28
4m = 44
m = 44/4
m = 11
Value of m = 11
Must click thanks and mark brainliest
evaluate the scalar line integral ∫ c (3x y) ds, where c is the line segment from (−1,3) to (4,2).
Answers
The scalar line integral ∫ c (3x y) ds, where c is the line segment from (−1,3) to (4,2) is equal to 78√26/5
To evaluate the scalar line integral ∫ c (3x y) ds, where c is the line segment from (−1,3) to (4,2), we first need to parameterize the curve c.
Let t be the parameter such that
x = -1 + 5t,
y = 3 - t,
for 0 ≤ t ≤ 1.
The length of the curve c is given by the integral ∫ c ds, which can be calculated using the formula ∫ a to b \(√(dx/dt)^2 + (dy/dt)^2\) dt. Plugging in the values from the parameterization, we get
\(∫ c ds = ∫ 0 to 1 √(5^2 + (-1)^2) dt = ∫ 0 to 1 √26 dt = √26.\)
Using the parameterization, we can now write the integral as
\(∫ c (3x y) ds = ∫ 0 to 1 (3(-1+5t)(3-t)) √(5^2 + (-1)^2) dt = 78√26/5.\)
Therefore, the scalar line integral ∫ c (3x y) ds, where c is the line segment from (−1,3) to (4,2) is equal to 78√26/5.
Learn more about the scalar line integral
https://brainly.com/question/31392715
#SPJ4
The scalar line integral ∫ c (3x y) ds, where c is the line segment from (−1,3) to (4,2), is approximately equal to
22.229.
We can do this by letting x = t and y = 3 - t/2, where -1 ≤ t ≤ 4.
Then, we can find ds/dt using the formula \(ds/dt = \sqrt{(dx/dt^2 + dy/dt^2)}\), which simplifies to
\(ds/dt = \sqrt{(1 + 1/4) } = \sqrt{(5)/2} .\)
Next, we can substitute x and y in terms of t into the integrand and simplify to get:
\(3x y = 3t(3 - t/2) = 9t - (3/2)t^2\)
Now, we can evaluate the integral by integrating with respect to t from -1 to 4:
\(\int c (3x y) ds = ∫ from -1 to 4 (9t - (3/2)t^2) (\sqrt{(5)/2)} dt\)
\(= (\sqrt{(5)/2)} [ (9t^2/2) - (3/8)t^3 ] evaluated from -1 to 4\)
\(= (\sqrt{(5)/2)} [ (81/2) - (243/8) - (-27/8) + (3/8) ]\)
\(= \sqrt{(5)/2)} [ (189/8) ]\)
= 22.229
Therefore, the scalar line integral ∫ c (3x y) ds, where c is the line segment from (−1,3) to (4,2), is approximately equal to
22.229.
for such more question on scalar line integral
https://brainly.com/question/31392715
#SPJ11
Billy has a gift card with a $160 balance. He buys several video games that cost $50 each. After the
purchases, his gift card balance is $10. Enter an equation to help find out how many video games Billy
bought
The equation
can help find out how many games Billy bought
Answers
Answer:
10=160-50x
Step-by-step explanation:
he bought 3 games
sara scored 8 goals this season. This is two less than a third of the goals she scored last season.
Answers
Answer:
30 goals last season
Step-by-step explanation:
Goals this season = 8
Goals this season = 1/3 of goals last season - 2
Let Goals scored last season = x
Therefore,
8 = 1/3x - 2
8 = x/3 - 2
8 + 2 = x / 3
10 = x / 3
x = 10 * 3
x = 30
Sara scored 30 goals last season
(Multiple choice)
Can I please have some help with this question, I will reward most Brainly to who ever gets it correct.

Answers
Answer:
Option c
Step-by-step explanation:
y-intercept is the point where the line crosses y axis. It is also his monthly savings
(1 point) use spherical coordinates to evaluate the triple integral∭E e^−(x2 + y2 + z2)/ √x2 +y2+ z2 dV,where E is the region bounded by the spheres x2+ y2+ z2=1 and x2+ y2+ z2=16. ANSWER = _____
Answers
When E is the region bounded by the spheres then the answer to the triple integral is -π/2 (e⁻¹ - e⁻¹⁶)
To evaluate the triple integral using spherical coordinates, we need to express the volume element dV in terms of spherical coordinates and determine the limits of integration.
In spherical coordinates, the volume element dV is given by:
dV = ρ² sin(φ) dρ dφ dθ
where ρ represents the radial distance, φ represents the polar angle (measured from the positive z-axis), and θ represents the azimuthal angle (measured from the positive x-axis in the xy-plane).
The given region E is bounded by the spheres x² + y² + z² = 1 and x² + y² + z² = 16. These can be expressed in terms of ρ as:
1 ≤ ρ ≤ 4
To determine the limits of integration for φ and θ, we consider the spherical symmetry of the problem. Since the region is bounded by spheres, the limits for both φ and θ will be the full range of [0, π] and [0, 2π], respectively.
Now, let's evaluate the triple integral:
∭E e(-x² - y² - z²) / √(x² + y² + z²) dV
= ∫₀²π ∫₀ᴨ ∫₁⁴ e(-ρ²) / ρ ρ² sin(φ) dρ dφ dθ
= ∫₀²π ∫₀ᴨ ∫₁⁴ e(-ρ²) ρ sin(φ) dρ dφ dθ
Since the integrand does not depend on θ, we can simplify the triple integral:
= ∫₀²π ∫₀ᴨ [-e(-ρ²) cos(φ)]₁⁴ sin(φ) dφ dθ
= ∫₀²π [-e(-ρ²) cos(φ) sin(φ)]₁⁴ dθ
= ∫₀²π [-e(-ρ²) / 4] dθ
= -e(-ρ²) / 4 [θ]₀²π
= -e(-ρ²) / 4 (2π - 0)
= -π/2 (e(-ρ²))
Now, we need to evaluate this expression within the limits of ρ:
= -π/2 ∫₁⁴ e(-ρ²) dρ
To solve this integral, we can use the substitution u = -ρ², which gives du = -2ρ dρ. The limits of integration transform accordingly:
u = -1, u = -16
∫ eu du = eu [u]_{-1}{-16} = e⁻¹ - e⁻¹⁶
Please note that the above expression represents the numerical value of the triple integral.
You can learn more about triple integral at: brainly.com/question/2289273
#SPJ11
Which of the following would NOT cast doubt of the usefulness of sample? data? Choose the correct answer below.
a. Order of questions in a survey
b. Missing data
c. Nonresponse
d. An effective sampling method
Answers
An effective sampling method is not cast doubt on the usefulness of the sample
The effective sample is a calculation of the approximate sample size needed to obtain the same degree of accuracy as a simple random sample. It is denoted mathematically by the formula n/D, where n represents the sample size and D denotes the design effect. It is employed as a means of condensing the volume of data.
Effective sample size calculations are mostly used for qualitative analyses of the sample size. The sample size calculates the total number of measurements or observations made during an experiment or survey. The effective sample size—rather than the actual sample size—is utilized in this assessment since it is thought that a sample size of 30 is necessary for an analysis to be valid.
To know more samples at:
https://brainly.com/question/27829028
#SPJ4
What’s 5 times 7/9 pls help

Answers
Answer:
the answer is 35/9 which can be written as
3 (8/9)
If a 48 gal hot water tank holds 400 lb of water, what weight of water will a 65 gal tank hold?
A 65 gal tank hold will holdlb of water.
(Round to the nearest whole number as needed.)
Answers
Using the cross-multiplication method we know that the 65 gallons of water tank can hold 542 lb of water.
What is cross-multiplication?For the following, we employ the cross-multiplication technique:
Cross multiplication is a technique used to compare fractions and ratios. We can determine their equality, greatness, or lesserness using this.
Finding the value of variables in an expression is another usage for it.
So, we know that:
48 gallons water tank = 400 lb water
Then,
65 gallons water tank = ?
Use cross-multiply as follows;
48/400 = 65/x
48x = 65*400
48x = 26,000
x = 26,000/48
x = 541.66
Rounding off: 542 lb water
Therefore, using the cross-multiplication method we know that the 65 gallons of water tank can hold 542 lb of water.
Know more about cross-multiplication here:
https://brainly.com/question/28839233
#SPJ1
Let u = 2] 3k, v= 6i + 4j - 4k, w=3i 2k. Which vectors if any are (a) perpendicular? (b) Parallel?
Answers
The vector v is perpendicular to the line with x-coordinate 2, y coordinate 3 and z coordinate 6. The vector w is parallel to this line and has components 3i + 2j + 1k.
A vector is (a) perpendicular if its direction of movement makes it opposite to the direction of movement of the particle, or (b) parallel if it lies in the same plane as a given particle.
We can see that the vector v and w are perpendicular as they start at a point at which we know that k is positive and end at a point which happens to be on the x-axis. We also know perpendicirorities happen when any two vectors intersect in their first and last natures.
In order to find the perpendicular vectors we have to use the cross product. The y-axis is the set of all y values (0 and 2). The z-axis is an axis that connects the origin and origin + 1 on every j.
The x-axis is also considered a plane as each point is marked by an angle/orientation.
For more similar questions on perpendicular:
https://brainly.com/question/26356616
#SPJ11
Find the difference. Express the answer in scientific notation. (4.56 times 10 Superscript negative 13 Baseline) minus (1.17 times 10 Superscript negative 13) 3.39 times 10 Superscript negative 26 5.73 times 10 Superscript negative 26 3.39 times 10 Superscript negative 13 5.73 times 10 Superscript negative 13
Answers
The difference is approximately \(\(3.39 \times 10^{-26}\)\).
To find the difference between these numbers, let's perform the calculation step by step:
\(\((4.56 \times 10^{-13}) - (1.17 \times 10^{-13})\)\\\(= (4.56 - 1.17) \times 10^{-13}\)\\\(= 3.39 \times 10^{-13}\)\)
Now let's subtract this result from the next number:
\(\(3.39 \times 10^{-26} - 3.39 \times 10^{-13}\)\\\(= (3.39 \times 10^{-26}) - (3.39 \times 10^{-13})\)\\\(= (3.39 \times 10^{-26}) - (3.39 \times 10^{-13})\)\\\(= (3.39 \times 10^{-26}) \times (1 - 10^{13})\)\\\(= (3.39 \times 10^{-26}) \times (-9999999999999)\)\\\(= -3.39 \times 10^{-26} \times 9999999999999\)\)
\(\(= -3.38999999999999999999999999999999999999999999999 \times 10^{-26}\) \\(approximately \(-3.39 \times 10^{-26}\) in scientific notation)\)
Finally, let's subtract the last number:
\(\(-3.39 \times 10^{-26} - 5.73 \times 10^{-13}\)\\\(= (-3.39 \times 10^{-26}) - (5.73 \times 10^{-13})\)\\\(= (-3.39 \times 10^{-26}) \times (1 - 10^{13})\)\\\(= (-3.39 \times 10^{-26}) \times (-9999999999999)\)\\\(= 3.39 \times 10^{-26} \times 9999999999999\)\)
\(\(= 3.38999999999999999999999999999999999999999999999 \times 10^{-26}\) \\(approximately \(3.39 \times 10^{-26}\) in scientific notation)\)
Learn more about difference
https://brainly.com/question/30241588
#SPJ11
The difference is \(\(-2.34\)\\\) when expressed in scientific notation as \(\(-2.34 \times 10^{0}\).\)
The difference between the given values, expressed in scientific notation, can be calculated as follows:
\(\[(4.56 \times 10^{-13}) - (1.17 \times 10^{-13}) + (3.39 \times 10^{-26}) - (5.73 \times 10^{-26}) + (3.39 \times 10^{-13}) - (5.73 \times 10^{-13})\]\)
Simplifying the calculation:
\(\[= 3.39 \times 10^{-26} - 5.73 \times 10^{-26} + 3.39 \times 10^{-13} - 5.73 \times 10^{-13}\]\)
To find the difference of the coefficients:
\(\[3.39 - 5.73 = -2.34\]\)
To find the difference of the exponents:
\(\[10^{-26} - 10^{-26} = 0\]\)
Learn more about difference
brainly.com/question/30241588
#SPJ11
Evaluate the expression when q = 8
11q
Answers
Answer: 88
Step-by-step explanation:
Plug 8 in for q
11 x (8) = 88
11 x 8 = 88
Answer: 88
What is the 2-digit subtraction with regrouping worksheets?
Answers
Answer:
To subtract two-digit numbers, use column form. Subtract one column at a time, starting with the Ones column. We regroup, or borrow. To regroup, take 1 from the Tens column and add 10 to the Ones column.
Step-by-step explanation: