Ava draws a rectangle on grid paper showing 4 for length and 6 for width. Ava says the area of the rectangle is 10 square units. Which explains Avas error and correctly solves for the area of the rectangle
Answers
Answer:
Ava's error is that she has calculated area using wrong formula. The correct formula for Area of Rectangle is Length x Width.
Using Ava's values of Length = 4 and Width = 6, the area of the rectangle is:
Area = Length x Width = 4 x 6 = 24 square units
Therefore, the correct area of the rectangle is 24 square units, not 10 as Ava calculated.
Related Questions
A constant force F = -31+43 - 2k is applied to an object that is moving along a straight line from the point (4,1,-5) to the point (-4,4,4). Find the work done if the distance is measured in meters and the force in newtons. Include units in your answer. (Note, units are case sensative. Clicking on the link units will give a list of units.)
Answers
I don't know if you meant
\(\vec F = (-3\,\vec\imath + 4\,\vec\jmath - 2\,\vec k)\,\mathrm N\)
or
\(\vec F = (-31\,\vec\imath + 43\,\vec\jmath - 2\,\vec k)\,\mathrm N\)
I'll assume the first force is correct.
The object in question undergoes a total displacement of
\(\vec d = (-4\,\vec\imath + 4\,\vec\jmath+4\,\vec k)\,\mathrm m - (4\,\vec\imath + \vec\jmath - 5\,\vec k)\,\mathrm m = (-8\,\vec\imath + 3\,\vec\jmath + 9\,\vec k)\,\mathrm m\)
Then the work W done by \(\vec F\) along this displacement is
\(W = \vec F \cdot \vec d = ((-3)\times(-8)+4\times3+(-2)\times9)=18\,\mathrm{Nm} = \boxed{18\,\mathrm J}\)
Another approach using calculus (it's overkill since \(\vec F\) is constant, but it doesn't hurt to check our answer): parameterize the line segment by
\(\vec r(t) = (1 - t)(4\,\vec\imath+\vec\jmath-5\,\vec k)\,\mathrm m + t(-4\,\vec\imath+4\,\vec\jmath + 4\,\vec k)\,\mathrm m \\\\ \vec r(t) = \left((4-8t)\,\vec\imath+(1+3t)\,\vec\jmath+(-5+9t)\,\vec k\right)\,\mathrm m\)
with 0 ≤ t ≤ 1.
Then the work W done by \(\vec F\) along the given path is equal to the line integral,
\(\displaystyle W = \int_0^1 \vec F(\vec r(t)) \cdot \frac{\mathrm d\vec r(t)}{\mathrm dt}\,\mathrm dt \\\\ W = \int_0^1 \left((-3\,\vec\imath+4\,\vec\jmath-2\,\vec k)\,\mathrm N\right) \cdot \left((-8\,\vec\imath+3\,\vec\jmath+9\,\vec k)\,\mathrm m\right) \,\mathrm dt \\\\ W = \int_0^1((-3)\times(-8)+4\times3+(-2)\times9)\,\mathrm{Nm}\,\mathrm dt \\\\ W = 18\,\mathrm{Nm} \int_0^1\mathrm dt \\\\ W = 18\,\mathrm{Nm} = \boxed{18\,\mathrm J}\)
Fill in the blanks below.
Find the slope of the line passing through the points (8,5) and (8,-4).
Answers
Step-by-step explanation:
please mark me as brainlest

P is the weighted average of point C and D. Find the coordinates of P under the given conditions
Answers
Coordinates of P = \([(3 * xB + xC + 4 * xD) / 8, (3 * yB + yC + 4 * yD) / 8]\)
Coordinates of P = \([(4 * xC + 2 * xD + 3 * xE) / 9, (4 * yC + 2 * yD + 3 * yE) / 9]\)
Leave the directions of point B alone (x1, y1) and the directions of point D be (x2, y2). Then, at that point, the directions of P are:
\([(3x1 + x2)/4, (3y1 + y2)/4]\)
Let the directions of point A be (x1, y1) and the directions of point E be (x2, y2). Then, at that point, the directions of P are:
\([(x1 + 2x2)/3, (y1 + 2y2)/3]\)
Leave the directions of point B alone (x1, y1), the directions of point C be (x2, y2), and the directions of point D be (x3, y3). Then, at that point, the directions of P are:
\([(3x1 + x2 + 4x3)/8, (3y1 + y2 + 4y3)/8]\)
Leave the directions of point C alone (x1, y1), the directions of point D be (x2, y2), and the directions of point E be (x3, y3). Then, at that point, the directions of P are:
\([(4x1 + 2x2 + 3x3)/9, (4y1 + 2y2 + 3y3)/9]\)
These recipes can be inferred utilizing the weighted typical equation:
\([(w1x1 + w2x2 + ... + wnxn)/(w1 + w2 + ... + wn), (w1y1 + w2y2 + ... + wnyn)/(w1 + w2 + ... + wn)]\)
where wi is the heaviness of point I and (xi, yi) are the directions of point I. Subbing the given loads and facilitates into the equations for every issue gives the directions of P for each situation.
To learn more about Coordinates, refer:
https://brainly.com/question/30533433
#SPJ1
The complete question is:
Find the coordinates of P that represent the weighted average of each set of points based on the given conditions. 12. Point B weighs three times as much as point D. 13. Point E weighs twice as much as point A. 14. Point B has a weight of 3 , point C has a weight of 1 , and point D has a weight of 4. 15. Point C has a weight of 4 , point D has a weight of 2 , and point E has a weight of 3.
The number of three-digit numbers with distinct digits that can be formed using the digits 1, 2, 3, 5, 8, and 9 is what
. The probability that both the first digit in the last digit of the three digit number are even numbers is what
Answers
The requried, probability that both the first digit in the last digit of the three-digit number is even numbers is 20%.
To count the number of three-digit numbers with distinct digits that can be formed using the digits 1, 2, 3, 5, 8, and 9, we can use the permutation formula:
P(n, r) = n! / (n-r)!
In this case, we have n = 6 (since we have 6 digits to choose from) and r = 3 (since we want to form three-digit numbers). Using the formula, we get:
P(6, 3) = 120
We can choose the first digit in two ways (2 or 8), and we can choose the last digit in three ways (2, 8, or 6). For the middle digit, we have four digits left to choose from (1, 3, 5, or 9), since we cannot repeat digits. Therefore, the number of three-digit numbers with distinct digits that have an even first and last digit is:
2 x 4 x 3 = 24
The total number of three-digit numbers with distinct digits is 120, so the probability that a randomly chosen three-digit number with distinct digits has an even first and last digit is:
24/120 = 0.2 or 20%
Learn more about probability here:
brainly.com/question/14290572
#SPJ1
Permutation-
Jake's school will have a field trip, Jake will join their Field Trip and they will go to TAAL VOLCANO in TAGAYTAY.
Combination -
2 boxes of red pens and 2 boxes of black pens, one box contains 15 pens, which cost 15 pesos, how many ways to sell the pens
pahelp po sa math plssssss
Answers
Step-by-step explanation:
If you want to sell the pens as individual items, there are 30 pens in total (15 red and 15 black), so you could sell them in 30 different ways. For example, you could sell the first red pen for 15 pesos, the second red pen for 14 pesos, the third red pen for 13 pesos, and so on, until you sell the last black pen for 1 peso.
If you want to sell the pens in boxes, you could sell the red pens and black pens separately or together. Here are the possible combinations:
1 box of red pens (15 pens) for 15 pesos
1 box of black pens (15 pens) for 15 pesos
2 boxes of red pens (30 pens) for 30 pesos
2 boxes of black pens (30 pens) for 30 pesos
1 box of red pens and 1 box of black pens (30 pens) for 30 pesos
Therefore, there are 5 ways to sell the pens in boxes.
Tommy made $55 for mowing 5 lawns. How much money would he make for mowing 8 lawns?
Answers
The kitchen is 20 feet long and 10 feet wide. How many square feet of flooring does Bob need to buy?
Answers
20 x 10 = 200
So the area is 200
Explain how you know that (3, 5) is not a solution to the given inequality by looking at the graph.
Answers
The reason that the point (3, 5) is not a solution to the given inequality is given below.
We are given that;
y > 2x + 1
Now,
The inequality y > 2x + 1 can be graphed by first graphing the boundary line y = 2x + 1. Since the inequality is strict (y >), we draw a dashed line to indicate that points on the line are not solutions to the inequality. Then, we shade the region above the line to indicate all points that satisfy the inequality.
If we have (3,5) as a point, we can see that it lies on the boundary line
y = 2x + 1.
Since the inequality is strict (y >), points on this line are not solutions to the inequality
Therefore, by the inequality the answer will be given above.
Learn more about inequality ;
brainly.com/question/14164153
#SPJ1
A park has grass and sand. Find the area of the part with grass.
(Sides meet at right angles.)

Answers
Answer:
area of park with grass is 19cm^2
Step-by-step explanation:
area of park
= 5 ✖ 5
= 25cm^2
area of part with sand
= 2 ✖ 3
= 6cm^2
area of park with grass
= 25 - 6
= 19cm^2
What is −183 ? Group of answer choices
a No answer choice is correct
b -5,832
c 5,832
Answers
Answer:
it is b because I did the problem yes it is b
Help please?..........

Answers
Answer:
The answer is b.
You invested $4000 between two accounts paying 3% and 4% annual interest. If the total interest earned for the year was $130, how much was invested at each rate?
Answers
You invested $3000 at 3% annual interest rate, and the remaining amount of $4000 - $3000 = $1000 was invested at 4% annual interest rate.
Let's assume you invested an amount, x, at 3% annual interest rate. This means the amount invested at 4% annual interest rate would be $4000 - x.
To calculate the interest earned from the investment at 3%, we multiply x by 3% (0.03). Similarly, the interest earned from the investment at 4% is calculated by multiplying ($4000 - x) by 4% (0.04).
According to the given information, the total interest earned from both investments is $130. So we can set up the equation:
0.03x + 0.04($4000 - x) = $130
Simplifying the equation:
0.03x + 0.04($4000 - x) = $130
0.03x + $160 - 0.04x = $130
-0.01x = $130 - $160
-0.01x = -$30
x = -$30 / -0.01
x = $3000
Therefore, you invested $3000 at 3% annual interest rate, and the remaining amount of $4000 - $3000 = $1000 was invested at 4% annual interest rate.
for such more question on interest rate
https://brainly.com/question/29451175
#SPJ8
I NEED HELP PLEASE, THANKS! :)
The senior class is having a fundraiser to help pay for the senior trip. Selling a box of chocolates yields a profit of $2.45, while selling a box of cookies yields a profit of $2.70. The demand for cookies is at least twice that of chocolates, but the amount of cookies produced must be no more than 550 boxes plus 3 times the number of chocolates produced. Assuming that the senior class can sell every box that they order, how many boxes of each should they order to maximize profit if they cannot order more than 1950 boxes combined? (Show work)
Answers
Answer:
1600 boxes of cookies; 350 boxes of chocolates
Step-by-step explanation:
Let x and y represent the numbers of boxes of cookies and chocolates to order, respectively. The constraints seem to be ...
x ≥ 2yx ≤ 550 +3yx + y ≤ 1950The objective function we want to maximize is ...
p = 2.70x +2.45y
_____
Looking at the problem, we see that cookies yield the most profit, so we'd like to maximize the boxes of cookies sold within the allowed limits.
There are two limits on x:
x ≤ 550 +3y
x ≤ 1950 -y
x will be as large as it can be if it bumps up against these limits simultaneously:
550 +3y = 1950 -y
4y = 1400 . . . . . . . . . add y-550
y = 350
x = 1950 -350 = 1600
Profit will be maximized for an order of 1600 boxes of cookies and 350 boxes of chocolates.
_____
The "feasible region" of the solution is where the three constraint inequalities overlap. It is a quadrilateral with vertices at (0, 0), (550, 0), (1300, 650), and (1600, 350). We can reject the ones with x or y being 0. Per our analysis above, the solution of interest is (x, y) = (1600, 350), since it maximizes x.
The usual protocol for solving a linear programming model like this is to evaluate the objective function at each of the vertices. With a little thought about the problem, we have saved some evaluation effort.

helpppppppppppppppppppppppppp

Answers
Simplify 5^2 · 5^9 1. 5^11 2. 5^18 3. 25^11 4. 25^18
Answers
Answer:
Answer choice 1
Step-by-step explanation:
\(5^2\cdot 5^9= \\\\5^{2+9}= \\\\5^{11}\)
Therefore, the correct answer choice is choice 1. Hope this helps!
How many times can a paper be folded to reach the moon
Answers
Answer:
42 folds. This fact is mentioned in search results [1], [2], [3], [4], [5], and [7]. It is also noted in search result [6] that the answer may be 45 folds instead of 42, but this is in the context of a discussion on exponential growth and is not a commonly accepted answer to the question. Finally, search result [10] poses a similar question but provides the same answer of 42 folds to reach the moon.
Step-by-step explanation:
A bag of baseballs costs $12. There are 15 balls in the bag. Another bag of baseballs costs $15 for 20 balls. Which bag prices individual baseballs lower?
Answers
Answer:
Step-by-step explanation:
For the first bag:
Price of the bag = $12
Number of balls in the bag = 15
Price per ball = Price of the bag / Number of balls
Price per ball for the first bag = $12 / 15 = $0.8
For the second bag:
Price of the bag = $15
Number of balls in the bag = 20
Price per ball = Price of the bag / Number of balls
Price per ball for the second bag = $15 / 20 = $0.75
Comparing the prices per ball, we find that the second bag has a lower price per baseball. Therefore, the second bag offers a better price for individual baseballs compared to the first bag.
Answer:
Step-by-step explanation:
To find out which bag prices individual baseballs lower, we can divide the total cost of each bag by the number of balls in the bag. This will give us the price per ball for each bag.
For the first bag, the price per ball is $12 / 15 = $0.80.
For the second bag, the price per ball is $15 / 20 = $0.75.
Therefore, the second bag prices individual baseballs lower.
Find the Area of the figure below, composed of a rectangle with a semicircle
removed from it. Round to the nearest tenths place.

Answers
Answer:
Area of the figure = 25.7 units²
Step-by-step explanation:
Area of the given figure = Area of rectangle ABCD - Area of the semicircle with diameter CD
Area of the rectangle ABCD = Length × Width
= BC × AB
= 8 × 4
= 32 units²
Area of the semicircle = \(\frac{1}{2}\pi r^{2}\)
Here, r = radius of the semicircle
r = \(\frac{\text{Diameter}}{2}\)
r = \(\frac{1}{2}(CD)\)
r = \(\frac{4}{2}\)
r = 2 units
Therefore, area of the semicircle = \(\frac{1}{2}\pi (2)^2\)
= 2π
= 6.28 units²
Area of the given figure = 32 - 6.28
= 25.72 units²
≈ 25.7 units²

11. What is the unknown number in
Sequence 2 in the chart?
Sequence Number
Sequence 1
Sequence 2
A 126
B 127
c 147
D 154
2 3 5
7
14 21 35 49
?
1
7
21 42 63 105
Answers
The missing number in Sequence 2 is 780.
To find the missing number in Sequence 2, let's analyze the pattern:
In Sequence 1, the numbers increase by 1 each time: 126, 127, 128, 129, ...
In Sequence 2, the numbers seem to follow a pattern where each number is obtained by multiplying the corresponding number in Sequence 1 by a certain factor:
2 x 126 = 252
3 x 127 = 381
4 x 128 = 512
5 x 129 = 645
...
Looking at this pattern, we can see that the missing number in Sequence 2 should be:
6 x 130 = 780
For similar question on Sequence.
https://brainly.com/question/7882626
#SPJ8
John left his house in Irvine at 8:35 a.m. to drive to a meeting in Los Angeles, 45 miles away. He arrived at the meeting at 9:50 a.m.. At 5:30 p.m. he left the meeting and drove home. He arrived home at 7:18 p.m.ⓐ What was his average speed on the drive from Irvine to Los Angeles? ⓑ What was his average speed on the drive from Los Angeles to Irvine? ⓒ What was the total time he spent driving to and from this meeting
Answers
A. The average speed was 36 mph.
B. The average speed on the drive from LA to Irvine was 25mph.
C. The total time he spent driving to and from the meeting was about 3 hours and 5 minutes.
find the length of wire required to fence a circular Park of diameter 280 M with three rounds
Answers
Answer:
840
Step-by-step explanation:
1st u have to multiple 280 by 3
C = π x 280
=879.65m
3 rounds = 3 x 879.65m
=2638.95 m
Hence the length of wire required is 2638.95 m
What completes this expression to make it a perfect square trinomial?
x2 + 4x + ___
Answers
x^2 + 4x + 4 is a perfect square trinomial that can be factored as (x + 2)^2.
Explanation:
To complete the expression x^2 + 4x + __ as a perfect square trinomial, we can take half of the coefficient of x (which is 4), square it, and then add it to the expression.
Half of 4 is 2, and 2 squared is 4, so we can add 4 to the expression to get:
x^2 + 4x + 4
This expression is a perfect square trinomial and can be factored as:
(x + 2)^2
Assume that the playbook contains 10 passing plays and 12 running plays. The coach randomly selects 9 plays from the playbook. What is the probability that the coach selects at least 3 passing plays and at least 3 running plays?
Answers
Answer:
6
Step-by-step explanation:
Vector addition obeys rules that are different from those for the addition of two scalar quantities.
a. True
b. False
Answers
Answer:
false
Step-by-step explanation:
false
Find the average rate of change of g(x) =– 1x - 5 between the points (-4,-1) and (1,-6)
Answers
Answer:
m= -1
Step-by-step explanation:
Choose the word or phrase that correctly completes the sentence.
Andrew wrapped his
sisters
sisteres'
sisters'
sister's
I don't know this yet.
present.
Answers
Answer:
answer is sister's.
Step-by-step explanation:
sisters mean more than one sister.
sisterses isn't a word.
sisters' also isn't a word.
sister's is a word and it means that she has possession of something or that she owns something.
Over what interval does f(x) = 2x
increase faster than g (X) =
¿x?
A. 1
B. x > 3
C.20
D. 1 > x > 21
Answers
If over what interval does f(x) = 2x increase faster than g (X): A. 1
What is over the interval?To determine over what interval f(x) = 2x increases faster than g(x) = x^2, we need to compare their derivatives. The derivative of f(x) is 2, which is a constant, and the derivative of g(x) is 2x. Therefore, f(x) increases at a constant rate of 2, while g(x) increases at a rate that depends on x.
To find the interval where f(x) increases faster than g(x), we need to find where the derivative of g(x) is less than 2. Setting 2x < 2 and solving for x, we get x < 1.
So, over the interval x < 1, f(x) = 2x increases faster than g(x) = x^2.
Therefore, the answer is A. 1.
Learn more about interval here:https://brainly.com/question/29130032
#SPJ1
What is the result when the number 84 is decreased by 50%?
Answers
Answer:
42
Step-by-step explanation:
Answer:
42
84 ×50%by100 =42. 84-42= 42. hope helpful answerPlease help me out!

Answers
Answer:
it is the there'd one
if this is wrong sorry
Please answer 1 and 2
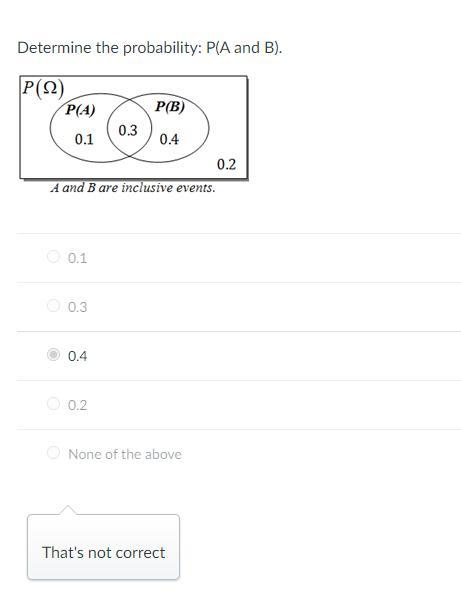

Answers
The probability of P(A and B) based on the information given will be E. None of the above.
How to calculate the probability?It should be noted that probability is used to illustrate the likelihood that something will occur.
In this case, P(A) = 0.1 and P(B) = 0.4. Therefore, the probability of P(A and B) will be
= P(A) + P(B)
= 0.1 + 0.4
= 0.5
Sine the value of 0.5 isn't given, the correct option is E.
Learn more about probability on:
brainly.com/question/24756209
#SPJ1