Average Rate of change from Table Nov 18, 9:58:13 AM Given the function defined in the table below, find the average rate of change simplest form, of the function over the interval 4 < < 12. 4 25 20 12 16 Submit Answer LIISWO

Answers
The average rate of change of the given function between x = 4 and x = 12 is computed as follows:
\(\frac{f(12)-f(4)}{12-4}\)Replacing with f(12) = 15 and f(4) = 25,
\(\frac{15-25}{12-4}=\frac{-10}{8}=-\frac{5}{4}\)The average rate of change is -5/4
Related Questions
PLEASE HELP I WILL MARK BRAINLIEST

Answers
Answer:
1:5
Step-by-step explanation:
Total flowers: 4+16 = 20
Ratio = gold:total = 4:20
This simplifies to 1:5
Answer:
1:5
Step-by-step explanation:
4 gold flowers out of 20 total gold and purple
makes it 4:20
reduced 4:20 is 1:5
Scott is 72 inches tall and Chris is 185 centimeters tall. Who is taller? Show your work. How do we convert this?

Answers
SOLUTION:
Step 1:
In this question, we are given the following:
Scott is 72 inches tall and Chris is 185 centimeters tall.
Who is taller? Show your work.
Step 2:
Scott is 72 inches tall
and
Chris is 185 centimeters tall
Converting cm to inches, we have that:
\(\begin{gathered} 1\operatorname{cm}\text{ = }0.393701\text{ inch} \\ 185\text{ cm = 185 x 0.393701 = }72.\text{ 834685 }\approx\text{ 72. 8 inch} \end{gathered}\)CONCLUSION:
Since Chris' height = 72.8 inch tall and Scott's height = 72 inch tall
Hence, Chris is taller.
please help and please show step by step :)
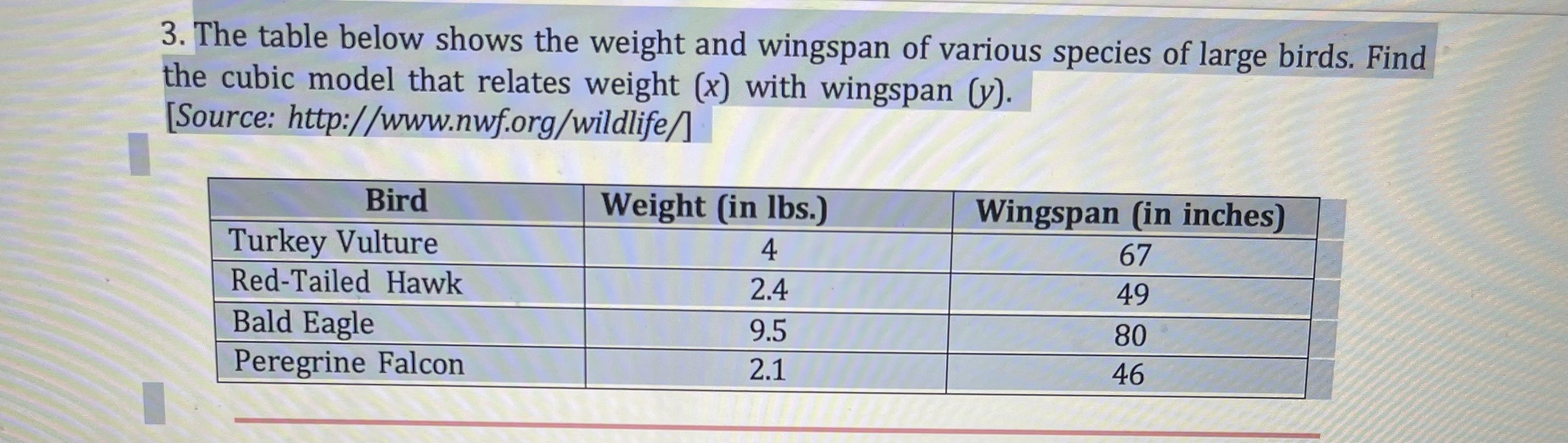
Answers
The cubic model that relates weight ( x ) to wingspan ( y ) can be found to be y = 0.4086x³ - 3.9140x² + 12.5943x + 25.8855.
How to find the cubic model ?To find the cubic model that relates the weight (x) with wingspan (y), we need to create a cubic equation of the form:
y = ax³ + bx² + cx + d
We have four pairs of data points, which we can use to create four equations to solve for the coefficients a, b, c, and d:
Turkey Vulture: (4, 67)
67 = a(4³) + b(4²) + c(4) + d
Red-Tailed Hawk: (2.4, 49)
49 = a(2.4³) + b(2.4²) + c(2.4) + d
Bald Eagle: (9.5, 80)
80 = a(9.5³) + b(9.5²) + c(9.5) + d
Peregrine Falcon: (2.1, 46)
46 = a(2.1³) + b(2.1²) + c(2.1) + d
When we solve for a, b, c, and d using a numerical method in a cubic model calculator, we get:
a = 0.4086
b = -3.9140
c = 12.5943
d = 25.8855
So, the cubic model relating weight (x) with wingspan (y) is:
y = 0.4086x³ - 3.9140x² + 12.5943x + 25.8855
Find out more cubic models at https://brainly.com/question/28990074
#SPJ1
Shown is a circle, centre C, with diameter AE.
PQ is a tangent to the circle, meeting it at E.
A EDQ is isosceles with ED= EQ.
Given that / EAD = 50°, calculate the size
of Z DQE.

Answers
Answer:
∠DQE = 65°
Step-by-step explanation:
You want to know the measure of Angle DQE in the given figure.
Right triangleTriangle ADE is inscribed in a semicircle, so is a right triangle. That makes acute angle AED complementary to acute angle EAD. The latter is given as 50°, so ...
∠AED = 90° -50° = 40°
TangentWe know that tangent line QE is perpendicular to diameter AE, so angle AEQ is a right angle. Segment ED divides that right angle into complementary angles, so ...
∠DEA +∠DEQ = 90°
∠DEQ = 90° -∠DEA = 90° -40° = 50°
Isosceles triangleA.pex angle DEQ in isosceles triangle DEQ being 50° means that the base angles QDE and DQE will have measure ...
∠DQE = (180° -50°)/2 = 130°/2
∠DQE = 65°
__
Alternate solution
Let angles DQE and QDE be represented by x. Then the sum of angles in quadrilateral ADQE is 360°:
50° +(90+x)° +x° +90° = 360°
230° +2x = 360° . . . . . . simplify
x = 130°/2 = 65° . . . . . solve for x
What is the area of this figure

Answers
Answer:
Step-by-step explanation:
3(3) = 9
15-3=12
12(9)= 108
7(9)=63
ans : 183
look at the picture please also be quick bec
Answers
5x +6 (x-2) -8 (x-3) please help
Answers
Answer:
3x + 12
Step-by-step explanation:
5x + 6(x - 2) - 8(x - 3) ← distribute parenthesis
= 5x + 6x - 12 - 8x + 24 ← collect like terms
==(5x + 6x - 8x) + (- 12 + 24)
= 3x + 12
A soda factory makes 9 flavours of soda. Each case of soda has 7 cans of each flavour. How many cans of soda does the factory put in 7 cases?
Answers
The factory will put 441 cans of soda in 7 cases.
If each case of soda contains 7 cans of each flavor, and the factory is putting 7 cases, we can calculate the total number of cans by multiplying the number of flavors, cans per flavor, and cases.
Given:
Number of flavors = 9
Cans per flavor = 7
Number of cases = 7
To calculate the total number of cans, we can multiply these values:
Total cans = Number of flavors × Cans per flavor × Number of cases
Total cans = 9 × 7 × 7
Total cans = 441
To arrive at this answer, we multiplied the number of flavors (9) by the number of cans per flavor (7) to get the number of cans per case (63). Then, we multiplied the cans per case (63) by the number of cases (7) to obtain the total number of cans (441).
Hence, the factory will put 441 cans of soda in 7 cases.
For more such questions on soda
https://brainly.com/question/29111121
#SPJ8
You are having a pizza-making party. You will need 6 ounces of dough and 4 ounces of sauce for each person at the party (including yourself, the host). Once you have a total count of guests, you buy exactly the needed amount of all the ingredients. The dough and sauce that you buy weigh 130 ounces all together.
How many ounces of dough did you buy?
Answers
Answer:
how many people are there, does it say?
Step-by-step explanation:
Answer: 78 ounces of dough
Step-by-step explanation:
You bought 78 ounces of dough and 52 ounces of sauce
Find the equation for a polynomial f(x) that satisfies
▪ Degree 5
▪ Root of multiplicity 2 at x =
= -4
▪ Root of multiplicity 1 at x = -1
■ Root of multiplicity 2 at x = -2
▪y-intercept of (0, 128)
Answers
The polynomial function that has the degree of 5 is written as f(x) = 2x⁵ + 26x⁴ + 128x³ + 296x² + 320x + 128
Polynomial function:
The equations formed with variables, exponents and coefficients are known as the polynomial equations. And it can have different exponents, where the higher one is called the degree of the equation.
Given,
Here we have the following things,
Degree = 5
Root of multiplicity 2 is x = -4
Root of multiplicity 1 at x = -1
Root of multiplicity 2 at x = -2
y-intercept of (0, 128)
Here we need to find the polynomial equation of the function.
According to the given roots of multiplicity, we have the factors of the polynomials are,
=> (x + 4)² (x + 1) (x + 2)²
So, the general form of the polynomial function can be written as,
=> f(x) = A (x + 4)² (x + 1) (x + 2)²
In order to find the 5 degree polynomial function, we have to calculate the value of A.
To Find the value of A, we have to apply the y - intercept point on the equation then we get,
=> 128 = A (0 + 4)² (0 + 1) (0 + 2)²
=> 128 = A (4)² (1) (2)²
=> 128 = A (16 x 1 x 4)
=> 128 = 64A
=> A = 2
Now, the polynomial function is written as,
=> f(x) = 2 (x + 4)² (x + 1) (x + 2)²
When we expand this one then we get,
=> f(x) =2 [x⁵ + 13x⁴ + 64x³ + 148x² + 160x + 64]
Therefore, the resulting polynomial function is,
=> f(x) = 2x⁵ + 26x⁴ + 128x³ + 296x² + 320x + 128
To know more about Polynomial function here.
https://brainly.com/question/12914312
#SPJ1
Solve the equation. 9x−(3x+9)=2x−25 Select the correct choice below and fill in any answer boxes in your choice. A. The solution set is (Simplify your answer.) B. There is no solution.
Answers
Answer: Choice A, x=-4
Step-by-step explanation:
1. 9x−(3x+9)=2x−25
2. 9x-3x-9=2x-25
3. 6x-9=2x-25
4. 6x-9=2x-25
-2x -2x
5. 4x-9=-25
+9 +9
6. 4x=-16 (divide both sides by 4)
7. x=-4
Please help me out :c I am also marking brainliest

Answers
Answer :
Step by step explanation
The image of (6,9) under a dialation is (4,6). The scale factor is. -2, 2/3, or -2,3
Answers
Answer:
The scale factor is 2/3
Step-by-step explanation:
The image of (6,9) under a dilation is (4,6).
As you might see, there is a ratio between the image after dilating and before dilating, which is 4/6 = 6/9 = 2/3.
=> The scale factor is 2/3.
I NEED HELP 30 POINT!!

Answers
Answer:
35
Step-by-step explanation:
You can easily graph this in desmos for a visual understanding.
The slope of a line is received by (y2-y1)/(x2-x1). Assuming that Days is X and the cost is Y, we get (160-90)/(4-2), and it makes 70/2, which equates out to 35. Because the prices become more and more expensive, the slope is positive 35.
Fill in the table using this function rule.
y=-3x-3
X
-2
-1
0
1
y
0
0
0
X
Ś

Answers
Answer:
X | y
---------------
-2 | 3
-1 | 0
0 | -3
1 | -6
Layla, Sofia, and Evelyn share a box of 754 pieces of colored paper. The ratio of Layla's pieces of colored paper to Sola's pieces of colored paper is 4: 3. The ratio of Sofia's pieces of coloredpaper to Evelyn's pieces of colored paper is 9 : 5.a How many piece of colored paper does Layla have?b How many more pieces of colored paper does Layla have than Evelyn?
Answers
Layla: Sofia
4:3
Multiply by 3
4*3 : 3*3
12 : 9
We know Sofia to Evelyn is 9:5
We can write this as one ratio
Layla : Sofia : Evelyn : total
12 : 9 5 : 12+9+5 = 26
We have 754 pieces of paper so divide by 26
754/26 =29
Multiply each number by 29
Layla : Sofia : Evelyn : total
12*29 : 9*29 : 5*29 : 26*29
348 261 145 754
Layla has 348 pieces
Layla - Evelyn
348-145
203
Layla has 203 more pieces
PLEASE HELP STAT AND FILL IN THE BLANKS
Alyson has 30 beads to make bracelets. Each bracelet has 7 beads. How many more beads does Alyson need so that all the beads she has are used? Complete the explanation. If Alyson made ? bracelets, she would use ? beads, leaving ? leftover. So she needs to make one more bracelet, which means she needs ? more beads
Answers
Answer: So if Allison has 30 beads and each bracelet has 7. She would make 4 bracelets with 2 left over. The sentence would be If Alyson made 4 bracelets she would use 28 beads, leaving 2 left over.
Step-by-step explanation: 30/7 is 4 with a remainder of 2
if f is a function from R to R such that f(x)f(y)=f(x+y)+f(z-y) and f(1)=3, then what is the value of f(7)?
Answers
I suppose you mean
\(f(x) f(y) = f(x + y) + f(x - y)\)
Let \(x=1\) and \(y=0\). Then
\(f(1) f(0) = f(1 + 0) + f(1 - 0) \implies f(1) f(0) = 2 f(1) \implies f(0) = 2\)
Now if we fix \(y=1\), the functional equation reduces to the recurrence relation
\(f(x) f(1) = f(x + 1) + f(x - 1) \implies f(x+1) - 3f(x) + f(x-1) = 0\)
and we can find \(f(7)\) with a few rounds of substitution.
\(x=1 \implies f(2) - 3f(1) + f(0) = 0 \implies f(2) = 3f(1) - f(0) = 7\)
\(x=2 \implies f(3) - 3f(2) + f(1) = 0 \implies f(3) = 3f(2) - f(1) = 18\)
\(x=3 \implies f(4) - 3f(3) + f(2) = 0 \implies f(4) = 3f(3) - f(2) = 47\)
\(x=4 \implies f(5) - 3f(4) + f(3) = 0 \implies f(5) = 3f(4) - f(3) = 123\)
\(x=5 \implies f(6) - 3f(5) + f(4) = 0 \implies f(6) = 3f(5) - f(4) = 322\)
\(x=6 \implies f(7) - 3f(6) + f(5) = 0 \implies f(7) = 3f(6) - f(5) = \boxed{843}\)
Maggie bought a pound of bananas and 4 boxes of cereal for $10.11. If each box of cereal cost $2.40, what was the price of a pound of bananas?
$0.51
$0.61
$1.51
$2.91
Answers
Answer:
$0.51
Step-by-step explanation:
If each box of cereal is $2.40, $2.40 multiplied by 4 is $9.60. $10.11 is the total, so $10.11 - $9.60 = $0.51.
Answer:
$0.51
Step-by-step explanation:
the person above me was correct
Lucy invested 4,000 into a mutual fund that grows at a rate of 3% per year in how many years will she have more than 5,000 in funds
Answers
Answer:
it will take at least 9 years for Lucy's investment to grow to more than $5,000. Since we can't have a fraction of a year, the answer is 10 years (rounded up from 9.09).
Step-by-step explanation:
We can solve this problem by using the formula for compound interest:
A = P(1 + r/n)^(nt)
where:
A = the amount of money in the account after t years
P = the initial investment (principal), which is $4,000 in this case
r = the interest rate per year, which is 3% expressed as a decimal (0.03)
n = the number of times the interest is compounded per year (we'll assume it's compounded annually, so n = 1)
t = the number of years
We want to find the number of years t it will take for the account balance to exceed $5,000. So we can set up the following inequality:
A > 5000
Substituting the values we have into the compound interest formula and simplifying, we get:
4000(1 + 0.03/1)^(1t) > 5000
Simplifying further, we get:
1.03^t > 1.25
To solve for t, we can take the logarithm of both sides of the inequality:
log(1.03^t) > log(1.25)
t*log(1.03) > log(1.25)
t > log(1.25) / log(1.03)
Using a calculator, we find that:
t > 9.09
Cam anyone answer these 4 questions?

Answers
Answer:
The first one is correct
Step-by-step explanation:
ITs all good
Find x
there is another question after this

Answers
Answer:
x = 2
Step-by-step explanation:
All three angles are equal in measurements which means all sides of the triangle is also equal in measurement. To solve for x, simply equal the two expressions together and isolate variable x.
9 = 3x + 3
Isolate variable x by subtracting both sides by 3:
6 = 3x
Isolate variable x by dividing both sides by 3:
2 = x
Check your work:
9 = 3(2) + 3
9 = 6 + 3
9 = 9
Correct!
Class 4B is having a sports day .There are 36 children in the class .The children need to be in equal groups.what group size are possible?
Answers
Answer:
2,3,4,..... teams
Step-by-step explanation:
there are many
Answer:
let's divide it 2
Step-by-step explanation:
by diving in two halves we get 18
so we will divide the childrens in two equal groups
The sum of two numbers is 48 and the difference is 22 what are the numbers
Answers
Answer:
35 and 13
Step-by-step explanation:
Sum: 35 + 13 = 48
Difference: 35 - 13 = 22
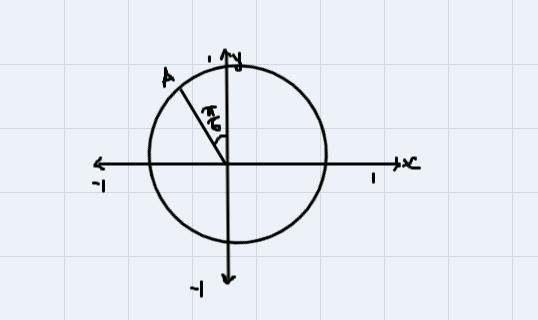
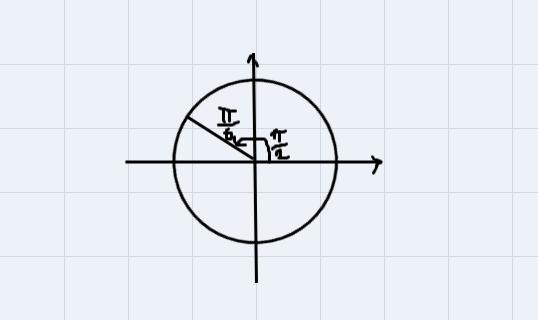
answer choices a. sin (pi/6)b. cos (pi/6)c. sin (pi/3)d. cos (pi/3)e. sin (2pi/3)f. cos (2pi/3)

Answers
The value of x - co-ordinate of point A is cos(2π/3)
Step - by - Step Explanation
Given that:
We know that if any unit circle which radius is r (r=1) and angle is θ then its coordinate will be:
x = rcos θ
y = r sinθ
In the figure given to us θ will be;
θ = π / 2 + π /6
\(\theta=\frac{\pi}{2}+\frac{\pi}{6}\)Simplify
\(\theta=\frac{3\pi+\pi}{6}\)\(=\frac{4\pi}{6}\)We can reduce the fraction above by 2.
\(=\frac{^2\cancel{4}\pi}{^3\cancel{6}}=\frac{2\pi}{3}\)But the x - coordinate is given by :
x= rcos θ
From the figure, it is a unit circle, so r = 1
We've calculated θ = 2π /3
Substitute the values into x = rcosθ
x = 1 . cos (2π /3)
Therefore, the value of x- coordinate is cos(2π/3)

Consider the function \(y=\sqrt{5x-5}+1\)
Which inequality is used to find the domain?
Answers
The inequality is used to find the domain of the given function is
5x-5 ≥ 0
What is a function?A function is a relation from a set of inputs to a set of possible outputs, where each input is related to exactly one output.
Given is function y = √(5x-5)+1, we have to find which inequality can be used to find the domain.
Since, the function is a square root function.
We know that,
The square root function is defined only for the positive values, including 0. i.e. the expression inside the square root must be greater than or equal to 0.
The expression inside the square root is (5x-5) so that must be greater than or equal to 0 which can be written as :
5x-5 ≥ 0
Hence, the inequality is used to find the domain of the given function is 5x-5 ≥ 0
Learn more about functions, click;
https://brainly.com/question/21145944
#SPJ1
Help me please and I will love you forever

Answers
Answer:
d²+4a+6 is the answer in ky opinion
f(x)=1/(x+5)-1. find the inverse operation
Answers
Answer: y=1/x+5-1
Step-by-step explanation:
A committee must be formed with 3 teachers and 3 students. If there are 10 teachers
to choose from, and 15 students, how many different ways could the committee be
made?
Answers
Answer:
54,600 ways
Step-by-step explanation:
i hope you understand this

Maya’s unpaid balance is $205.16. Her APR is 14.4% and she has $82.10 in new transactions. What is her new balance? Responses $289.72 $289.72 $290.71 $290.71 $316.80 $316.80 $533.45
Answers
Based on the information given the new balance is $289.72.
New balanceFirst step is to calculate the finance charge
\(\text{Finance charge}=205.16\times(0.144\div12 \ \text{months})\)
\(\text{Finance charge}=205.16\times0.012\)
\(\text{Finance charge}=2.46\)
Second step is to calculate the New balance
\(\text{New balance}=205.16+2.46+82.10\)
\(\text{New balance}=\bold{\$289.72}\)
In conclusion, the new balance is $289.72.
Learn more about new balance here:
brainly.com/question/25610363