Answers
Answer:
75%
Step-by-step explanation:
I had this question and got it right!
Answer:
the answer 75%
hope this helps
Related Questions
im cant figure out how to do this one ((-3)^2)^-3
Answers
Answer:
\(\dfrac{1}{729}\)
Step-by-step explanation:
\(\left(\dfrac{}{}(-3)^2\dfrac{}{}\right)^{-3}\)
First, we should evaluate inside the large parentheses:
\((-3)^2 = (-3)\cdot (-3) = 9\)
We know that a number to a positive exponent is equal to the base number multiplied by itself as many times as the exponent. For example,
\(4^3 = 4 \, \cdot\, 4\, \cdot \,4\)
↑1 ↑2 ↑3 times because the exponent is 3
Next, we can put the value 9 into where \((-3)^2\) was originally:
\((9)^{-3}\)
We know that a number to a negative power is equal to 1 divided by that number to the absolute value of that negative power. For example,
\(3^{-2} = \dfrac{1}{3^2} = \dfrac{1}{3\cdot 3} = \dfrac{1}{9}\)
Finally, we can apply this principle to the \(9^{-3}\):
\(9^{-3} = \dfrac{1}{9^3} = \boxed{\dfrac{1}{729}}\)
Find the solution to the following system by substitution.
4x + y = 40
y = 4x
OA. (0,40)
OB. (5,20)
O C. (10,40)
D. (10,0)

Answers
2-simplifica
1)x²-5x-16
x+2=
2)6an²-3b²n²
b4-4ab²+4a²=
3)4x²-4xy+y²
5y-10x
4)n+1-n³-n²
n³-n-2n²+2=
5)17x³y4z6
34x7y8z10=
6)12a²b³
60a³b5x6=
Answers
1. x² - 5x - 16 can be written as (x - 8)(x + 2).
2. 6an² - 3b²n² = n²(6a - 3b²).
3. This expression represents a perfect square trinomial, which can be factored as (2x - y)².
4. Combining like terms, we get -n³ - n² + n + 1 = -(n³ + n² - n - 1).
5. 17x³y⁴z⁶ = (x²y²z³)².
6. 12a²b³ = (2a)(6b³) = 12a6b³ = 12a⁷b³x⁶.
Let's simplify the given expressions:
Simplifying x² - 5x - 16:
To factorize this quadratic expression, we look for two numbers whose product is equal to -16 and whose sum is equal to -5. The numbers are -8 and 2.
Therefore, x² - 5x - 16 can be written as (x - 8)(x + 2).
Simplifying 6an² - 3b²n²:
To simplify this expression, we can factor out the common term n² from both terms:
6an² - 3b²n² = n²(6a - 3b²).
Simplifying 4x² - 4xy + y²:
This expression represents a perfect square trinomial, which can be factored as (2x - y)².
Simplifying n + 1 - n³ - n²:
Rearranging the terms, we have -n³ - n² + n + 1.
Combining like terms, we get -n³ - n² + n + 1 = -(n³ + n² - n - 1).
Simplifying 17x³y⁴z⁶:
To simplify this expression, we can divide each exponent by 2 to simplify it as much as possible:
17x³y⁴z⁶ = (x²y²z³)².
Simplifying 12a²b³:
To simplify this expression, we can multiply the exponents of a and b with the given expression:
12a²b³ = (2a)(6b³) = 12a6b³ = 12a⁷b³x⁶.
Learn more about expression from
https://brainly.com/question/723406
#SPJ11
a
4.2 x 34 =
42 (tenths)
x 34
Answers
Step-by-step explanation:
4.2×34=142.
or
714/5, 142 4/5
can someone please help for brainlest

Answers
Answer:
B
Step-by-step explanation:
can i have brainliest
Answer:
B
Step-by-step explanation:
You multiply 9 ×3 and get 27
Then you multiply 27×4 and get 108
And then you check your work.
Since 9×4 is 36 you multiply 36×3 and get 108.
So the new area is 3 times the old area.
A major automobile company claims that its new electric powered car has an average range of more that 300 miles. A random sample of 40 new electric cars was selected to test the claim. Assume that the population standard deviation is 12 miles. A 5% level of significance will be used for the test. A) what would be the consequences of making a type ii error in this problem? b) compute the probability of making a type ii error if the true population mean is 305 miles. C) what is the maximum probability of making a type i error in this problem?
Answers
Answer:
Step-by-step explanation:
urmom
HELP ME PLSSS, DONT GET IT WRONG PLSS

Answers
\(\tt \dfrac{-5u^2}{10u^{-7}}=-0.5u^9\)
One month before an election, a poll of 630 randomly selected voters showed 55% planning to vote for a certain candidate. A week later it became known that he had had an extramarital affair, and a new poll showed only 53% of 1010 voters supporting him. Do these results indicate a decrease in voter support for his candidacy?
Determine the test statistic. z= (Round to two decimal places as needed.)
Find the P-value.
estimate that difference, p1−p2, with a 95% confidence interval
Answers
The statistics are as follows:
- Test Statistic: The calculated test statistic is approximately 1.02.
- P-value: The P-value associated with the test statistic of 1.02 is approximately 0.154.
- Confidence Interval: The 95% confidence interval for the difference in proportions is approximately -0.0186 to 0.0786.
To solve the problem completely, let's go through each step in detail:
1. Test Statistic:
The test statistic can be calculated using the formula:
z = (p1 - p2) / √[(p_cap1 * (1 - p-cap1) / n1) + (p_cap2 * (1 - p_cap2) / n2)]
We have:
p1 = 0.55 (proportion in the first poll)
p2 = 0.53 (proportion in the second poll)
n1 = 630 (sample size of the first poll)
n2 = 1010 (sample size of the second poll)
Substituting these values into the formula, we get:
z = (0.55 - 0.53) / √[(0.55 * (1 - 0.55) / 630) + (0.53 * (1 - 0.53) / 1010)]
z = 0.02 / √[(0.55 * 0.45 / 630) + (0.53 * 0.47 / 1010)]
z ≈ 0.02 / √(0.0001386 + 0.0002493)
z ≈ 0.02 / √0.0003879
z ≈ 0.02 / 0.0197
z ≈ 1.02 (rounded to two decimal places)
Therefore, the test statistic is approximately 1.02.
2. P-value:
To find the P-value, we need to determine the probability of observing a test statistic as extreme as 1.02 or more extreme under the null hypothesis. We can consult a standard normal distribution table or use statistical software.
The P-value associated with a test statistic of 1.02 is approximately 0.154, which means there is a 15.4% chance of observing a difference in proportions as extreme as 1.02 or greater under the null hypothesis.
3. Confidence Interval:
To estimate the difference in proportions with a 95% confidence interval, we can use the formula:
(p1 - p2) ± z * √[(p_cap1 * (1 - p_cap1) / n1) + (p_cap2 * (1 - p_cap2) / n2)]
We have:
p1 = 0.55 (proportion in the first poll)
p2 = 0.53 (proportion in the second poll)
n1 = 630 (sample size of the first poll)
n2 = 1010 (sample size of the second poll)
z = 1.96 (for a 95% confidence interval)
Substituting these values into the formula, we get:
(0.55 - 0.53) ± 1.96 * √[(0.55 * (1 - 0.55) / 630) + (0.53 * (1 - 0.53) / 1010)]
0.02 ± 1.96 * √[(0.55 * 0.45 / 630) + (0.53 * 0.47 / 1010)]
0.02 ± 1.96 * √(0.0001386 + 0.0002493)
0.02 ± 1.96 * √0.0003879
0.02 ± 1.96 * 0.0197
0.02 ± 0.0386
The 95% confidence interval for the difference in proportions is approximately (0.02 - 0.0386) to (0.02 + 0.0386), which simplifies to (-0.0186 to 0.0786).
To know more about confidence intervals, refer here:
https://brainly.com/question/32546207#
#SPJ11
What is the equation of a circle with center (-3, -5) and radius 4?
O A. (x+3)2 + (y + 5)² = 16
O B. (x-3)2 + (y- 5)² = 4
O C. (x+3)2 + (y + 5)² = 4
O D. (x-3)2+(vy- 5)² = 16
Answers
The equation of a circle with center (-3, -5) and radius 4 is (x+3)^2+(y+5)^2=16.
In the given equation we have to find the equation of circle with center and radius.
The given center is (-3, -5).
Radius = 4
The standard equation of a circle with center and radius.
(x−a)^2+(y−b)^2=r^2
where a and b represent a point of center and r represent a radius.
So a=-3, b=-5 and r=4.
Putting the value in the standard equation.
(x−(-3))^2+(y−(-5))^2=(4)^2
Simplifying
(x+3)^2+(y+5)^2=16
Hence, the equation of a circle with center (-3, -5) and radius 4 is (x+3)^2+(y+5)^2=16.
To learn more about equation of a circle link is here
brainly.com/question/16569537
#SPJ1
What is the slope of the line shown below?
A.
\( \frac{1}{2} \)
B.
\( - \frac{1}{2} \)
C.
\(2\)
D.
\( - 2\)
![What is the slope of the line shown below? A. [tex] \frac{1}{2} [/tex]B.[tex] - \frac{1}{2} [/tex]C.](https://i5t5.c14.e2-1.dev/c-qa-images/contents/attachments/GkjtmeDSmtgm7tK7tzEPPGa41VzLiPsS.jpeg)
Answers
Answer:
The choose C. 2
Step-by-step explanation:
\(slope = \frac{y2 - y1}{x2 - x1} \\ = \frac{ - 2 + 4}{3 - 2} = \frac{2}{1} = 2\)
Answer:
option C is correct
Step-by-step explanation:
slope = \( \frac{ - 2 + 4}{3 - 2} \)\( = \frac{2}{1} \)\( = 2\)Hope it is helpful....
![What is the slope of the line shown below? A. [tex] \frac{1}{2} [/tex]B.[tex] - \frac{1}{2} [/tex]C.](https://i5t5.c14.e2-1.dev/h-images-qa/answers/attachments/D6TJDNSj249wasBUkaE4CoP2zN8BMlRw.jpeg)
AC is a diameter of OE, the area of the
circle is 289 units2, and AB = 16 units.
Find BC and mBC.
B
A
C
E. plssss hurry !!

Answers
The measure of arc BC is 720 times the measure of angle BAC.
Given that AC is the diameter of the circle and AB is a chord with a length of 16 units, we need to find BC (the length of the other chord) and mBC (the measure of angle BAC).
To find BC, we can use the property of chords in a circle. If two chords intersect within a circle, the products of their segments are equal. In this case, since AB = BC = 16 units, the product of their segments will be:
AB * BC = AC * CE
16 * BC = 2 * r * CE (AC is the diameter, so its length is twice the radius)
Since the area of the circle is given as 289 square units, we can find the radius (r) using the formula for the area of a circle:
Area = π * r^2
289 = π * r^2
r^2 = 289 / π
r = √(289 / π)
Now, we can substitute the known values into the equation for the product of the segments:
16 * BC = 2 * √(289 / π) * CEBC = (√(289 / π) * CE) / 8
To find mBC, we can use the properties of angles in a circle. The angle subtended by an arc at the center of a circle is double the angle subtended by the same arc at any point on the circumference. Since AC is a diameter, angle BAC is a right angle. Therefore, mBC will be half the measure of the arc BC.
mBC = 0.5 * m(arc BC)
To find the measure of the arc BC, we need to find its length. The length of an arc is determined by the ratio of the arc angle to the total angle of the circle (360 degrees). Since mBC is half the arc angle, we can write:
arc BC = (mBC / 0.5) * 360
arc BC = 720 * mBC
Therefore, the length of the arc BC equals 720 times the length of the angle BAC.
for such more question on measure of arc
https://brainly.com/question/25716982
#SPJ8
Tamara has a cell phone plan that charges $0.07 per minute plus a monthly fee o $ 19.00. She budgets 29.50 per month for total cell phone expenses without taxes. What is the maximum number of minutes Tamara could use her phone each month in order to stay within her budget
Answers
Answer:
150min
Step-by-step explanation:
Please answer will mark brainleast if correct its algebra.

Answers
the simple form of the given number will be 8√3.
What is square root?
A value known as the square root of a number is one that, when multiplied by itself, yields the original number. An alternative to square rooting a number is to use it. Therefore, the concepts of squares and square roots are connected. The original number is equal to the square root of any integer, which is equivalent to a number.
Let's assume that m is an integer that is positive, such that (m.m) = (m2) = m.
A square root function in mathematics is described as a one-to-one function that accepts a positive number as input and outputs the square root of the specified input number.
The given number is √192
= √ (2*2*2*2*2*2*3)
=8√3
Hence the simple form of the given number will be 8√3.
Learn more about square roots, by the following link.
https://brainly.com/question/428672
#SPJ1
Consider the system of equations.
x = 8 - 2y
x + 3y = 12
What value of x satisfies the system of equations?
Α. 4
B. 0
C. 5
D. 16
Answers
if f(x) = 3x -8, find f(6)

Answers
Answer:
f(6) = 3×6 - 8 = 10Step-by-step explanation:
To understand this, you have to take the f(6) as a x-value(input) and it will give output as 10.First multiply 6 by 3 and subtract 8 from it.I am sure that this helps ☺️❤️Please mark me as the brainliestAnswer:
The answer is 10
Step-by-step explanation:
f(x) = 3x - 8; f(6)
f(6) = 3(6) - 8
f(6) = 18 - 8
f(6) = 10
Thus, The answer is 10
-TheUnknownScientist 72
In Exercises below, is it possible for ABC XYZ and to be similar? Explain your reasoning.

Answers
Answer:
6) No, two angles are not equal
7) yes, two angles are equal
What is the area of a triangle whose vertices are R(3, 4), S(6, 2), and T(7, 10)?
Enter your answer in the box.
Answers
According to Heron's formula, the area of a triangle whose vertices are R(x, y) = (3, 4), S(x, y) = (6, 2) and T(x, y) = (7, 10) has a value of approximately 14.525 units.
How to calculate the area of a triangle by knowing its sides
In this question we must determine the lengths of the three sides of the triangle by Pythagorean theorem and then the area is determined by Heron's formula.
First, we determine the length of each side:
\(RS = \sqrt{(6-3)^{2}+(2-4)^{2}}\)
\(RS = \sqrt{3^{2}+(-2)^{2}}\)
\(RS = \sqrt{13}\)
\(ST = \sqrt{(7-6)^{2}+(10-2)^{2}}\)
\(ST = \sqrt{1^{2}+8^{2}}\)
\(ST = \sqrt{65}\)
\(RT = \sqrt{(7-3)^{2}+(10-4)^{2}}\)
\(RT = \sqrt{4^{2}+6^{2}}\)
\(RT = \sqrt{80}\)
Now we calculate the area of the triangle by Heron's formula:
\(s = \frac{\sqrt{13}+\sqrt{65}+ \sqrt{80}}{2}\)
s ≈ 10.306
\(A = \sqrt{10.306 \cdot (10.306 - \sqrt{13})\cdot (10.306 - \sqrt{65})\cdot (10.306 - \sqrt{80})}\)
A ≈ 14.525
According to Heron's formula, the area of a triangle whose vertices are R(x, y) = (3, 4), S(x, y) = (6, 2) and T(x, y) = (7, 10) has a value of approximately 14.525 units.
To learn more on triangles: https://brainly.com/question/2773823
#SPJ1
0.5x + 0.25(x + 16) = 4 + 0.75x
Answers
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️
\(0.5x + 0.25(x + 16) = 4 + 0.75x \\ \)
\( \frac{1}{2} x + \frac{1}{4} (x + 16) = 4 + \frac{3}{4} x \\ \)
\( \frac{1}{2} x + \frac{1}{4} x + \frac{16}{4} = 4 + \frac{3}{4} x \\ \)
\( \frac{2}{4} x + \frac{1}{4}x + 4 = \frac{3}{4} x + 4 \\ \)
\( \frac{3}{4} x + 4 = \frac{3}{4} x + 4 \\ \)
The sides are exactly have like terms ,
Thus we have infinite solution for the equation.
♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️♥️
You have 8 songs, 10 podcasts, and 12 audio books on your iCloud. How many ways can you choose 1 of each to download to your iPhone? PLEASE HELP
Answers
Answer:
960 ways
Step-by-step explanation:
Multiplication Principle: if there are n ways to do one thing and m ways to do another, then there are m x n ways to do both.
That can expand to include three things (or more).
8 x 10 x 12 = 960
The number of pounds that can be safely loaded onto an elevator, x, can be represented by
the inequality 2 <1,200. Which number line correctly represents this inequality?

Answers
\(\mathfrak{\huge{\orange{\underline{\underline{AnSwEr:-}}}}}\)
Actually Welcome to the Concept of the Inequalities.
Since the equation given as,
x is less that equalt to 1200 , then logically all the values before 1200 which are positive and also including 1200 should be there.
hence the correct number line is
Option 3.) that is number line with a red spot on 1200
helppp answer with right answer pleaseee

Answers
Answer:
the slope of the graph is 2
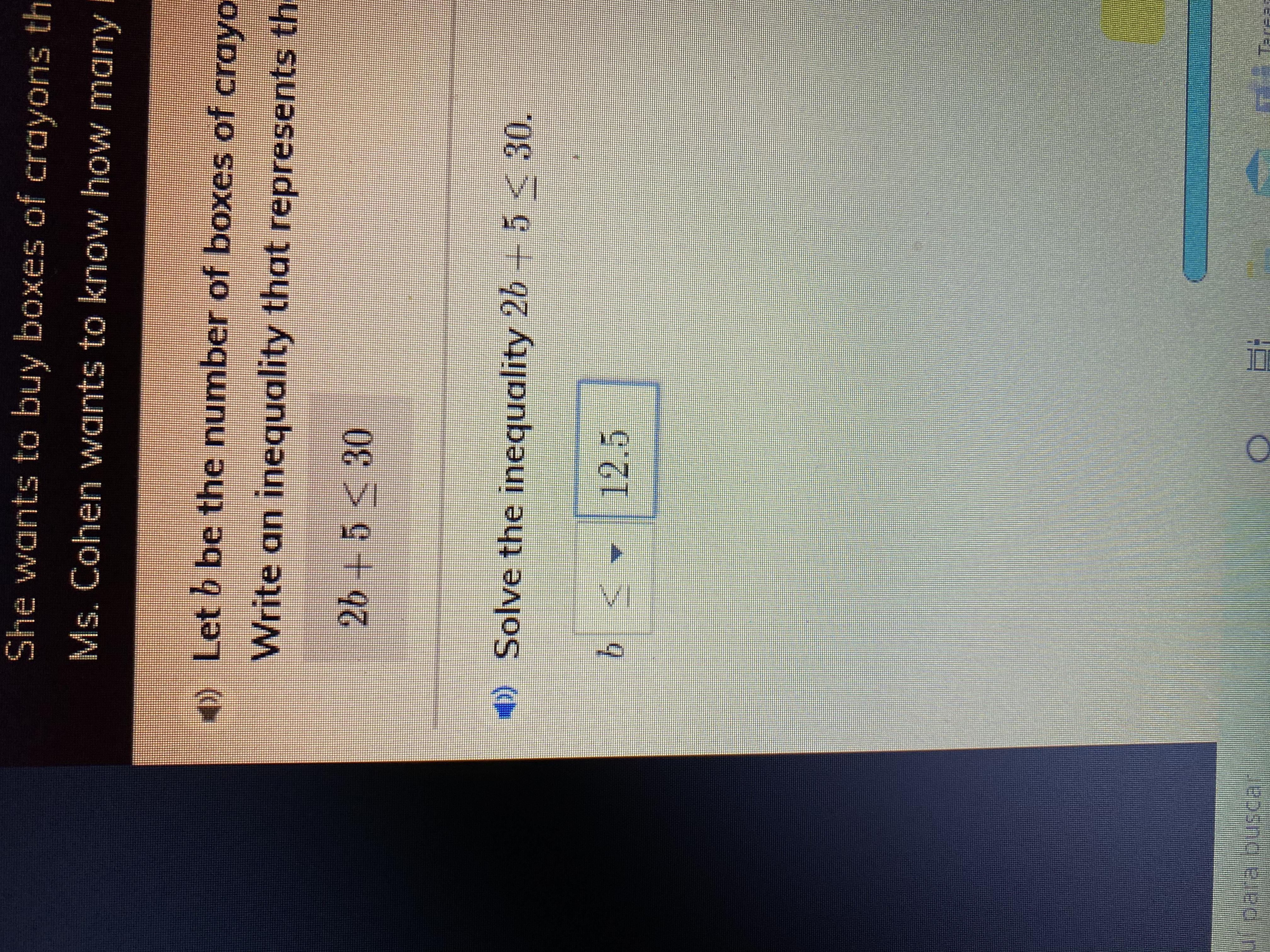
-) Ms. Cohen is buying supplies for her kindergarten classroom. She can spend at most $30.
She wants to buy boxes of crayons that cost $2 per box. She also buys a poster for $5.
Ms. Cohen wants to know how many boxes of crayons she can buy.
1) Let b be the number of boxes of crayons Ms. Cohen buys.
Write an inequality that represents the situation.
+
30
5
2
b.
Yes I give brainless!!

Answers
Answer:
Step-by-step explanation:
Nadia and Sasha are measuring different lengths of ribbon.
Use the drop-down menus to complete the statements about Nadia's and Sasha’s measurements.
Nadia's
Measurement Actual
Length
4 cm 5 cm
Sasha's
Measurement Actual
Length
96 cm 100 cm
Nadia was(blank)
cm off the actual measurement of 5 cm.
That is a percent error of
(blank)%.
Sasha was
(blank) cm off the actual measurement of 100 cm.
That’s a percent error of(blank)
%.
Because Nadia’s ribbon is(shorter/longer)
than Sasha’s ribbon, each centimeter of error in measurement results in a(shorter/longer)
percent error.
Answers
Nadia was 1cm off the actual measurement of 5 cm. That is a percent error of 20%.
Sasha was 4 cm off the actual measurement of 100 cm. That’s a percent error of 4%.
How to calculate the percentage error?From the information, the measurement was 4cm and the actual measurement was 5cm. Therefore, the difference will be:
= 5cm - 1cm
= 4cm
The percentage error will be:
= Difference in measurement / Actual measurement × 100
= 1/5 × 100
= 20%.
The percentage error for Sasha will be:
= 4/100 × 100
= 4%
Learn more about percentages on:
brainly.com/question/24304697
#SPJ1
(8^3x+1)/(2^x)
CORRECT ANSWER GETS BRAINLIEST! ASAP
Answers
Answer:
512x+1 over 2^x ........................
Step-by-step explanation:
:)
Each of the following statements contains a blunder. Explain in each case what is wrong...
(a) There is a high correlation between the age of American workers and their occupation.
The relationship between age and American workers' occupation has a curved relationship and correlation measures the strength of only the linear relationship between two variables
.Because occupation has a categorical (nominal) scale, we cannot compute the correlation between occupation and anything. Age would not have any association with American workers' occupation.
Occupation of American workers can not be correlated with other variables because it is too complex.
(b) We found a high correlation
(r = 1.17) between students' ratings of faculty teaching and ratings made by other faculty members.
The relationship between students' ratings of faculty teaching and ratings made by other faculty members would have more of a curved relationship and correlation measures the strength of only the linear relationship between two variables.
Because students' ratings of faculty teaching has a categorical (nominal) scale, we cannot compute the correlation between these ratings and anything.
The correlation between students' ratings of faculty teaching and ratings made by other faculty members would not be this high.
A correlation r = 1.17 is impossible because −1 ≤ r ≤ 1 always.
(c) The correlation between the sex of a group of students and the color of their cell phone was r = 0.29.
The relationship between sex and cell phone color has a curved relationship and correlation measures the strength of only the linear relationship between two variables.
There is no correlation between sex and cell phone color.
Because neither variable (sex and cell phone color) is quantitative, we cannot compute the correlation between them.
The correlation between sex and cell phone color would be much higher.
Answers
(a), occupation is a categorical variable, and correlation measures the linear relationship between two quantitative variables, so calculating a correlation between age and occupation is not appropriate. Secondly, in statement (b), student ratings of faculty teaching and ratings by other faculty members may have a curved relationship, and correlation only measures linear relationships.(c), the variables "sex" and "cell phone color" are both categorical, and correlation is only applicable to quantitative variables..
(a) There is a high correlation between the age of American workers and their occupation.
- The blunder here is that occupation is a categorical (nominal) variable, not a quantitative variable. Correlation measures the strength of the linear relationship between two quantitative variables, so it cannot be calculated between age and occupation.
- Furthermore, even if occupation were a quantitative variable, the relationship between age and occupation is unlikely to be linear. It may have a more complex or curved relationship, which correlation does not capture.
(b) We found a high correlation (r = 1.17) between students' ratings of faculty teaching and ratings made by other faculty members.
- The mistake in this statement is that student ratings of faculty teaching are typically based on ordinal scales, not nominal scales. Correlation calculations are appropriate for quantitative variables, but not for categorical variables or ordinal scales.
- Additionally, a correlation coefficient (r) cannot exceed 1 in absolute value. The range for correlation coefficients is always between -1 and 1, inclusive. Therefore, a correlation of 1.17 is impossible.
(c) The correlation between the sex of a group of students and the color of their cell phone was r = 0.29.
- Similar to the previous statements, the blunder here is that both variables, "sex" and "cell phone color," are categorical variables. Correlation is only applicable to quantitative variables, so it cannot be calculated between these variables.
- As the variables are categorical, they do not have a numerical relationship that correlation can measure. Therefore, stating a specific correlation coefficient (r = 0.29) between these variables is incorrect.
Learn more about quantitative : brainly.com/question/32236127
#SPJ11
What is the value of X?
A: 15
B: 27
C: 45
D: 12

Answers
Answer:
D
Step-by-step explanation:
WORTH 10 POINTS
can someone help me solve this question? Thank you.

Answers
Answer:
-10
Step-by-step explanation:
Variable k is denoted as vertical movement. In this case, the -10 is k. Therefore, the graph is moving 10 units down from the parent graph.
Jacob bought a book that cost $11.95 and magazines that cost $4.95 each. Isabella bought a book that cost $15.79 and magazines that cost $3.99 each. Jacob spent the same amount or less money than Isabella. Write an inequality to represent this real world problem.
Answers
Answer: 16.90 ≤ 19.78
Step-by-step explanation:
if this is what you mean, then here you go!
Which expressions are equivalent to 3/8(y + 16)
A) 3(8y+128)
B) 3(1/8y+2)
C) 3/8y + 6
D) 3/8y + 2
E) 1/8(3y+48)
Answers
Answer:
B,C, and E
Step-by-step explanation:
Multiply the stuff inside by the numbers outside
Answer:
B), C), E)
Step-by-step explanation:
In inferential statistics, the objective is to determine how probable it is that:
The alternative hypothesis is true.
The null hypothesis is true.
The alternative hypothesis is false.
The null hypothesis is false.
Answers
In inferential statistics, the objective is to determine the probability of the alternative hypothesis being true or the null hypothesis being true.
This involves using sample data to make inferences and draw conclusions about a larger population. By analyzing the data and performing statistical tests, we assess the likelihood of the alternative hypothesis or the null hypothesis being accurate.
The alternative hypothesis represents a claim or statement that contradicts the null hypothesis and suggests that there is a significant relationship or difference between variables. To determine its probability, statistical methods such as hypothesis testing and p-values are employed. These methods evaluate the strength of evidence against the null hypothesis and support the alternative hypothesis when the evidence is substantial.
Know more about null hypothesis here:
https://brainly.com/question/28098932
#SPJ11
