I1
30
mirror
50 ft
A student is walking to school and sees a mirror on the ground.
She stops a short distance from the mirror to practice her Geometry.
She knows her own height and the height of the school. She also knows
the distance from the mirror to the school from a previous Geometry activity,
She would like to calculate the distance she is
standing from the mirror.
The height of the school is 30 ft.
The distance from the school to the mirror is 50 ft.
The height of the student is 4.5 ft.
What is the distance d between the student and the mirror?
Show all your work.

Answers
BE is equal to (3) times the length of AD.
Using the Pythagorean theorem, we get:
The side opposite angle C is AC, which has length 2 times the side opposite angle A. Therefore, AC = 2AD.
The side opposite angle B is BC, which has length √(3) times the side opposite angle A. Therefore, BC = sqrt(3)AD.
AD = 1/2 AC = 1/2 (2AD) = AD
Therefore, AD = DC = AD, which means that AD = DC = AC/2.
AB²= AE² + BE²
We know that AB = 2AD (since triangle ABC is a 30-60-90 triangle), and we found that AD = AC/2. Therefore, AB = 2AC/2 = AC.
We also know that AE = AC/2, so we can substitute and simplify:
AC² = (AC/2)² + BE^2 4AC² = AC²+ 4BE² 3AC² = 4BE² BE² = 3AC² BE = sqrt(3)/2 AC
Substituting the expression we found for AC, we get:
BE = √(3)/2 (2AD) = √(3) AD
Therefore, BE is equal to √(3) times the length of AD.
To know more about Pythagorean theorem, visit:
https://brainly.com/question/14930619
#SPJ1
Related Questions
Cell Phone and Brain Cancer. In a study of 420,095 cell user in Denmark, it was found that 135 developed cancer of the brain or nervous system. If we assume that the use of cell phones has no effect on developing such cancer, then the probability of a person having such a cancer is 0.000340.
a) Assuming that cell phones have no effect on developing cancer, find the mean and standard deviation for the number of people in groups of 420,095 that can be expected to have cancer of the brain or nervous system.
b) Based on the results from part (a), it is unusual to find that among 420,095 people, there are 135 cases of cancer of the brain or nervous system? Why or why not?
c) What do these results suggests about the publicized concern that cell phones are a health danger because they increase the risk of cancer of the brain or nervous system?
Answers
a) Assuming that cell phones have no effect on developing cancer, the mean (μ) for the number of people in groups of 420,095 who can be expected to have cancer of the brain or nervous system.
It is given by μ = np, where n is the sample size and p is the probability of having the cancer. In this case, μ = 420,095 * 0.000340 = 143.03. The standard deviation (σ) can be calculated using the formula σ = √(np(1-p)). Plugging in the values, we get σ = √(420,095 * 0.000340 * (1-0.000340)) = 11.87.
b) It is unusual to find exactly 135 cases of cancer of the brain or nervous system among 420,095 people if we assume that cell phones have no effect on developing cancer. This is because the observed number of cases (135) is more than 3 standard deviations away from the mean (143.03). According to the empirical rule (or 68-95-99.7 rule), approximately 99.7% of the data should fall within 3 standard deviations of the mean in a normal distribution. Therefore, the observed number of cases is considered statistically significant and deviates from what would be expected under the assumption of no effect.
c) These results suggest that the observed number of cases of cancer of the brain or nervous system among cell phone users in the study is higher than what would be expected if cell phones had no effect on cancer risk. The fact that 135 cases were found among 420,095 people indicates a higher probability than the assumed 0.000340. This raises concerns about the potential link between cell phone use and an increased risk of cancer. However, it is important to note that this study alone does not provide conclusive evidence of a causal relationship. Further research is needed to establish a more definitive link between cell phone use and the development of brain or nervous system cancer.
To learn more about probability click here:
brainly.com/question/32117953
#SPJ11
Identify the period and determine where two asymptotes occur for each function.
y=tan3 θ /2
Answers
The period of the function y = tan(3θ/2) is π/3, and the asymptotes occur at θ = -π/6 and θ = π/6.
The general form of the tangent function is y = tan(bθ), where b is a constant that affects the period of the function. In this case, b = 3/2, so we need to determine the period based on this value. The period of the tangent function is given by π/|b|. Therefore, in this case, the period is π/(3/2) = 2π/3, which simplifies to π/3.
To determine the locations of the asymptotes, we set the argument of the tangent function equal to nπ, where n is an integer. In this case, we have: 3θ/2 = nπ
Solving for θ, we find: θ = (2nπ)/3
The asymptotes occur when the tangent function is undefined, which happens when the cosine of the angle is equal to zero. Since the cosine function is the reciprocal of the tangent function, we can find the asymptotes by setting the cosine of the angle equal to zero: cos(θ) = 0
Solving for θ, we find two solutions: θ = -π/6 and θ = π/6
Therefore, for the function y = tan(3θ/2), the period is π/3, and the asymptotes occur at θ = -π/6 and θ = π/6.
LEARN MORE ABOUT asymptotes here: brainly.com/question/32038756
#SPJ11
Can someone help me with this please and thank you!

Answers
Answer:
A
Step-by-step explanation:
I had this same question on a quiz the other day
help its iready ill give brainiest

Answers
Answer:
7(1/2)
Step-by-step explanation:
A punch recipe calls for 4 1/3
cups of pineapple juice per serving. Julie is making 1 2/5
servings.
Which equation shows the amount of pineapple juice
Answers
Answer:
The equation is 4 1/3(2/5+1)
Step-by-step explanation:
So, it's 4 1/3+1 11/15=
65/15+26/15=91/15
6 1/15
2/5x4 1/3
Which is 2/5 x 13/3
Which is 26/15
Which is 1 11/5
Please help write these into algebric expressions...
1) 18 is less than x is at most 42.
2) The product of z and 5 is less than or equal to 27.
3) The quotient of x and 2 is no more than 50.
Answers
=Z and 5 (less than equal to sign) 27
= X and 2 > 50
Two cards are dealt from a shuffled deck. What is the probability that the first card is an ace and the second card is a face card or a ten?
Answers
The probability that the first card is an ace and the second card is a face card is 1.78% and 12/52 respectively.
What is the probability of cards?
Probability is measured by counting and using basic math, such as addition and division. For example, you can multiply the number of spades in a complete deck (13) by the total number of cards in the deck (52) to get the probability of drawing a spade at random: 13 in 52, or 25%.
If you are dealt two cards successively from a standard 52-card deck, find the probability of getting an ace card on the first card and a face on the second.
you want to draw an ace. There are 4 aces out of the 52 cards in the deck so the probability of drawing an ace = 4/52.
So, the combined probability \((\frac{12}{52}) . (\frac{4}{52}) = (\frac{3}{13}) . (\frac{1}{13}) = (\frac{3}{169})\)
= 1.78%.
The probability of a face card on the second draw = 12/52 because there are 12 face cards (J, Q, K x 4 of each) and 52 cards in the deck.
Hence, the probability that the first card is an ace and the second card is a face card is 1.78% and 12/52 respectively.
To learn more about the probability of cards visit,
https://brainly.com/question/15812320
#SPJ4
HELP WITH GEOMETRY PLSSS

Answers
Does -3(x-4)= -3x=12 have one solution, many solutions, or no solutions at all?
Answers
Answer:
If the expression is:
-3x + 12 = -3x + 12, infinite solutions
If the expression is:
-3x + 12 = -3x - 12, no solutions
Step-by-step explanation:
Step 1: Distribute
-3x + 12 = 3x = 12
If both lines have the same slope and same y-int, they are the same line and therefore have infinite solutions.
If both lines have the same slope but different y-int, then they are parallel and therefore have no solutions.
Find mZHFG if mZEFG = 2x + 52, mZEFH = 21°, and mZHFG = 1 + 31

Answers
Answer:
m<HFG = 31
Step-by-step explanation:
We can say that m<EFH + m<HFG = m<EFG because of angle addition postulate. Now, we can substitute the values of the angles in.
21 + x + 31 = 2x + 52
x + 52 = 2x + 52
x = 0
Now, we substitute x into the expression of m<HFG:
m<HFG = x + 31
m<HFG = 0 + 31
m<HFG = 31
In the school store, all markups are 25%. If the store paid $12 for a t-shirt, what is the selling price?
Answers
Answer:
$15
Step-by-step explanation:
as the size of the sample is increased, the mean of increases. a. true b. false
Answers
It depends on the distribution of the population from which the sample is drawn.
If the population has a normal distribution and the sample is random, then as the size of the sample increases, the mean of the sample will approach the mean of the population. This is known as the law of large numbers.
However, if the population does not have a normal distribution, or if the sample is not random, then it is possible that the mean of the sample could increase or decrease as the sample size increases.
Learn more about distribution from
https://brainly.com/question/23286309
#SPJ11
HELPP MEE pleaseeeee

Answers
Answer:
-8,-4,-3,2,5
Step-by-step explanation:
There are 12 eggs in a dozen. Write an algebraic expression for the number of eggs in d dozen.
Answers
Answer:
4d=48
Step-by-step explanation:
What is the perimeter of △XYZ?
perimeter=

Answers
Step-by-step explanation:
(5+6+7)*2 = 36 correct answer
Answer:
36
Step-by-step explanation:
if a confidence interval is given from 43.85 up to 61.95 and the mean is known to be 52.90, what is the margin of error?
Answers
The margin of error can be calculated using the formula:
Margin of error = (upper limit of the confidence interval - lower limit of the confidence interval) / 2
In this case, a margin of error of 9.55 suggests that the sample mean is quite precise, since it's relatively close to the true population mean (which we know to be 52.90).
In this case, the lower limit of the confidence interval is 43.85 and the upper limit is 61.95.
Margin of error = (61.95 - 43.85) / 2
Margin of error = 9.55
Therefore, the margin of error is 9.55. This means that if the sample size were to be repeated, we would expect the sample mean to be within 9.55 units of the true population mean 95% of the time.
It's worth noting that the confidence interval provides a range of values within which we can be reasonably certain that the true population mean lies. The margin of error, on the other hand, gives us an indication of the precision of our estimate. However, if the margin of error were larger, this would indicate that our estimate is less precise and that we need a larger sample size to obtain a more accurate estimate of the population mean.
For more questions on population mean
https://brainly.com/question/26941429
#SPJ11
Why do we simplify fractions?
Answers
We simplify fractions because doing so always makes them easier to work with or compute.
A fraction is a piece of the entire. The number is shown as a quotient in mathematics, where the numerator and denominator are split. Both are integers in a straightforward fraction. A fraction appears in a complex fraction's numerator or denominator. The numerator of a valid fraction is smaller than the denominator. A fraction is a mathematical concept that denotes a component of a whole or, more generally, any number of equal parts. As employed in conversational English, a fraction—such as one-half, eight-fifths, and three-quarters—indicates the number of components of a particular size. With fractions, smaller portions of a whole are represented. The whole may be made up of one thing or several things. In any case, they collectively constitute what is referred to as a whole.
Learn more about fractions here
https://brainly.com/question/17220365
#SPJ4
Write the prime factorization of 21. Use exponents when appropriate and order the factors from least to greatest (for example, 2235).
Answers
The prime factorization of the number 21 is:
21 = 3*7
How to write the prime factorization?We want to write the prime factorization of 21.
To do so, we just need to divide the number by prime numbers.
The first prime number we can try is 2, if we divide by 2 we get:
21/2 = 10.5
This is not an integer, so 2 is not a factor.
The next one is 3:
21/3 = 7
Now we can rewrite:
21 = 3*7
Where 3 and 7 are prime numbers, so that is the prime factorization.
Learn more about prime factorizations:
https://brainly.com/question/1081523
#SPJ1
Can someone please help me on this?

Answers

Please solve this and show the steps on how you got your answer

Answers
well, let's take a look at the tickmarks on the triangle, the tickmarks mean that AC = CB, both AC and CB stemming out of vertex C, and both twin sides will make twin angles at the "base" or namely ∡A = ∡B, that means that 34 = x - 5, let's also recall that the sum of all interior angles in a triangle is 180°, so
\(34=x-5\implies \boxed{39=x} \\\\[-0.35em] ~\dotfill\\\\ \stackrel{\measuredangle A}{34}~~ + ~~\stackrel{\measuredangle B}{34}~~ + ~~\stackrel{\measuredangle C}{4y}~~ = ~~180\implies 68+4y=180 \\\\\\ 4y=112\implies y=\cfrac{112}{4}\implies \boxed{y=28}\)
Answer:
y = 28
Step-by-step explanation:
The dashes on line segments AC and BC indicate that the line segments are equal in length.
Therefore, as the triangle has two sides of equal length, it is an isosceles triangle.
The base angles of an isosceles triangle are equal, therefore ∠A = ∠B.
Interior angles of a triangle sum to 180°.
Therefore:
⇒ ∠A + ∠B + ∠C = 180°
⇒ 34° + 34° + 4y° = 180°
⇒ 68° + 4y° = 180°
⇒ 4y° = 112°
⇒ y = 112° ÷ 4°
⇒ y = 28
Has the marrying age of a man changed over the years? The United States Bureau of the Census takes a formal count of everyone in the U.S. every 10 years and has provided the following data that gives the median age of an American man at the time of his first marriage.
Year
1910
1920
1930
1940
1950
1960
1970
1980
1990
2000
Median Age
25.1
24.6
24.3
24.3
22.8
22.8
23.2
24.7
26.1
26.8
Determine the average rate of change in median age per year from 1930 to 1960.
a.
-0.5 years of age per year
b.
20 years of age per year
c.
-0.05 years of age per year
d.
+0.05 years of age per year
Please select the best answer from the choices provided
A
B
C
D
Answers
7 + 9 – (–3) – 1 + 2
pls help me
Answers
Answer:
20
Hope this helps! Please mark Brainliest!
Answer:20
Step-by-step explanation:
Hope it helps.
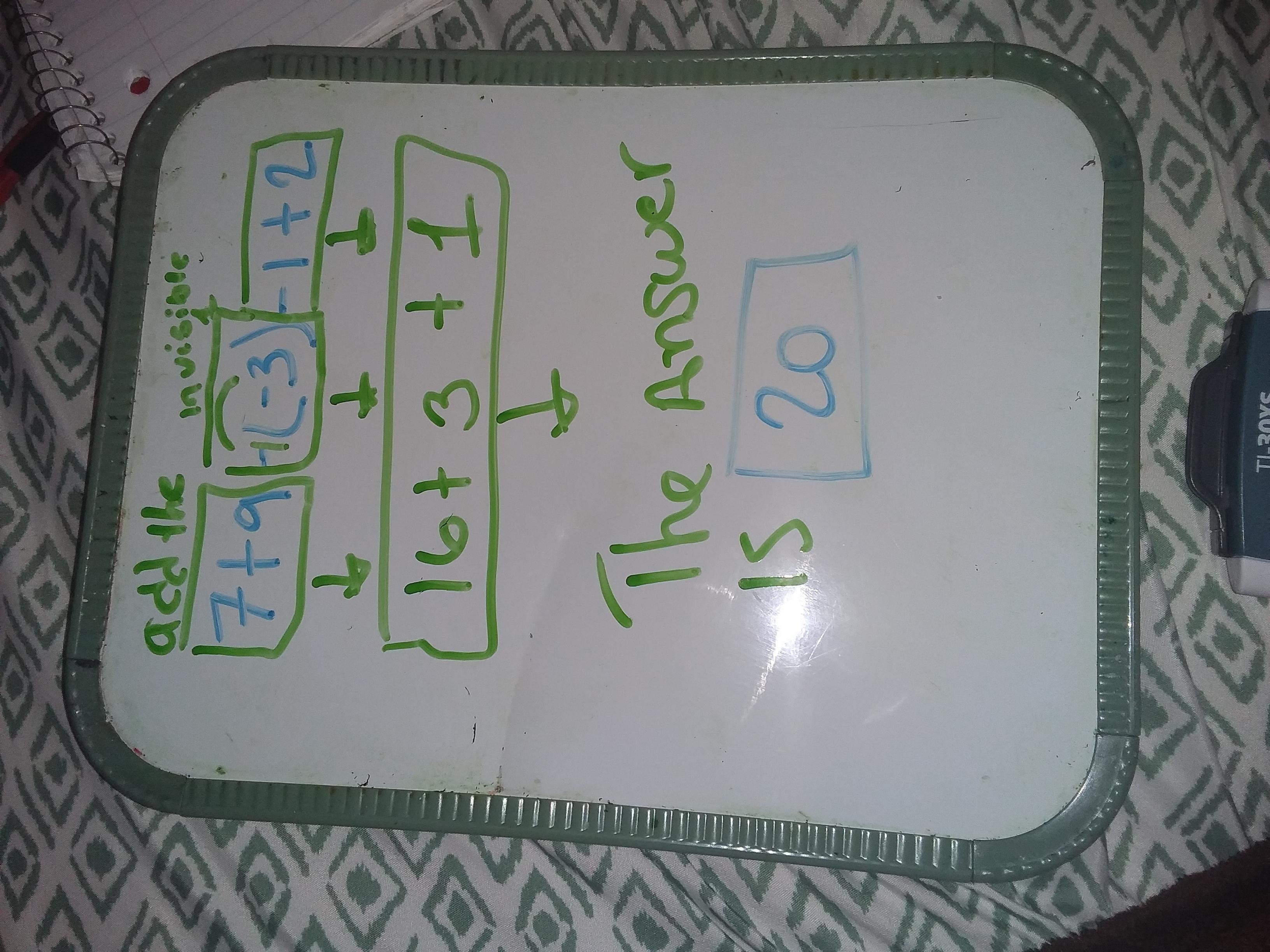
The numerator of a fraction is 2 less then its denominator. If 2 is subtracted from the numerator and 1 is the added to the denominator then the fraction becomed 1/2. Find the fraction.
Answers
Answer:
Probably, 3/1.
Step-by-step explanation:
Just do the reverse of the instructions and you get your answer.
1+2=3
2-1=1
The average number of misprints per page in a magazine is whixch follows a Poisson's Probability distribution. What is the probability that the number of misprints on a particular page of that magazine is 2?
Answers
The probability that a particular book is free from misprints is 0.2231. option D is correct.
The average number of misprints per page (λ) is given as 1.5.
The probability of having no misprints (k = 0) can be calculated using the Poisson probability mass function:
\(P(X = 0) = (e^{-\lambda}\times \lambda^k) / k!\)
Substituting the values:
P(X = 0) = \((e^{-1.5} \times 1.5^0) / 0!\)
Since 0! (zero factorial) is equal to 1, we have:
P(X = 0) = \(e^{-1.5}\)
Calculating this value, we find:
P(X = 0) = 0.2231
Therefore, the probability that a particular book is free from misprints is approximately 0.2231.
To learn more on probability click:
https://brainly.com/question/11234923
#SPJ4
Question 13: The average number of misprints per page of a book is 1.5.Assuming the distribution of number of misprints to be Poisson. The probability that a particular book is free from misprints,is B. 0.435 D. 0.2231 A. 0.329 C. 0.549
a critical value, z subscript alphazα, denotes the _______.
Answers
A critical value, z subscript alpha (zα), denotes the boundary or cutoff point for a statistical test where the level of significance, also known as alpha (α), is set.
A critical value, z subscript alpha (zα), denotes the value at which the probability of observing a test statistic in the tail(s) of the sampling distribution equals the pre-determined significance level (alpha).
The critical value can be defined as the value that is compared with the parameter value in the hypothesis test to determine whether the null hypothesis will be rejected. If the value of the parameter is less than the critical value, the null hypothesis is rejected.
However, if the measured value is higher than the critical value, reject the null hypothesis and accept the alternative hypothesis. In other words, cropping divides the image into acceptable and unacceptable areas. If the value of the index falls within the rejection range, the rejection of the fact is rejected, otherwise the negative hypothesis is rejected.
Learn more about Critical value:
brainly.com/question/30168469
#SPJ11
find range (R):27,12,15,21,24,9
Answers
Have a nice day!
Answer:
18
Step-by-step explanation:
The range is the highest data minus the smallest data.
Hence range is 27 - 9 = 18.
a coin is tossed 7 times. what is the probability of getting all heads? express your answer as a simplified fraction or a decimal rounded to four decimal places.
Answers
the probability of getting all heads after 7 tosses of a coin is 1/128, expressed as a simplified fraction or a decimal rounded to four decimal places is 0.0078.
When a coin is tossed, the probability of getting either heads or tails is 1/2. This probability remains constant regardless of the number of tosses.
In order to determine the probability of getting all heads after 7 tosses of a coin, we must calculate the probability of getting a head on each toss and multiply the individual probabilities together:
Probability of getting a head on the first toss = 1/2
Probability of getting a head on the second toss = 1/2
Probability of getting a head on the third toss = 1/2
Probability of getting a head on the fourth toss = 1/2
Probability of getting a head on the fifth toss = 1/2
Probability of getting a head on the sixth toss = 1/2
Probability of getting a head on the seventh toss = 1/2
The probability of getting all heads = (1/2) x (1/2) x (1/2) x (1/2) x (1/2) x (1/2) x (1/2) = 1/128
Therefore, the probability of getting all heads after 7 tosses of a coin is 1/128, expressed as a simplified fraction or a decimal rounded to four decimal places is 0.0078.
Answer: 0.0078
learn more about probability here
https://brainly.com/question/13604758
#SPJ11
Please help me with 1,2,3,4 and show me how you get it the answers

Answers
Answer:
Step-by-step explanation:
Use the formula of distance between the two points \((x_1,y_1)\) and \((x_2,y_2)\),
Distance = \(\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
1. Distance between A(3, 4) and B(8, 12),
AB = \(\sqrt{(8-3)^2+(12-4)^2}\)
AB = \(\sqrt{25+64}\)
= \(\sqrt{89}\)
≈ 9.43
2. Distance between C(-2, 10) and D(3, -2),
CD = \(\sqrt{(3+2)^2+(-2-10)^2}\)
= \(\sqrt{25+144}\)
= \(\sqrt{169}\)
= 13.00
3. Distance between X(20, 4) and Y(17, 0),
XY = \(\sqrt{(20-17)^2+(4-0)^2}\)
= \(\sqrt{3^2+4^2}\)
= \(\sqrt{25}\)
= 5.00
4. Distance between E(2, -1) and M(6, 9),
EM = \(\sqrt{(2-6)^2+(-1-9)^2}\)
= \(\sqrt{16+100}\)
= \(\sqrt{116}\)
= 10.77
Replace the polar equation rcosθ+rsinθ=1
with an equivalent Cartesian equation. Then identify the graph.
Answers
Since r = √(x² + y²) in Cartesian coordinates, we can substitute it into the equation: x + y = √(x² + y²).
How can we convert a polar equation to a Cartesian equation?To convert the polar equation rcosθ + rsinθ = 1 into a Cartesian equation, we can use the trigonometric identities cosθ = x/r and sinθ = y/r.
Substituting these identities, we get x + y = r. Since r = √(x² + y²) in Cartesian coordinates, we can substitute it into the equation: x + y = √(x² + y²).
To identify the graph, we can rearrange the equation to form x² + y² = (x + y)², which simplifies to x² + y² = x² + 2xy + y². Canceling out the x² and y² terms, we obtain 2xy = 0.
This equation represents two perpendicular lines, one along the x-axis and the other along the y-axis, intersecting at the origin.
Learn more about Cartesian
brainly.com/question/11676110
#SPJ11
√15
3√6
This question is for radicals I have to do it for credit for this assignment and need help
Answers
Answer:
Step-by-step explanation:
And here is how to use it:
Example: simplify √12
12 is 4 times 3:
√12 = √(4 × 3)
Use the rule:
√(4 × 3) = √4 × √3
And the square root of 4 is 2:
√4 × √3 = 2√3
So √12 is simpler as 2√3
Another example:
Example: simplify √8
√8 = √(4×2) = √4 × √2 = 2√2
(Because the square root of 4 is 2)
And another:
Example: simplify √18
√18 = √(9 × 2) = √9 × √2 = 3√2
It often helps to factor the numbers (into prime numbers is best):
Example: simplify √6 × √15
First we can combine the two numbers:
√6 × √15 = √(6 × 15)
Then we factor them:
√(6 × 15) = √(2 × 3 × 3 × 5)
Then we see two 3s, and decide to "pull them out":
√(2 × 3 × 3 × 5) = √(3 × 3) × √(2 × 5) = 3√10
Fractions
There is a similar rule for fractions:
root a / root b = root (a / b)
Example: simplify √30 / √10
First we can combine the two numbers:
√30 / √10 = √(30 / 10)
Then simplify:
√(30 / 10) = √3
Some Harder Examples
Example: simplify
√20 × √5
√2
See if you can follow the steps:
√20 × √5
√2
√(2 × 2 × 5) × √5
√2
√2 × √2 × √5 × √5
√2
√2 × √5 × √5
√2 × 5
5√2
Example: simplify 2√12 + 9√3
First simplify 2√12:
2√12 = 2 × 2√3 = 4√3
Now both terms have √3, we can add them:
4√3 + 9√3 = (4+9)√3 = 13√3