BRAINLIEST FOR THE CORRECT AWNSER! The slope of the line below is –3. Use the coordinates of the labeled point to find a point-slope equation of the line.


Answers
Answer: y+7 = 3(x-5) A
Step-by-step explanation: Plug in the points into y-y1= m(x-b)
Related Questions
Question 26 2 pts A century ago, the average height of adult women in the United States was 63 inches. Researchers believe that the average might be greater today. A random sample of 40 adult women was selected from the population. The sample had mean 64.2 inches and standard deviation 2.9 inches. Assuming all conditions for inference are met, the researchers will perform an appropriate hypothesis test to investigate their belief. Which of the following is the correct test statistic for the hypothesis test? 0.4137 0 -0.2617 O-0.4137 0.2617
Answers
The correct test statistic for this hypothesis test is 3.21 or 0.2617
To determine the appropriate test statistic for this hypothesis test, we need to first state the null and alternative hypotheses.
In this case, the null hypothesis is that the population mean height of adult women is equal to 63 inches, while the alternative hypothesis is that the population mean height is greater than 63 inches.
Next, we can use the formula for a t-test to calculate the test statistic:
t = (sample mean - hypothesized mean)/(sample standard deviation/sqrt(sample size))
Plugging in the given values, we get:
t = (64.2 - 63)/(2.9√40) = 3.21 or 0.2617
Therefore, the correct test statistic for this hypothesis test is 3.21. or 0.2617
To determine whether this test statistic is statistically significant, we would need to compare it to a critical value from the t-distribution with 39 degrees of freedom (since we have a sample size of 40 and are estimating one parameter, the population mean). If the test statistic is greater than the critical value, we can reject the null hypothesis and conclude that the population mean height of adult women is greater than 63 inches at a given level of significance.
In summary, the correct test statistic for this hypothesis test is 3.21. To determine whether this test statistic is statistically significant, we would need to compare it to a critical value from the t-distribution with 39 degrees of freedom.
To know more about test statistic: click here:
brainly.com/question/14128303
#SPJ4
Jane figures that her monthly car insurance payment of $170 is equal to 30% of the amount of her monthly auto loan payment. What is her total combined monthly expense for auto loan payment and insurance (rounded to the nearest dollar)?
Answers
Jane's total combined monthly cost for auto loan payment and insurance is $736.67 (rounded to the nearest dollar).
If Jane's monthly car insurance payment of $170 is same to 30% of her monthly car loan fee, then we are able to set up the subsequent equation:
0.3x = 170
Where x is the monthly auto loan fee. To solve for x, we will divide each facets by using 0.3:
x = 170 / 0.3 = $566.67
So, Jane's monthly auto loan charge is $566.67.
To discover her general combined monthly price for auto loan price and insurance, we simply upload her monthly car coverage charge to her monthly auto loan payment:
$566.67 + $170 = $736.67
Consequently, Jane's total combined monthly cost for auto loan payment and coverage is $736.67
Learn more about auto loan payment:-
https://brainly.com/question/31440727
#SPJ4
Rewrite the fraction as a decimal.
7
4
=
Answers
it would be 1.75
Answer: 1.75
Step-by-step explanation:
7/4 = 7 divided by 4, so the answer is 1.75
A bakery offers a sale price of 2.55 for 4 muffins.what is the price per dozen?
I hope someone answers fast
And explain what you did
Answers
Answer: $7.65 for a dozen/12
Step-by-step explanation: 2.55 x 3
Which multiplication equation can be used to explain the solution to 15/ by 1/3 of a fraction?
Answers
Answer:
Multiply by the inverse.
Answer: 45
Step-by-step explanation:
(15)/(1/3) is the same thing as multiplying the top (numerator), by the inverse of the bottom (denominator).
So we have the following: \(\frac{15/1}{1/3}\)
Taking the inverse means flipping the fraction. The inverse of \(\frac{1}{3}\) is \(\frac{3}{1}\). So we can keep the top, and multiply by the inverse.
\(\frac{15}{1}\) X \(\frac{3}{1}\) = 15 x 3 = 45.
Does this make sense?
J={x | x is an integer and -4

Answers
J = {-3, -2, -1}
Explanation:J={x | x is an integer and -4 < x ≤ to -1}
Integers are negative and positive whole numbers. This means that decimals will not be included in the set
From the set, x will start from integers greater than -4 but stop at -1.
The given set can be rewritten by listing as:
J = {-3, -2, -1}
Anyone know how to do 5c???? Please help!!!!!

Answers
Answer:
71° F
Step-by-step explanation:
Consider the rational part of f(t)
\(\frac{40t^3}{t^3+100}\) ( divide numerator and denominator by t³
= \(\frac{40}{1+\frac{100}{t^3} }\)
As t → infinity then \(\frac{100}{t^3}\) → 0 , the expression simplifies to
\(\frac{40}{1+0}\) = \(\frac{40}{1}\) = 40
Thus after driving for a long time
f(t) = 31 + 40 = 71° F
for the normal distribution, the mean /- 1.96 standard deviations will include about what percent of the observations?
Answers
The statement that the mean ± 1.96 standard deviations will include approximately 95% of the observations is in line with the empirical rule. This rule provides a rough estimate of the proportion of observations within a certain number of standard deviations from the mean in a normal distribution. In the case of ±1.96 standard deviations, it captures about 95% of the data.
For the normal distribution, the mean ± 1.96 standard deviations will include approximately 95% of the observations.
This is based on the empirical rule, also known as the 68-95-99.7 rule, which states that for a normal distribution:
- Approximately 68% of the observations fall within one standard deviation of the mean.
- Approximately 95% of the observations fall within two standard deviations of the mean.
- Approximately 99.7% of the observations fall within three standard deviations of the mean.
Since ±1.96 standard deviations captures two standard deviations on either side of the mean, it covers approximately 95% of the observations, leaving only about 5% of the observations outside this range.
Therefore, about 95% of the observations will be included within the range of the mean ± 1.96 standard deviations.
learn more about "mean ":- https://brainly.com/question/1136789
#SPJ11
Brad, Janet, and Kim went to a yard sale. They each spent $8. [Assume items of the same type had the same price.] • Brad bought 4 boxes of books. • Janet bought 3 boxes of books and 4 candles. • Kim bought 2 boxes of books, 1 box of t-shirts, and 3 candles. What was the price of one candle?
Answers
Consider "Since some shoes are sneakers, some non-sneakers are non-shoes." This inference, drawn by contraposition, is:
A) Valid
B) Not valid
C) Valid by limitation
Answers
The correct option is B) Not valid. Consider the statement “Since some shoes are sneakers, some non-sneakers are non-shoes.”
We know that all sneakers are shoes, but all shoes are not sneakers. Hence, some shoes are sneakers. But, this statement does not imply that “some non-sneakers are non-shoes.” This inference cannot be drawn by contraposition. So, it is not valid.Inferences cannot be drawn in contraposition all the time. Contraposition is a type of logical statement that involves reversing and negating the terms of an original proposition. It is a logical relationship between a proposition and its converse. If a proposition is true, the contrapositive is always true.An example of contraposition can be shown as follows:“If a person is a human, then they are mortal.”We can write the contrapositive of this statement as:“If a person is not mortal, then they are not human.”
To know more about contraposition visit:
https://brainly.com/question/18120338
#SPJ11
the cost of buying shoes is shown in the table below

Answers
Answer:
Part AThe graph is attachedPart BFor zero number the cost is zero and the rate of change is 25.
0 - 0, 1 - 25, 2 - 50, 3 - 75, 4 - 100This is the indication of the proportional relationship
Part CIt is a constant change as the difference is same for each step - 25The ordered pair (1, 25) shows the unit rate is $25 per pair of shoesPart DConstant of proportionality is 25Part EThe equation is:
y = 25x,where y - cost, x - number of pairs of shoes, 25 - constant of proportionality

I need help on this problem it’s very hard
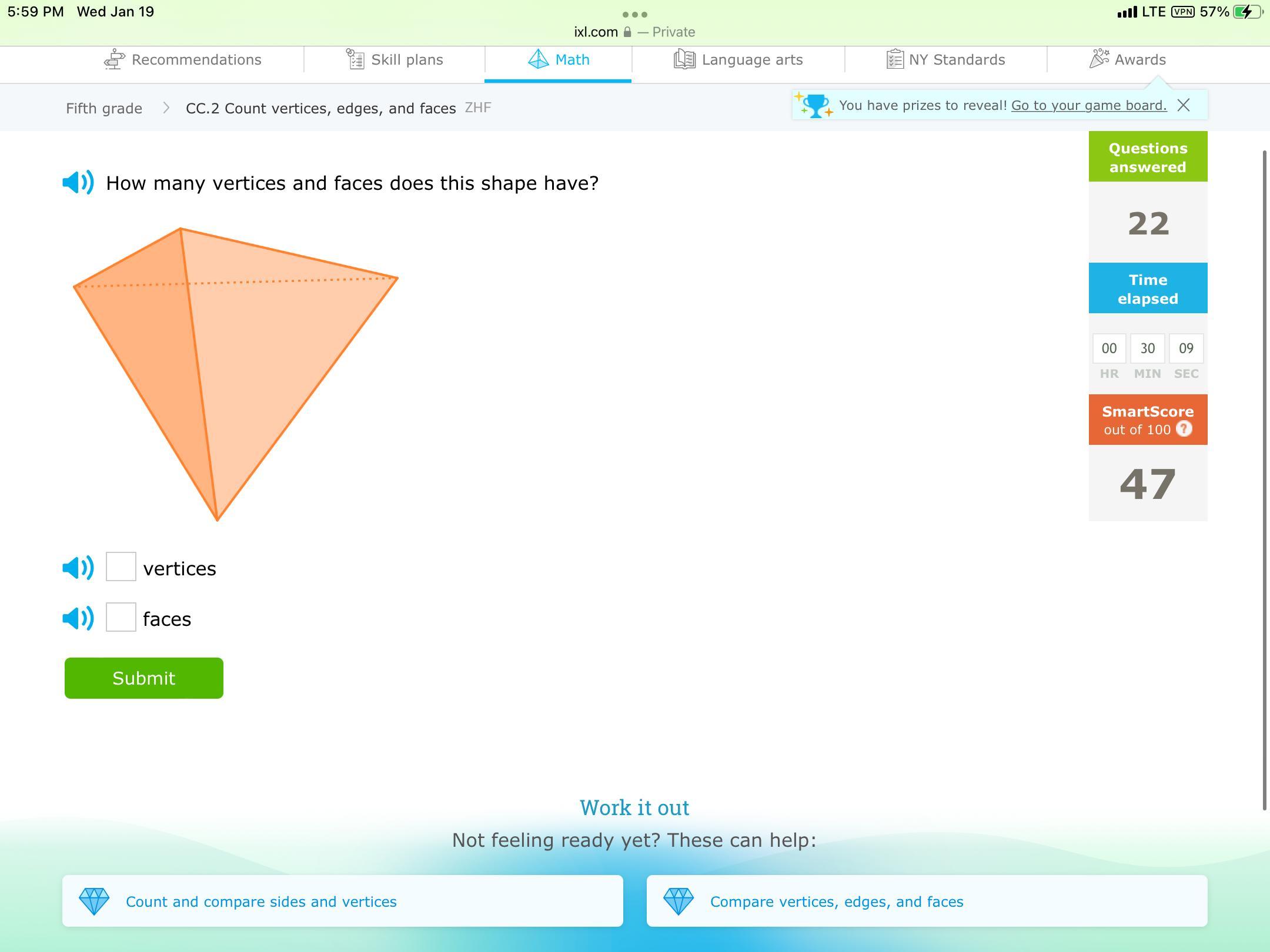
Answers
Answer:
3 faces and 4 verticies sorry if im wrong
Answer:
I would say 3 faces and 4 vertices
list the set of ordered pairs that had a domain that consists of multiples of 3 from -3 to 15
Answers
Answer:
Multiple of 3 from -3 to 15 are:-
3, 6, 9, 12 , 15
hope it is helpful to you ☺️
The ratio of brown tiles to white tiles in Mrs.Harris kitchen in 4 to 5. There are 180 white tiles in the kitchen. What is the total number of tiles in mrs. Harris kitchen
Answers
Answer:
405
Step-by-step explanation:
Don't be confused about setting up the proportion. White has to go with white which is 4 and brown goes with 5.
The total is 9.
so proportion will look like this.
4/9 = 180/x Cross Multiply
Before I solve this, notice that the white data is on top of the fraction, and the total is on the bottom of the fraction on either side of the proportion.
4x = 180 * 9
4x = 1620
x = 1620 / 4
x = 4 0 5
Notice that the brown is going to be bigger than the white but not by much
White 180
Brown 405 - 180 = 225
Total 405
A bag contains 10 blue marbles, 3 yellow marbles, and 12 orange marbles. If a blue marble is drawn you win $10. If a yellow marble is drawn you win $15. if an orange marble is drawn you lose $10. it cost $1 to play. Should you play the game?

Answers
Answer:
The correct option is -
Yes, because the Expected value is 1 and not negative which would imply that there is a chance of winning.
Step-by-step explanation:
Given - A bag contains 10 blue marbles, 3 yellow marbles, and 12 orange marbles. If a blue marble is drawn you win $10. If a yellow marble is drawn you win $15. if an orange marble is drawn you lose $10. it cost $1 to play.
To find - Should you play the game?
Formula used -
Expected value , E[x] = ∑x p(x)
where p(x) is the probability.
Proof -
Given that,
Total blue marbles in a bag = 10
Total yellow marbles in a bag = 3
Total orange marbles in a bag = 12
So,
Total number of marbles in a bag = 10 + 3 + 12 = 25
Now,
Probability of getting blue marble = \(\frac{10}{25}\)
Probability of getting yellow marble = \(\frac{3}{25}\)
Probability of getting orange marble = \(\frac{12}{25}\)
So,
Expected value , E[x] = ∑x p(x)
= (10)( \(\frac{10}{25}\)) + (15)(\(\frac{3}{25}\)) + (-10)(\(\frac{12}{25}\))
= \(\frac{100}{25} + \frac{45}{25} - \frac{120}{25}\)
= \(\frac{100 + 45 - 120}{25}\)
= \(\frac{25}{25}\)
= 1
So,
Expected value = 1
So,
The correct option is -
Yes, because the Expected value is 1 and not negative which would imply that there is a chance of winning.
solve the given initial-value problem. dy/dt 2(t+1)y2 = 0, y(0) = − 1/15 y(t) = 1/t^2 + 2t + 15Give the largest interval i on which the solution is defined. (enter your answer using interval notation.)
Answers
The largest interval on which the solution is defined is (-∞, -1) ∪ (-1, ∞). The interval notation for the largest interval is (-∞, -1) U (-1, ∞).
What is the initial-value problem?An initial-value problem is a type of boundary-value problem in mathematics, particularly in the field of differential equations.
The given initial-value problem is a separable differential equation, which can be written as:
dy/dt = -2(t + 1)y²
Integrating both sides, we get:
(1/y) = t² + 2t + C
where C is the constant of integration.
Since we have an initial condition, we can use it to find the value of C:
y(0) = -1/15
C = -1/15
Solving for C, we get:
C = -1/15
So, the solution to the differential equation is:
(1/y) = t² + 2t -1/15
y = 1 / (t² + 2t -1/15)
The solution is defined for all t ≠ -1, since y = 0 is not defined. So, the largest interval on which the solution is defined is (-∞, -1) ∪ (-1, ∞). The interval notation for the largest interval is (-∞, -1) U (-1, ∞).
Learn more about the initial value problem here:
https://brainly.com/question/30503609
#SPJ1
Jonah had 3/4 cup of water in his cup. He drank 1/2 cup of water. How
much water did he have left?
Answers
Answer:
1/4th Cup left
Step-by-step explanation:
1/2 cup is equal to 2/4 cup, so subtract that from 3/4th and you end with 1/4th
A boat is heading towards a l, whose beacon-light is 113 feet above the water. The boat’s crew measures the angle of elevation to the beacon, 11 ∘ ∘ . What is the ship’s horizontal distance from the lighthouse (and the shore)?
Answers
113 % = 1893per capacity
Step-by-step explanation:
first you have to calculate the 113 feet with its equation then calculate the quation with the number 69 divisible by it then divide it and your answer is there
As a reminder, here again is the example from the previous page.A group of engineers developed a new design for a steel cable. They need to estimate the amount of weight the cable can hold. The weight limit will be reported on cable packaging.The engineers take a random sample of 45 cables and apply weights to each of them until they break. The mean breaking weight for the 45 cables is768.2x¯=768.2 lb. The standard deviation of the breaking weight for the sample of cables is s = 15.1 lb.In the example we concluded the following. We are 95% confident that the mean breaking weight for all cables of this type is between 763.7 lb and 772.7 lb.In other words the 95% confidence interval is (763.7, 772.7).Here again is the applet.T-distribution Inverse CalculatorSuppose the engineers want to make a 99% confidence interval. They plan to use the same sample of 45 cables. Use the applet to find the critical T-value they will use in the computation of the margin of error. Construct the 99% confidence interval. Enter the lower bound of the interval (the smaller number). If necessary, round to two decimal places. What is the upper bound of the 99% confidence interval? If necessary, round to two decimal places. In general, does increasing the confidence level "does not affect/increases/decreases" the margin of error.
Answers
To find the critical T-value for a 99% confidence interval with a sample size of 45, we need to enter the degrees of freedom (df = n-1 = 44) and the confidence level (CL = 99%) into the T-distribution Inverse Calculator applet. This gives us a T-value of 2.691.
To construct the 99% confidence interval, we use the formula:
x¯ ± T × s/√n
where x¯ is the sample mean (768.2 lb), s is the sample standard deviation (15.1 lb), n is the sample size (45), and T is the critical T-value (2.691). Plugging in these values, we get:
768.2 ± 2.691 × 15.1/√45
which simplifies to:
(755.2, 781.2)
Therefore, the lower bound of the 99% confidence interval is 755.2 lb (rounded to two decimal places) and the upper bound is 781.2 lb (also rounded to two decimal places).
Increasing the confidence level typically increases the margin of error. This is because a higher confidence level requires a wider interval to capture a larger proportion of possible sample means.
To construct a 99% confidence interval for the mean breaking weight of the steel cables using the given sample, we'll need to find the critical T-value. For a sample of 45 cables, the degrees of freedom (df) is 44 (n-1). Using a T-distribution Inverse Calculator with a 99% confidence level and 44 degrees of freedom, we find the critical T-value to be approximately 2.69.
Now, we can compute the margin of error:
Margin of Error = T-value * (s/√n) = 2.69 * (15.1/√45) ≈ 6.03
The 99% confidence interval is then calculated as follows:
Lower Bound: x- Margin of Error = 768.2 - 6.03 ≈ 762.17
Upper Bound: x + Margin of Error = 768.2 + 6.03 ≈ 774.23
So, the 99% confidence interval is (762.17, 774.23).
In general, increasing the confidence level increases the margin of error, as we need a wider interval to be more confident that it contains the true population mean.
Visit here to learn more about confidence interval brainly.com/question/24131141
#SPJ
A baseball player has a batting average of 0.250, which means he gets a hit 25% of the time he is at bat. If he bats 450 times during the season, how many hits should he expect to get?
Answers
Answer:
112.5
Step-by-step explanation:
25% of 450 = .25 x 450 = 112.5
Directions: Transform the following equations into standard form.
1. x2 + 2x = -1
2. 4 + x2 = 7x
3. x2 - x = 1
4. x2 + 9x = 10
5. 4x + 3 = x2
6. x2 + 5x +5
7. 2x5 + 7x = 3x
8. x2 = 3x + 8
9. x(x + 3) = 1
10. x2 - 3x = -2
11. y2 - 7y = -6
12. z2 - 5z = -4
Answers
The standard form is:
ax² + bx + c = 0Convert each equation:
1. x² + 2x = -1 add 1 to both sides
⇒ x² + 2x + 1 = 0
2. 4 + x² = 7x add - 7x to both sides and rearrange
⇒ x² - 7x + 4 = 0
3. x² - x = 1 add - 1 to both sides
⇒ x² - x - 1 = 0
4. x² + 9x = 10 add - 10 to both sides
⇒ x² + 9x - 10 = 0
5. 4x + 3 = x² add -4x - 3 to both sides
⇒ x² - 4x - 3 = 0
6. x² + 5x = 5 add -5 to both sides
⇒ x² + 5x - 5 = 0
7. 2x² + 7x = 3 add -3 to both sides
⇒ 2x² + 7x - 3 = 0
8. x² = 3x + 8 add -3x - 8 to both sides
⇒ x² - 3x - 8 = 0
9. x(x + 3) = 1 distribute and add - 1 to both sides
⇒ x² + 3x - 1 = 0
10. x² - 3x = -2 add 2 to both sides
⇒ x² - 3x + 2 = 0
11. y² - 7y = -6 add 6 to both sides
⇒ y² - 7y + 6 = 0
12. z² - 5z = -4 add 4 to both sides
⇒ z² - 5z + 4 = 0
Answer: ^^
1. x2 + 2x + 1 = 0
2. x^2 - 7x + 4 = 0
3. x^2 - x - 1 = 0
4. x^2 + 9x - 10 = 0
5. -x^2 + 4x + 3 = 0
6. x^2 + 5x - 5 = 0
7. 2x^5 + 7x - 3 = 0 or 2x^5 + 4x = 0
8. x^2 - 3x - 8 = 0
9. x^2 + 3x - 1 = 0
10. x^2 - 3x + 2 = 0
11. y^2 - 7y + 6 = 0
12. z^2 - 5z + 4 = 0
Step-by-step explanation:
:)
Factors of 64a3 - 343b 3 are
Answers
\(\huge\mathfrak\green{Constant}\)
Constant is the number that cannot change the value
Im not sure..but you can copy it
please answer fast thank you

Answers
I think it would be the red line its going to be Negative
around 0 to 2
please help find the area!!

Answers
Answer:
163.73
Step-by-step explanation:
Triangle area:
16x12 = 192cm²
Circle area:
pi(3²) = 28.27cm²
Difference in the area:
192-28.27 = 163.73cm²
Hi there can someone please help me?
What is z and k when k+4z=42
Answers
Answer:
Solution given:
we have
k+4z=42
k=42-4z
and
k+4z=42
4z=42-k
dividing both side by 4
\(\frac{4z}{4}\)=\(\frac{42-k}{4}\)
z=\(\bold{\frac{42-k}{4}}\)
please help me with right answers xoxo

Answers
simplify (-4)(9)/(-6)
Answers
-36 divided by -6 = 6
The answer is 6.
While eating in the cafeteria at school, 65%, or 26, of the students in the class ate pizza. Find the number of students in the entire class
Answers
The number of students in the entire class is 40.
How to calculate the value?A percentage is a value or ratio that may be stated as a fraction of 100. If we need to calculate a percentage of a number, we should divide it's entirety and then multiply it by 100. The percentage therefore refers to a component per hundred.
From the information given, when eating in the cafeteria at school, 65%, or 26, of the students in the class ate pizza.
Let the total number of people be illustrated as x.
This will be:
65% × x = 26
0.65x = 26
Divide
x = 26/0.65
x = 50
The total students is 40.
Learn more about percentages on:
brainly.com/question/24877689
#SPJ1
Graph the function f(x) = 2x. Which features are correctly stated?
A) x-intercept: none
B) y-intercept: (0, 2)
C) asymptote: x = 0
D) as x → ∞, f(x) → ∞
E) as x → −∞, f(x) → 0
Answers
Answer:
a, d, e
Step-by-step explanation:
on usatestprep
The features of the function are given as follows:
Horizontal asymptote: y = 5.
Vertical asymptote: x = 2.
x-intercept: No x-intercept.
y-intercept: (0,-5).
Hole: No holes.
What are the features of the function?The horizontal asymptote is the limit of f(x) as x goes to infinity. To obtain this limit, we consider only the terms with the highest exponent of the numerator and of the denominator, hence:
here, we have,
g(x) = -5x/x -> y = 5 is the horizontal asymptote.
The vertical asymptote is the value of x for which the function is not defined, hence it is the zero of the denominator, thus:
x - 2 = 0 -> x = 2.
The x-intercept is the point (x,0), in which x is found when y = 0, hence:
-5x + 10 = 0
-5x = -10
5x = 10
x = 2.
However, the function is not defined at x = 2, meaning that it has no x-intercept.
The y-intercept is the value of y when x = 0, hence:
y = 10/-2 = -5.
The coordinates are (0,-5).
The entire function can be simplified as follows:
(-5x + 10)/(x - 2) = [-5(x - 2)]/(x - 2) = -5.
Meaning that the function has no holes.
More can be learned about functions at
brainly.com/question/24808124
#SPJ2
complete question:
Determine each feature of the graph of the given function.
f(x) = -5+10
2
Horizontal Asymptote: y =
Vertical Asymptote: x =
x-Intercept:
y-Intercept: (0,
Hole: (
,0)
No horizontal asymptote
No vertical asymptote
No x-intercept
No y-intercept
No hole
what is a similarity statement, and how do you write one?
Answers
Answer:
A similarity statement is a statement used in geometry to prove exactly why two shapes have the same shape and are in proportion. You can write one by labeling all the angles. Write down all the congruent angles (for example, angle ABC is congruent to angle DEF, angle BCA is congruent to angle EFD, etc.). Then, calculate all the lengths of the sides of the triangles and confirm that they are in proportion. After that, you are ready to write the similarity statement.
Step-by-step explanation: