can earn 5 coins
Dimitri pays 9% sales tax on a bag of groceries that costs 46 dollars. How much does the sales tax add to
the purchase price?
Answers
Answer:4.14
Step-by-step explanation:
Answer:
474
Step-by-step explanation:
took the test on edg
Related Questions
Write an equation of the line perpendicular to line MN that goes through point Q.
Francisco has solved the problem for you, but made a mistake.
Find the error in the work and correct the mistake. Show your work for full credit.
Francisco’s work:
Step 1: Slope of MN: 1/4
Step 2: Slope of the line perpendicular: 4
Step 3: y - y = m(x - x) Q(6, -2)
y - (- 2) = 4 (x - 6)
Step 4: y + 2 = 4x - 24
Step 5: y + 2 - 2 = 4x - 24 - 2
Step 6: y = 4x - 26
Step completed incorrectly: ___
(I believe the step completed incorrectly is 2? But I’m not very sure on the showing my work part as well.)
Answers
Answer:
Step completed incorrectly: 2
Correct Answer: y = -4x + 22
Step-by-step explanation:
The graph is a straight line through points M(4, -1) and N(8, 0). Point Q is located at (6, -2).
To calculate the slope of the line, substitute the points into the slope formula:
\(\textsf{Slope $(m)$}=\dfrac{y_2-y_1}{x_2-x_1}=\dfrac{0-(-1)}{8-4}=\dfrac{1}{4}\)
Therefore, the slope of MN is 1/4, so step 1 of Francisco's calculations is correct.
If two lines are perpendicular to each other, the slopes of these lines are negative reciprocals. The negative reciprocal of a number is its negative inverse.
The negative reciprocal of 1/4 is -4.
Therefore, the slope of the perpendicular line is -4.
So Francisco has made an error in his calculation in step 2 by not making the perpendicular slope negative.
Corrected work
\(\textsf{Step 1:} \quad \sf slope\;of\;MN:\; \dfrac{1}{4}\)
\(\textsf{Step 2:} \quad \sf slope\;of\;the\;line\;perpendicular:\; -4\)
\(\begin{aligned}\textsf{Step 3:} \quad y-y_1&=m(x-x_1)\;\; \sf Q(6,-2)\\y-(-2)&=-4(x-6)\end{aligned}\)
\(\textsf{Step 4:} \quad y+2=-4x+24\)
\(\textsf{Step 5:} \quad y+2-2=-4x+24-2\)
\(\textsf{Step 6:} \quad y=-4x+22\)
Therefore, step 2 has been completed incorrectly.
The correct answer is y = -4x + 22.

A multiple choice quiz has 7 of questions each with 4 choices. Eric guesses on every question. What is the probability that he gets fewer than 5 questions correct?
Answers
Answer:
the probability that Eric gets fewer than 5 questions correct is approximately 0.857, or 85.7%.
Step-by-step explanation:
Let X be the number of questions Eric gets correct. Since there are 7 questions and each has 4 choices, the probability of guessing any one question correctly is 1/4, and the probability of guessing any one question incorrectly is 3/4. Since Eric is guessing on every question, we can model X as a binomial random variable with n = 7 and p = 1/4.
To find the probability that Eric gets fewer than 5 questions correct, we need to calculate:
P(X < 5) = P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4)
Using the binomial probability mass function, we get:
P(X = k) = (n choose k) * p^k * (1 - p)^(n - k)
where (n choose k) is the binomial coefficient, which gives the number of ways to choose k items from a set of n items.
Plugging in n = 7 and p = 1/4, we get:
P(X = k) = (7 choose k) * (1/4)^k * (3/4)^(7 - k)
Therefore, we have:
P(X < 5) = P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4)
= (7 choose 0) * (1/4)^0 * (3/4)^7 + (7 choose 1) * (1/4)^1 * (3/4)^6
+ (7 choose 2) * (1/4)^2 * (3/4)^5 + (7 choose 3) * (1/4)^3 * (3/4)^4
+ (7 choose 4) * (1/4)^4 * (3/4)^3
≈ 0.857
Therefore, the probability that Eric gets fewer than 5 questions correct is approximately 0.857, or 85.7%.
The following data represent the number of grams of fat in breakfast meals offered at a local fast food restaurant. (a) Construct an ordered stem-and-leaf plot and (b) describe the shape of the distribution.
(a) Construct the ordered stem-and-leaf plot below.
0 A vertical line used to separate stems and leaves.
01234
(b) The distribution is _______
skewed right.
bell-shaped.
uniform.
skewed left.
Enter your answer in each of the answer boxes.
Answers
Answer:
The following are the answer to this question:
Step-by-step explanation:
In the question some data is missing, which is defined in the attached file please find it.
The table for point a:
plot for the Stem-and-leaf:
0 3 4 5 7 4
1 2 2 4 4 5 7 7 7
2 0 3 4 4 5 8 6
3 3 4 7 9 4
4 1
In point b:
Its distributed skewed is correct because in its points 1 is a tail on its right side.
please find the attached file.


Giving brainly
Sienna's height is 5 inches less than her brother's height.
Her brother's height is unknown.
Which expression shows Sienna's height? Use x for her brother's height.
O A.
B. x+ 5
C. X-5
D. 5x

Answers
1. Calculate the expected value of the number of cups of coffee Alex drinks each day. 00.200 cups 00.163 cups 00.976 cups 01.881 cups
Answers
The expected value of the number of cups of coffee Alex drinks each day is 00.805 cups.
the mean/ average is gotten by dividing the sum of the values in the set by their number.
The concept of mean/average is used in this ques to calculate the expected value of the number of cups of coffee Alex drinks each day.
Mean/ Average= sum of values
number of values
Substituting the values into the equation we have:Mean/ Average= 00.200 cups +00.163 cups+ 00.976 cups+ 01.881 cups
4
Mean/ Average= 00.805 cups
Hence, The expected value of the number of cups of coffee Alex drinks each day is 00.805 cups.
Learn more about mean/ average on
https://brainly.com/question/28145873
#SPJ1
A probability model includes p(red)=2/7 and P(blue)=3/14. Select all the probabilities that could complete the model.
Answer Key:
A: P(green)= 2/7, P(yellow)=2/7
B: P(green)=3/8, P(yellow)=1/8
C: P(green)=1/4 P(yellow)=1/4
D: P(green)= 5/21 Pyellow)= 11/21 E. P(green)= 3/7 P(yellow)=1/14
Answers
The probabilities that could complete the model and maintain a total sum of 1 are options B and E.
B: P(green) = 3/8, P(yellow) = 1/8
E: P(green) = 3/7, P(yellow) = 1/14
To determine the probabilities that could complete the model, we need to consider the sum of all probabilities. In a probability model, the sum of all probabilities should equal 1.
Given the probabilities P(red) = 2/7 and P(blue) = 3/14, let's calculate the sum:
P(red) + P(blue) = 2/7 + 3/14 = 4/14 + 3/14 = 7/14 = 1/2
Since the sum of P(red) and P(blue) is 1/2, we need to find the probabilities for P(green) and P(yellow) that, when added to 1/2, give a total sum of 1.
Looking at the answer key options:
A: P(green) = 2/7, P(yellow) = 2/7
B: P(green) = 3/8, P(yellow) = 1/8
C: P(green) = 1/4, P(yellow) = 1/4
D: P(green) = 5/21, P(yellow) = 11/21
E: P(green) = 3/7, P(yellow) = 1/14
Let's check the sums:
A: P(red) + P(blue) + P(green) + P(yellow) = 2/7 + 3/14 + 2/7 + 2/7 = 4/14 + 3/14 + 4/14 + 4/14 = 15/14 ≠ 1 (incorrect)
B: P(red) + P(blue) + P(green) + P(yellow) = 2/7 + 3/14 + 3/8 + 1/8 = 4/14 + 3/14 + 6/14 + 1/14 = 14/14 = 1 (correct)
C: P(red) + P(blue) + P(green) + P(yellow) = 2/7 + 3/14 + 1/4 + 1/4 = 4/14 + 3/14 + 7/14 + 7/14 = 21/14 ≠ 1 (incorrect)
D: P(red) + P(blue) + P(green) + P(yellow) = 2/7 + 3/14 + 5/21 + 11/21 = 6/14 + 3/14 + 10/14 + 11/14 = 30/14 ≠ 1 (incorrect)
E: P(red) + P(blue) + P(green) + P(yellow) = 2/7 + 3/14 + 3/7 + 1/14 = 4/14 + 3/14 + 6/14 + 1/14 = 14/14 = 1 (correct)
Based on the calculations, the probabilities that could complete the model and maintain a total sum of 1 are options B and E. So, the correct answers are:
B: P(green) = 3/8, P(yellow) = 1/8
E: P(green) = 3/7, P(yellow) = 1/14
To learn more about the probability;
brainly.com/question/11234923
#SPJ1
Find the distance between the points (-5,1) and (-2,-5)
Answers
Answer:
d= 3√5
Step-by-step explanation:
Assume the random variable X has a binomial distribution with the given probability of obtaining a success. Find the following probability, given the number of trials and the probability of obtaining a success. Round your answer to four decimal places.
P(X<4), n=7, p=0.5
Answers
The probability P(X < 4) is approximately 0.2734 when X has a binomial distribution with n = 7 and p = 0.5.
When X has a binomial distribution with n = 7 and p = 0.5, we can use the cumulative distribution function (CDF) or the formula for the probability mass function (PMF) of the binomial distribution to determine the probability P(X 4) by adding the probabilities for X = 0, 1, 2, and 3.
Binomial CDF usage:
P(X 4) = P(X 3) = binom.cdf (3, n = 7, p = 0.5) 0.2734
Binomial PMF usage:
P(X 4) = P(X = 0), P(X = 1), P(X = 2), and P(X = 3) = binom.pmf(0, n=7, p=0.5), binom.pmf(1, n=7, p=0.5), binom.pmf(2, n=7, p=0.5), and binom.pmf(3, n=7, p=0.5), and binom.pmf(3, n=7, p=0.5) = 0.2734.
Since X has a binomial distribution with n = 7 and p = 0.5, the chance P(X 4) is roughly equal to 0.2734.
Learn more about probability here:
https://brainly.com/question/30034780
#SPJ1
Write the equation of a line with a slope of 1 and a y-intercept of 3. Do not use spaces in your response. *
Answers
Answer:
Y=X+3
Step-by-step explanation:
4) Determine if the following situation is arithmetic or geometric. Then answer the question using the formula.Eric calls 4 people within one hour of his son's birth to pass along the news. They each pass along the news to 4 new people every hour and so on. Howmuch has the news multiplied by and how many people have heard the news in 6 hours?

Answers
it is a geometric situation and the formula is the following
\(P(t)=4^t\)so after 6 hours we get
\(P(6)=4^6=4096\)Someone help with this equation

Answers
The answer is:
g(x + 1) = 6x + 1
g(4x) = 24x -5
Work/explanation:
To evaluate, I plug in x + 1 into the function:
\(\sf{g(x)=6x-5}\)
\(\sf{g(x+1)=6(x+1)-5}\)
Simplify
\(\sf{g(x+1)=6x+6-5}\)
\(\sf{g(x+1)=6x+1}\)
------------------
Do the same thing with g(4x)
\(\sf{g(4x)=6(4x)-5}\)
\(\sf{g(4x)=24x-5}\)
Hence, these are the answers.
you estimate the mean sat score for a population of students with a 90% confidence interval. assume that the population standard deviation is 100 if you want the marinof error to be no more than 10 you will need a minimum sample size of approximately
a.1476
b. 38
c.100
d.271
Answers
Rounding up the result to the nearest integer, we get the minimum sample size required as 271. Correct option is D.
To estimate the mean SAT score for a population of students with a 90% confidence interval, we need to determine the minimum sample size required to achieve a maximum margin of error of 10, assuming that the population standard deviation is 100.
The formula to calculate the sample size required is:
n = [(zα/2 * σ) / E]^2
Where:
zα/2 is the z-value for the desired confidence level (90% confidence interval in this case) = 1.645
σ is the population standard deviation = 100
E is the maximum margin of error = 10
Substituting the values in the formula, we get:
n = [(1.645 * 100) / 10]^2 = 270.7225
Therefore, option (d) is the correct answer.
In summary, to estimate the mean SAT score for a population of students with a 90% confidence interval and a maximum margin of error of 10, we need a minimum sample size of approximately 271 students, assuming that the population standard deviation is 100.
To learn more about sample size click on,
https://brainly.com/question/30647695
#SPJ4
14Y - 7y = 35. solve for y
Answers
Answer:
y = 5
Step-by-step explanation:
\(14y-7y=35\\7y=35\\y=5\)
14 minus 7 is 7
7Y is equal to 35
divide both sides by 7 is equal to 5
The graph shows the speed of a car while it is
slowing down to a stop.
a) Using an appropriate triangle with two of its
vertices on the curve, estimate the distance
travelled by the car during this time.
b) Is your answer an underestimate or an
overestimate?
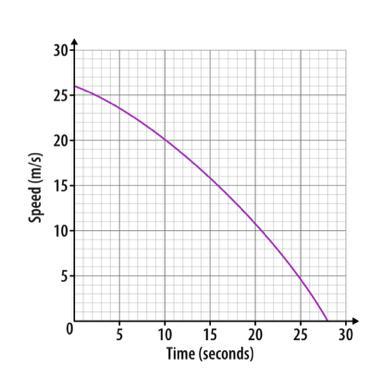
Answers
party trays, which cost $14.00 to make not including labor, are sold for $35.00. if two people work 8 hour shifts making the trays at $7.00 per hour, how many trays must be sold to cover all cost including labor?
Answers
Answer:
I think its $76
Step-by-step explanation:
please help https://brainly.com/question/20406553
Ibrahim owns a rectangular shaped property having a width of 1.9 miles and length of 3.6 miles. Once a week, Ibrahim walks around the perimeter of his property at a pace of 4.2 miles per hour. How many hours does it take Ibrahim to walk around the perimeter of his property once?
Answers
Answer:
about 2.62 hours
Step-by-step explanation:
add 1.9 and 3.6 twice
11
divide the parameter by 4.2
To compare freshmen’s knowledge of mathematics in two departments of a university, a certain professor in Mathematics got a sample of Education and Nursing students and gave a special examination. A sample of 25 Education students has a mean score of 88.50 with standard deviation of 7.5. A sample of 29 Nursing students have a mean score of 90.25 with a standard deviation of 8.2. Is there a significant difference between the two sample means? Use α = .01 level of significance.
Answers
There is no significant difference between the mean scores of Education and Nursing students in mathematics.
Is there a significant difference between the mean scores?Null hypothesis (H₀): There is no significant difference between the mean scores of Education and Nursing students in mathematics.
Alternative hypothesis (H₁): There is a significant difference between the mean scores of Education and Nursing students in mathematics.
We will use significance level (α) of 0.01.
Given:
Sample mean for Education students (x₁) = 88.50
Sample mean for Nursing students (x₂) = 90.25
Standard deviation for the Education student sample (s₁) = 7.5
Standard deviation for the Nursing student sample (s₂) = 8.2
Sample size for Education students (n₁) = 25
Sample size for Nursing students (n₂) = 29
The t-test statistic for two independent samples is:
t = (x1₁ - x2) / sqrt((s₁² / n₁) + (s₂² / n₂))
t = (88.50 - 90.25) / sqrt((7.5² / 25) + (8.2² / 29))
t ≈ -0.8187
In this case, df = 24.
The critical value for α = 0.01 and df = 24 is approximately ±2.797.
Since |-0.8187| < 2.797, the absolute value of the t-test statistic is smaller than the critical value.
Therefore, we fail to reject the null hypothesis.
Read more about mean scores
brainly.com/question/29432998
#SPJ1
I'm in need of some desperate help

Answers
Answer:
77/134 or 0.5746
Step-by-step explanation:
The approximate relative frequency is "the number of times that the event occurs during experimental trials, divided by the total number of trials conducted."
So we need to identify the number of times the event occured, the amount of times children chose sweet snacks, and the total number of trials conducted. So since the question wants the approximate relative frequency by column, we use the number 134 for the total number, and 77 for the number of times the event occurred. We then divide 77 by 134, which looks like this: 77/134. In decimal form, this equals 0.5746. It can't be simplified anymore in fraction form, so 77/135 would be another way to answer.
Shanice invests $4,668 in a savings account with a fixed annual interest rate of 2% compounded continuously. What will the account balance be after 9 years?
Answers
Answer:
$5588.61Step-by-step explanation:
Given:
Investment P = $4668Interest rate r = 2% or 0.02Compound number = continuousTime t = 9 yearsCalculate the balance:
\(A = Pe^{rt}\)\(A = 4668*e^{0.02*9}=4668*e^{0.18}=5588.61\)In this picture B and Fare
midpoints.
F
B
2x
с
88
'E
X=[ ? ]
Enter
![In this picture B and Faremidpoints.FB2x88'EX=[ ? ]Enter](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/8EF0kRGnGcu5WYWm0CUxEsUBOFLe7DkZ.png)
Answers
9514 1404 393
Answer:
x = 22
Step-by-step explanation:
The midsegment BF is half length of the base segment CE, so you have ...
2x = 88/2
x = 22 . . . . . divide by 2
I need help with question 8

Answers
Answer:
i think it is 11.6 not for sure
Step-by-step explanation:
Triangles WILL GIVE BRAINLIEST

Answers
Answer:
A
Step-by-step explanation:
A
Answer:
Q9 - Option 1---- A - 15.6 Square Units
Q10 --Option --- (B) RECTANGLE
Step-by-step explanation:
Q9 --
Analyze:we know area of triangle = 1/2 ab sin theta
Where theta is the angle included between sides A and side B
Calculate:A = 5.2
B = 7
theta = 121 degrees
Area:1/2 * 5.2 * 7 * sin 121 degrees = 15.6 Square Units
Conclusion
The area of the triangle is 15.6 Square Units
Q10 - The cross-section of a right cylinder which is perpendicular to its base is a RECTANGLEOption --- (B) RECTANGLE
Hope this helps!
Mean=3, find the probability that X is at most 3
Answers
Answer: honestly the only thing i know is that mean is another way of saying expected value
Step-by-step explanation:
please help me with my geometry i cant fail this class or i’ll have to move out of my parents house :(

Answers
Applying the tangent and the circle theorems, we have:
22. x ≈ 9.4; 23. Perimeter of triangle GHI = 168 units
24. m<NSR = 110°; 25. x = 10
How to Find the Missing Measures Using Circle and Tangent Theorems?22. Based on the tangent theorem, the triangle is a right triangle since the line is tangent to the radius of the circle. Therefore, based on the Pythagorean theorem, we have:
x = √[(7.4 + 4.6)² - 7.4²]
x ≈ 9.4
23. JH and HK are tangents, therefore they are equal. Thus:
7x + 4 = 13x - 20
7x - 13x = -4 - 20
-6x = -24
x = 4
Perimeter = GI + GJ + JH + HK + KI
GI = 52
HK = JH = 7x + 4 = 7(4) + 4 = 32
KI = 15
GJ = 52 - 15 = 37
Perimeter of triangle GHI = 52 + 37 + 32 + 32 + 15 = 168 units
24. Based on the angle of intersecting chords theorem, we have:
m<NSR = 1/2(170 + 50)
m<NSR = 110°
25. Based on the inscribed angle theorem, we have:
2(4x - 5) + 290 = 360
Solve for x:
8x - 10 + 290 = 360
8x = 360 - 280
8x = 80
x = 10
Learn more about the tangent theorems on:
https://brainly.com/question/28039126
#SPJ1
If y varies directly as x, and y is 18 when x is 5, which expression can be used to find the value of y when x is 11? (In fraction form)
Answers
The expression that can be used to find the value of y when x is 11 is y = (198/5) in fraction form.
If y varies directly as x, it means that y and x are proportional to each other. We can write this relationship as y = kx, where k is the constant of variation.
To find the value of y when x is 11, we need to determine the value of k. We can use the given information that when x is 5, y is 18.
Substituting these values into the equation, we get:
18 = k * 5
To find the value of k, divide both sides of the equation by 5:
k = 18/5
Now that we have the value of k, we can use the equation y = kx to find the value of y when x is 11.
Substituting x = 11 and k = 18/5 into the equation, we get:
y = (18/5) * 11
Simplifying the expression, we have:
y = (198/5)
Therefore, the expression that can be used to find the value of y when x is 11 is y = (198/5) in fraction form.
for such more question on fraction form
https://brainly.com/question/1622425
#SPJ8
where should the graph of the line y=2x - 2 intersect the y axis
Answers
The graph of the line y = 2x - 2 intersects the y-axis at the point (0, -2).
Calculating where the graph intersects the y-axisGiven that we have the following equation
y = 2x - 2
The equation of a straight line in the standard form is y = mx + c, where m is the slope of the line, and c is the y-intercept. The y-intercept is the point at which the line intersects the y-axis.
The equation of the line is y = 2x - 2. To find where the line intersects the y-axis, we need to set x = 0 and solve for y.
So, when x = 0, we get:
y = 2(0) - 2
y = -2
Therefore, the line intersects the y-axis at the point (0, -2).
Read more about linear relation at
https://brainly.com/question/30318449
#SPJ1
Please help me and read this carefully
A) The histogram includes data from a voluntary response sample which is not representative of the population.
b) The histogram is missing data from those that take the subway.
c) The histogram includes data from a random sample which produces a normal distribution.
d) The histogram includes mileage amounts from residents throughout New York City.

Answers
Answer:
if the subway is an auto mobile, then b , if not then c
Step-by-step explanation:
hope this helps, if not lets talk in the comments
BRAILNLEST PLZZZZZ
Find the value of -A-B + C, when A= -8, B = 6 and C = -2
-4, 0, 4, -2
Answers
Answer:
0Step-by-step explanation:
Put A = -8, B = 6 and C = -2 to the expression -A - B + C
-(-8) - 6 + (-2) = 8 - 6 - 2 = 0
Compare negative three and five tenths repeating and negative ten thirds
Answers
The decimal number -3.3 repeating is greater than the decimal number -3.5 repeating.
What is Algebra?Algebra is the study of abstract symbols, while logic is the manipulation of all those ideas.
The acronym PEMDAS stands for Parenthesis, Exponent, Multiplication, Division, Addition, and Subtraction. This approach is used to answer the problem correctly and completely.
The numbers are given below.
- 10/3 and \(- 3 {\dfrac{\bar{5}}{10}}\)
Simplify the expression, then we have
- 10/3 = -3.33....
\(- 3 {\dfrac{\bar{5}}{10}} = -3.\bar5 = -3.55....\)
The decimal number - 3.3 rehashing is more noteworthy than the decimal number - 3.5 rehashing.
More about the Algebra link is given below.
https://brainly.com/question/953809
#SPJ1
Mariette bought a 6 pack of donuts to share with her friends
at school. The donuts cost $18.00 all together.
What is the unit rate for the donuts in ?
donut
1
What is the unit rate for the donuts
Answers
Answer: The unite rate is $3 per pack.
Step-by-step explanation:
The unit rate will the the cost of one pack of donuts.
If 6 packs is equal to $18 dollars, then to find the unit rate we will divide 18 by 6.
18/6 = 3
This means one pack of of donut will cost $3.