cos5xcosx-sin5xsinx=??????????????????
Answers
Answer:
cos6x.
Step-by-step explanation:
We use the identity
cosAcosB - sinAsinB = cos(A+B)
so cos5xcosx - sin5xsinx = cos(5x + x)
= cos6x.
Related Questions
Dental insurance costs $130 annually. How much should you budget monthly for dental insurance? $30 $20 $18 $11. Tell me in the COMMENTS if the answer choices are wrong I don't know. If you know the answer then help is appreciated.
Answers
Answer:
11
Step-by-step explanation:
there are 12 months in a year
we want to equally split the 130 into 12 equal payments
130/12=10.833 or 11
Solve for x in the diagram below.
32°
80°
2x
Answers
Answer:
Assuming you're talking about the angles of a triangle, X would be \(34^{.}\)
Step-by-step explanation:
The sum of the angles in a triangle is 180, 180 - 32 - 80 = 68, \(\frac{68}{2}\) is 34, so X is equal to \(34^{.}\).
writing equations of lines parallel and perpendicular to a given line through a point
Answers
To find the equation of a line parallel or perpendicular to a given line through a point, determine the slope and substitute the point's coordinates into the slope-intercept form.
To find the equation of a line parallel or perpendicular to a given line through a specific point, follow these steps:
1. Determine the slope of the given line. If the given line is in the form y = mx + b, the slope (m) will be the coefficient of x.
2. Parallel Line: A parallel line will have the same slope as the given line. Using the slope-intercept form (y = mx + b), substitute the slope and the coordinates of the given point into the equation to find the new y-intercept (b). This will give you the equation of the parallel line.
3. Perpendicular Line: A perpendicular line will have a slope that is the negative reciprocal of the given line's slope. Calculate the negative reciprocal of the given slope, and again use the slope-intercept form to substitute the new slope and the coordinates of the given point. Solve for the new y-intercept (b) to obtain the equation of the perpendicular line.
Remember that the final equations will be in the form y = mx + b, where m is the slope and b is the y-intercept.Therefore, To find the equation of a line parallel or perpendicular to a given line through a point, determine the slope and substitute the point's coordinates into the slope-intercept form.
To learn more about intercept click here
brainly.com/question/29142047
#SPJ11
Which is a terminating decimal?

Answers
Answer:
0.125
Step-by-step explanation:
It has not been bar notated unlike all of the other ones. Bar notation is used for repeating decimals. So therefore if it has not been bar notated it is not a repeating decimal, it is a terminating decimal. Meaning it ends at the last digit and does not go any further.
Hope this helps!
POSSIBLE POINTS 16A student owns a snowball stand and wants to calculate the profit (if the student has even earned a profit). The problem is that the student's little brotherkeeps giving snowballs away to his friends. Every time he does this, the student loses money.Part A: Each snowball costs the student $0.30 to make and he sells them for $1.25 each. What is the profit per snowball?Part B: Last week, the little brother gave away 12 snowballs. Assuming the student would have sold these 12 snowballs, and using your answerfrom part A, calculate how much money the student lost in profits. Show your work.
Answers
Part A:
Each snowball costs $0.30 to make and is sold for $1.25 each.
The profit per snowball is:
P = $1.25 - $0.30 = $0.95
The profit is $0.95 per snowball
Part B:
Little brother gave away 12 snowballs. The student lost the profit of those 12 snowballs:
12 x $0.95 = $11.40
The student lost $11.40 in profits
Note: The student also lost the $0.30 for the cost to make each snowball, but it's not accounted for.
PLEASE HELP ASAP! WILL GOVE BRAINLIEST!!! I NEED THIS DONE PLEASE FOLLOW DIRECTIONS!

Answers
Answer:
I'm not sure but
Step-by-step explanation: The area is 9.5 feet.
Which of the expressions are equivalent to the one below? Check all that
apply.
(1 + 3) = 4
A. (3+1) = 4
B. 1. (3 + 4)
C. (3 + 4). (1+4)
D. (1 + 4) = 3

Answers
Answer:
A
Step-by-step explanation:
The answer to the first expression is 1.
A =1
B= 7
C=28
D=5/3
For the function f(x) = 4x – 6, find x if f(x) = 22
Answers
4x = 22 + 6
4x = 28
X = 7
Answer:
x=7
Step-by-step explanation:
What is the value of x in the equation 1.5(x 4) – 3 = 4.5(x – 2)?
Answers
Answer:
The value of x in the equation 1.5(x + 4)-3 = 4.5 (x-2) is 4.
Step-by-step explanation:
1.5( x + 4)-3 = 4.5 (x - 2)
Using the distributive property muntiply the term in the equation.
1.5x + 1.5×4-3 = 4x - 4.5 × 2
1.5x + 6-3 = 4.5x - 9
isolate the variable x using transporting method and solve for x
4.5x - 1.5x = 6 - 3 + 9
3x = 12
therefore, x = 12/3 = 4
Hope This Helps You!!!
Let C be the event that a student received a grade of B or better in Calculus I and let S be event that a student received a grade of A in Statistics I. Which of the following denotes the probability that a student received an A in statistics given that the student received less than a B grade in Calculus I.
a. P(S'|C')
b. P(CIS)
c. P(SIC)
d. P(SIC)
Answers
The probability that a student received an A in Statistics I given that the student received less than a B grade in Calculus I can be denoted as P(S|C'). The correct option is (a) P(S'|C').
To represent the probability that a student received an A in Statistics I given that the student received less than a B grade in Calculus I, we need to consider the complement of the events.
The complement of event C (grade of B or better in Calculus I) is C' (grade less than B in Calculus I). Similarly, the complement of event S (grade of A in Statistics I) is S' (grade other than A in Statistics I).
Therefore, the probability that a student received an A in Statistics I given that the student received less than a B grade in Calculus I is denoted as P(S|C'). This notation indicates the conditional probability of event S occurring given that event C' has occurred.
Among the given options, (a) P(S'|C') correctly represents the desired probability. Option (b) P(CIS) represents the joint probability of events C, I, and S, which is not relevant to the given question.
Option (c) P(SIC) represents the joint probability of events S, I, and C, which is also not relevant. Option (d) P(SIC) is a duplicate of option (c) and does not represent the desired probability.
To learn more about probability click here: brainly.com/question/32117953
#SPJ11
help me asap please i dont understand

Answers
Answer:
We have 2 rational solutions
0 irrational solutions
0 complex solutions
Step-by-step explanation:
a^2 + 8a + 12 = 0
Using the discriminant
b^2 -4ac where ax^2 + bx+ c
so a =1 b = 8 and c = 12
8^2 -4(1)*12
64 - 48
16
Since the discriminant is greater than 0, we have 2 real solutions
since we can take the square root of 16, we have rational solutions
We have 2 rational solutions
Since this is a quadratic equations, there are only 2 solutions so there are
0 irrational solutions
0 complex solutions
Answer:
2 Rational Solutions
0 Irrational Solutions
0 Complex Solutions
Step-by-step explanation:
The discriminant of the quadratic formula is the name given to the portion underneath the radical (or the square root)"
\(x = \frac{1}{2} (-b\frac{ + }{ - } \sqrt{ {b}^{2} - 4ac })\)
Discriminant = D = b²-4ac
If D is less than 0 you have two complex solutions.
If D is equal to 0 you'll have one real solution.
If D is bigger than 0 you'll get two real solutions.
So here we have:
a=1
b=8
c=12
Which means D=64-4(1)(12)=64-48=16>0
D is bigger than 0, so you'll have two real solutions. And since 16 is a perfect square, they'll both be rational numbers.
What does the 14th Amendment mean ?.
Answers
hotel pool a hotel owner is trying to calculate how many square feet of fabric he will need to make a pool covering for winter. if the pool is in the shape of a regular hexagon with a side-to-side length of 30 feet, how many square feet of fabric will the owner need to construct the cover? round to the nearest square foot.
Answers
The hotel owner needs approximately 2,248 square feet of fabric to make a pool covering for the winter for their regular hexagonal pool with a side-to-side length of 30 feet.
To calculate the area of the pool, we first need to find the apothem (the distance from the center of the hexagon to the midpoint of any side). For a regular hexagon, the apothem is equal to the side length times the square root of 3 divided by 2. So, the apothem of this hexagonal pool is:
apothem = 30 × √3/2 = 25.980762
The area of a regular hexagon is given by the formula:
area = 3 × √3/2 × apothem^2
Substituting the value of the apothem, we get:
area = 3 × √3/2 × 25.980762^2 = 2247.72
Rounding this to the nearest square foot, the hotel owner will need approximately 2,248 square feet of fabric to construct the cover for the pool.
To know more about hexagon pool:
https://brainly.com/question/23568234
#SPJ4
A box with a square base and open top must have a volume of 13,500 cm3. Find the dimensions of the box that minimize the amount of material used.
sides of base=___cm
height=____cm
Answers
The dimensions of the box that minimize the amount of material used are:
Sides of the base = 30 cm
Height = 15 cm
To minimize the amount of material used, we need to minimize the surface area. Let's express the surface area of the box in terms of s and h. The total surface area (A_total) is the sum of the area of the base (A_base) and the areas of the four sides (A_sides):
A_total = A_base + A_sides
= s² + 4sh
Now, let's express A_total in terms of a single variable. We can do this by substituting the volume equation, 13,500 = s²h, into the equation for A_total:
A_total = s² + 4sh
= s² + 4s(13,500/s²) [substituting h with 13,500/s²]
= s² + 54,000/s
To find the dimensions that minimize the amount of material used, we need to find the minimum value of A_total. To do this, we can take the derivative of A_total with respect to s, set it to zero, and solve for s.
dA_total/ds = 2s - 54,000/s²
Setting this derivative to zero and solving for s:
2s - 54,000/s² = 0
2s = 54,000/s²
2s³ = 54,000
s³ = 27,000
s = ∛27,000
s ≈ 30 cm
Now that we have the value of s, we can substitute it back into the volume equation to find the value of h:
13,500 = s²h
13,500 = (30 cm)²h
13,500 = 900h
h = 13,500/900
h = 15 cm
To know more about volume here
https://brainly.com/question/11168779
#SPJ4
I don't get it my teacher tried to help me but I still don't get it

Answers
Answer:
The mean would be 82
Step-by-step explanation:
To find the mean you add up all the numbers.
\(68 + 73 + 95 + 81 + 85 + 90 + 77 + 84 + 75 + 92 = 820\)
Then you divide that be the amount of numbers are given to you.
\(820 \div 10 = 82\)
And that is how you find the mean.
Sorry if it is wrong I'm tired.
Cho sells kakigori, a Japanese frozen dessert flavored with sweet syrup, at a street stand.
The scatter plot shows the daily high temperature and the number of servings of kakigori
Cho sells each day for 12 days. Based on a line of best fit for the data, about how many
servings of kakigori will Cho sell on a day when the high temperature is 29° Celsius?
Number of Servings Sold
115
110
105
100
95
90
85
80
75
70
65
60
55
50
0
18 19 20 21 22 23 24 25 26 27 28 29 30
Temperature (°C)
94
101
88
111
Answers
We can estimate that Cho will sell around 100 servings of kakigori on a day when the high temperature is 29° Celsius.
Based on the line of best fit for the data, we can estimate the number of servings of kakigori Cho will sell on a day when the high temperature is 29° Celsius.
From the scatter plot, we can see that the line of best fit is increasing as the temperature increases.
By estimating the value on the line of best fit for the temperature of 29° Celsius, we can approximate the number of servings sold. Based on the scatter plot, it appears that the number of servings sold is around 100 when the temperature is 29° Celsius.
Therefore, we can estimate that Cho will sell around 100 servings of kakigori on a day when the high temperature is 29° Celsius.
Learn more about Scatter Plot here:
https://brainly.com/question/29231735
#SPJ1
Find the sale price of the item. Round to two decimal places if necessary.
Original price: $259.21
Markdown: 70%
The sale price is $
Answers
Answer:
259.21/100 X 30 = 77.763
sale price = $77.76
The path of a fast moving particle traces a circle with equation (x-3)² + (y-4)² = 25. It starts at point (3,-1), moves counter clockwise, and passes the point (8,4) for the first time after traveling 7 microseconds. Where is the particle after traveling for 20 microseconds.
Answers
The particle is at the point (-1.112,3.909) after traveling for 20 microseconds.
We have been given a circle,
(x-3)²+(y-4)²=25
whose center is (3,4)
and with a radius of 5 units.
The particle starts at (3,-1) which is the 6 o'clock position in the circle, and ends at (8,4) after 7 microseconds which are at the 3 o'clock position in the circle.
So, the particle traveled 1/4 of the circumference of the circle in 7 microseconds
therefore according to the unitary method it will travel 5/7 of the circumference of the given circle.
so it will subtend 2π×5/7 of the circle from (3,-1)
from the polar form of the circle and the shifting of center of the circle we can conclude
that it's location will be
(5 × cos (10π/7) - 3 , 5 × sin (10π/7) - 4)
= (-1.112,3.909)
Learn more about the equation of a circle on
https://brainly.com/question/29288238?referrer=searchResults
#SPJ4

What is the 8-bit binary (two's-complement) representation of signed decimal integers -16?
Answers
The representation of did sign decimal integers in 8-bit binary (two's complement) -16 = 11110000.
The correct option is C.
What does an 8-bit number mean?A maximum of 256 values, or 0 through 255, can be represented by 8 bits. The total number of states that may be represented and the number of bits in a binary number are both listed in Table 5.1. G = gigabits; K = kilobits; M = megabits; 1,048,576 = gigabits; 1,0737,41,824 = kilobits.
Why does a byte consist of 8 bits?The most common number of bits in a byte is eight. A byte is a unit of digital information. The byte is indeed the smallest addressable unit of recollection in many computer architectures because historically, a single character of text was encoded in a computer using a byte's worth of bits.
To know more about 8-bit number visit:
https://brainly.com/question/13107546
#SPJ4
What is the 8-bit binary (two's-complement) representation of signed decimal integers -16?
A. 11111011
B. 11010110
C. 11110000
D. 10111000
r/20-5=(-4) Help please :)
Answers
Answer:
r=20
Step-by-step explanation:
r/20−5=−4
r/20=−4+5
r/20=1
r=1×20
r=20
Answer:
20
step by step
James has 14{,}500\text { g}14,500 g14, comma, 500, start a text, space, g, end text of sand in his sandbox. He brings home another 7{,}400\text { g}7,400 g7, comma, 400, start a text, space, g, end text of sand from the beach to add to his sandbox. How many kilograms of sand does James have in his sandbox now? \text{kg}kg
Answers
Question:
James has 14,500g of sand in his sandbox. He brings home another 7,400g of sand from the beach to add to his sandbox. How many kilograms of sand does James have in his sandbox now?
Answer:
The quantity of sandbox James has is 21.9kg
Step-by-step explanation:
Given
Initial quantity of sandbox = 14,500 g
Additional quantity = 7,400 g
Required
Total quantity of sandbox in kg
Tp get the total quantity of sandbox, we simply add in the initial and additional quantity of sandbox together;
This is represented mathematically as follows;
Total = Initial Quantity + Additional Quantity
Total = 14,500 g + 7,400 g
Total = 21,900 g
But the question says the answer should be in kg
1000 g is equivalent to 1 kg
21,900 g will be equivalent to \(\frac{21,900}{1,000} kg\)
\(21,900g = \frac{21,900}{1,000} kg\)
\(21,900g = 21.9 kg\)
Hence, the quantity of sandbox James has is 21.9kg
Jake scored 28 points in his basketball game by shooting only two-point and three-point shots. He scored a total of 13 baskets.
Write a system of equations to represent the number of each type of basket Jake scored.
Answers
The system of equations that represents the number of each type of basket Jake scored is given by:
x + y = 13
2x + 3y = 28
What is a system of equations?A system of equations is when two or more variables are related, and equations are built to find the values of each variable.
In this problem, the variables are:
Variable x: Number of two-point shots.Variable y: Number of three-point shots.Jake scored 28 points in his basketball game by shooting only two-point and three-point shots, hence:
2x + 3y = 28.
He scored a total of 13 baskets, hence:
x + y = 13.
More can be learned about a system of equations at https://brainly.com/question/24342899
Click the picture I need help please

Answers
Answer:
11
Step-by-step explanation:
x=2
5*2+1=10+1=11
Answer: g(2) = 11
Step-by-step explanation:
So, you the 2 as x for 5x + 1, and you get 10 + 1. Then you can get an 11 as your answer.
Kayla has 52 CDs in her music collection. Let c
represent the number of country music CDs in
Kayla's collection. Which expression can be used
to find the number of CDs that are not country
music?
A. c-52
B. 52+ c
C.
52
D. 52-c
Answers
The expression can be used to find the number of CDs that are not country music is 52-c. The correct option is D.
What is an expression?The mathematical expression combines numerical variables and operations denoted by addition, subtraction, multiplication, and division signs.
Mathematical symbols can be used to represent numbers (constants), variables, operations, functions, brackets, punctuation, and grouping. They can also denote the logical syntax's operation order and other properties.
Given that Kayla has 52 CDs in her music collection. Let c represent the number of country music CDs in Kayla's collection.
The expression can be used to find the number of CDs that are not country music is written as below:-
E = 52-c
The correct option is D for the required expression.
To know more about an expression follow
https://brainly.com/question/13882635
#SPJ1
the population of bacteria in a culture grows at a rate proportional to the number of bacteria present at time t. after 3 hours it is observed that 500 bacteria are present. after 10 hours 5000 bacteria are present. what was the initial number of bacteria? (round your answer to the nearest integer.)
Answers
The initial number of bacteria in the culture was approximately 116. We can solve this using differential equation.
Let N(t) be the number of bacteria present at time t, and let k be the constant of proportionality for the growth rate. Then the differential equation that describes the growth of bacteria is:
dN/dt = kN
We can solve this differential equation to get:
N(t) = N0e\(^({kt)}\)
Where N0 is the initial number of bacteria at time t=0.
Using the given information, we can set up a system of two equations:
500 = N0e\(^{(3k)}\)
5000 = N0e\(^{(10k)}\)
Dividing the second equation by the first equation, we get:
10 = e\(^{(7k)}\)
Taking the natural logarithm of both sides, we get:
ln(10) = 7k
Solving for k, we get:
k = ln(10)/7
Substituting this value of k into either of the two equations above, we can solve for N0:
500 = N0e\(^{(3ln(10)/7)}\)
N0 = 116 (rounded to the nearest integer)
Therefore, the initial number of bacteria in the culture was approximately 116.
To know more about differential equation click here
brainly.com/question/14620493
#SPJ11
There is money to send four of nine city council members to a conference in Honolulu. All want to go, so they decide to choose the members to go to the conference by a random process. How many different combinations of four council members can be selected from the nine who want to go to the conference
Answers
Answer:
126
Step-by-step explanation:
There are 9 city council members.
We have to choose 4 of them.
We have to use the combination as :
\($^9C_4$\)
where, 9 is the population size
4 is the sample size.
Therefore, the total number of possible samples without replacement is given as :
\($^9C_4=\frac{9!}{4!(9-4)!}$\)
\($=\frac{9!}{5! \ 4!}$\)
\($=\frac{9 \times 8 \times 7 \times 6}{4 \times 3 \times 2 \times 1}$\)
= 126
A cube has an edge of 3 feet. The edge is increasing at the rate of 2 feet per minute. Express the volume of the cube as a function of m, the number of minutes elapsed. Hint: Remember that the volume of a cube is the cube (third power) of the length of a side. V (m) = 3 feet?
Answers
The volume of the cube as a function of m, the number of minutes elapsed, is V(m) = 4m^3 + 18m^2 + 30m + 27.
The volume of a cube with an edge length of 3 feet is given by V = s^3, where s is the length of a side.
Since the edge is increasing at a rate of 2 feet per minute, the length of the side can be expressed as s = 3 + 2m, where m is the number of minutes elapsed.
Substituting this expression for s into the volume formula, we get:
V(m) = (3 + 2m)^3
Expanding this expression, we have:
V(m) = (3 + 2m)(3 + 2m)(3 + 2m)
= (9 + 6m + 6m + 4m^2)(3 + 2m)
= (9 + 12m + 4m^2)(3 + 2m)
= 27 + 18m + 12m + 8m^2 + 6m^2 + 4m^3
= 4m^3 + 18m^2 + 30m + 27
Therefore, the volume of the cube as a function of m, the number of minutes elapsed, is V(m) = 4m^3 + 18m^2 + 30m + 27.
Learn more about volume of the cube here:
https://brainly.com/question/29275443
#SPJ11
Can someone help please please

Answers
Answer:
c!
Step-by-step explanation:
Determine whether or not the function is one-to-one, and if it is, determine its inverse function
Answers
Determine the conditions for when a function has an inverse. Use the horizontal line test to recognize when a function is one-to-one. Find the inverse of a given function.
What is a horizontal line test?It is simple to establish whether the inverse of a function is also a function using the horizontal line test.
Because it fails the vertical line test, a function's inverse might not actually be a function.
Graph of the line
f\left( x \right) = - x + 2
f(x)=−x+2.
To know more about Horizontal line test, visit:
https://brainly.com/question/28208081
#SPJ4
please solve this!
slope of dotted line:
slope of reflection line:
equation of reflection line:
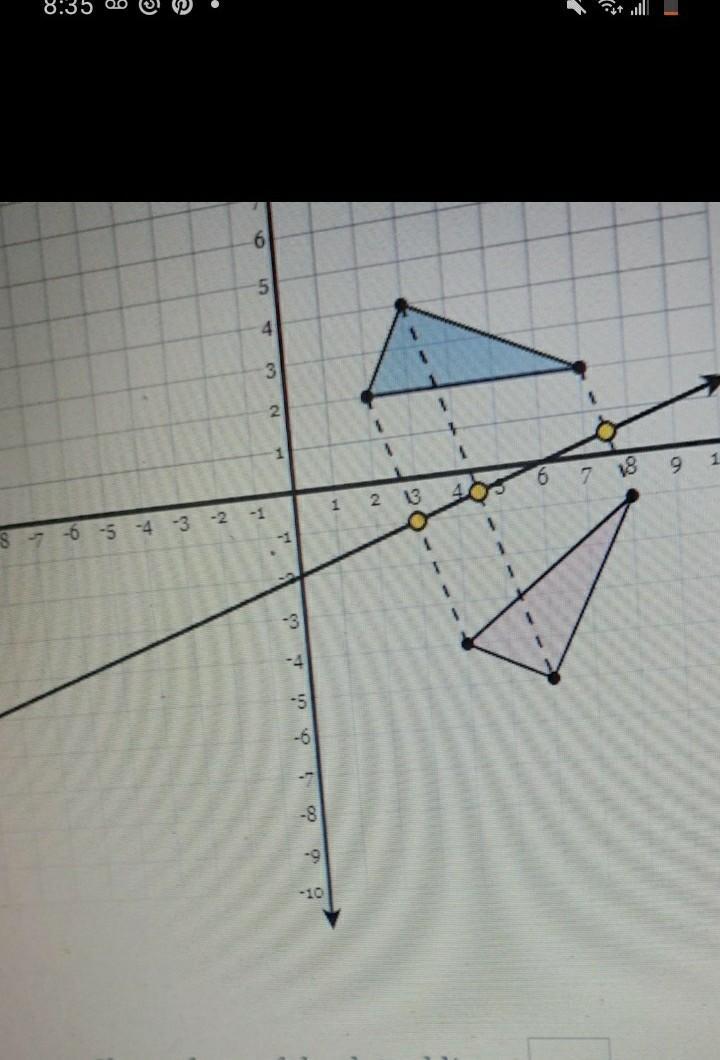
Answers
Answer:
I-
Step-by-step explanation:
) 5)8%2=80)4)88=5=5=88=9=5)5=
Answer:
Slope of dotted/dashed line: -3
Slope of Reflection line: 0.25
Equation of Reflection line: y = 0.25x - 2
Step-by-step explanation:
Slope of dotted line: rise/run. So 3/1 = 3. It is a negative slop because of the way it is facing ( \ ).
Slope of reflection line: rise/run. So 1/4 = 0.25. It is a positive slope ( / ).
Equation of reflection line: y = mx + b
m = slope
b = y-intercept
y = 0.25x - 2