determine the center and radius of
x²+y² = 9
Answers
Answer:
Center: ( 0 , 0 )
Radius: 3
Step-by-step explanation:
mark me brainleist plz
Related Questions
find x and y pls help
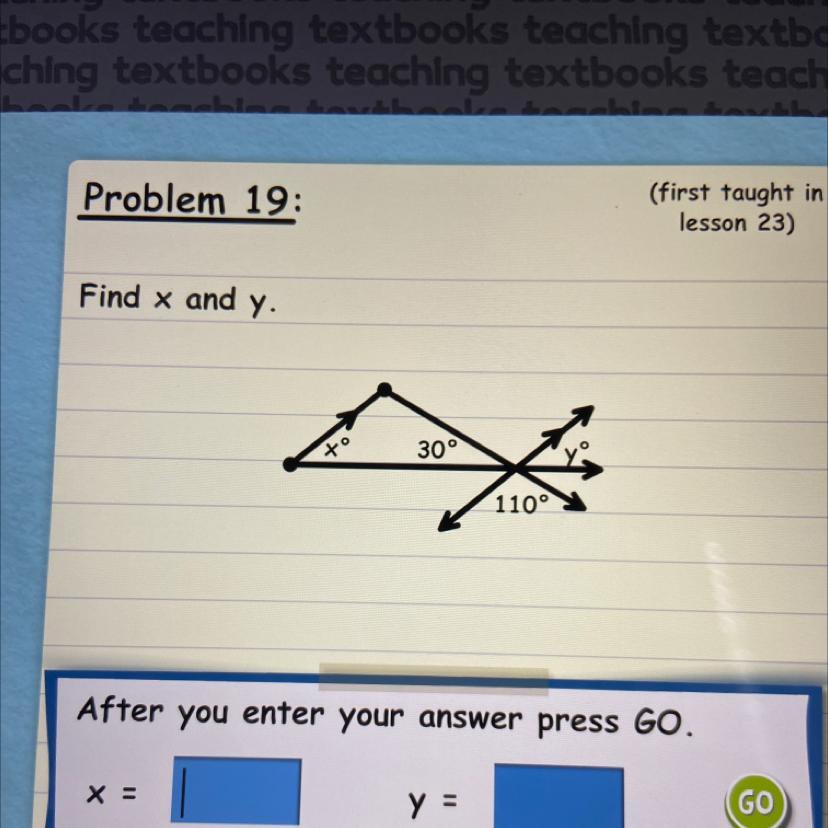
Answers
30 + 110 + 30 + 110 = 280
360-280 = 80
2y = 80
y = 40 degrees
If y is 40. Then the angle next to 30 is also y due to vertically opposite angles.
30 + 40 = 70
The line with 2 arrows is parallel to the line with x degrees. So, I use co-interior angles adds to 180.
In that c shaped, we got the bottom angle to be 70 & the top to be 110 because co-interior angles =180
Forget the c shape exists, and move onto the triangle that's visible. The top angle is 110 as found.
Angles in triangle add to 180.
180 - 110 - 30 = 40.
Thus, x = 40 degrees
There's a short cut I realise now because we can have alternate angles are equal; creating a z shape to find what x is equal to & that turns out to be the same value as y. ( due to vertically opposite angles)
Hope this helps!
The density in gold is 27.2 grams per cm. A gold gar in the shape of a rectengular prism, has a length of 23.5 and a width of 28 cm. The mass of the entire bar is 43,892.5 grams. What is the height of the bar?
Answers
Answer:2.381 cm.
Step-by-step explanation:
The volume of the gold bar can be calculated using the formula for the volume of a rectangular prism:
Volume = Length x Width x Height
We know the length and width of the bar, so we can substitute those values:
Volume = 23.5 cm x 28 cm x Height
We can then solve for the height by rearranging the equation:
Height = Volume / (Length x Width)
To find the volume, we can use the density of gold and the mass of the bar:
Density = Mass / Volume
Volume = Mass / Density
Substituting the values we have:
Volume = 43,892.5 g / 27.2 g/cm³
Volume = 1610.049 cm³
Now we can substitute the volume into the equation we derived earlier to solve for the height:
Height = 1610.049 cm³ / (23.5 cm x 28 cm)
Height = 2.381 cm
Therefore, the height of the gold bar is approximately 2.381 cm.
A prism and two nets are shown below: 3 in 5 In. 8.6 In. 4 In. Prism B A A D Not A Not B Part A: Which is the correct net for the prism? Explain your answer. (2 points) Part B: Write the measurements of Sides AB, BC, and CD of the correct net. (4 points) Part C: What is the surface area of the prism? Show your work. (4 points)

Answers
Answer:
Part A: The correct net is net A. It is because if you look at the 2, one trainable isn't aligned with the other, but it wouldn't fit together as a prism-like Net A would.
ca i get brainlies
Determine if the lines are parallel, perpendicular or neither: x = 5 and x = -2
Answers
In a standard deck of cards (NOT including jokers), there are:
4 suits (diamonds, hearts, clubs, spades)
13 number and face cards (Ace, 2, 3, 4, 5, 6, 7, 8, 9, 10, Jack, Queen, King)
2 colors (red, black)
Face cards are Jack, Queen, King
a) What is the probability of drawing a red or black card?
b) What is the probability of drawing a 7 of spades?
c) What is the probability of NOT drawing a Diamond?
d) What is the probability of drawing a black 2?
Answers
hmm im not sure, but I'm going to try to help you!! but I think it is B or D. Maybe but I'm not too sure. though. :((
Solve the equation mx" + Bx' = mg for r(t), given that you step off the bridge-no jumping, no diving! Stepping off means (0) = -100, x' (0) = 0, You may use mg = 160, B = 1, and g = 32. Use the solution from Problem 1 to compute the length of time t, that you freefall (the time it takes to go the natural length of the cord: 100 feet).
Answers
The general solution for mx" + Bx' = mg for r(t) is x(t) = 25e^(-x/5) + 5x -125
According to the question,
The given equation = mx" + Bx' = mg
Also , mg = 160, B = 1, and g = 32
=> m = 160/g
=> m = 160/32 = 5
Substituting the values in equation ,
5x" + x' = 160 , x(0) = -100, x' (0) = 0
The characteristic equation of the homogeneous differential equation is
5x" + x' = 0
=> 5r² + r = 0
=> r = 0 , -1/5
The complementary solution is x = c₁e⁰ + c₂e⁻⁰°²
Solution for complementary is yc = ax + b
Substitute yc = ax + b in 5x" + x' = 160 to get
5(0) + a = 160
=> a = 160
So the general solution is
x(t) = 25e^(-x/5) + 5x -125
To know more about Differential equation here
https://brainly.com/question/14620493
#SPJ4
Find the following limit. Limit of StartFraction StartRoot x + 2 EndRoot minus 3 Over x minus 7 EndFraction as x approaches 7 Which statements describe finding the limit shown? Check all that apply. Multiply by StartFraction StartRoot x + 2 EndRoot + 3 Over StartRoot x + 2 EndRoot + 3 EndFraction. Get x – 1 in the numerator. Get (x -7)(StartRoot x + 2 EndRoot minus 3) in the denominator. Divide out a common factor of x – 7. Calculate the limit as StartFraction 1 Over 6 EndFraction.
ANSWERS: A,D,E

Answers
Answer:
a,d,e
Step-by-step explanation:
In this question, we apply limit concepts to get the desired limit, finding that the correct options are: A, D and E, leading to the result of the limit being \(\frac{1}{6}\).
Limit:
The limit given is:
\(\lim_{x \rightarrow 7} \frac{\sqrt{x+2}-3}{x-7}\)
If we apply the usual thing, of just replacing x by 7, the denominator will be 0, so this is not possible.
When we have a term with roots, we rationalize it, multiplying both the denominator and the denominator by the conjugate.
Multiplication by the conjugate:
The term with the root is:
\(\sqrt{x+2} - 3\)
It's conjugate is:
\(\sqrt{x+2}+3\)
Multiplying numerator and denominator by the conjugate, meaning option A is correct:
\(\lim_{x \rightarrow 7} \frac{\sqrt{x+2}-3}{x-7} \times \frac{\sqrt{x+2}+3}{\sqrt{x+2}+3}\)
We do this because at the numerator we can apply:
\((a+b)(a-b) = a^2 - b^2\)
Thus
\(\lim_{x \rightarrow 7} \frac{\sqrt{x+2}-3}{x-7} \times \frac{\sqrt{x+2}+3}{\sqrt{x+2}+3} = \lim_{x \rightarrow 7} \frac{(\sqrt{x+2})^2 - 3^2}{(x-7)(\sqrt{x+2}+3)} = \lim_{x \rightarrow 7}\frac{x+2-9}{(x-7)(\sqrt{x+2}+3)} = \lim_{x \rightarrow 7}\frac{x-7}{(x-7)(\sqrt{x+2}+3)}\)
Thus, we can simplify the factors of x - 7, meaning that option D is correct, and we get:
\(\lim_{x \rightarrow 7} \frac{1}{\sqrt{x+2}+3}\)
Now, we just calculate the limit:
\(\lim_{x \rightarrow 7} \frac{1}{\sqrt{x+2}+3} = \frac{1}{\sqrt{7+2}+3} = \frac{1}{3+3} = \frac{1}{6}\)
Thus, option E is also correct.
Using a limit calculator, as given by the image below, we have that 1/6 is the correct answer.
For more on limits, you can check https://brainly.com/question/12207599

Find all points on the x-axis that are 16 units from the point (5,-8)
Answers
To find all points on the x-axis that are 16 units away from the point (5, -8), we can use the distance formula. The distance between two points (x₁, y₁) and (x₂, y₂) is given by the formula:
d = √((x₂ - x₁)² + (y₂ - y₁)²)
In this case, the y-coordinate of the point (5, -8) is -8, which lies on the x-axis. So, any point on the x-axis will have a y-coordinate of 0. Let's substitute the given values and solve for the x-coordinate.
d = √((x - 5)² + (0 - (-8))²)
Simplifying:
d = √((x - 5)² + 64)
Now, we want the distance d to be equal to 16 units. So, we set up the equation:
16 = √((x - 5)² + 64)
Squaring both sides of the equation to eliminate the square root:
16² = (x - 5)² + 64
256 = (x - 5)² + 64
Subtracting 64 from both sides:
192 = (x - 5)²
Taking the square root of both sides
√192 = x - 5
±√192 = x - 5
x = 5 ± √192
Therefore, the two points on the x-axis that are 16 units away from the point (5, -8) are:
Point 1: (5 + √192, 0)
Point 2: (5 - √192, 0)
In summary, the points on the x-axis that are 16 units away from the point (5, -8) are (5 + √192, 0) and (5 - √192, 0).
For more such questions on distance formula
https://brainly.com/question/28551043
#SPJ8
A 78.0 kg sprinter starts a race with an acceleration of 1.64 m/s2. If the sprinter accelerates at that rate for 25 m, and then maintains that velocity for the remainder of the 100 m dash, what will be his time (in s) for the race?
Answers
The sprinter will complete the race in approximately 17.07 seconds.
To calculate the time for the race, we need to consider two parts: the acceleration phase and the constant velocity phase.
Acceleration Phase:
The acceleration of the sprinter is 1.64 m/s², and the distance covered during this phase is 25 m. We can use the equation of motion to calculate the time taken during acceleration:
v = u + at
Here:
v = final velocity (which is the velocity at the end of the acceleration phase)
u = initial velocity (which is 0 since the sprinter starts from rest)
a = acceleration
t = time
Rearranging the equation, we have:
t = (v - u) / a
Since the sprinter starts from rest, the initial velocity (u) is 0. Therefore:
t = v / a
Plugging in the values, we get:
t = 25 m / 1.64 m/s²
Constant Velocity Phase:
Once the sprinter reaches the end of the acceleration phase, the velocity remains constant. The remaining distance to be covered is 100 m - 25 m = 75 m. We can calculate the time taken during this phase using the formula:
t = d / v
Here:
d = distance
v = velocity
Plugging in the values, we get:
t = 75 m / (v)
Since the velocity remains constant, we can use the final velocity from the acceleration phase.
Now, let's calculate the time for each phase and sum them up to get the total race time:
Acceleration Phase:
t1 = 25 m / 1.64 m/s²
Constant Velocity Phase:
t2 = 75 m / v
Total race time:
Total time = t1 + t2
Let's calculate the values:
t1 = 25 m / 1.64 m/s² = 15.24 s (rounded to two decimal places)
Now, we need to calculate the final velocity (v) at the end of the acceleration phase. We can use the formula:
v = u + at
Here:
u = initial velocity (0 m/s)
a = acceleration (1.64 m/s²)
t = time (25 m)
Plugging in the values, we get:
v = 0 m/s + (1.64 m/s²)(25 m) = 41 m/s
Now, let's calculate the time for the constant velocity phase:
t2 = 75 m / 41 m/s ≈ 1.83 s (rounded to two decimal places)
Finally, let's calculate the total race time:
Total time = t1 + t2 = 15.24 s + 1.83 s ≈ 17.07 s (rounded to two decimal places)
Therefore, the sprinter will complete the race in approximately 17.07 seconds.
For such more questions on Sprinter Race Time Calculation
https://brainly.com/question/18963548
#SPJ8
Which subset does 22 belong in
Answers
A large company event was held in a park on a hot day. Afterward, many of the employees got sick. Some blamed the potato salad. To investigate, a random sample of 20 sick employees and a random sample of 20 non-sick employees are selected. Each selected individual is asked if they ate the potato salad. The results are displayed in the table.
Management would like to know if there is convincing evidence that the distribution of responses about eating the potato salad differs for all employees who are sick versus those who are not sick. What are the appropriate hypotheses for this test?
H0: There is no difference in the distribution of responses among those who are and are not sick.
Ha: There is a difference in the distribution of responses among those who are and are not sick.
H0: There is a difference in the distribution of responses among those who are and are not sick.
Ha: There is no difference in the distribution of responses among those who are and are not sick.
H0: There is no difference in the distribution of responses for the 20 employees who are sick and the 20 employees who are not sick.
Ha: There is a difference in the distribution of responses for the 20 employees who are sick and the 20 employees who are not sick.
H0: There is a difference in the distribution of responses for the 20 employees who are sick and the 20 employees who are not sick.
Ha: There is no difference in the distribution of responses for the 20 employees who are sick and the 20 employees who are not sick.
Answers
The appropriate hypotheses for this test are: H0: There is no difference in the distribution of responses. Ha: There is a difference in the distribution of responses.
The chosen hypotheses are based on the objective of the investigation, which is to determine if there is convincing evidence that the distribution of responses about eating the potato salad differs between employees who are sick and those who are not sick.
The null hypothesis (H0) assumes that there is no difference in the distribution of responses about eating the potato salad between the sick and non-sick employees. This means that the proportion of employees who ate the potato salad would be the same in both groups, and any observed difference in the distribution of responses is due to random chance or factors unrelated to being sick or not sick.
On the other hand, the alternative hypothesis (Ha) proposes that there is a difference in the distribution of responses about eating the potato salad between the sick and non-sick employees. This suggests that the proportion of employees who ate the potato salad would be significantly different in the two groups, indicating a possible association between consuming the potato salad and getting sick.
By formulating these hypotheses, the investigation aims to evaluate whether the data provides convincing evidence to reject the null hypothesis in favor of the alternative hypothesis, indicating a meaningful relationship between eating the potato salad and falling sick.
Therefore, the correct answer is:
H0: There is no difference in the distribution of responses about eating the potato salad among those who are and are not sick.
Ha: There is a difference in the distribution of responses about eating the potato salad among those who are and are not sick.
For more such questions on hypotheses , Visit:
https://brainly.com/question/25263462
#SPJ11
In 2012, the population of a city was 6.51 million. The exponential growth rate was 2.75% per year. a) Find the exponential growth function. b) Estimate the population of the city in 2018. c) When will the population of the city be 10 million? d) Find the doubling time.
Answers
The exponential growth function is P = 6.51 (1.0275)^t. The population of the city in 2018 will be 7.66 million. The population of the city will be 10 million in 15.82258467 years and the doubling time is 25.55035862 years
How to find the exponential growth function?The exponential growth function is of the form P = P₀(1+r)^t
where P₀ is the current population, r is the growth rate and t is the time
Given: P₀ = 6.51 million, r = 2.75% per year
a) The exponential growth function
P = 6.51 (1+0.0275)^t
P = 6.51 (1.0275)^t
b) The population of the city in 2018
t = 2018-2012 = 6 years
P = 6.51 (1.0275)^t
P = 6.51 (1.0275)⁶ = 7.66 million
c) When the population of the city will be 10 million
P = 6.51 (1.0275)^t
10 = 6.51 (1.0275)^t (Solve for t)
(1.0275)^t =10/6.51
log (1.0275)^t = log(10/6.51)
t × log (1.0275) = log(10/6.51)
t = [log(10/6.51)] / [log (1.0275)]
t = 15.82258467 years
d) The doubling time
P = 6.51 (1.0275)^t will become:
2 = 1 (1.0275)^t
1.0275^t = 2
log(1.0275)^t = log 2
t log(1.0275) = log 2
t = log 2 / log 1.0275
t = 25.55035862 years
Learn more about population growth function on:
brainly.com/question/25896797
#SPJ1
tom had 14 kg of candy if he wanted to split the candy into four bags how much should it be in each bag between what what two whole numbers does your answer lie
Answers
The number of candies each bag would contain is given by the inequality relation 3 < A < 4 , where A is the number of candies
What is an Inequality Equation?Inequalities are the mathematical expressions in which both sides are not equal. In inequality, unlike in equations, we compare two values. The equal sign in between is replaced by less than (or less than or equal to), greater than (or greater than or equal to), or not equal to sign.
In an inequality, the two expressions are not necessarily equal which is indicated by the symbols: >, <, ≤ or ≥.
Given data ,
Let the inequality equation be represented as A
Now , the value of A is
Substituting the values in the equation , we get
Now , the total number of candies with Tom = 14 candies
The number of bags = 4 bags
So , the number of candies each bag = total number of candies with Tom / number of bags
On simplifying , we get
The number of candies each bag A = 14 / 4 = 3.5 candies
Now , the value 3.5 lies between the whole numbers 3 and 4
Hence , the inequality is 3 < A < 4
To learn more about inequality equations click :
https://brainly.com/question/11897796
#SPJ1
Hi, can you help me with this question, please, thank you:)

Answers
Looking at the scenario,
it is either a person chosen is in favor of the project or not.(Either success or failure)
The outcome is independent
Thus, we would apply binomial distribution. It is expressed as
P(x) = nCx * p^x * q^(n - x)
Where
x = number of successes
p = probability of success
q = probability of failure
n = number of trials
From the information given
p = 35% = 35/100 = 0.35
q = 1 - p = 1 - 0.35 = 0.65
n = 7
x = 5
We wan to find P(x = 5)
Thus,
P(x = 5) = 7C5 * 0.35^5 * 0.65^(7 - 5)
P(x = 5) = 7C5 * 0.35^5 * 0.65^2
P(x = 5) = 0.0466
Thus, the probability that exactly 5 of them favor the new project is 0.0466
42 divided by 7 get a decimal answer
Answers
Answer:
6
Step-by-step explanation:
just put 6.00 bro it divides evenly
Find the value of L that Will Maximize the profit Q=L²e^0.01L
Answers
The minimum profit occurs at L = 0, where Q = 0.
To find the value of L that maximizes the profit Q = L²\(e^{(0.01L)\).
We need to differentiate Q with respect to L and find the critical points where the derivative equals zero.
Then we can determine whether each critical point is a maximum or a minimum by examining the second derivative.
Testing for critical points:Q = L²\(e^{(0.01L)\)
Q' = \(2Le^{(0.01L)\) + \(0.01 L^2e^{(0.01L)\)
= 0(2L + 0.01L²) \(e^{(0.01L)\)
= 0L (critical point) or 200 \(e^{(0.01L)\)
= 0 (extraneous, ignore)
2L + 0.01L² = 0L(2 + 0.01L) = 0L = 0 or L = -200 (extraneous, ignore)
The only critical point is at L = 0.
Testing for maximum or minimum:Q'' = \(2e^{(0.01L)\) + 0.02Le^(0.01L) + 0.0001L²\(e^{(0.01L)Q''(0)\)
= \(2e^{(0)\) = 2Since Q''(0) > 0,
The critical point at L = 0 is a minimum.
Therefore, there is no value of L that maximizes the profit.
For more related questions on minimum profit:
https://brainly.com/question/32510275
#SPJ8
The values of L that will maximize the profit are 0 and -200
Finding the value of L that will maximize the profitFrom the question, we have the following parameters that can be used in our computation:
\(Q = L\²e^{0.01L\)
Differentiate the function
So, we have
\(Q' = \frac{L \cdot (L + 200) \cdot e^{0.01L}}{100}\)
Set the equation to 0
\(\frac{L \cdot (L + 200) \cdot e^{0.01L}}{100} = 0\)
Cross multiply
\(L \cdot (L + 200) \cdot e^{0.01L} =0\)
When expanded, we have
L = 0, L + 200 = 0 and \(e^{0.01L} =0\)
When solved for L, we have
L = 0 and L = -200
Hence, the values of L are 0 and -200
Read more about functions at
https://brainly.com/question/32616074
#SPJ1
The base of a prism has an area of 6.6 cm2. The height is 2.4 cm. What is the volume
Answers
So the volume is 15.84 cm The formula for the volume of a prism is given by the product of the area of the base and height of the prism. Thus, the volume of a prism can be given as V = B × H where V is the volume, B base area, and H height of the prism.
Between which two numbers is Negative 1.5 located on a number line?
Answers
Answer:
-2 and -1
have a good day
Myrna is buying a new rug for her living room. It it spilt into 3 panels, as shown in the diagram. What is the area of the rug, in the square feet?

Answers
Answer:
The answer is 96 square feet because if you look at the first rectangle (the one on the top left), you should multiply 5 times 6 which equals 30. (The reason you would do this is because you want to find the area of all of the rectangles first and then add them together in order to get the total area of the rug.) Then, you would look at the second rectangle (the one on the right), and you would multiply 8 times 6 which is 48. Next, you would look at the last rectangle (the one on the lower left) and multiply 6 times 3 which is 18. Finally, you have to add 30+48+18 and then you would get your final answer which is 96 square feet.
A twelve-sided die with sides labeled 1 through 12 will be rolled once. Each number is equally likely to be rolled. What is the probability of rolling a number greater than 10?
Answers
ok so there’s 2 numbers greater then ten so if u role it once it would be 2/12 chance but if you roll it twice it will double becoming 4/12 or 1/3 chance! hope this helped
PLEASE HELP ITS URGENT I HAVE 3 MINS AND IM FAILING I REALLY NEED A A ASAP PLEASE HELP

Answers
Answer:
D
Step-by-step explanation:
show that 0.235555......can be expressed in the form
\( \frac{p}{q} \)
where p and q are integers and q =/= 0.
Answers
Answer and Step-by-step explanation:
Suppose x = 0.2355555... .
Multiply x by 100 and 1000:
100x = 23.5555....
1000x = 235.5555....
Let's subtract the first equation from the second:
1000x - 100x = 235.5555.... - 23.555.....
Because the decimal part for each goes on forever, we can simply cancel them out in our subtraction statement:
900x = 235 - 23 = 212
Divide both sides by 900:
x = 212/900 = 53/225
Thus, since x = 0.235555..., we know that:
0.23555.... = 53/225
Since p = 53 and q = 225 are integers, we have proven that this repeating decimal can be written as a fraction.
~ an aesthetics lover
8 over 12 divide 7 over 8
Answers
Answer: 19/25
Step-by-step explanation: 8/12 / 7/8 is (rounded) 0.76, and 0.76 as a fraction is 19/25.
Joe makes tomato sauce to sell at a craft fair. He makes it in 5 gallon batches. How many pint jars does he need to hold 5 gallons of sauce?
Answers
By doing a change of units, we know that he will need 40 pint jars to hold the whole volume of sauce.
How many pint jars does he need?
We know that Joe makes tomato sauce to sell at a craft fair, and we know that he did make that sauce in 5-gallon batches.
We want to see how many jars do he need to hold these 5 gallons, so we need to perform a change of units.
Remember the relation:
1 gallon = 8 pints.
So, for each gallon, he will need 8 pint jars, then for the 5 gallons, he will need:
5*8 pint jars = 40 pint jars.
Learn more about changes of units:
https://brainly.com/question/1106482
#SPJ1
Add or Subtract to Simplify:
(x5 + x3) - (6x - x3 + 6x5)
Answers
Answer:
\( \boxed{ \bold{ \boxed{ \sf{ - 5 {x}^{5} + 2 {x}^{3} - 6x}}}}\)
Step-by-step explanation:
\( \sf{( {x}^{5} + {x}^{3} ) - (6x - {x}^{3} + 6 {x}^{5}) }\)
When there is a ( - ) in front of an expression in parentheses , change the sign of each term.
Also, remove the parentheses
⇒\( \sf{ {x}^{5} + {x}^{3} - 6x + {x}^{3} - 6 {x}^{5} }\)
Collect like terms
⇒\( \sf{ {x}^{5} - 6 {x}^{5} + {x}^{3} + {x}^{3} - 6x}\)
⇒\( \sf{ - 5 {x}^{5} + 2 {x}^{3} - 6x}\)
Hope I helped!
Best regards!!
Please help ASAP! Thank you

Answers
Answer:
a. lines intersecting at a single point
b. one solution
Step-by-step explanation:
a. The equations can be rewritten as:
y = -5x+23 and y = -1/6x+1
Comparing the equations with standard equation: y=mx+c, where m is the gradient of the line formed by the linear equation.
Since the gradient of the lines are different then the lines cannot be parallel and will intersent at one point.
b. The equation will have one solution as below.
The equations can be rewritten as:
5x+y=23
5x+30y=30
Subtracting both equation will result in,
29y=7
=> y=7/29
Hence x= 660/29
what is the Y intercept of function g if g(x)=2f(x)+1? a. 0,3 b.0,2 c 0,1 d 0,-1

Answers
Answer:
d
Step-by-step explanation:
The y- intercept is where the graph crosses the y- axis
The graph crosses the y- axis at (0, 1 ) ← y- intercept
Volume
5
Betty purchased a fish tank. The length, width, and height of the fish tank are shown below.
SEA
1 Ft
2 ft
Picture not drawn to scale
What is the volume of the fish tank?
OA
105 cu ft
Answers
Answer:
be brief can't see any picture
Answer:
10 5/16
Step-by-step explanation:
HELP PLEASE : )
Simplify the expression:
6/3(13−18)+2^4÷6
Answers
Answer:
-7.33
Step-by-step explanation:
Answer:
-7.333
Step-by-step explanation:
To solve this you have to use B O D M A S theorem and we have to solve the given expression according to the theorem.
That is,
B ⇒ Brackets
O ⇒ Of
D ⇒ Division
M ⇒ Multiplication
A ⇒ Addition
S ⇒ Subtraction
Let us solve it now.
\(\frac{6}{3} (13-18)+2^{4}\) ÷ \(6\)
2 ( -5) + 16÷ 6
-10 + 2.667
-7.333
Solve the following system of equations a2+b2 ; 3a2 -2ab-b2
Answers
The system has an infinite number of solutions, but the only solution is (a, b) = (0, 0).
The given system of equations can be solved using the substitution method. We can begin by solving the first equation,\(a^2 + b^2\), for either a or b. Let's solve for a:
\(a^2 + b^2 = 0\)
\(a^2 + b^2 = 0\)
\(a^2 = -b^2\)
\(a = \pm\sqrt(-b^2)\)
We can substitute this expression for a into the second equation, \(3a^2 - 2ab - b^2 = 0\), and simplify:
\(3(\pm\sqrt(-b^2))^2 - 2(\pm\sqrt(-b^2))b - b^2 = 0\)
\(3b^2 - 2b^2 - b^2 = 0\)
0 = 0
Since 0 = 0, this means that the system of equations has an infinite number of solutions. In other words, any values of a and b that satisfy the equation \(a^2 + b^2 = 0\) will also satisfy the equation \(3a^2 - 2ab - b^2 = 0\)
However, the equation \(a^2 + b^2 = 0\) only has a single solution, which is a = b = 0. Therefore, the solution to the system of equations is (a, b) = (0, 0).
Learn more about substitution here:
brainly.com/question/22340165
#SPJ1