Determine the value of the angle.



Angle BDC=

The statement is true that 95% of all possible sample means will fall above 76.71.

We know that the sample mean can be calculated using the formula;

\($\bar{X}=\frac{\sum X}{n}$\).

Given that the mean is 80 and the variance is 400 for the population and the sample size is 100. The standard deviation of the population is given by the formula;

σ = √400

= 20.

The standard error of the mean can be calculated using the formula;

SE = σ/√n

= 20/10

= 2

Substituting the values in the formula to get the sampling distribution of the mean;

\($Z=\frac{\bar{X}-\mu}{SE}$\)

where \($\bar{X}$\) is the sample mean, μ is the population mean, and SE is the standard error of the mean.

The sampling distribution of the mean will have the mean equal to the population mean and standard deviation equal to the standard error of the mean.

Therefore,

\(Z=\frac{76.71-80}{2}\\=-1.645$.\)

The probability of the Z-value being less than -1.645 is 0.05. Since the Z-value is less than 0.05, we can conclude that 95% of all possible sample means will fall above 76.71.

Conclusion: Therefore, the statement is true that 95% of all possible sample means will fall above 76.71.

To know more about means visit

https://brainly.com/question/521227

#SPJ11

Answer:

32°

Step-by-step explanation:

Given:

∠DMQ = 58º

In this circle, the radius is DM. Since AD is tangent to the circle M, at point D, and the angle between a tangent and a radius is 90°

Therefore, ∠MDQ = 90°

The total angle in a triangle is 180°. Since we have the values of ∠MDQ and ∠DMQ, ∠DQM will be calculated as:

180 = ∠DMQ + ∠MDQ + ∠DQM

Solving for ∠DQM, we have:

∠DQM = 180 - ∠DMQ - ∠MDQ

∠DQM = 180 - 90 - 58

∠DQM = 32°

The measure of ∠DQM is 32°

the one wayyy on the top

Answer:

i think its the first option im not sure

Step-by-step explanation:

Answer:

1/6

Step-by-step explanation:

gradient= change in y/ change in x

-4--5/2--4= 1/6

1/6 is the gradient

The gradient of the line segment in its simplest form is: 1/6.

Given the following points, (-4,-5) and (2,-4) are on a line segment:

Gradient (m) = change in y / change in x

Gradient (m) = (-4 -(-5))/(2 -(-4))

Gradient (m) = 1/6

Therefore, the gradient of the line segment in its simplest form is: 1/6.

Learn more about gradient of a line segment on:

https://brainly.com/question/1413251

The slope of the equation is 25 and it represents a monthly membership charge and the y-intercept of the equation is 50 and it represents the charges of a one-time fee for a gym.

A gym charges a one-time fee of $50 and a monthly membership charge of $25 the total cost c of being a member of the gym is given by

c (t) = 50 + 25t

where c is the total cost you pay after being a member for t months.

The slope of the equation is 25 and it represents a monthly membership charge.

The y-intercept of the equation is 50 and it represents the charges of a one-time fee for a gym.

To learn more on slope intercept form click:

https://brainly.com/question/9682526

#SPJ1

Answer:

C

Step-by-step explanation:

I think because there is no space or a sign in-between the f and 5, which means you multiply.

Answer:

  11.4

Step-by-step explanation:

You want the distance between C(-5, 5) and D(4, -2).

The distance formula is ...

  d = √((x2 -x1)² +(y2 -y1)²)

For the given points, the distance is computed to be ...

  d = √((4 -(-5))² +(-2 -5)²) = √(81 +49) = √130

  d ≈ 11.4

The length of CD is about 11.4 units.

__

Additional comment

The distance formula is an application of the Pythagorean theorem. It computes the hypotenuse of a right triangle whose legs are the differences in x- and y-coordinates.

Answer:

8

Step-by-step explanation:

\(x^2-2x \\x=4\\(4)^2-2(4)\\16-8\\8\)

Answer:

80 mins

Step-by-step explanation:

80 mins from 8.25 will be 10.15

The frequency table for the data in this problem is given as follows:

The frequency table is constructed considering the number of observations that are in each interval.

Then the observations in each interval are given as follows:

That is, each observation was attributed to one of the intervals of the frequency table, and then the table was constructed in which the frequencies are the number of observations in each interval.

More can be learned about frequency tables at https://brainly.com/question/16148316

#SPJ1

Answer:

x= 7

Step-by-step explanation:

\(\boxed{ \sinθ = \frac{opp}{hyp} }\)

\( \sin A = \frac{a}{c} \)

\( \frac{a}{c} = x + 2\)

\(\boxed{ \cosθ = \frac{adj}{hyp} }\)

\( \cos B = \frac{a}{c} \)

\( \frac{a}{c} = 2x - 5\)

Equating both expressions for a/c together:

x +2= 2x -5

Bring x terms to 1 side, constants to the other:

2x -x= 2 +5

x= 7

Answer:

X=7

Step-by-step explanation:

Hi, I took the quiz and the right answer is x=7.

The degrees of freedom associated with within treatments are 12 within the variation sum of squares degrees of freedom mean square f between treatments 64 within treatments (error) 96.

The ANOVA table divides the overall variance into variation between treatments and variation within treatments. Each source of variation's degrees of freedom (DF) is also provided.

According to the information provided, there are three treatments and a total of 15 observations. As a result, the total degrees of freedom is:

\(DF_{total}\) = n - 1

where n is the number of observations

\(DF_{total}\)= 15 - 1 = 14

The degrees of freedom between treatments are equal to the number of treatments minus one:

\(DF_{between}\) = k - 1

where k is the number of groups being compared

\(DF_{between}\)= 3 - 1 = 2

The number of treatments minus one equals the degrees of freedom between treatments, that is 2

We may use the following formula to calculate the degrees of freedom within treatments:

\(DF_{within}\) = \(DF_{total} - DF_{between}\)

Substituting the values we have:

\(DF_{within}\) = 14 - 2 = 12

\(DF_{within}\) = 12

As a result, the answer is 12. The degrees of freedom associated with within treatments are 12.

Learn more about ANOVA:

https://brainly.com/question/15084465

#SPJ4

Answer:

y = -1/2x +5

Step-by-step explanation:

The perpendicular slope will be the negative reciprocal of the original slope

the reciprocal of 2 is 1/2, and the negative of that is -1/2

Answer:

C

Step-by-step explanation:

The y-intercept is at x=0, y=3.

Answer:

asking if there are any questions

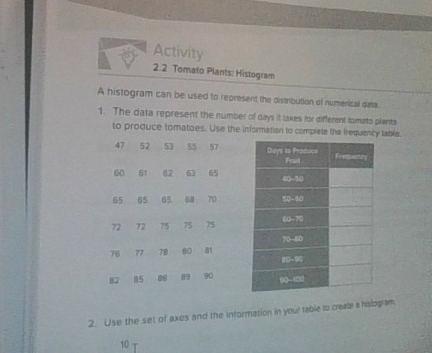
Answer:

Step-by-step explanation:

If sh starts reading at 3:25 and reads for 15 minutes, she is finished at 3:40. The hour hand is on the 3 and the minute hand is on the 8, so there is a difference of 25 minutes between the hour hand and the minute hand. Each 5-minute increment is 30 degrees (360 / 12) so 30 x 5 = 150 degrees. This is an obtuse angle. Not sure what kind of an answer you're looking for so I gave both the degree measure of the angle formed by the hour hand and the minute hand, and the classification of the angle.

Answer:

90 degrees, which is a right angle

Step-by-step explanation:

The time indicates when she starts reading is irrelevant. The only thing to notice is that she reeds for 15 minutes. Whenever you read for 15 minutes the angle that is formed by the hour and minute hands of the clock will always be the same, regardless of the starting time!

60 minutes comes down to 360 degrees.

1 minute = 60/60 and that comes down to 360/60 = 6 degrees.

15 minutes = 15 times 1 minute....

15 minutes comes down to 15* 6 = 90 degrees

90 degrees is a right angle

Answer:

x = 10

Step-by-step explanation:

64 + 23 + 60

=

147

Answer: 1. A translation takes a line to a parallel line or itself.

Step-by-step explanation:

I took the quiz myself.

When a line is translated, it means the line is moved from one position to another. The true statement is:

(1). A translation takes a line to a parallel line or itself.

In transformation, translation does not change the orientation of a line. This means that:

Point (2) above means that;

Using the above analysis, we can conclude that: option (1) is true

Read more about translations at:

https://brainly.com/question/12463306

Step-by-step explanation:

the x-intercepts are the points, where y=0.

x² - 6x + 5 = 0

with experience I see right away, this is true for x=1 and x=5.

but let's solve this formally.

the general solution to a quadratic excision is

x = (-b ± sqrt(b² - 4ac))/(2a)

in our case

a = 1

b = -6

c = 5

x = (6 ± sqrt((-6)² - 4×1×5))/2 = (6 ± sqrt(36-20))/2 =

= (6 ± sqrt(16))/2 = (6 ± 4)/2 = 3 ± 2

x1 = 3+2 = 5

x2 = 3-2 = 1

so, the 2 x-intercept points are

(1, 0) and (5, 0)

Answer:

549.5 cm³

Step-by-step explanation:

___________________________________________________________

FORMULA USED IN THE QUESTION :-

Volume of a cylinder whose height is 'h' and radius is 'r' = \(\pi \times r^2 \times h\)

___________________________________________________________

According to the question ,

Height of cylinder (h) = 7 cm

Radius of cylinder (r) = 5 cm

Volume of cylinder = \(\pi \times 5^2 \times 7 = 3.14 \times 25 \times 7 = 549.5 \: cm^3\)

Answer:

A. √2 > 1

Step-by-step explanation:

A: The √2 is more than one but less than 2. It's approximated as 1.414. This answer choice is correct.

B: The √4 is 2, which is less than π. Pi is approximated as 3.141. Therefore, this answer choice is incorrect.

C: The √2 is less than pi but greater than one. It's approximated as 1.414. This answer choice is incorrect.

D: The √3 is less than 2 but greater than one. It's approximated as 1.732. Therefore, this is incorrect.

Therefore, the correct answer is A. √2 > 1.

The output obtained after executing the java code snippet,

public static void main(string[] args)

{

int value = 3;

value++;

system.out.println(value);
}

will be 4.

As per the question statement, we are provided with a java code snippet, which goes as:

public static void main(string[] args)

{

int value = 3;

value++;

system.out.println(value);
}

We are required to determine the output, that we will obtain on executing the above mentioned code.

That is, on executing the code

public static void main(string[] args)

{

int value = 3;

value++;

system.out.println(value);
}

We will obtain an output of 4, as "++" is the post increment function.

To learn more about Java Code snippets and their Outputs, click on the link below

https://brainly.com/question/28400793

#SPJ4

By applying the Gram-Schmidt process solutions are:

(a) The orthonormal basis ß for V is ß = {u₁, u₂, u₃} = {(1/√2, 0, 1/√2), (-1/2√3, √3/2, 1/2√3), (1/2√21, √21/2, √21/2)}.

(b) The Fourier coefficients of x relative to ß are c₁ = 1/√2 + 2 + √2, c₂ = √3, and c₃ = √21/2 + 1.

(a) Applying the Gram-Schmidt process to S = {(1,0,1), (0,1,1), (1,3,3)}:

First, let v₁ = (1,0,1).

Normalize v₁ to obtain u₁ = v₁ / ||v₁||:

u₁ = (1,0,1) / sqrt(1² + 0² + 1²) = (1,0,1) / sqrt(2).

Next, find v₂' by subtracting the projection of v₂ = (0,1,1) onto u₁:

v₂' = v₂ - projₙ(u₁, v₂),

where projₙ(u₁, v₂) = (v₂ · u₁)u₁,

and · denotes the dot product.

v₂' = v₂ - ((v₂ · u₁)u₁)

    = (0,1,1) - ((0 + 1 + 1) / 2)(1,0,1)

    = (0,1,1) - (1/2)(1,0,1)

    = (0,1,1) - (1/2,0,1/2)

    = (-1/2, 1, 1/2).

Normalize v₂' to obtain u₂ = v₂' / ||v₂'||:

u₂ = (-1/2, 1, 1/2) / sqrt((-1/2)² + 1² + (1/2)²)

   = (-1/2, 1, 1/2) / sqrt(3/2)

   = (-1/2√3, √3/2, 1/2√3).

Finally, find v₃' by subtracting the projection of v₃ = (1,3,3) onto u₁ and u₂:

v₃' = v₃ - projₙ(u₁, v₃) - projₙ(u₂, v₃).

projₙ(u₁, v₃) = (v₃ · u₁)u₁ = (1 + 3 + 3) / 2 * (1,0,1) = (7/2, 0, 7/2).

projₙ(u₂, v₃) = (v₃ · u₂)u₂ = (1 + 3 + 3) / 3 * (-1/2, 1, 1/2) = (-1/2, 1, 1/2).

v₃' = v₃ - projₙ(u₁, v₃) - projₙ(u₂, v₃)

    = (1,3,3) - (7/2, 0, 7/2) - (-1/2, 1, 1/2)

    = (1,3,3) - (7/2, 0, 7/2) + (1/2, -1, -1/2)

    = (1/2, 2, 2).

Normalize v₃' to obtain u₃ = v₃' / ||v₃'||:

u₃ = (1/2, 2, 2) / sqrt((1/2)² + 2² + 2²)

   = (1/2, 2, 2) / sqrt(21/2)

   = (1/2√(21/2), √(21/2), √(21

/2))

   = (1/2√21, √21/2, √21/2).

Now, let's compute the Fourier coefficients of x = (1, 1, 2) relative to ß.

The Fourier coefficients are given by the inner products of x with the elements of ß:

c₁ = (x, u₁) = (1, 1/√2) + (1, 0) + (2, 1/√2) = 1/√2 + 1 + 2/√2 = 1/√2 + 2 + √2.

c₂ = (x, u₂) = (1, -1/2√3) + (1, √3/2) + (2, 1/2√3) = -1/2√3 + √3/2 + 1/√3 = -1/2√3 + 3/2√3 + 1/√3 = √3.

c₃ = (x, u₃) = (1, 1/2√21) + (1, √21/2) + (2, √21/2) = 1/2√21 + √21/2 + 2√21/2 = 2√21/2 + √21/2 + 1/2√21 = √21/2 + 1.

To verify our result using Theorem 6.5, we can reconstruct x using the Fourier coefficients and the orthonormal basis ß:

x = c₁u₁ + c₂u₂ + c₃u₃.

Substituting the values, we have:

x = (1/√2 + 2 + √2)(1/√2, 0, 1/√2) + √3(-1/2√3, √3/2, 1/2√3) + (√21/2 + 1)(1/2√21, √21/2, √21/2).

Simplifying, we get:

x = (1/2 + 1 + 1/2, 0, 1/2 + 2 + 1/2) + (0, 1, 0) + (1/2 + 1/2, √21/2, √21/2)

 = (2, 1, 3) + (0, 1, 0) + (1, √21/2, √21/2)

 = (3, 2 + √21/2, 3 + √21/2).

We can see that x is indeed equal to the original vector (1, 1, 2).

To know more about the Gram-Schmidt, click here: brainly.com/question/32249875

#SPJ11

Answer: 16 meters

Step-by-step explanation:

1 cm         4cm

--------    =  ---------

4m             16m

(x^2 + 43x + 10) - (-10x^2 - 5x + 10) = 11x^2 + 48x

Given polynomial (x^2 + 43x + 10) and (-10x^2 - 5x + 10)

We have to subtract the given polynomials.

Consider  (x^2 + 43x + 10) and (-10x^2 - 5x + 10)

Subtract (1) - (2) , we have,

(x^2 + 43 + 10) - (-10x^2 - 5x + 10)

x^2 + 43x + 10 + 10x^2 - 5x + 10

x^2 + 10x^2 + 48x + 5x + 10 - 10

Add similar elements : 43x + 5x = 48x

= x^2 + 10x^2 + 48x + 10 - 10

Add similar elements : x^2 + 10x^2 =  11x^2

= 11x^2 + 48x + 10 - 10

Solving , we get,

= 11x^2 + 48x

Thus, (x^2 + 43 + 10) - (-10x^2 - 5x + 10) = 11x^2 + 48x

Answer:

9.52

Step-by-step explanation:

Answer: abrimos

Step-by-step explanation: That's what it is on my quiz.

In the sentence "Nosotros _____ la ventana", the correct verb form is "abrimos". The best answer is option D.

The verb "abrir" is a Spanish verb that means "to open", and the first person plural form of this verb is "abrimos". So the sentence "Nosotros abrimos la ventana" translates to "We open the window".

The other verb forms in the options ("compartimos", "abro", and "escribimos") do not fit the context of opening the window. "Compartimos" means "we share", "abro" means "I open" (first person singular), and "escribimos" means "we write". So these forms are not the correct form to complete the sentence.

To know more about Spanish verb visit:

https://brainly.com/question/14977504

#SPJ1