Does anybody know this ?

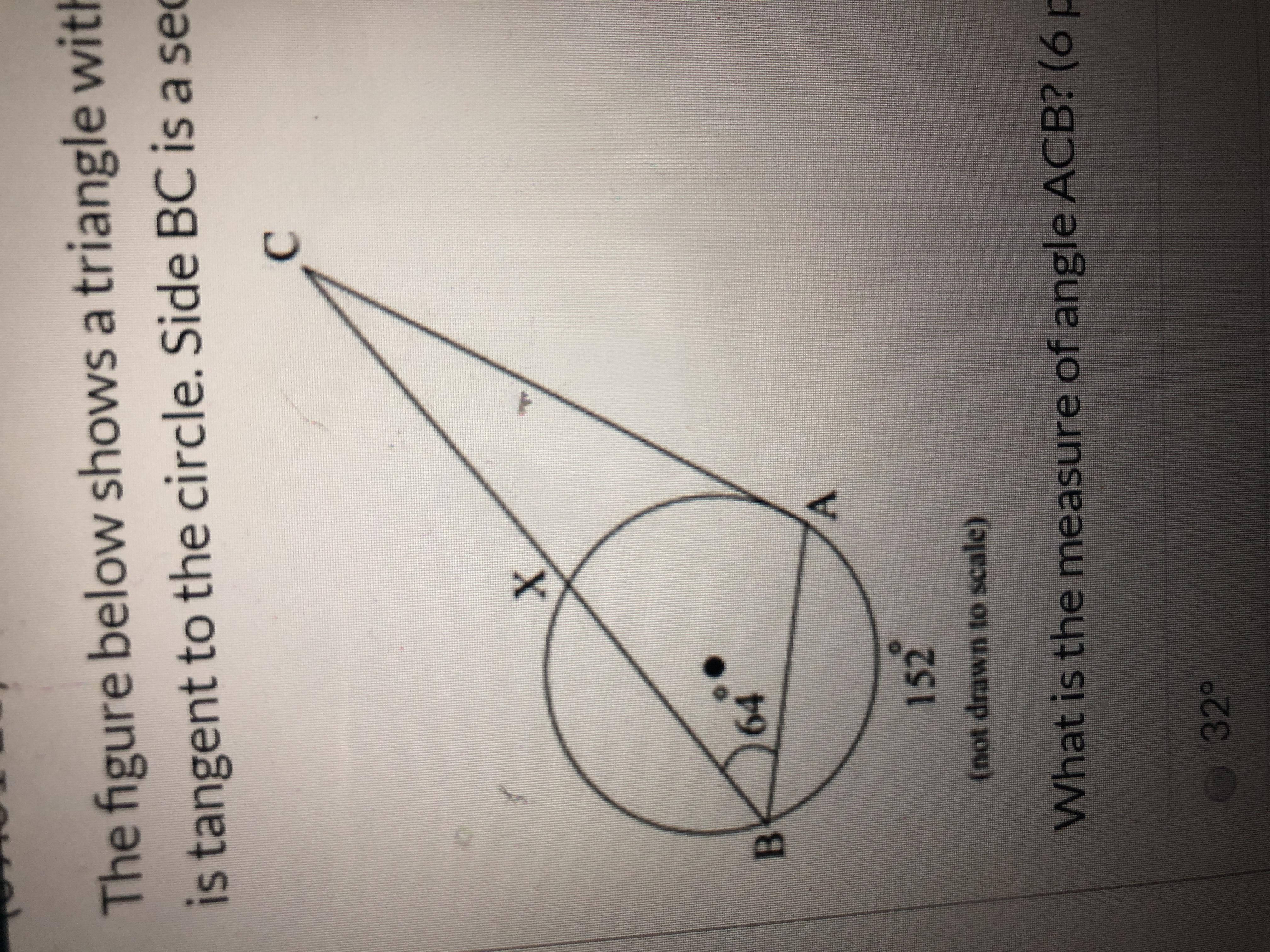
Answer:

Step-by-step explanation:

Confidence Level - "P" values  

99%  2.58

Confidence Interval - "P" values  

(0.5289 , 0.6097 )  

Reference=0.523 Reject H0

For any rational numbers r and s, 2r + 3s is rational. This is a corollary of Theorem 4.3.2.

Since r and s are rational numbers, they can be expressed as a ratio of integers:

r = a/b

s = c/d

where a, b, c, and d are integers and d and b are nonzero.

Then,

2r + 3s = 2(a/b) + 3(c/d)

= (2ad + 3bc)/(bd)

Since the product and sum of any two integers is also an integer, we know that 2ad and 3bc are integers. Thus, the numerator is the sum of two integers, which means it is also an integer. The denominator is the product of two nonzero integers, which means it is also nonzero. Therefore, 2r + 3s is a ratio of integers, and hence it is a rational number.

Therefore, for any rational numbers r and s, 2r + 3s is rational. This is a corollary of Theorem 4.3.2.

To know more about rational numbers refer to-

https://brainly.com/question/24540810

#SPJ11

The complementary solution for the given differential equation is:

**y_c(x) = c1e^x + c2e^(2x)**

(A) To find the complementary solution for the given differential equation **y'' - 3y' + 2y = e^(3x)(-1 + 2x + x^2)**, we need to solve the homogeneous version of the equation, which is obtained by setting the right-hand side to zero.

The homogeneous differential equation is: **y'' - 3y' + 2y = 0**

To solve this equation, we assume a solution of the form **y = e^(mx)**, where **m** is a constant.

Substituting this solution into the homogeneous equation, we get:

**m^2e^(mx) - 3me^(mx) + 2e^(mx) = 0**

Factoring out **e^(mx)**, we have:

**e^(mx)(m^2 - 3m + 2) = 0**

For this equation to hold true for all values of **x**, the factor **e^(mx)** must not be zero, so we focus on the expression inside the parentheses:

**m^2 - 3m + 2 = 0**

This is a quadratic equation that can be factored:

**m^2 - 3m + 2 = (m - 1)(m - 2) = 0**

Therefore, we have two possible values for **m**: **m = 1** and **m = 2**.

Hence, the complementary solution for the given differential equation is:

**y_c(x) = c1e^x + c2e^(2x)**

where **c1** and **c2** are arbitrary constants.

(B) You have not provided any specific instructions or questions regarding part (B) of your query. Please provide further details or specific questions related to part (B) so that I can assist you accordingly.

Learn more about differential equation here

https://brainly.com/question/1164377

#SPJ11

Answer:

by helping some one fast

Here, we are given two functions and we want to find the difference between the two functions;

Looking at what we have;

(f-g)x

What this mean is that we are to subtract g(x) from f(x);

Mathematically;

Solution:

Let the total number of company's employee be x;

Thus, 34.2% of the comapany's employee is 23,300. That is;

The total com

Answer:

Step-by-step explanation:

B

Answer:

2u-24

Step-by-step explanation:

Answer:2u-24

Step-by-step explanation:

There are a total of three terms in this expression.

Lets start by finding out the first two terms

-4(u+6)

-4*u= -4u

-4*6=-24

your first two terms are -4u and -24, which can be written as -4u-24.

The third term is 6u, and notice that 6u and -4u are like terms.

6u-4u=2u

And then we input -24 with 2u

2u-24

Answer:

y = 1

Step-by-step explanation:

The probability that a randomly selection of 20 toasters for testing, no more than one is defective is: 0.9401

To solve this exercise the formula and the procedure that we have to use for binomial distribution is:

Where:

Information about the problem:

Applying the binomial distribution formula, we get:

P(x) = n! /[x! *(n-x)!] * pˣ * (1 - p)ⁿ⁻ˣ

P(x) = 20! /[x! *(20-x)!] * 0.02ˣ * (1 - 0.02)²⁰⁻ˣ

Using the P formula to calculate the probability that no more than one of these toasters is defective, we have:

P = P(0) + P(1)

P(0) = 20! /[0! *(20-0)!] * 0.02⁰ * (1 - 0.02)²⁰⁻⁰

P(0) = 20! /(0!*20!) * 0.02⁰ * (0.98)²⁰

P(0) = 1/0! * 1 * 0.6676

P(0) = 1/1 * 1 * 0.6676

P(0) = 0.6676

P(1) = 20! /[1! *(20-1)!] * 0.02¹ * (1 - 0.02)²⁰⁻¹

P(1) = 20! /(1!*19!) * 0.02¹ * (0.98)¹⁹

P(1) = (20*19!) / (1!*19!) * 0.02¹ * (0.98)¹⁹

P(1) = 20/1! * 0.02 * 0.6812

P(1) = 20 * 0.02 * 0.6812

P(1) = 0.2725

P = P(0) + P(1)

P =  0.6676 + 0.2725

P =  0.9401

Probability is the function that measures the chances that an outcome of a random event will be as expected. In this way you can measure how likely it is that an event will happen.

Learn more about probability at: brainly.com/question/251701

#SPJ4

The margin of error for a 95% confidence interval for the population mean is approximately 2.57. So, correct option is A.

To find the margin of error for a 95% confidence interval for the population mean (μ), we can use the formula:

Margin of Error = Z * (σ / √n),

where Z is the z-score corresponding to the desired confidence level, σ is the population standard deviation, and n is the sample size.

For a 95% confidence interval, the corresponding z-score is approximately 1.96 (obtained from the standard normal distribution table).

Plugging in the values, we have:

Margin of Error = 1.96 * (8.5 / √42).

Calculating this expression, we find:

Margin of Error ≈ 2.57.

The correct answer is option a) 2.57.

To learn more about margin of error click on,

https://brainly.com/question/32499508

#SPJ4

The smallest value of n for which the remainder term is less than 0.001. This can vary depending on the specific series.

To find the minimum number of terms needed to ensure the sum of the infinite series has an error less than 0.001, we can use the concept of partial sums. By calculating the partial sums and comparing them with the desired error threshold, we can determine the minimum number of terms infinite series required.

Considering the given options, the minimum number of terms needed would be 1050 terms. With this many terms, the partial sum of the series would be accurate within the desired error range. Adding more terms would further improve the precision, but 1050 terms would be sufficient to achieve the specified level of accuracy.

Learn more about infinite series here:

https://brainly.com/question/29062598

#SPJ11

This will produce a plot of the vector field for the function f(x,y) = x^4, y^4.

The vector field for f(x,y) = x^4, y^4 can be plotted by considering the gradient of the function, which gives us the direction and magnitude of the vector at each point (x,y).

The gradient of f(x,y) is given by:

grad(f) = (∂f/∂x)i + (∂f/∂y)j

= 4x^3 i + 4y^3 j

This tells us that at each point (x,y), the vector has a magnitude of sqrt((4x^3)^2 + (4y^3)^2) = 4sqrt(x^6 + y^6) and is pointing in the direction of (4x^3, 4y^3).

To plot the vector field, we can choose a set of points (x,y) on a grid and calculate the corresponding vectors. We can then draw an arrow at each point (x,y) with the length and direction given by the corresponding vector.

Alternatively, we can use software such as MATLAB or Python with a suitable library like matplotlib to plot the vector field. Here is an example code in Python:

import numpy as np

import matplotlib.pyplot as plt

# Define the vector field function

def f(x, y):

   return np.array([x**4, y**4])

# Define the grid of points to plot

x = np.linspace(-1, 1, 20)

y = np.linspace(-1, 1, 20)

X, Y = np.meshgrid(x, y)

# Evaluate the vector field at each point

U, V = f(X, Y)

# Plot the vector field

plt.quiver(X, Y, U, V)

# Add labels and show the plot

plt.xlabel('x')

plt.ylabel('y')

plt.title('Vector Field for f(x,y) = x^4, y^4')

plt.show()

This will produce a plot of the vector field for the function f(x,y) = x^4, y^4.

Learn more about vector field here

https://brainly.com/question/31400700

#SPJ11

Answer:

The 9th grade science book has, on average, longer words and, by extension, more difficult material covered than the fifth grade science book.

The y intercept has been moved down, and the g(x) graph is less steep than the f(x) graph.

the fundamental duty of a family. Y = x or f(x) = x is the parent function for linear functions. Transformation: Modification of a figure's size or position. Child Function: The parent function is f(x) = x for all linear functions. y = x.

The simplest function in a family of functions that yet preserves the definition (or shape) of the entire family is known as a parent function in mathematics.

When the independent variable (often 'x') varies by a constant amount and the dependent variable (typically expressed by 'y') changes by a constant amount, the function is said to be linear.

Therefore, the correct answer is option C. The graph of g(x) is less steep than the graph of f(x) and the y intercept has been shifted down.

To learn more about linear parent function refer to:

https://brainly.com/question/12198807

#SPJ1

Answer:

The second answer, 7y - 4x =12 would make the system have no solution.

Answer:

38 and 28

Step-by-step explanation:

let the 2 numbers be a and b

(a - b)= 10.................................................1

1/6(a + b) = 11

hence (a + b) = 66...................................2

adding 1 and 2

a -b + a + b = 10 + 66

2a=76

a = 38

b=28

Answer:

A

Step-by-step explanation:

when dilating by a scale factor of 6, you are starting at the origin and just making it larger by a factor of 6, so you multiply the coordinates by 6 and get A

Answer:

1. M= 4

P= 12

2. g=3

k=3

3. b=40

Step-by-step explanation:

You relate the expression and look for the equivalent

Answer:

20/27

Step-by-step explanation:

There are six possible pairings that can be made from the four students: (Lubna, Kayla), (Lubna, Ibrahim), (Lubna, Talal), (Kayla, Ibrahim), (Kayla, Talal), and (Ibrahim, Talal). The probability of each selection can be determined based on the total number of possible pairings.

(a) To determine the probability of selecting at least one of the top two candidates, we count the number of pairings that include either Lubna or Kayla. There are three such pairings: (Lubna, Kayla), (Lubna, Ibrahim), and (Kayla, Ibrahim). The probability is 3 out of 6, or 1/2.

(b) To determine the probability of selecting both top two candidates, we count the number of pairings that include both Lubna and Kayla. There is only one such pairing: (Lubna, Kayla). The probability is 1 out of 6, or 1/6.

(c) To determine the probability of selecting neither of the top two candidates, we count the number of pairings that do not include Lubna or Kayla. There is only one such pairing: (Ibrahim, Talal). The probability is 1 out of 6, or 1/6.

(d) If we know that Lubna has been selected, the remaining pairing can only be (Lubna, Kayla). The probability of Kayla being selected in this case is 1 out of 1, or 1.

(e) If we know that Lubna has been selected, the remaining pairing can be (Lubna, Ibrahim) or (Lubna, Talal). The probability of either Talal or Ibrahim being selected in this case is 2 out of 2, or 1.

Learn more about probability here:

https://brainly.com/question/31828911

#SPJ11

The measurement of altitude BE is 4 unit.

As the average level of the sea's surface, sea level is used to measure altitude. A high altitude is defined as being significantly higher than sea level, such as Mount Everest. It is referred to as having a low altitude when something is closer to the ground, like a plane coming in to land.

As ABCD is rectangle

AD = BC = 12

ΔABC = ΔBCD

BE = FD

5² = 3²+BE²

AE = 3

BE = √(5²-3²)

BE = 4

Thus, The measurement of altitude BE is 4 unit.

To learn mote about altitude refer to :

https://brainly.com/question/18530806

#SPJ1

The most direct way to measure how dispersed a set of scores is, is by using the range. The range is calculated by subtracting the lowest score from the highest score in the dataset, which gives a quick and easy measure of the spread of the scores.

However, the range can be influenced by extreme scores and may not provide a complete picture of the variability. Other measures of variability, such as the standard deviation and variance, take into account the variability of all scores in the dataset and provide a more robust measure of variability.

To know more about range and standard deviation, visit:

https://brainly.com/question/30713935

#SPJ11

The generating function for the sequence {an} is given by a(x) = (1 + 2x) / (1 - x - 6x^2). It captures the terms of the sequence {an} as coefficients of the powers of x.

To find the generating function of the sequence {an}, we can use the properties of generating functions and solve the given recurrence relation.

The given recurrence relation is: an = an-1 + 6an-2

We are also given the initial conditions: a0 = 1 and a1 = 3.

To find the generating function, we define the generating function A(x) as:

a(x) = a0 + a1x + a2x² + a3x³ + ...

Multiplying the recurrence relation by x^n and summing over all values of n, we get:

∑(an × xⁿ) = ∑(an-1 × xⁿ) + 6∑(an-2 × xⁿ)

Now, let's express each summation in terms of the generating function a(x):

a(x) - a0 - a1x = x(A(x) - a0) + 6x²ᵃ⁽ˣ⁾

Simplifying and rearranging the terms, we have:

a(x)(1 - x - 6x²) = a0 + (a1 - a0)x

Using the given initial conditions, we have:

a(x)(1 - x - 6x²) = 1 + 2x

Now, we can solve for A(x) by dividing both sides by (1 - x - 6x^2²):

a(x) = (1 + 2x) / (1 - x - 6x²)

Therefore, the generating function for the given sequence is a(x) = (1 + 2x) / (1 - x - 6x²).

Read more on Functions here: https://brainly.com/question/29890699

#SPJ11

Answer:

\(1\frac{16}{25}\)

Step-by-step explanation:

So we have the decimal:

\(1.64\)

We can rewrite this as:

\(=1+0.64\)

0.64 is the same as 64/100. So:

\(=1+\frac{64}{100}\)

We can divide both layers by 4. This gives us:

\(=1+\frac{64/4}{100/4}\)

Divide:

\(=1+\frac{16}{25}\)

So, as a mixed fraction, this is:

\(1\frac{16}{25}\)

And we're done!

Answer:

1 16/25

Step-by-step explanation:

You write 1.64 as 1 64/100

Then you simplify it to 1 16/25

Answer:

the answer is 12 degrees

The measure of angle ACB when the secant BC and the tangent AC intersect is 12°

When a secant and a tangent meet outside the circle, thwe product of the length of the secant and its external segment equals the square of the length of the tangent segment.

Therefore,

arc XA = 2(64) = 128 degrees.

Hence,

m∠C = 1 / 2 (152 - 128)

m∠C = 1 / 2 (24)

m∠ACB = 12°

Therefore, angle ACB is 12 degrees.

learn more on secant and tangent here: https://brainly.com/question/27248564