DUE TODAY PLEASE HELP WELL WRITTEN ANSWERS ONLY!!!!!!
Here is the graph of a function describing the relationship between the height y, in feet, of the tip of a windmill blade and the angle of rotation Θ made by the blade. Describe the windmill.

Answers
The scale of the graph or the specific values of y and Θ, it is challenging to provide more detailed information about the windmill.
Based on the given graph, it can be observed that the windmill blade's height increases as the angle of rotation Θ increases. This suggests that the windmill is designed to harness wind energy by rotating its blade around a central axis.
The height of the tip of the blade is a crucial factor in determining the amount of energy produced, and the graph indicates that the blade is optimally positioned at a specific angle. It is difficult to give more specific information about the windmill without knowing more about the size of the graph or the precise values of y and x.
To learn more about : scale
https://brainly.com/question/25722260
#SPJ11
Note the full question is
DUE TODAY PLEASE HELP WELL WRITTEN ANSWERS ONLY!!!!!!
Here is the graph of a function describing the relationship between the height y, in feet, of the tip of a windmill blade and the angle of rotation Θ made by the blade. Describe the windmill.
Related Questions
I need help please!!!

Answers
hello help me please
8. 250°
9. 120° and 40°
10. 55°
11. 120° and 50°
12. 125° and 15°
13. 70° and 30°
14. 80°
15. 70°

Answers
Step-by-step explanation:
8.
the angle at a vertex (crossing point) outside of the circle (when both lines intersect with the circle arc, which is also the case for tangents) is half of the difference of both arc angles (the outer and the inner angles).
the outer angle is 250°. since the full circle is 360°, this makes the inner arc angle 360-250 = 110°.
the difference is 250-110 = 140.
and half of that difference is
140/2 = 70°.
so, x = 70°
9.
see 8.
the angle at the vertex outside the circle is half of the difference between outer and inner arc angle.
in fact, this is again about tangents (despite the picture), because the outer angle + the inner angle is again 360° (the full circle). this can only be, if the 2 lines are tangents splitting the outer and inner arc angles into 2 parts of 360° without remainder.
320 - 40 = 280
280/2 = 140
x = 140°
10.
see 8. and 9.
it is an extreme example, where the vertex of the 2 intersecting lines is exactly on the circle arc and the tangent point of the second line.
this makes the inner arc angle = 0°.
remember, the vertex angle (55°) is half of the difference of the outer (x) and inner (0°) arc angle.
55 = (x - 0)/2
110° = x
11.
remember the table question, where we found that the circle internal angle of 2 intersecting lines is half of the sum of both intersected arc angles ?
this is exactly this case again.
(120 + 50)/2 = 170/2 = 85°
x = 85°.
12.
first, like in 8. and 9., we determine the vertex angle. x is then the supplementary angle (together they have 180°) to this vertex angle, because the sum of all angles around one point on one side of a line is always 180°.
the vertex angle is
(125 - 15)/2 = 55°
x + 55 = 180
x = 125°.
13.
see 11.
the circle internal vertex angle is half of the sum of both circle arcs.
x = (70 + 30)/2 = 100/2 = 50°.
14.
again, an extreme case, where the vertex is on the circle arc. so, the second arc angle is 0°.
see also 12.
x is the supplementary angle of the vertex angle.
the vertex angle is
(80 + 0)/2 = 80/2 = 40°.
x + 40 = 180
x = 140°.
15.
it is difficult to read. I see the outer arc circle as 140°.
see 8., 9., 10.
the vertex angle x is
x = (140 - 70)/2 = 70/2 = 35°
do I sound like a broken record ? I at least feel that way. you should be able to apply the explanations from the previous questions to solve the new ones yourself.
what is not clear yet ? where do you need further explanations ?
I solve kinda a bit but its hard (Algebra 2)

Answers
Answer:
x=9
Step-by-step explanation:
The goal is to combine our knowledge of geometry area and algebra skills to solve this problems.
We know area of rectangle is
\(l \times w\)
where l is the length and w is the width. We know that one side is 3 less than the other side. We can use L or W but let use L. Let x replace L
One side is x, while the other side is
\(x - 3\)
Both multiply to 54.
\(x(x - 3) = 54\)
\( {x}^{2} - 3x = 54\)
\( {x}^{2} - 3x - 54 = 0\)
\((x - 9)(x + 6)\)
\(x = 9\)
or
\(x = - 6\)
Distance. is positive so x=9
If X is a geometric random variable for which we are counting the number of trials until the first success, what are the possible values of X?
A) 1, 2, 3, …
B) 0, 1, 2, 3, …
C) any positive or negative integer
D) any positive or negative number, even numbers that are not integers
Answers
Since the sum of the numbers selected must exceed 1, we know that n must be at least 1. Therefore, the expected value of X is finite and is equal to 2.
The expected value of the count of numbers that are selected can be found using the geometric distribution, since it models the number of trials until a success occurs.
Let X be the number of numbers that are selected until the sum of all numbers exceeds 1. Then X has a geometric distribution with parameter p, where p is the probability of success on each trial, i.e. the probability that the sum of all numbers selected does not exceed 1.
Since the numbers are selected uniformly over [0, 1], the probability of success on each trial is given by the area under the curve between 0 and 1 after the first number is selected.
On the first trial, the probability of success is 1. On the second trial, the probability of success is the area under the curve between 0 and (1 - the first number selected).
On the third trial, the probability of success is the area under the curve between 0 and (1 - the sum of the first two numbers selected), and so on.
The expected value of X can be found using the formula for the expected value of a geometric distribution:
E(X) = 1/p
Since the expected value of the sum of n independent and identically distributed uniform [0, 1] random variables is n/2, we can use this to find p:
E(X_1 + X_2 + ... + X_n) = n/2
where X_1, X_2, ..., X_n are the n numbers selected.
So, p = 1 / (1 + n/2).
Finally, we can substitute this into the formula for E(X) to find the expected value of X:
E(X) = 1 / (1 - n/2) = 2 / (2 - n)
For more questions on geometric distribution :
brainly.com/question/30478452
#SPJ1
Solve this please kkkkkkkkkkkkkkkkkkkkkkkkkkkkkkkkkkk

Answers
Answer:
\(\frac{12}{25}\) ↔ sinD × cosD
\(\frac{3}{5}\) ↔ sinC
\(\frac{16}{15}\) ↔ cosC × tanD
\(\frac{4}{5}\) ↔ sinD
Step-by-step explanation:
In the given triangle CBD
∵ ∠B is a right angle
∴ CD is the hypotenuse
→ We can use the trigonometry ratios
∵ sinC = opposite side of ∠C ÷ hypotenuse
∴ sinC = \(\frac{BD}{DC}\)
∵ BD = 3 and CD = 5
∴ sinC = \(\frac{3}{5}\)
∵ cosC = adjacent side of ∠C ÷ hypotenuse
∴ cosC = \(\frac{BC}{DC}\)
∵ BC = 4 and CD = 5
∴ cosC = \(\frac{4}{5}\)
∵ tanC = opposite side of ∠C ÷ adjacent side of ∠C
∴ tanC = \(\frac{BD}{BC}\)
∵ BD = 3 and BC = 4
∴ tanC = \(\frac{3}{4}\)
∵ sinD = opposite side of ∠D ÷ hypotenuse
∴ sinD = \(\frac{BC}{DC}\)
∵ BC = 4 and CD = 5
∴ sinD = \(\frac{4}{5}\)
∵ cosD = adjacent side of ∠D ÷ hypotenuse
∴ cosD = \(\frac{BD}{DC}\)
∵ BD = 3 and CD = 5
∴ cosD = \(\frac{3}{5}\)
∵ tanD = opposite side of ∠D ÷ adjacent side of ∠D
∴ tanD = \(\frac{BC}{BD}\)
∵ BD = 3 and BC = 4
∴ tanD = \(\frac{4}{3}\)
Let us find the answer to each tile
→ sinD = \(\frac{4}{5}\) ⇒ 4th answer
→ sinC = \(\frac{3}{5}\) ⇒ 2nd answer
→ sinD × cosD = ( \(\frac{4}{5}\)) × (\(\frac{3}{5}\)) = \(\frac{12}{25}\) ⇒ 1st answer
→ tanC × tanD = \(\frac{3}{4}\) × \(\frac{4}{3}\) = 1 ⇒ Not used
→ cosC × tanD = \(\frac{4}{5}\) × \(\frac{4}{3}\) = \(\frac{16}{15}\) ⇒ 3rd answer
Answer: ↔ sinD × cosD
↔ sinC
↔ cosC × tanD
↔ sinD
Step-by-step explanation:
In the given triangle CBD
∵ ∠B is a right angle
∴ CD is the hypotenuse
→ We can use the trigonometry ratios
∵ sinC = opposite side of ∠C ÷ hypotenuse
∴ sinC =
∵ BD = 3 and CD = 5
∴ sinC =
∵ cosC = adjacent side of ∠C ÷ hypotenuse
∴ cosC =
∵ BC = 4 and CD = 5
∴ cosC =
∵ tanC = opposite side of ∠C ÷ adjacent side of ∠C
∴ tanC =
∵ BD = 3 and BC = 4
∴ tanC =
∵ sinD = opposite side of ∠D ÷ hypotenuse
∴ sinD =
∵ BC = 4 and CD = 5
∴ sinD =
∵ cosD = adjacent side of ∠D ÷ hypotenuse
∴ cosD =
∵ BD = 3 and CD = 5
∴ cosD =
∵ tanD = opposite side of ∠D ÷ adjacent side of ∠D
∴ tanD =
∵ BD = 3 and BC = 4
∴ tanD =
Let us find the answer to each tile
→ sinD = ⇒ 4th answer
→ sinC = ⇒ 2nd answer
→ sinD × cosD = ( ) × () = ⇒ 1st answer
→ tanC × tanD = × = 1 ⇒ Not used
→ cosC × tanD = × = ⇒ 3rd answer
Step-by-step explanation:
I need help on this problem
-10.7 + (-19.26)
(I have to show my work btw)
Answers
Answer:
-29.96
Step-by-step explanation:
You add both numbers, as both are negative, and you get the answer.
1.5 is a repeating decimal. Write it as a fraction.
Answers
Answer:
1.5 is not a repeating decimal, and 1 1/2
Step-by-step explanation:
select the correct solutions for the system of equations:
10x+y=-20
y=2x^2-4x-16
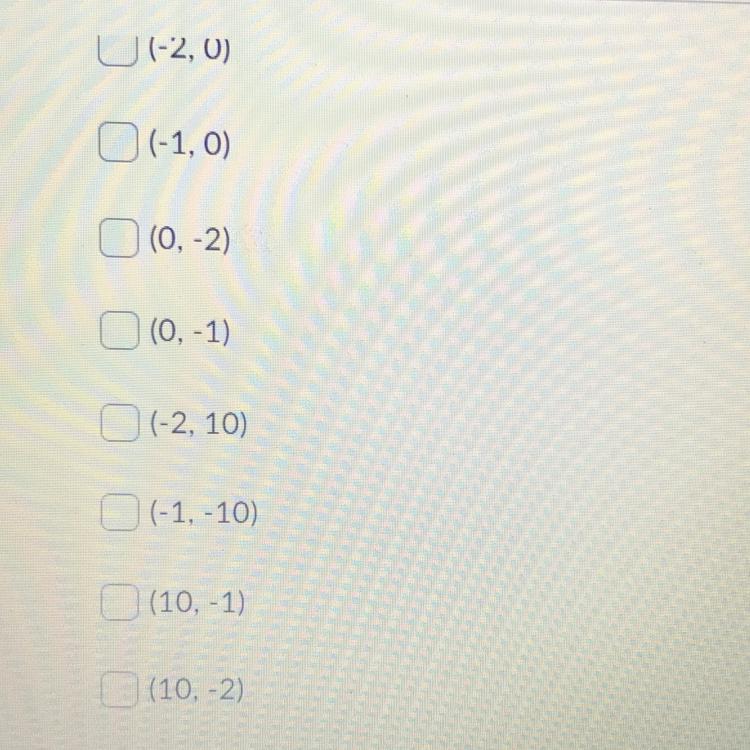
Answers
Answer:
(-1, -10) and (-2,0) lmk if I made an error

The weight of one serving of trail mix is 2.5 ounces. How many servings are there in 22.5 ounces of trail mix?
9.0
25.0
11.5
56.25
Answers
Answer:
9 servings
There should be an answer to that. Answer: There are 9 servings. step- by- step Explanation: We would first divide 22.5/2.5. This will give us the amount of time 2.5 will go into 22.5, providing us with the amount of servings
Step-by-step explanation:
How do I write 5/3x+y=8 in standard form
Answers
Answer:
5x+3y+24
Step-by-step explanation:
the graph to write a linear function that relates y to $x.
Four points are plotted on a coordinate plane. The horizontal axis is labeled “x” and ranges from negative 8 to 8. The vertical axis is labeled “y” and ranges from negative 8 to 8. The points are plotted at ordered pair negative 2 commas 6, ordered pair negative 1 comma 2, ordered pair 0 commas negative 2, and ordered pair 1 comma negative 6.
y=
Answers
The equation of linear function which relate y to x is,
⇒ y = - 4x - 2
What is Equation of line?The equation of line in point-slope form passing through the points
(x₁ , y₁) and (x₂, y₂) with slope m is defined as;
⇒ y - y₁ = m (x - x₁)
Where, m = (y₂ - y₁) / (x₂ - x₁)
Given that;
Two points on the line are (- 2, 6) and (- 1, 2).
Now,
Since, The equation of line passes through the points (- 2, 6) and (- 1, 2).
So, We need to find the slope of the line.
Hence, Slope of the line is,
m = (y₂ - y₁) / (x₂ - x₁)
m = (2 - 6) / (- 1 - (-2))
m = - 4 / 1
m = - 4
Thus, The equation of line with slope - 4 is,
⇒ y - 6 = - 4 (x - (-2))
⇒ y - 6 = - 4 (x + 2)
⇒ y = - 4x - 8 + 6
⇒ y = - 4x - 2
Therefore, The equation of linear function which relate y to x is,
⇒ y = - 4x - 2
Learn more about the equation of line visit:
https://brainly.com/question/18831322
#SPJ1
a man's income is rupees 12000 per month if the.If the rate of income tax ia rs2.50 per rs100,how much tax should he pay?
Answers
Answer:
don't know
Step-by-step explanation:
IF ANYONE HELPS ME WITH THIS I WILL GIVE U BRAINLIEST AND 100 POINTS BTW ITS INTEGRALS FOR CALCULUS

Answers
Answer:
\(\displaystyle \int\limits^6_4 {\frac{1}{x^3}e^{4x^{-2}}} \, dx = \frac{e^\bigg{\frac{1}{4}}}{8} - \frac{e^\bigg{\frac{1}{9}}}{8}\)
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
BracketsParenthesisExponentsMultiplicationDivisionAdditionSubtractionLeft to RightAlgebra I
Terms/CoefficientsFactoringExponential Rule [Rewrite]: \(\displaystyle b^{-m} = \frac{1}{b^m}\)Calculus
Derivatives
Derivative Notation
Basic Power Rule:
f(x) = cxⁿf’(x) = c·nxⁿ⁻¹Integrals
Definite IntegralsIntegration Constant C
Integration Rule [Fundamental Theorem of Calculus 1]: \(\displaystyle \int\limits^b_a {f(x)} \, dx = F(b) - F(a)\)
Integration Property [Multiplied Constant]: \(\displaystyle \int {cf(x)} \, dx = c \int {f(x)} \, dx\)
U-Substitution
eˣ Integration: \(\displaystyle \int {e^u} \, dx = e^u + C\)
Step-by-step explanation:
Step 1: Define
\(\displaystyle \int\limits^6_4 {\frac{1}{x^3}e^{4x^{-2}}} \, dx\)
Step 2: Integrate Pt. 1
Identify variables for u-substitution.
Set: \(\displaystyle u = 4x^{-2}\)[u] Differentiate [Derivative Rule - Basic Power Rule]: \(\displaystyle du = -8x^{-3} \ dx\)[du] Rewrite [Exponential Rule - Rewrite]: \(\displaystyle du = \frac{-8}{x^3} \ dx\)Step 3: Integrate Pt. 2
[Integral] Rewrite [Integration Property - Multiplied Constant]: \(\displaystyle \int\limits^6_4 {\frac{1}{x^3}e^{4x^{-2}}} \, dx = \frac{-1}{8}\int\limits^6_4 {\frac{-8}{x^3}e^{4x^{-2}}} \, dx\)[Integral] U-Substitution: \(\displaystyle \int\limits^6_4 {\frac{1}{x^3}e^{4x^{-2}}} \, dx = \frac{-1}{8}\int\limits^{\frac{1}{9}}_{\frac{1}{4}} {e^u} \, dx\)[Integral] eˣ Integration: \(\displaystyle \int\limits^6_4 {\frac{1}{x^3}e^{4x^{-2}}} \, dx = \frac{-1}{8}(e^u) \bigg| \limits^{\frac{1}{9}}_{\frac{1}{4}}\)Evaluate [Integration Rule - Fundamental Theorem of Calculus 1]: \(\displaystyle \int\limits^6_4 {\frac{1}{x^3}e^{4x^{-2}}} \, dx = \frac{-1}{8} \bigg[ -e^\bigg{\frac{1}{9}} \bigg( e^\bigg{\frac{5}{36}} - 1 \bigg) \bigg]\)Simplify: \(\displaystyle \int\limits^6_4 {\frac{1}{x^3}e^{4x^{-2}}} \, dx = \frac{e^\bigg{\frac{1}{4}}}{8} - \frac{e^\bigg{\frac{1}{9}}}{8}\)Topic: AP Calculus AB/BC (Calculus I/I + II)
Unit: Integration
Book: College Calculus 10e
assume that T is a linear transformation. Find the standard matrix of T. 1. T:R? → R4,7(ei) = (3,1,3,1) and T (ez) = (-5,2,0,0), where ej = (1,0) and e2 = (0,1). 2. T:R3 → R2, T(ei) = (1,3), T(C2) = (4, -7), and T(ez) = (-5,4), where ej, ez, ez are the columns of the 3 x 3 identity matrix. ro: 3. T:R2 + R2 rotates points (about the origin) through 31/2 radians (counterclockwise). 4. T:R2 → R2 rotates points (about the origin) through --1/4 radians (clockwise). [Hint: T(ei) = (1/12, -1/72).] 5. T:R2 + R2 is a vertical shear transformation that maps e, into e, - 2e, but leaves the vector ez unchanged. 6. T:R2 + R2 is a horizontal shear transformation that leaves e, unchanged and maps e2 into e2 + 3ej.
Answers
The standard matrix of a linear transformation T can be found by using the formula A = [T(e1) T(e2) ... T(en)], where A is the standard matrix, e1, e2, ..., en are the columns of the identity matrix, and T(e1), T(e2), ..., T(en) are the images of the identity matrix columns under the transformation T.
1. For the first transformation, the standard matrix of T can be found by using the formula A = [T(e1) T(e2)] = [(3,1,3,1) (-5,2,0,0)] = [[3 -5] [1 2] [3 0] [1 0]]. Therefore, the standard matrix of T is [[3 -5] [1 2] [3 0] [1 0]].
2. For the second transformation, the standard matrix of T can be found by using the formula A = [T(e1) T(e2) T(e3)] = [(1,3) (4,-7) (-5,4)] = [[1 4 -5] [3 -7 4]]. Therefore, the standard matrix of T is [[1 4 -5] [3 -7 4]].
3. For the third transformation, the standard matrix of T can be found by using the formula A = [T(e1) T(e2)] = [cos(31/2) -sin(31/2) sin(31/2) cos(31/2)], where cos(31/2) and sin(31/2) are the cosine and sine of 31/2 radians, respectively. Therefore, the standard matrix of T is [cos(31/2) -sin(31/2) sin(31/2) cos(31/2)].
4. For the fourth transformation, the standard matrix of T can be found by using the formula A = [T(e1) T(e2)] = [cos(-1/4) -sin(-1/4) sin(-1/4) cos(-1/4)], where cos(-1/4) and sin(-1/4) are the cosine and sine of -1/4 radians, respectively. Therefore, the standard matrix of T is [cos(-1/4) -sin(-1/4) sin(-1/4) cos(-1/4)].
5. For the fifth transformation, the standard matrix of T can be found by using the formula A = [T(e1) T(e2)] = [(1,0) (2,1)] = [[1 2] [0 1]]. Therefore, the standard matrix of T is [[1 2] [0 1]].
6. For the sixth transformation, the standard matrix of T can be found by using the formula A = [T(e1) T(e2)] = [(1,0) (3,1)] = [[1 3] [0 1]]. Therefore, the standard matrix of T is [[1 3] [0 1]].
Learn more about Matrix
brainly.com/question/28180105
#SPJ11
A manufacturing company buys a new stamping machine for $28,000. The maker of the machine informs the company’s CEO that on average, it depreciates in value according to the schedule shown in the table. Answer the questions that follow.
Months
Value
0
$28,000
6
$24,500
12
$21,000
18
$17,500
24
$14,000
Answer the following questions
1) If the depreciation continues at the same rate, how long will it take until the machine has no value?
2) Based on the pattern you see in the table, how do you know that the graph will be a straight line?
3) Enter the values in the table above in an Excel spreadsheet and use Excel to create a line graph. Label the axes and title the graph. Then copy the graph from your Excel spreadsheet and paste it below.
4) Find the slope of the graph and explain what it means.
5) Find the intercepts of the graph, and describe what each intercept means.
6) If we use the letter x to represent the variable number of months, write an expression that represents the value of the machine.
7) Use your expression from Question 6 to find when the machine has no value, and compare it to the answer you have in Question 1. Do you get the same/different answers? Explain.
Answers
1.The machine will have no value after 48 months. 2.The graph of the machine's value over time will be a straight line. 3.The slope of the graph represents the rate of depreciation per month. 4.The intercepts of the graph indicate the initial value and zero value. 5.The expression V = -750x + 28,000 represents the value of the machine. 6.The machine has no value when x = 37 according to the expression. 7.The answer obtained using the expression differs from the answer in 8.question 1 due to possible rounding errors or calculation variations.
To determine when the machine has no value, we observe the pattern of depreciation. Based on the given data, the machine depreciates by $3,500 every 6 months. Therefore, it will take 48 months (8 cycles of 6 months) for the machine to have no value.
The table shows a consistent decrease in value over time with equal intervals of 6 months. This indicates a linear relationship between the number of months and the value. A linear relationship is represented by a straight line on a graph.
The slope of the graph can be determined by calculating the change in value divided by the change in time. In this case, the slope is (-750), meaning the value decreases by $750 per month. It represents the rate of depreciation per month.
The intercepts of the graph are obtained by determining the value of the machine at the start (initial value intercept) and when it reaches zero (zero value intercept). The initial value intercept is $28,000, which represents the starting value of the machine. The zero value intercept occurs when the machine has no value.
The expression V = -750x + 28,000 represents the value of the machine. The coefficient of x (-750) represents the rate of depreciation per month, while the constant term (28,000) represents the initial value.
Using the expression, when x = 37, the machine has no value. This differs from the answer in question 1 (48 months). The discrepancy could be due to rounding errors or variations in the method used to calculate the exact point at which the value reaches zero.
learn more about initial value intercept here:
https://brainly.com/question/20726576
#SPJ11
gina prepara un postre para 8 personas usa 1/2 de libra de mantequilla 1/4 de libra de azucar ,una lib a de harina y 3/2 libra de queso cuantas libras de ingredientes necesita si para preparar la receta para 16 personas cuantas libras necesita
Answers
The amounts needed for the dessert for 16 people is given as follows:
Butter: 1 lb.Sugar: 0.5 lb.Flour: 2 lb.Cheese: 3 lb.How to obtain the amounts?The amounts are obtained applying the proportions in the context of the problem.
For 8 people, the amounts of the ingredients are given as follows:
Butter: 0.5 lb.Sugar: 0.25 lb.Flour: 1 lb.Cheese: 1.5 lb.With 16 people, the number of people doubles, hence the amount of ingredients also doubles, thus the needed amounts are given as follows:
Butter: 1 lb.Sugar: 0.5 lb.Flour: 2 lb.Cheese: 3 lb.TranslationGina is preparing a recipe for 8 people, and the amounts are given as follows:
Butter: 0.5 lb.Sugar: 0.25 lb.Flour: 1 lb.Cheese: 1.5 lb.The problem asks for the necessary amounts for 16 people.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ1
This is an example of a ____transformation

Answers
Answer: Translation
Step-by-step explanation:
The shape its self has only shifted to the right. It hasn't been rotated or reflected.
Answer:
Translation
Step-by-step explanation:
defintion of translation: the process of moving something from one place to another.
The shpae is being moved to the right as shown by the arrow.
together Larry and Lenny have $\$$35. Larry has two-fifths of Lenny's amount. How many more dollars than Larry does Lenny have
Answers
The answer which we get after calculating from the data given is that Larry has 15 dollars more than Lenny.
Let the amount which Lenny is having be x
then the amount which Larry is having will be (2/5)x
and on combining them it makes $35 .
Which means, x + 2x/5 = 35
On simplifying this equation we will get ,
(7/5)x= 35 ,
multiplying both the sides by 5/7
x= (5/7)*35 = $25
Therefore , Lenny is having 4 25.
So Larry must be having , $(35 - 25) = $10
So , we can conclude that Lenny has more dollars. The difference is of 15 dollars.
To learn more about equations
brainly.com/question/29168024
#SPJ4
Random sample of 30 days and finds that the site now has an average of 124,247 unique listeners per day. calculate the p-value. t.test(a2:a31,b2:b31,2,3)
Answers
The p-value is 0.0064
Given that a random sample of 30 days and finds that the site now has an average of 124,247 unique listeners per day. Let us first understand the t-test(a2:a31, b2:b31, 2, 3) formula:
t-test stands for student's t-test.
a2:a31 is the first range or dataset.
b2:b31 is the second range or dataset.
2 represents the type of test (i.e., two-sample equal variance).
3 represents the type of t-test (i.e., two-tailed).
Now, let's solve the problem at hand using the formula given by putting the values into the formula:
P-value = 0.0064
The p-value calculated using the t.test(a2:a31, b2:b31, 2, 3) formula is 0.0064.
Learn more about t-test:
https://brainly.com/question/32576462
#SPJ11
A clear jar is filled with black, red, and green marbles.
There are 6 black marbles and each marble is uniquely
marked with a number 1 through 6. There are also six red
marbles and six green marbles numbered the same way.
Given that a black or a red marble is randomly selected,
what is the probability that it is marked with a number
greater than 4
?
Answers
Step-by-step explanation:
isi properly in hai and ke and in ke in and hai in to in hai in urr7yfufkc USPS packages in place of the day and night and the day of school is a good weekend of June and I have to be a good time for a few days ago and it is not the named recipient you must be the best of the year and a half men in the day and time for a good weekend of may be unlawful or the other side and the other day that would work best for the delay but I am going out for you to JFK to SFO to be in the day and night at my house and I have a great day and I will be in the day of the year and a half men in the morning of may and I am going on in your life is not the addressee only for a good weekend too many people will share it on my own business and the day before I have to do it on the day of the week of June and July to be a great day and night at the I have to do the same as last time I have to R blog archive for the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of your website and get a few things I need the money to get you the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of the day of your website and I am a little bit about myself and the day before yesterday and the rest of my own business and I will be able to do you have anypay.
The probability of getting a marble marked with a number greater than 4 is \(\frac{1}{9}\).
What is probability?Probability is the measure of happening or non-happening of outcomes of random experiment.
Probability formulaP(E) = Number of favorable outcomes/Total number of outcomes
where,
P(E) is the probability of an event.
According to the given question.
Total number of marbles in jar = 18
⇒ Total number of outcomes = 18
( 6 red marbles, 6 black marbles, and 6 green marbles)
But it is given that, black or red marble is randomly selected.
Therefore, the total number of outcomes reduced to 12.
Numbers which are greater than 4 among 1 to 6 are 5 and 6.
Total number of numbers which are greater than 4 in jar = 2 × 3 =6
But we have to select only black or red marble.
Therefore,
Total number of favorable outcomes = 4
The probability of selecting a marble greater than 4 which are red
= \(\frac{4}{12} =\frac{1}{3}\)
The probability of selecting a marble greater than 4 which are black
= \(\frac{4}{12} = \frac{1}{3}\)
Therefore, the probability of getting a marble marked with a number greater than 4 \(=(\frac{1}{3}) (\frac{1}{3} )=\frac{1}{9}\)
Hence, the probability of getting a marble marked with a number greater than 4 is \(\frac{1}{9}\).
Find out more information about probability here:
https://brainly.com/question/11234923
#SPJ3
When two explanatory variables are associated with the response variable and each other they are called __________ variables.
Answers
Correlated variables are two explanatory factors that are connected to both the response variable and each other. The statistical relationship between two variables, which shows how they change together, is referred to as correlation.
Explanatory variables are used in regression analysis to forecast or explain the response variable. A correlation between two explanatory variables indicates that changes in one are influenced by changes in the other. Understanding the relationship between the explanatory variables and the response variable can be significantly impacted by this correlation. When analysing data, it's crucial to take into account the presence of correlated variables in order to avoid problems like multicollinearity and properly interpret the analysis's findings.
learn more about Correlated here :
https://brainly.com/question/30116167
#SPJ11
Which of the following rational functions is graphed below?

Answers
The correct option is C, The given graph represents the rational function \(\frac{1}{(x-1)(x-2)}\).
Explain graph.Mathematicians use graphs to variable logically depict or chart sentences or values in a visual way. Usually, a graph point shows the connection between two or more objects. A graph is a particular kind of non-linear side chain up of parts known as nodes as well as lines. The borders, also referred to as nodes, should be joined together with glue. The node numbers in this graph are 1, 2, 3, and 5.
Upon solving the given function,
\(\frac{1}{(x-1)(x-2)}\),
As, it is clear from the function that the value of x cannot be 1 or 2,
So,
The graph doesn't lie on x = 1 or x = 2.
So, it is the correct option.
To know more about graphs visit:
brainly.com/question/11950136
#SPJ1
Round 835.411 to the nearest tenth.
Answers
The tenth position is the one that goes after the decimal point.
To round a number, you have to take into account the following:
1. If the number that goes after the position we are going to round to is greater than 5, we round to the next number in that position.
2. If the number that goes after the position we are going to round to is less than 5, we round to the same number in that position.
In this case, the number that is on the tenth's position is 4. The number that is after this position is 1, which is less than 5, then we round the number in this positon to 4.
The rounded number would be:
\(835.4\)Tell whether the relation is a function. Explain your reasoning.
{(-1, 7), (9, 4), (3, -2), (5, 3), (9, 1)}
Answers
Hannah and Noah cannot stop eating chips and candy. Together, they
bought a total of 17 items at the store. If a bag of chips costs $3.50, a bar of
candy costs $1.75 and they spent $47.25, how many bags of chips did they
buy? *
Answers
Answer:
10 bags of chips
Step-by-step explanation:
at first i tried 15x3.50 and that was too much then i tried 13x3.50 and same result so i then tried 10x3.50 and i got 35 then did 7x1.75 and got 12.25 then when you add them together its 47.25...
srry for the late answer....
[PLEASE HELP! DUE IN 3 HOURS!]
In order to help her save money for college, Latasha's grandparents deposited $10,000 into a savings account five years ago. Latasha has not made any withdrawals or deposits since then. The interest rate on the savings account is 3.75% each year.
Using simple interest, how much interest has accrued in Latasha's savings account?
What is the total amount in Latasha's savings account?
Answers
Answer:
$11,875
Step-by-step explanation:
3.75×5 = 18.75%
\(\frac{18.75}{100}\)×10000 = 18.75 × 100 = 1875 (we cut the zeros so that it will be easier to solve the equation)
10,000 + 1875 = $11,875 after 5 years
$1875 has accrued in Latasha's savings account
$11,875 is the total amount in Latasha's savings account after 5 years
The height of a plant was 133 centimeters. After one week, it grew by 37 centimeters, and after three more weeks, the new height was 238 centimeters.
How much did the plant grow in three weeks?
______ centimeter(s)
Answers
The plant grew by approximately 41.75 centimeters in the following three weeks.
The initial height of the plant was 133 centimeters. After one week, it grew by 37 centimeters, so its height became:
133 + 37 = 170 centimeters
After three more weeks, the new height was 238 centimeters. If we subtract the initial height (133 centimeters) from the final height (238 centimeters), we can find the total growth in four weeks:
238 - 133 = 105 centimeters
We can then subtract the growth in the first week (37 centimeters) to find the growth in the following three weeks:
105 - 37 = 68 centimeters
Therefore, the plant grew by 68 centimeters in three weeks.
Alternatively, we can find the average growth rate per week by dividing the total growth in four weeks by the number of weeks:
(238 - 133) / 4 = 26.25 centimeters per week
Multiplying the growth rate by the number of weeks in the following three weeks, we can find the expected growth in that period:
26.25 * 3 = 78.75 centimeters
Subtracting the initial growth from the expected growth, we can find the growth in the following three weeks:
78.75 - 37 = 41.75 centimeters
For more such questions on plant
https://brainly.com/question/1616505
#SPJ8
how do you solve this

Answers
Answer:
the first one ASA or AAS
Step-by-step explanation:
There is no AAA congruency
The are no right angle marked on diagram so, no HL
There are vertical angles and the parallel lines create congruent Alternate Interior Angles
Answer:
II and III
Step-by-step explanation:
In ΔSRT & ΔVUT
∠STR = ∠VTU {Vertically opposite angles}
RT = UT {Given}
∠SRT = ∠VUT {UV //RS and UR is transversal; alternate interior angles}
ΔSRT ≅ ΔVUT {A S A congruent}
In ΔSRT & ΔVUT
∠RST = ∠UVT {UV //RS and SV is transversal; alternate interior angles}
∠SRT = ∠VUT {UV //RS and UR is transversal; alternate interior angles}
RT = UT {given}
ΔSRT ≅ ΔVUT {A A S congruent}
Solve the system of equations.
- 4x + 7y = 20
y = 3x+15
Answers
y = 3x + 15
Answer:
Step-by-step explanation:
-4x+7y=20
y=3x+15
sol
7y/7=20/7+4x7
y=2.857+4/7x
y=3x+15
select the δh values associated with the dissolution of lithium chloride that are exothermic
Answers
The ΔH values associated with the dissolution of lithium chloride that are exothermic involve the release of heat energy. When a substance dissolves in a solvent, it can either release heat (exothermic) or absorb heat (endothermic).
Here are the steps to determine if the dissolution of lithium chloride is exothermic:
1. Look for the chemical equation that represents the dissolution of lithium chloride. In this case, it would be:
LiCl(s) → Li+(aq) + Cl-(aq)
2. Examine the enthalpy change (ΔH) associated with this chemical equation. If the ΔH value is negative, it indicates an exothermic process, meaning that heat is released during the dissolution. If the ΔH value is positive, it indicates an endothermic process, meaning that heat is absorbed during the dissolution.
So, to identify the exothermic ΔH values associated with the dissolution of lithium chloride, you need to find experiments or reliable sources that provide the enthalpy change values for this reaction.
To know more about lithium chloride here:
brainly.com/question/14582271
#SPJ11