Dylan wants to buy a book for 16.95 and a magazine for 4.50
Answers
Dylan needs $ 21.45 for the purchase of both books and the magazine.
Addition refers to the basic operation of mathematics where we find the sum of two or moe numbers.
We are given that:
Dylan wants to buy two things:
1. she wants to buy a book for $ 16.95
2. she wants to buy a magazine for $ 4.50
So, the total money that she needs will be calculated by adding the prices of both the products.
So, by using addition, we get the total money required by Dylan as:
Total money = $ 16.95 + $ 4.50
Total money = $ 21.45
Therefore, we get that, Dylan needs $ 21.45 for the purchase of both books and the magazine.
Learn more about addition here:
https://brainly.com/question/25421984
#SPJ9
Related Questions
Solve this equation what is 5/6+4/6=
Answers
Answer:
\(1\frac{2}{3}\)
Step-by-step explanation:
\(\frac{5}{6} + \frac{4}{6} = \frac{10}{6} = \frac{5}{3} = 1\frac{2}{3}\)
The temperature in a hotel is 21 °C.
The temperature in the hotel is 26,7°C warmer than at the top of the mountain.
The temperature at the top of the mountain is 3.2°C colder than at the bottom of the mountain.
Work out the temperature at the bottom of the mountain.
Answers
The temperature at the bottom of the mountain is 50.9 °C.
Let's work through the given information step by step to find the temperature at the bottom of the mountain.
The temperature in the hotel is 21 °C.
The temperature in the hotel is 26.7 °C warmer than at the top of the mountain.
Let's denote the temperature at the top of the mountain as T_top.
So, the temperature in the hotel can be expressed as T_top + 26.7 °C.
The temperature at the top of the mountain is 3.2 °C colder than at the bottom of the mountain.
Let's denote the temperature at the bottom of the mountain as T_bottom.
So, the temperature at the top of the mountain can be expressed as T_bottom - 3.2 °C.
Now, let's combine the information we have:
T_top + 26.7 °C = T_bottom - 3.2 °C
To find the temperature at the bottom of the mountain (T_bottom), we need to isolate it on one side of the equation. Let's do the calculations:
T_bottom = T_top + 26.7 °C + 3.2 °C
T_bottom = T_top + 29.9 °C
Since we know that the temperature in the hotel is 21 °C, we can substitute T_top with 21 °C:
T_bottom = 21 °C + 29.9 °C
T_bottom = 50.9 °C
Therefore, the temperature at the bottom of the mountain is 50.9 °C.
for such more question on temperature
https://brainly.com/question/14820864
#SPJ8
I NEED HELP ASPPPP! GIVE POINT IF YOU HELP!

Answers
Answer: All you have to do is since one batch needs 1 1/3 cup of walnuts multiply 1 1/3 to 3 3/4 = 3 1/4 cups
Step-by-step explanation:
PLEASE HELP I REALLY NEED TO GET THIS DONE
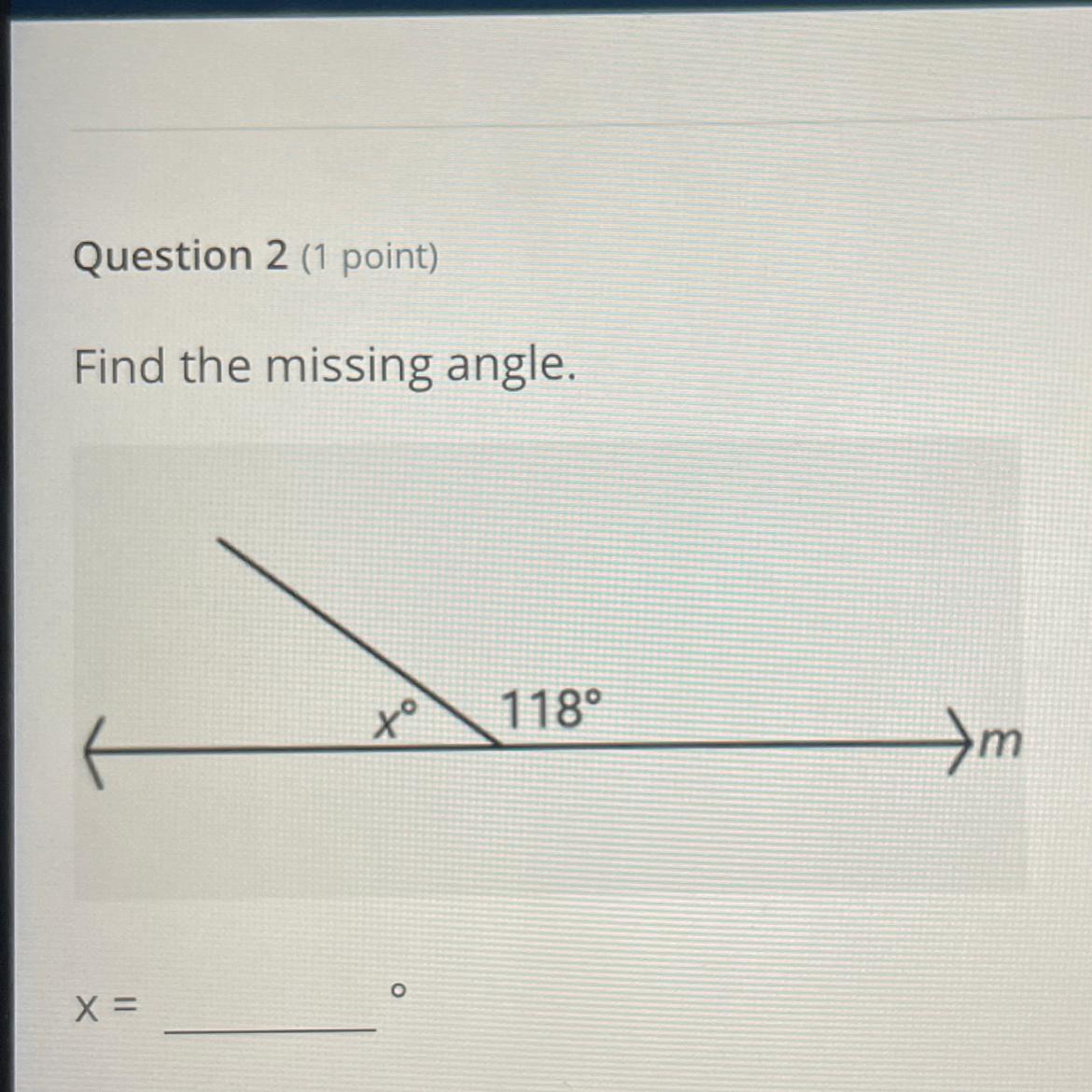
Answers
Hello!
flat angle = 180°
so x = 180° - 118° = 62°
x = 62°
Answer:
62
Step-by-step explanation:
the whole equation is at a 180 degree angle, so to make up the rest, it would be 62 degrees
A swimmer dove below the surface of the ocean. After 2 minutes, she was 12 meters below the surface. At what rate was she diving?
a: -12+x=2
b: 2+x=-12
c: -2x=-12
d: 2x=-12
Answers
The equation of the rate of swimmer diving is b)2+x=-12.
What is equation?
The definition of an equation in algebra is a mathematical statement that demonstrates the equality of two mathematical expressions. For instance, the equation 3x + 5 = 14 consists of the two equations 3x + 5 and 14, which are separated by the 'equal' sign.
Here according to the given after 2 minutes, she was 12 meters below the surface.
Then let us take x as the total timing of the dove below then,
after 2 minutes then
=> x+ 2
Swimmer is 12 meters below the surface then
=> x+2 = -12.
Hence the correct option is b)2+x=-12.
To learn more about equation refer the below link
https://brainly.com/question/29336774
#SPJ1
solve for x=? what is the answer

Answers
x = 5.5
we can solve this by using tanø formula i.e p/b

318÷53 with a remainder
Answers
Answer:
6
Step-by-step explanation:
318 / 53 = 6
the remainder is 0
Si tengo 10 melones y voy a repartir entre 15 niños cuánto le toca a cada uno
Answers
Answer:
0.66 melones por niño o 2/3
Step-by-step explanation:
10/15=2/3=0.66
PLEASE HELP !!!! WHOEVER GETS IT RIGHT GETS BRAINLIEST

Answers
Step by step below

Answer:
x = 22
y = 6
Step-by-step explanation:
GIVEN :-
AB ⊥ CF∠ABE = (4y + 2)°∠FBE = (3x - 2)°∠FBD = (5x + 6)°TO FIND :-
The value of x & yGENERAL CONCEPT TO BE USED IN THIS QUESTION :-
Complementary angles :- Two angles are said to be complementary if sum of measure of both angles is equal to 90°. Linear Pair :- Two angles are said to be a linear pair if both the angles lie on a straight line & sum of both angles is equal to 180°.SOLUTION :-
In the figure given in the question , ∠FBE & ∠FBD are linear pair.
⇒ (3x - 2)° + (5x + 6)° = 180°
⇒ 8x + 4 = 180
⇒ 8x = 176
⇒ x = 22°
Also , AB⊥CF.
⇒ ∠ABE & ∠FBE are complementary angles.
⇒ (4y + 2)° + (3x - 2)° = 90°
⇒ 4y + (3×22) = 90 [∵ Putting x = 23]
⇒ 4y + 66 = 90
⇒ 4y = 24
⇒ y = 6
Now that we know that 2π is about 6.28, π2 is about 9.86, and 58−−√ is between 7 and 8, which choice represents the correct order of these expressions from least to greatest: 58−−√, 2π, π2, 8?
Answers
Answer:
use miss r sir hope I helped you
Which of the following is true about the figure?
a. m MUC - m MUP = m PUC
b. m DUC + m VUA = m DUV
C. m MUC = m MUV = m VUA = m AUD
d. m CUA + m DUP = m PUM

Answers
Answer:
a. m<MUC - m<MUP = m<PUC
Step-by-step explanation:
From the options given, the only statement that is true of the figure shown is:
a. m<MUC - m<MUP = m<PUC
Rationale:
m<MUC = m<MUP + m<PUC (Angle Addition Postulate)
Subtract m<MUP from each side
m<MUC - m<MUP = m<PUC
2 3/4 of 500grams in step by step calculator
Answers
Answer:
To calculate 2 3/4 of 500 grams, follow these steps:
1. Convert the mixed number to an improper fraction:
2 3/4 = (2 x 4 + 3)/4 = 11/4
2. Multiply the improper fraction by 500:
11/4 x 500 = (11 x 500)/4 = 2,750/4
3. Simplify the fraction by dividing the numerator and denominator by their greatest common factor, which is 2:
2,750/4 = (2 x 1,375)/(2 x 2) = 1,375/2
Therefore, 2 3/4 of 500 grams is equal to 1,375/2 grams or 687.5 grams.
Step-by-step explanation:
A $25,000 deposit for 4 years at a 3.56% compounded
continuously.
Principal:
Interest:
Balance:
Answers
Answer:
B. principal
Step-by-step explanation:
There is more than one integer greater than 1 which leaves a reminder of1 when divided by each of the four smallest primes
Answers
Answer:
210
Complete question found at brainly(ID): 18678557 is stated below.
There is more than one integer, greater than 1, which leaves a remainder of 1 when divided by each of the four smallest primes. What is the difference between the two smallest such integers?
Step-by-step explanation:
Prime numbers are numbers that can only be divided by itself and 1
The smallest of the prime numbers we have = 2, 3, 5, 7
Since the integers greater than 1 are said to be divided by the four smallest prime numbers, we would assume the number of integers are 4 in total.
Let the integers be T
From the question:
Integer/(prime number) = quotient + (remainder/prime number)
Integer/(prime number) = Q + R/P
Let the different quotients derived from all 4 prime number = w, x, y, z
For prime 2:
T/2 = w + 1/2
T/2 - 1/2 = w
(T-1)/2 = w
T = 2w + 1
T-1 = 2w
Following the above solution
For prime 3:
T = 3x + 1
T-1 = 3x
For prime 5:
T = 5y + 1
T-1 = 5y
For prime 7:
T = 7z + 1
T-1 = 7z
T-1= T-1 = T-1 = T-1
2w = 3x = 5y = 7z
T-1 = LCM of all the prime numbers
T- 1 = 2×3×5×7
T-1 = 210
T = 210+1 = 211
T = 211
The smallest of the integer greater than 1 that leaves a remainder of 1 = 1(T-1) + 1 = 211
The next after the smallest number: 2(T-1) +1= 2(210) + 1 = 421
The two smallest number = 1(T-1) + 1 and 2(T-1) +1 respectively
The difference between the two smallest such integers = 421-211 = 210
What is blank + 1/16 equals 3/2
Answers
Answer:
1 7/16
Step-by-step explanation:
Let that blank be x
x + 1/16 =3/2
Then, find the common denominator:
3/2 - 1/16
LCM = 16
3/2 *8 = 24/16
24/16 - 1/16
Last, subtract:
23/16
OR
1 7/16
Hope this helps!
mark brainliest if possible!
helppppppppppppppppppppppppppp

Answers
Answer:
14 cm
Step-by-step explanation:
One side of the composite has a length of 6 and the other side has a length of 8.
If we add these two numbers, we'll get the missing side length of the rectangle
6 + 8 = 14 cm
At the end of an advertising campaign, weekly sales declined according to the equation y=19,000(5−0.04x) dollars, where x is the number of weeks after the end of the campaign.a. Determine the sales at the end of the ad campaign.b. Determine the sales 7 weeks after the end of the campaign.c. Does this model indicate that sales will eventually reach $0?

Answers
The function that gives us the weekly sales in dollars as a function of the number of weeks after the end of the ad campaign is
\(y=19000(5^{-0.04x})\)a)
Just after the end of the ad campaign, x=0 (Immediately after the campaign, not even a day has passed). Then, set x=0 and solve for y as shown below
\(\begin{gathered} x=0 \\ \Rightarrow y=19000(5^{-0.04(0)})=19000(5^0)=19000(1)=19000 \\ \Rightarrow y(0)=19000 \end{gathered}\)The answer to part a is 19000
b) 7 weeks after the end of the campaign is equivalent to set x=7 and solving for y
\(\begin{gathered} x=7 \\ \Rightarrow y(7)=19000(5^{-0.04(7)})=19000(5^{-0.28})=12107.15213\ldots\approx12107.15 \\ \Rightarrow y(7)=12107.15 \end{gathered}\)The answer to part b is 12107.15.
c) Set y=0 and solve for x, as shown below
\(\begin{gathered} 0=19000(5^{-0.04x}) \\ \Rightarrow5^{-0.04x}=0 \\ \Rightarrow x\to\infty \end{gathered}\)The sales reach a value of zero after an infinite number of weeks.
Sales can never reach zero because an infinite number of weeks would be needed for that to happen.
4. Solve the system of equations. (6 points)2x-3y = 21-5x+2y = ?a.) Explain the steps you would take to solve the system by eliminating the x-termsb.) Explain the steps you would take to solve the system by eliminating the y-termsc.) Choose either of the methods described in a or b to solve the system of equations. Write your answer as an ordered pair. Show your work.
Answers
Solution
We have the following system of equations given:
2x - 3y = 21 (1)
-6x +2y = 7 (2)
Part a
We can multiple the first equation by 3 and we can add the result to equation (2) and we have:
6x -9y = 63
-6x +2y =7
____________
-7y = 70
y= 70/-7 = -10
Part b
We can multiply the first equation by 2/3 and we can add the result to the second equation and we got:
4/3x - 2y = 14
-6x +2y= 7
____________
-14/3 x = 21
x= -9/2 = -4.5
Part c
We can use other methods for example substitution in order to solve the problem. For example we can solve for x in the first equation and we have:
\(x=\frac{21+3y}{2}\)And replacing in the second one we got:
\(-5(\frac{21+3y}{2})+2y=7\)And after solve for x and y we got:
x= -9/2
y = -10
Do the operation and express the answer in a + bi form. Use fractions in your answer.
1/(8 + i)
Answers
9514 1404 393
Answer:
8/65 -(1/65)i
Step-by-step explanation:
When there is a complex number in the denominator, the denominator can be made real by multiplying numerator and denominator by the conjugate of the denominator.
\(\dfrac{1}{8+i}=\dfrac{8-i}{(8+i)(8-i)}=\dfrac{8-i}{8^2-i^2}=\boxed{\dfrac{8}{65}-\dfrac{1}{65}i}\)
lying Addition and Subtraction of Integers
A bus makes a stop at 2:30, letting off 15 people and letting on 9. The
bus makes another stop ten minutes later to let off 4 more people.
How many more or fewer people are on the bus after the second stop
compared to the number of people on the bus before the 2:30 stop?
Answers
After the second stop, there are 10 fewer people on the bus compared to the number of people on the bus before the 2:30 stop.
Before the 2:30 stop, the bus let off 15 people and let on 9 people. The total change in the number of people at that stop is -15 (let off) + 9 (let on) = -6.
Therefore, there are 6 fewer people on the bus after the 2:30 stop compared to before that stop.
Ten minutes later, the bus makes another stop and lets off 4 more people. This additional change needs to be considered.
Since the previous calculation only accounted for the changes up until the 2:30 stop, we need to adjust the total change by including the subsequent stop.
Adding the change of -4 (let off) to the previous total change of -6, we get a new total change of -10.
Therefore, after the second stop, there are 10 fewer people on the bus compared to the number of people on the bus before the 2:30 stop.
For more such questions on subsequent stop
https://brainly.com/question/29636800
#SPJ8
Identify the percent of change as an increase or a decrease.
9 points to 4 points
o increase
decrease
Find the percent of change. Round to the nearest tenth of a percent if necessary.
The percent of change is
%.
Answers
Answer:
.44%
Step-by-step explanation:
just divide
Find the volume of the solid obtained by rotating the region in the first quadrant bounded by the given curve
about the y-axis.
y = 0.25 - (x-4)²
Answers
Answer:
4π/3 ≈ 4.18879 cubic units
Step-by-step explanation:
You want the volume of revolution formed by revolving the 1st-quadrant area under y = 0.25 -(x -4)² around the y-axis.
Volume
There are several ways to find the volume of revolution. One can integrate a differential of volume, where that differential is a cylindrical shell or a washer. Alternatively, one can multiply the area under the curve by the centerline length of the path of revolution. We choose this latter approach.
AreaThe area in the first quadrant of the region being rotated can be found as the integral between its x-intercepts.
The x-intercepts are found by ...
0 = 1/4 -(x -4)²
x -4 = ±√(1/4) = ±1/2
x = 4 ± 1/2 = {3.5, 4.5} . . . . . . x-intercepts
Then the area is ...
\(\displaystyle\int_{3.5}^{4.5}(0.25-(x-4)^2)\,dx=\left[0.25x-\dfrac{1}{3}(x-4)^3\right]_{3.5}^{4.5}\\\\\\=0.25(1)-\dfrac{1}{3}(0.5^3-(-0.5)^3)=\dfrac{1}{6}\qquad\text{square units}\)
RevolvedThe radius from the y-axis to the line of symmetry of the area is 4 units, so the length of the rotation path is
C = 2πr = 2π(4) = 8π
The product of this path length and the area is ...
V = C·A = (8π)(1/6) = 4π/3
The volume of the solid is 4π/3 cubic units.
__
Additional comment
The area under a symmetrical portion of a parabola is 2/3 of the area of the enclosing rectangle. The portion of the parabola in the 1st quadrant is 1 unit wide and 0.25 units high, so its area is (1)(1/4)(2/3) = 1/6 square unit, as we found above.
The second attachment shows the volume found using the cylindrical shell method.
<95141404393>


the sum of interior angle of a polygon of n side is 2160,find n
Answers
Answer:
3.9/5
46
let the number of side = n
a/q n-2×180=2160
n-2=2160/180=12
n=12+2=14
no. of side this polygon have =14
Step-by-step explanation:
Create a pattern for the rule a +4.
Answers
As you can see, each number in the pattern is obtained by adding 4 to the previous number.
What is equation?An equation is a mathematical statement that shows the equality of two expressions, usually with an equal sign "=" in between them. An equation can contain variables, constants, and operators. The variables are represented by letters and can take on different values, while constants are fixed values that do not change. Operators include mathematical symbols like plus, minus, multiplication, and division, as well as exponents and logarithms.
Here,
Sure, here's a pattern for the rule a + 4:
a = 1: 1 + 4 = 5
a = 2: 2 + 4 = 6
a = 3: 3 + 4 = 7
a = 4: 4 + 4 = 8
a = 5: 5 + 4 = 9
a = 6: 6 + 4 = 10
a = 7: 7 + 4 = 11
a = 8: 8 + 4 = 12
a = 9: 9 + 4 = 13
a = 10: 10 + 4 = 14
To know more about equation,
https://brainly.com/question/2228446
#SPJ1
What’s the quotient ? I’m helping out with my sisters homework once again and I don’t wanna explain it to her. Please help me ;-;

Answers
Answer:
quotient is the answer to division. So if 7/2=3 with a remainder of 1, then the quotient is 3
Coach Walker has a cooler that can hold 5 1/2 gallons of a sports drink. He fills 3/4 of
the cooler with the sports drink to bring to football practice.
B. How many players can fill their water bottles with the sports drink before the cooler is empty? (1 Gallon = 8 pints)
Answers
The answer of the question based on the players can fill their water bottles with the sports drink before the cooler is empty is 15 players.
What is Amount?An amount may refer to quantity of something that can be measured, such as amount of substance or amount of the time.
The cooler can hold 5 1/2 gallons of sports drink, and Coach Walker fills 3/4 of it, which means he fills:
5 1/2 gallons * 3/4 = (5 * 2/2 + 1/2) * 3/4 = 15/4 gallons
We know that 1 gallon equals 8 pints, so we can convert the 15/4 gallons to pints:
15/4 gallons * 8 pints/gallon = 30 pints
Therefore, there are 30 pints of sports drink in the cooler. Assuming each player's water bottle can hold 2 pints of sports drink, we can find how many players can fill their bottles before the cooler is empty by dividing the total amount of sports drink by the amount used by each player:
30 pints / 2 pints/player = 15 players
Therefore, 15 players can fill their water bottles with the sports drink before the cooler is empty.
To know more about Pints visit:
https://brainly.com/question/17416955
#SPJ1
What is the value of y?

Answers
Answer:
The answer is C (46)
Step-by-step explanation:
Since the measurement of the triangle is 180 then you must subtract 180 by 88 to create 92 and divided that 92 by 2 to get 46.
Pearl writes down seven consecutive integers, and adds them up. The sum of the integers is equal to $\frac{21}{4}$ times the largest of the seven integers. What is the smallest integer that Pearl wrote down?
Answers
The smallest integer that Pearl wrote down is -12.Let's assume the smallest integer that Pearl wrote down is represented by the variable "x."
According to the given information, the sum of the seven consecutive integers is equal to $\frac{21}{4}$ times the largest integer.
The sum of consecutive integers can be expressed as the sum of an arithmetic series. The sum of an arithmetic series can be calculated using the formula:
Sum = (n/2)(first term + last term)
In this case, the first term is x and the last term is x + 6 (since there are seven consecutive integers). Therefore, the sum of the integers can be written as:
Sum = (7/2)(x + (x + 6))
Simplifying the equation:
(7/2)(2x + 6) = (21/4)(x + 6)
Multiplying both sides by 4 to eliminate the fractions:
14x + 42 = 21x + 126
Subtracting 14x from both sides:
42 = 7x + 126
Subtracting 126 from both sides:
-84 = 7x
Dividing both sides by 7:
-12 = x.
For more such questions on Smallest integer:
https://brainly.com/question/30076540
#SPJ8
Name the property for the given statement 2(10 + 15) = 2(10) + 2(15)
Answers
Evaluate
O 15
O1
What’s the answer?????
