fair coin is tossed 3 times. Show that the events A:{ at least two heads } and B:{ one or two tails } are independent.
Answers
If P(A ∩ B) = P(A) × P(B), so we can conclude that events A and B are independent.
The events A and B are independent if and only if the probability of their intersection, P(A ∩ B), is equal to the product of their individual probabilities, P(A) and P(B).
In this case, event A represents getting at least two heads when tossing a fair coin 3 times, and event B represents getting one or two tails. To show that A and B are independent, we need to demonstrate that P(A ∩ B) = P(A) × P(B).
To calculate P(A), we can determine the probability of getting exactly two heads and the probability of getting all three heads and then sum them up. P(A) = P(exactly two heads) + P(all three heads).
Similarly, to calculate P(B), we can determine the probability of getting one tail and the probability of getting two tails and then sum them up. P(B) = P(one tail) + P(two tails).
Next, we calculate P(A ∩ B), which represents the probability of getting at least two heads and one or two tails simultaneously.
If P(A ∩ B) = P(A) × P(B), then we can conclude that events A and B are independent.
Learn more about Probability here:
#SPJ11
Related Questions
suppose f(x,y)=xyf(x,y)=xy, p=(3,4)p=(3,4) and v=−1i−4jv=−1i−4j. a. find the gradient of ff.
Answers
The gradient of the function f(x, y) = xy is a vector that represents the rate of change of the function with respect to its variables. The gradient of f is ∇f = (y, x).
The gradient of a function is a vector that contains the partial derivatives of the function with respect to each variable.
For the function f(x, y) = xy, we need to find the partial derivatives ∂f/∂x and ∂f/∂y.
To find ∂f/∂x, we differentiate f with respect to x while treating y as a constant.
The derivative of xy with respect to x is simply y, as y is not affected by the differentiation.
∂f/∂x = y
Similarly, to find ∂f/∂y, we differentiate f with respect to y while treating x as a constant.
The derivative of xy with respect to y is x.
∂f/∂y = x
Thus, the gradient of f is ∇f = (∂f/∂x, ∂f/∂y) = (y, x).
In this specific case, given that p = (3, 4), the gradient of f at point p is ∇f(p) = (4, 3).
The gradient vector represents the direction of the steepest increase of the function f at point p.
Note that v = -i - 4j is a vector that is not directly related to the gradient of f. The gradient provides information about the rate of change of the function, while the vector v represents a specific direction and magnitude in a coordinate system.
Learn more about derivative here:
https://brainly.com/question/30401596
#SPJ11
Simplify (7x) - (-2x).
A.-9x
B.-5x
C.9x
D.5x
Answers
Answer:
Step-by-step explanation:
7x - (-2x) = 7x + 2x
= 9x
Let A be an nxn matrix and let B=A+I. Is it possible for A and B to be similar? Explain.
Answers
No, it is not possible for matrix A and B to be similar when B = A + I, where A is an nxn matrix and I is the identity matrix of the same size.
Two matrices A and B are said to be similar if there exists an invertible matrix P such that \(B = P^{(-1)}AP\). In this case, A and B have the same eigenvalues, and their corresponding eigenvectors can be related through the matrix P.
Let's consider the eigenvalues of B. Since B = A + I, the eigenvalues of B are obtained by adding 1 to each eigenvalue of A. In other words, if λ is an eigenvalue of A, then λ + 1 is an eigenvalue of B.
Now, suppose A and B are similar. This means that they have the same eigenvalues. However, the eigenvalues of B are shifted by 1 compared to the eigenvalues of A. Therefore, it is not possible for A and B to have the same eigenvalues, unless A has eigenvalues that are all equal to -1.
Since the eigenvalues of A and B are different, we can conclude that A and B cannot be similar.
Learn more about invertible matrix here: https://brainly.com/question/31062095
#SPJ11
Cho sells kakigori, a Japanese frozen dessert flavored with sweet syrup, at a street stand.
The scatter plot shows the daily high temperature and the number of servings of kakigori
Cho sells each day for 12 days. Based on a line of best fit for the data, about how many
servings of kakigori will Cho sell on a day when the high temperature is 29° Celsius?
Number of Servings Sold
115
110
105
100
95
90
85
80
75
70
65
60
55
50
0
18 19 20 21 22 23 24 25 26 27 28 29 30
Temperature (°C)
94
101
88
111
Answers
We can estimate that Cho will sell around 100 servings of kakigori on a day when the high temperature is 29° Celsius.
Based on the line of best fit for the data, we can estimate the number of servings of kakigori Cho will sell on a day when the high temperature is 29° Celsius.
From the scatter plot, we can see that the line of best fit is increasing as the temperature increases.
By estimating the value on the line of best fit for the temperature of 29° Celsius, we can approximate the number of servings sold. Based on the scatter plot, it appears that the number of servings sold is around 100 when the temperature is 29° Celsius.
Therefore, we can estimate that Cho will sell around 100 servings of kakigori on a day when the high temperature is 29° Celsius.
Learn more about Scatter Plot here:
https://brainly.com/question/29231735
#SPJ1
consider a polynomial f (λ) with real coefficients. show that if a complex number λ0 is a root of f (λ), then so is its complex conjugate, λ0.
Answers
If a complex number λ0 is a root of a polynomial with real coefficients, then its complex conjugate, λ0, is also a root of the polynomial.
Let's consider a polynomial with real coefficients, f(λ), which can be expressed as f(λ) = a_n\(\lambda^n\) + a_{n-1}\(\lambda^{n-1}\) + ... + a_1λ + a_0, where a_n, a_{n-1}, ..., a_1, a_0 are real coefficients and n is a positive integer. Now, suppose λ0 is a complex number that satisfies f(λ0) = 0, which means λ0 is a root of the polynomial.
To prove that the complex conjugate of λ0, denoted as λ0, is also a root of f(λ), we can utilize the fact that the coefficients of f(λ) are real. Consider the polynomial f(λ*) = a_n\((\lambda*)^{n}\) + a_{n-1}\((\lambda*)^{n-1}\) + ... + a_1λ* + a_0, where λ* denotes the complex conjugate of λ.
Since the coefficients of f(λ) are real, we know that a_i* = a_i for all i. Therefore, when we substitute λ* for λ in f(λ), we obtain f(λ*) = a_n\((\lambda*)^{n}\) + a_{n-1}\((\lambda*)^{n-1}\)+ ... + a_1λ* + a_0.
Now, we can observe that f(λ*) is the complex conjugate of f(λ). Since λ0 satisfies f(λ0) = 0, it implies that f(λ0*) = 0 as well. Thus, λ0* is also a root of f(λ).
In conclusion, if a complex number λ0 is a root of a polynomial with real coefficients, then its complex conjugate, λ0*, is also a root of the polynomial.
Learn more about complex number here:
https://brainly.com/question/20566728
#SPJ11
Help please and thank you :)

Answers
Answer:
y ≥ 3x -1x +3y ≤ 6Step-by-step explanation:
Given a graph showing two solid lines that cross in an X pattern with the left quadrant shaded, you want to know the system of equations that is being graphed.
LinesThe answer choices tell you that the boundary lines have equations ...
y = 3x -1x +3y = 6The boundary lines on the graph are solid, so they are included in the solution set. Both inequalities will include the "or equal to" case. (This eliminates choices B and C.)
ShadingThe shading on the graph is to the left of each line, where x-values are less than those on the line. The shading is above the line with positive slope.
To tell where the shading is for a given inequality, look at the inequality symbol and a variable with a positive coefficient. (Coefficient and constant values do not matter for the purpose of determining shading.)
y > . . . . shading is above the dashed line. Shaded y-values are greater than those on the line.y ≤ . . . . shading is below the solid line≥ x . . . . shading is left of the solid line (equivalent to x ≤ )x ≥ . . . . shading is right of the solid lineKnowing how the shading works, you can reject choice D:
y ≤ 3x -1 . . . . shading will be below (to the right of) this linex +3y ≥ 6 . . . . shading will be above (to the right of) this lineThat is, for this system of equations, the shading would be in the right quadrant of the X where the lines cross.
System of inequalitiesThe system of inequalities you're looking for is the one shown in the attachment (choice A). These have shading to the left of the solid line in both cases:
≥ x . . . . . from y ≥ 3x -1x ≤ . . . . . from x +3y ≤ 6
An electrically neutral atom of beryllium has four protons. Which statement is true? There are four protons in the nucleus. There is a charge of −4 on an atom of beryllium. There are four neutrons in the nucleus. There is a charge of +4 on an atom of beryllium.
Answers
The right answer is that there are four electrons.
What is an atomic number?The charge number of an atomic nucleus is the chemical element's atomic number, also known as the nuclear charge number.
For conventional nuclei, this is comparable to the proton number, or the number of protons found in the nucleus of each atom of that element.
Equal numbers of protons and electrons make up a neutral atom. This figure establishes the atom's atomic number.
The fourth element on the periodic table is beryllium.
Number of protons in Be-atom = 4
Number of electrons in Be-atom = 4
Therefore, it is true that there are four electrons.
To know more about an atomic number follow
https://brainly.com/question/11353462
#SPJ1
Please help me
20 points




Answers
Answer:
1). Simplify the expression.
Exact Form:
( \(\frac{37}{25}\) )
Decimal Form:
1.48
Mixed Number Form:
( \(1\frac{12}{25}\) )
2). Simplify the expression.
91.46
3). Simplify the expression.
Exact Form:
\(\frac{17}{24}\)
Decimal Form:
0.7083 ( bar notation)
4). Simplify the expression.
81.49
On Saturday, a local hamburger shop sold a combined total of 369 hamburgers and cheeseburgers. The number of cheeseburgers sold was two times the
number of hamburgers sold. How many hamburgers were sold on Saturday?
hamburgers
Answers
Answer:
123
Step-by-step explanation:
Let 'x' = number of hamburgers.....then cheeseburgers = '2x'
added together they = 369
x + 2x = 369
3x = 369
x = 123 hamburgers
write the equation of a line in slope-intercept form that has a slope of 2/3 and a y-intercept of (0,4)
Answers
Answer:
2/3+0x4=2/3
Step-by-step explanation: that was math right there
an old friend tells you that 30 years ago she nailed a sign into a tree trunk at a height of 1 meter. she now says the sign is 25 meters up in the tree. should you believe her? why or why not?
Answers
You should not believe her .
Given that,
You learn from an old friend that thirty years ago, she nailed a sign into the trunk of a tree at a height of one meter. She now claims that the sign is located 25 meters up a tree.
Meristems that are found at the tips of branches allow trees to grow taller. Apical meristems are the name for these meristems. Due to apical meristems, roots also spread into the soil by developing at their terminals. Apical meristems can be seen in every tree bud you can see. Another meristem, the vascular cambium, which was previously noted, is responsible for the expansion of trunk diameter. The trunk, branches, and roots continue to get bigger because the vascular cambium creates new xylem and phloem every year. Have you ever witnessed a fence board or wire transform into a tree?
The vascular cambium is responsible for that outcome. Height development prevents the fence wire or board from rising into the air.
Therefore, you should not believe her .
To learn more about height click here:
brainly.com/question/10726356
#SPJ4
how many sides do a polygon have if the sum of the interior angles is 1,260
Answers
1260 = 180(n-2)
1260/180 = n-2
7 = n-2
N = 9
If4x-2y=6, then y =?
Answers
4x-2y=6, move the x to the other side by subtracting 4x from both sides
-2y=-4x+6, leave the y by itself and divide by -2, since that’s what y is being multiplied by
you get y=2x-3
please help :(
Write a story for this equation: $10 - $4.05 = c.
Answers
Answer:
Mr Singh went to the shops to buy some Coke. He wanted a $10 dollar pack which had 12 coke cans. He had a coupon which took off $4.05. Using the information above, what did Mr Singh pay?
Answer:
Step-by-step explanation:
John is given $10 pocket money. After spending $4.05 in the local store he has c dollars left.
I AM REAALY STUCK!!!!! I NEED THIS ASAP ONLY GOT 1 MIN, NO SCAMS PLS
What is the median of Restaurant B's food quality ratings?
2
3
4
1
5

Answers
The median of Restaurant B's food quality ratings is 4.
What is the median?
The median is the middle number in a sorted, ascending or descending, list of numbers and can be more descriptive of that data set than the average. The median is sometimes used as opposed to the mean when there are outliers in the sequence that might skew the average of the values.
Food Quality Frequency CF
1 3 3
2 4 7
3 5 12
4 7 19 ⇒ median
5 6 25
Total we have 25 ratings, out of those 25 ratings 13th rating is the middle term so it will be our 4.
Thus, the median of Restaurant B's food quality ratings is 4.
Learn more about Median from :
https://brainly.com/question/21396105
#SPJ1
There are 13 red roses 6 pink roses and 16 white roses in patricks garden what percentage are pink roses
Answers
Answer:
17.1429% ?
Step-by-step explanation:
Prove the following using direct or indirect proof. Use the appropriate postulates and theorems to prove that: ∆KOF ≅ ∆VOA when O is the midpoint of side KV.

Answers
Answer:
Step-by-step explanation:

A two digit number is 6 times its singles digit. The sum of the digits is 6. Find the number
Answers
When the area in square units of an expanding circle is increasing twice as fast as its radius in linear units, the radius is Select one: A. 1/(4Pi) B. 1/Pi C. 1/4 D. 1 E. Pi
Answers
When the area in square units of an expanding circle is increasing twice as fast as its radius in linear units, the radius is E. Pi.
The area of a circle is given by the formula A = π\(r^2\), where A is the area and r is the radius. If the area of the circle is increasing twice as fast as its radius, we can express this relationship as:
dA/dt = 2(dr/dt)
Here, dA/dt represents the rate of change of the area with respect to time, and dr/dt represents the rate of change of the radius with respect to time.
Since we are considering an expanding circle, both dA/dt and dr/dt are positive.
Now, let's solve for the relationship between dA/dt and dr/dt:
dA/dt = 2(dr/dt)
π\(r^2\) = 2(dr/dt)
Dividing both sides by \(r^2\):
π = 2(dr/dt) / \(r^2\)
Simplifying further:
π = 2(dr/dt) / \(r^2\)
π = 2/r
Therefore, the relationship between the rate of change of the area and the rate of change of the radius is π = 2/r.
To find the value of r, we rearrange the equation:
r = 2/π
Thus, the radius is 2/π, which is approximately 0.6366.
To know more about area, refer here:
https://brainly.com/question/15906335
#SPJ4
roslyn has twenty boxes. thirteen of the boxes contain pencils, nine of the boxes contain pens, and three of the boxes contain neither pens nor pencils. how many boxes contain both pens and pencils?
Answers
The number of boxes containing both pens and pencils is 2.
What is set?
A set is defined as a well-defined collection of distinct objects. It is denoted by the capital letters of the English alphabet. Its value remains the same for every individual.
Calculation for the number of boxes containing both pens and pencils
Total number of boxes is given by the union of two sets A and B; i.e. A∪B= 20
Boxes having pencils is given by the set A = 13
Boxes having pens is given by the set B = 9
Boxes having neither pens nor pencils, A'∩B' = 3
Boxes containing both pens and pencils are given by the intersection of the sets, A∩B
A∩B = A + B - A∪B
= 13 + 9 - 20
= 2
Hence, the number of boxes containing both pens and pencils is 2.
To learn more about sets, visit here:
https://brainly.com/question/24462379
#SPJ4
Justify a Solution The expression -3m - [2m + (5 - m)] + 7 was simplified as 2 - 4m. Without simplifying, explain how you can show that it has been simplified correctly.
Answers
Step-by-step explanation:
Given the expression
\(-3m - [2m + (5 - m)] + 7\)
Step one
Firstly we have to open the inner bracket we have
\(-3m - [2m + 5 - m] + 7\)
Step two
we proceed further by opening up the next bracket we have
\(-3m - 2m -5 +m+ 7\)
Step three
we then have to collect like terms of m and rearrange.
\(=-3m - 2m+m -5+ 7\\=-4m+2\\=2-4m\)
What is the value of the function at x= -2?
Y=-4
Y=0
Y=2
Y=3
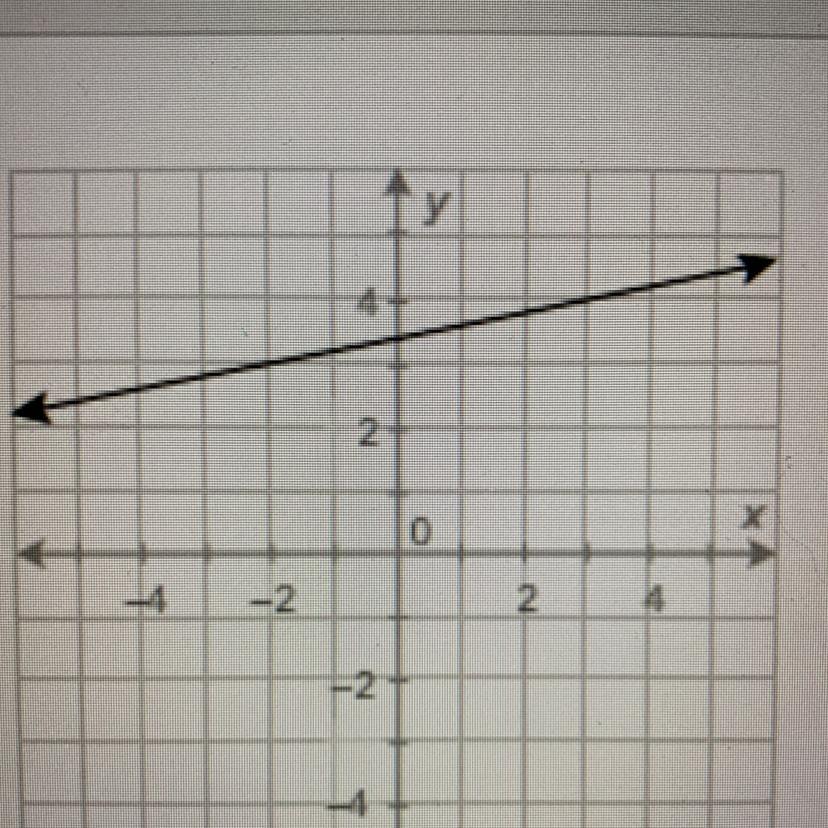
Answers
Answer:
y = 3
Step-by-step explanation:
When x = -2, y = 3. You can tell because when you go to the -2 on the graph, and you move upwards, towards the line, the line lays on the point 3.
A small toy car costs $3. A large toy car costs 5 times as much as the small one. Aaron wants to buy one of each. Which equation can he use to find the cost (a) of the two cars?
Answers
Answer: He can use 3 x 5 = 15 and 15 + 3.
Step-by-step explanation:
Since a small car is $3, and the large car is 5x the price of the small car, he can use the equation 3 x 5 = 15, because the small car is $3, and the large car is 5x the price. You can use 15 + 3 = 18, because the small car is $3, so you also have to add that.
Here to help!
The equation is x + 5x = 18 , where x is the cost of small toy car and the total cost of the two cars = $ 18
What is an Equation?Equations are mathematical statements with two algebraic expressions flanking the equals (=) sign on either side.
It demonstrates the equality of the relationship between the expressions printed on the left and right sides.
Coefficients, variables, operators, constants, terms, expressions, and the equal to sign are some of the components of an equation. The "=" sign and terms on both sides must always be present when writing an equation.
Given data ,
Let the total cost of the two cars be A
Now , the equation will be
Let the cost of the small toy car be = x
The cost of small toy car = $ 3
The cost of the large car = 5 x cost of small toy car
Substituting the values in the equation , we get
The cost of the large car = 5 x 3
The cost of the large car = $ 15
So , the cost of two cars = x + 5x
Substituting the values in the equation , we get
The total cost of the two cars A = 15 + 3
The total cost of the two cars A = $ 18
Therefore , the value of A is $ 18
Hence , the equation is A = x + 5x
To learn more about equations click :
https://brainly.com/question/19297665
#SPJ2
Define Q as the region that is bounded by the graph of the
function g(y)=−2y−1‾‾‾‾‾√, the y-axis, y=4, and y=5. Use the disk
method to find the volume of the solid of revolution when Q
Question == Define as the region that is bounded by the graph of the function g(y) = the disk method to find the volume of the solid of revolution when Q is rotated around the y-axis. -2√y — 1, th
Answers
The region Q is bounded by the graph of the function g(y) = -2√y - 1, the y-axis, y = 4, and y = 5. To find the volume of the solid of revolution when Q is rotated around the y-axis, we can use the disk method.
Using the disk method, we consider an infinitesimally thin disk at each value of y in the region Q. The radius of each disk is given by the distance between the y-axis and the graph of the function g(y), which is |-2√y - 1|. The height of each disk is the infinitesimally small change in y, which can be denoted as Δy.
To calculate the volume of each disk, we use the formula for the volume of a cylinder: V = πr^2h, where r is the radius and h is the height. In this case, the radius is |-2√y - 1| and the height is Δy.
To find the total volume of the solid of revolution, we integrate the volume of each disk over the interval y = 4 to y = 5.
The integral will be ∫[4,5] π|-2√y - 1|^2 dy. Evaluating this integral will give us the volume of the solid of revolution when Q is rotated around the y-axis.
Learn more about area of the region bounded by the graphs :
https://brainly.com/question/32301637
#SPJ11
In the figure below, all lines intersect at point W. What is the value of angle VWX?*

Answers
Answer: 55
Step-by-step explanation:
Angle VWX and the 55 degree angle are vertical angles.
what is the area of this shape?

Answers
Answer:
34
Step-by-step explanation:
34
Answer:
24
Step-by-step explanation:
4×6=24
you need to multiply one side with the other side
first side - 4
second side - 6
I will mark you brainliest!!!! What is the first step in adding these equations to eliminate y?

Answers
Answer:
I believe D
Step-by-step explanation:
If you multiplied -3 times 3 that would make -9
you can then add 9 + -9 and cross y off
graph each function determine which of the functions are linear. find the y-intercept of each function and the slope
Answers
The graph of f(x) = 2x + 3 is a linear function and its y-intercept is y = 2x + 3.
What is linear function?Any function is referred to as linear if it represents a straight line on the coordinate plane. For instance, the linear function represented by the equation y = 3x – 2 looks like a straight line on a coordinate plane. Due to the fact that f(x) can substitute for y, this function can be written as f(x) = 3x - 2.
First find the slope of the line
y - y₁ = m(x - x₁)
0 - 3 = m(-1.5 - 0)
m = 2
From the graph, we can take any of the two points i.e.(0, 3) and put it in slope formula
y - y₁ = m(x - x₁)
So,
y - 3 = 2(x - 0)
y = 2x + 3 (y-intercept)
Learn more about linear function
https://brainly.com/question/20286983
#SPJ1
Full question:
Graph each function determine which of the functions are linear. find the y-intercept of each function and the slope.
f(x) = 2x + 3

Help greatly appreciated.
Find the values of x and y. I need help knowing which formula to use and what to plug into the formula. Thank you.

Answers
The value of x is 4√6. The value of y is 4√2.
What are similar triangles?
If two triangles have the same ratio of corresponding sides and an equal pair of corresponding angles, they are similar. When two or more figures have the same shape but differ in size, they are referred to as similar figures.
Consider △ABC:
Height = AC = x, Hypoteneous = BC = 4+8 = 12.
Consider △ADC:
Height = DC = 8, Base = AD = y, Hypoteneous = AC = x
Consider △ABD:
Height = AD = y, Base = 4.
The triangle ABC is similar to ADC, and ABC is similar to ABD. Since all have one right angle and one angle is common.
The ratio of the corresponding sides of similar triangles is constant.
Consider △ABC and △ADC:
AC/DC = BC/AC
x/8 = 12/x
x² = 12×8
x = 4√6.
Since ABC is similar to ADC and ABC is similar to ABD. Then ADC is similar to ABD.
Consider △ADC and △ABD:
DC/AD = AD/BD
8/y = y/4
y² = 4×8
y = 4√2
To learn more about similar triangles, click on the below link:
https://brainly.com/question/16364919
#SPJ1
Dorris deposits $200 into a savings account that has a simple interest rate of 4. 1% Max deposits 300 dollars into a savings account that has a simple interest rate of 1. 9%, who earn more interest in 15 months round to the nearest cent if necessary
Answers
Dorris earns $12.25 in interest over 15 months, while Max earns $2.84 in interest over the same period. So, Dorris earns more interest than Max.
To find out who earns more interest in 15 months, we can calculate the interest earned by each person using the formula:
Interest = Principal x Rate x Time
For Dorris, we have:
Interest = $200 x 0.041 x (15/12)
Interest = $12.25
For Max, we have:
Interest = $300 x 0.019 x (15/12)
Interest = $2.84
Interest refers to the cost of borrowing money, usually expressed as a percentage of the amount borrowed over a specific period of time. When someone borrows money, they are required to pay back not only the amount borrowed but also an additional amount, which is the interest. Interest is how lenders make money and is an important component of the financial system.
There are different types of interest rates, including fixed and variable rates. A fixed interest rate remains the same throughout the loan term, while a variable interest rate may change based on market conditions. Interest rates can also be compounded, which means that interest is added to the principal amount, and future interest payments are calculated based on the new higher amount.
To learn more about Interest visit here:
brainly.com/question/30393144
#SPJ4