Finance Suppose that $4.100 is invested at 4 6% annual interest rate compounded monthly How much money will be in the account in (A) 11 months? (B) 4 years? (A) Amount after 11 months $_______(Round to the nearest cent) (B) Amount after 4 years $_______ (Round to the nearest cent) .
Answers
Amount after 4 years $5,076.55 (Round to the nearest cent).
Hence, the answer is $5,076.55.
Given: Amount = $4,100;
Rate of interest = 4.6%
annual interest rate compounded monthly,
Time = n(A) 11 months
We can use the formula to calculate compound interest:
Compound Interest formula:
A = P(1 + r/n)^(nt)
where
A = Amount
P = Principal amount invested
r = Rate of Interest
n = number of times interest applied per period
t = time (in years)
Part (A) Calculation:
Amount after 11 months = $_______(Round to the nearest cent)
First, we need to calculate the monthly interest rate.i.e, Rate of interest
r = 4.6% / 12
= 0.003833
n = 11/12 (since the interest is compounded monthly)
P = $4,100
As per the formula
,A = P(1 + r/n)^(nt)
A = 4100 (1 + 0.003833)^(11/12)
A = $4,271.02
Therefore, Amount after 11 months $4,271.02 (Round to the nearest cent).
Hence, the answer is $4,271.02.
Part (B) Calculation:
Amount after 4 years $_______ (Round to the nearest cent)
P = $4,100n = 4 × 12 = 48
As per the formula,
A = P(1 + r/n)^(nt)
A = 4100 (1 + 0.003833)^(48)
A = $5,076.55
Therefore, Amount after 4 years $5,076.55 (Round to the nearest cent).
Hence, the answer is $5,076.55.
To know more about nearest cent visit:
https://brainly.com/question/12963743
#SPJ11
Related Questions
The Blackburn family has a square field where they keep their cattle. The area of the field is 40,000 ft square, and Mr. Blackburn wants to put a fence diagonally through the field. What should the length of the fence be?
Answers
If area of "square-field" is 40000 ft square, and Mr. Blackburn is putting a fence diagonally in field, then the length of fence be is 282.84 ft.
The area of the square-field is = 40000 ft²,
We equate this with area formula,
We get,
⇒ (side)² = 40000,
⇒ side = 200,
substituting the side-length as 200 ft, in the diagonal formula,
we get,
⇒ Length of diagonal of field is = (side)√2,
⇒ Length of diagonal of field is = (200)√2,
⇒ Length of diagonal of field is ≈ 282.84 ft.
Therefore, the length of the fence of the field is 282.84 ft.
Learn more about Area here
https://brainly.com/question/30578725
#SPJ1
Can you please help me and also explain

Answers
Answer:
We can begin by simplifying each equation:
A. 52 = 8n + 4 can be rewritten as 48 = 8n or 6 = n
B. 4(2n + 1) = 52 can be simplified to 8n + 4 = 52 or 8n = 48 or 6 = n
C. 4=52-8n can be rewritten as 8n = 48 or 6 = n
D. 4n = 48 can be simplified to n = 12
Therefore, equations A, B, and C are equivalent, and equation D is not equivalent to the others.
So, the correct answers are A, B, and C.
Answer:
Step-by-step explanation:first you have to multiply then divide then add then subtract and then substitute and then you will get the answer.
When interpreting f(7, 31) = 4.78, p > 0.05, how many subjects were tested in this simple one-way anova?
Answers
39 subjects were tested in this simple one-way ANOVA.
The df for F distribution is (treatment df, error df)
Using given information
Treatment df = 7
Error df = 31
Total df= 7+31 = 38
Again, total df = N-1, N= number of subjects tested
Then, N-1 = 38
=> N= 39
One-way ANOVA is typically used when there is a single independent variable or factor and the goal is to see whether variation or different levels of that factor have a measurable effect on the dependent variable.
The t-test is a method of determining whether two populations are statistically different from each other, and ANOVA determines whether three or more populations are statistically different from each other.
Learn more about one-way ANOVA here: https://brainly.com/question/24157862
#SPJ4
Chris requires three fifths liter of paint to complete a poster. He has one half liter of paint. Which equation can be used to find the fraction of a liter of paint Chris still needs to complete the poster? (1 point) a the expression with the fractions three fifths minus one half is equal to the expression with the fractions three fifths minus one fifth is equal to the fraction two fifths b the expression with the fractions three fifths minus one half is equal to the expression with the fractions three tenths minus one tenth is equal to the fraction two tenths c the expression with the fractions three fifths minus one half is equal to the expression with the fractions three fifths minus two fifths is equal to the fraction one fifth d the expression with the fractions three fifths minus one half is equal to the expression with the fractions six tenths minus five tenths is equal to the fraction one tenth
Answers
Answer:
d the expression with the fractions three fifths minus one half is equal to the expression with the fractions six tenths minus five tenths is equal to the fraction one tenth
Step-by-step explanation:
Chris requires three fifths liter of paint to complete a poster. He has one half liter of paint. Which equation can be used to find the fraction of a liter of paint Chris still needs to complete the poster?
The fraction of a liter Chris requires to complete his painting is given as:
3/5 liter = 1/2 liter + x
x = 3/5 - 1/2
Lowest Common Denominator = 10
x = 6 - 5/10
x = 1/10
option d is the correct option.( the expression with the fractions three fifths minus one half is equal to the expression with the fractions six tenths minus five tenths is equal to the fraction one tenth)
PLEASE HELP I WILL GIVE BRAINLIEST due today please helpp

Answers
Answer:
Step-by-step explanation:
Jada is putting a border around her rectangular garden. The garden is 12.63 meters long and 3.28 wide. Jada estimates the perimeter of her garden by rounding the length and width to the nearest whole number. What equation shows her estimate?
Answers
Answer: 12.63 rounded to 13 and 3.28 rounded to 3 the perimeter is 32
Step-by-step explanation: If you need any more help Im
usually available between 9a.m - 3:50 pm
Answer
Part A: C
Part B: B
Part C: 15.91
Step-by-step explanation:
first round the 2 numbers by the tenths. Then round the numbers by ones(whohle number). After that, since ther are four sides we do 12.63 + 3.28 + 12.63 + 3.28 which equals to 15.91.
Please Help
2 and 23/75 as a percentage
Answers
Answer:230.666667%
Step-by-step explanation: Convert the fraction to a decimal: Divide the numerator by the denominator
Add this decimal number to the whole number part of the mixed number
A mixed number is a whole number plus a fraction. To find the decimal form of a fraction just divide the numerator by the denominator using a calculator or long division. Then add the decimal number to the whole number.
find a polynomial function with integer coefficients that has the given zeros
Answers
To find a polynomial function with integer coefficients that has the given zeros, we can use the factor theorem and the zero-product property. Therefore, the function is f(x) = (x + 2)(x - 1)(x - 3) = x^3 - 2x^2 - 5x + 6 .
In summary, to find a polynomial function with integer coefficients that has the given zeros, we can use the factor theorem and the zero-product property to obtain the factors of the polynomial, and then multiply them together to get the polynomial function.
For example, suppose we want to find a polynomial function with integer coefficients that has zeros at -2, 1, and 3. We can start by using the factor theorem to obtain the factors of the polynomial:
(x + 2)(x - 1)(x - 3)
We can then multiply these factors together to obtain the polynomial function:
f(x) = (x + 2)(x - 1)(x - 3) = x^3 - 2x^2 - 5x + 6
This is a polynomial function with integer coefficients that has zeros at -2, 1, and 3. Note that we can also check this by using the zero-product property, which states that if a product of factors is equal to zero, then at least one of the factors must be zero. We can plug in each of the zeros (-2, 1, and 3) into the polynomial function and verify that the result is zero, which confirms that these are indeed the zeros of the polynomial.
To learn more about polynomial click here, brainly.com/question/11536910
#SPJ11
a red mustang is a(n) _____ of the car class.
Answers
INSTANCE.
In my opinion a red mustang is an instance of the car class.
You can learn more about this through the link below:
https://brainly.com/question/22143309#SPJ4
I need help please with 6, 7 and 8
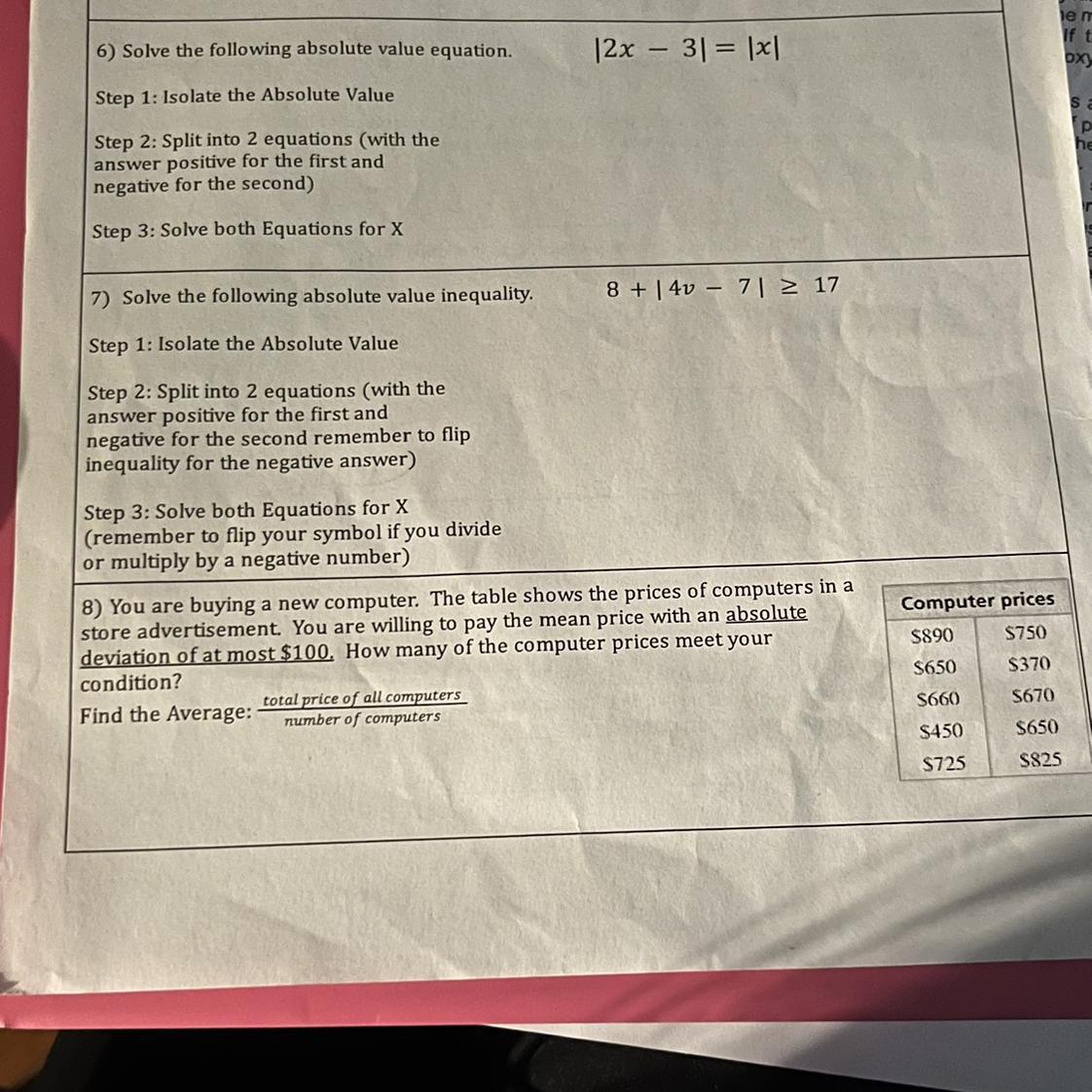
Answers
6. Solution for |2x-3| = |x| are x = 1,3
7. Solution for 8 + |4v - 7| ≥ 17 is,
v ≥ 4 , v ≤ -1/2
8. Number of computer meets in the condition = 6
Average:
Average of given number is given by sum of all numbers divided by total number of numbers.
6. |2x-3| = |x|
Case I :
when x<0
|2x-3| = |x|
-(2x-3) = -x
-2x + 3 = -x
2x - x = 3
x = 3 ( Not possible as x<0 )
No solution in this case.
Case II :
when 0< x< 3/2
|2x-3| = |x|
-(2x -3) = x
-2x + 3 = x
2x +x = 3
3x = 3
x =1 (Valid solution)
Case III :
when x>3/2
|2x-3| = |x|
2x-3 = x
2x -x =3
x = 3 (Valid solution)
Thus solution for |2x-3| = |x| are x = 1,3
7.
8 + |4v - 7| ≥ 17
|4v - 7| ≥ 17 -8
|4v - 7| ≥ 9
⇒ 4v - 7 ≥ 9
4v ≥ 9+7
v ≥ 4
and,
4v - 7 ≤ - 9
4v ≤ -2
v ≤ -1/2
Thus solution for 8 + |4v - 7| ≥ 17 is,
v ≥ 4 , v ≤ -1/2
8.
We know,
Average of quantity = sum of quantity / Number of quantity
For the given question,
Sum of price of all computer = $ (890+650+ 660+450+ 725+750+370+670+650+825)
= $ 6640
and Number of computer = 10
Average price of computer = $ 6640/10
= $ 664
Absolute deviation is $100
So i can pay price between
($ 664 - $100) to ($ 664 + $100)
that is $564 to $764
Number of computer meets in this condition = 6
To learn more about Average visit:
https://brainly.com/question/16956746
#SPJ9
The equation of line EF is y = 1 over 2x + 6. Write an equation of a line parallel to line EF in slope-intercept form that contains point (0, −2).
Answers
Answer:
y = 1/2x -2
Step-by-step explanation:
You want the equation of a line parallel to y = 1/2x +6 that contains the point (0, -2), written in slope-intercept form.
SlopeThe slope of the line you want will be the same as the slope of the line you have. That is because parallel lines have the same slope.
The equation you are given is written in slope-intercept form:
y = mx + b . . . . . . where m is the slope and b is the y-intercept
Comparing this form to the given equation, you see that ...
m = 1/2 . . . . . the slope of the line you want
InterceptThe "intercept" in the "slope-intercept" form is the value of y when x=0. The point you are given, (0, -2), tells you that y = -2 when x = 0. So, the "intercept" in your slope-intercept equation is -2.
EquationThe equation you want is the equation of a line with slope 1/2 and a y-intercept of -2.
y = 1/2x -2

The equation of the line that is parallel to line EF and passes through point (0, -2) is y = (1/2)x - 2.To find an equation of a line that is parallel to line EF and passes through point (0, -2), we need to use the fact that parallel lines have the same slope. The slope of line EF is 1/2, so the slope of the parallel line will also be 1/2.
We can start by using the point-slope form of the equation of a line: y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line. Plugging in m = 1/2 and (x1, y1) = (0, -2), we get:
y - (-2) = (1/2)(x - 0)
Simplifying this equation gives:
y + 2 = (1/2)x
To get this equation in slope-intercept form (y = mx + b), we can isolate y:
y = (1/2)x - 2
So the equation of the line that is parallel to line EF and passes through point (0, -2) is y = (1/2)x - 2. Note that this line intersects the y-axis at y = -2, which is the y-intercept.
learn more about slope here: brainly.com/question/16949303
#SPJ11
HELP PLEASEE
The linear function m = 3a represents the amount m in dollars) of money a customer pays after buying a phone apps. Each customer can buy a maximum of three apps.
1. Identify the independent and dependent variables.
2. Is the domain discrete or continuous? Explain.
Answers
Answer:
1. The independent variable is a and the dependent variable is m
2. The domain is discrete
Step-by-step explanation:
let us explain the difference between discrete or continuous domains
Discrete domain: It is a set of input values that consists of only certain numbers in an interval.
Continuous domain: It is a set of input values that consists of all numbers in an interval.
In the question
m = 3a, where
m is the amount of money m in dollars
Each customer can buy a maximum of three apps
1.
∵ m is depended on the value of 3a
∴ m is the dependent variable ⇒ (output)
∴ a is the independent variable ⇒ (input)
∴ The independent variable is a and the dependent variable is m
2.
∵ The domain the input values
∴ Each customer can buy a maximum of three apps
→ That means it is a certain numbers
∴ The domain is discrete
Which figure best demonstrates the setup for the box method of finding the area of a triangle?.
Answers
The figure that best demonstrates the setup for the box method of finding the area of a triangle is a rectangle divided into two triangles with a diagonal line.
The box method is a visual representation that helps find the area of a triangle by dividing it into two smaller triangles that can be rearranged to form a rectangle. To set up the box method, start with a rectangle and draw a diagonal line from one corner to the opposite corner, dividing the rectangle into two triangles. The base and height of each triangle are labeled, and the total area of the rectangle is equal to the sum of the areas of the two triangles. By using this method, the area of a triangle can be easily calculated by multiplying the base and height and then dividing by two. The box method provides a visual and intuitive approach to understanding the formula for finding the area of a triangle
Learn more about diagonal line here
brainly.com/question/1045307
#SPJ11
Exercise 3.3.7: Prove Corollary 3.3.12: Suppose f: [a,b] R is a continuous function. Prove that the direct image ([a,b]) is a closed and bounded interval or a single number. Exercise 3.3.10: Suppose f: 10.1] → [0,1] is continuous. Show that f has a fixed point, in other words, show that there exists an x € (0.1) such that f(x) = x.
Answers
Combining the above results, we have shown that the direct image f([a, b]) of a continuous function f : [a, b] → R is a closed and bounded interval or a single number.
To prove that the direct image f([a, b]) of a continuous function f : [a, b] → R is a closed and bounded interval or a single number, we need to show two things:
The direct image f([a, b]) is a closed set.
The direct image f([a, b]) is a bounded set.
Let's prove each of these statements:
The direct image f([a, b]) is a closed set:
To show that f([a, b]) is closed, we need to prove that it contains all its limit points.
Let y be a limit point of f([a, b]). This means that there exists a sequence (yₙ) in f([a, b]) such that yₙ → y as n approaches infinity.
Since (yₙ) is a sequence in f([a, b]), there exists a sequence (xₙ) in [a, b] such that f(xₙ) = yₙ.
Since [a, b] is a closed and bounded interval, the sequence (xₙ) has a subsequence (xₙₖ) that converges to some x ∈ [a, b] (by the Bolzano-Weierstrass theorem).
Since f is continuous, we have f(xₙₖ) → f(x) as k approaches infinity. But f(xₙₖ) = yₙₖ, and since yₙₖ → y, we have f(xₙₖ) → y as k approaches infinity.
Therefore, we have shown that for any limit point y of f([a, b]), there exists a corresponding point x in [a, b] such that f(x) = y. Hence, y is in f([a, b]), and f([a, b]) contains all its limit points. Thus, f([a, b]) is a closed set.
The direct image f([a, b]) is a bounded set:
Since [a, b] is a closed and bounded interval, the continuous function f([a, b]) is also bounded by the Extreme Value Theorem. In other words, there exist M, m ∈ R such that for all x ∈ [a, b], m ≤ f(x) ≤ M.
Therefore, f([a, b]) is a bounded set.
Therefore, Combining the above results, we have shown that the direct image f([a, b]) of a continuous function f : [a, b] → R is a closed and bounded interval or a single number.
To know more about direct image check the below link:
https://brainly.com/question/30769577
#SPJ4
Incomplete question:
Suppose that f : [a, b] → R is a continuous function. Prove that the direct image f ([a, b]) is a closed and bounded interval or a single number.
The cost of 2 kg of mushrooms and 2.5 kg of turnips is £8.55. The cost of 3 kg of mushrooms and 4 kg of turnips is £13.10. Work out the cost of a) 1 kg of turnips. b) 1 kg of mushrooms.

Answers
(a) The cost of 1 kg of turnips is £71.95, and
(b) The cost of 1 kg of mushrooms is £89.50.
To solve this problem, let's assign variables to the unknowns.
Let the cost of 1 kg of mushrooms be 'm' and the cost of 1 kg of turnips be 't'.
According to the given information:
Equation 1: 2m + 2.5t = 8.55
Equation 2: 3m + 4t = 13.10
We can solve these equations simultaneously to find the values of 'm' and 't'.
To eliminate the decimals, let's multiply both sides of Equation 1 by 10 and Equation 2 by 20:
20m + 25t = 85.50
60m + 80t = 262.00
Now, let's multiply Equation 1 by 12 and subtract Equation 2 multiplied by 5:
240m + 300t - (60m + 80t) = 1026.00 - 1310.00
180m + 220t = -284.00
We have a new equation:
180m + 220t = -284.00 ---(3)
Next, let's multiply Equation 1 by 18 and subtract Equation 2 multiplied by 4:
36m + 45t - (60m + 80t) = 154.35 - 524.00
-24m - 35t = -369.65
We have another new equation:
-24m - 35t = -369.65 ---(4)
Now, we have a system of linear equations with two variables. Let's solve this system by eliminating one variable.
Multiply Equation 3 by 35 and Equation 4 by 220:
6300m + 7700t = -9940.00 ---(5)
-5280m - 7700t = -81323.00 ---(6)
Adding Equations 5 and 6, we eliminate 't':
1020m = -91263.00
m = -91263.00/1020
m = -89.50
Substituting the value of 'm' back into Equation 3:
180(-89.50) + 220t = -284.00
-16110 + 220t = -284.00
220t = -284.00 + 16110
220t = 15826.00
t = 15826.00/220
t = 71.95.
For similar question on linear equations.
https://brainly.com/question/25858757
#SPJ11
TRUE / FALSE. roofs having a span of 40 feet or less and a pitch of ? or greater, and roofs having a span of more than 40 feet and a pitch of 1/4 or greater are considered to be pitched.
Answers
The statement is true. Roofs with a span of 40 feet or less and a pitch of ? or greater, as well as roofs with a span of more than 40 feet and a pitch of 1/4 or greater, are considered to be pitched.
In roofing terminology, the pitch refers to the steepness or slope of a roof. A pitched roof has a slope that allows water to drain efficiently. The pitch is typically measured as a ratio of vertical rise to horizontal span.
According to the statement, roofs with a span of 40 feet or less and a pitch of ? (undefined or not specified) or greater are considered to be pitched. This means that as long as the roof has a certain degree of slope or steepness, it is categorized as a pitched roof.
Similarly, roofs with a span of more than 40 feet and a pitch of 1/4 or greater are also considered to be pitched. In this case, the requirement for a minimum pitch of 1/4 (or a steeper slope) applies to roofs with a larger span.
The classification of roofs as pitched or not pitched depends on their span and the minimum pitch requirements specified. Roofs that meet the specified criteria for span and pitch are considered to be pitched roofs.
Learn more about roofing terminology here:
https://brainly.com/question/31918563
#SPJ11
Can anyone solve these? Pre-Calc BC

Answers
Answer:
79. [7, 12], [1, 6]
80. The total revenue in the month of May is $11,575
81. The total revenue in the month of November is $4,630
82. Values obtained from the model and actual values are almost the same, so the given model is precise.
Step-by-step explanation:
79.
The domain of a function is the set of x-values that we are allowed to plug into our function.
The graph below represents f(x),
the graph that the domain of the first part of the function is [7,12]. The domain of the second part of the function is [1,6]. The solution is:
\(f(x)=\left \{ {{-1.97x+26.3, 7 \leq x \leq 12} \atop {0.505x^{2} -1.47x+6.3, 1 \leq x \leq 6}} \right. \\\\\)
80.
1 ≤ 5 ≤ 6, so we'll plug the value 5 in the function f(x) = 0.505x² - 1.47x + 6.3
f(x) = y = (0.505)(5)² - 1.47(5) + 6.3
f(x) = y = (0.505)(25) - 7.35 + 6.3
f(x) = y = 12.625 - 1.05
f(x) = y = 11.575
For x=5 which represents 5th month May, we got y=11.575
Therefore, the total revenue in the month of May is 11.575 thousands dollars, ie 11,575 dollars.
81.
7 ≤ 11 ≤ 12, so we'll plug the value 11 in the function f(x) = -1.97x + 26.3
f(x) = − 1.97(11) + 26.3
= − 21.67 + 26.3
= 4.630.
For x=11 which represents 11th month November, we got y=4.630 Therefore, the total revenue in the month of November is 4.630 thousands dollars, 4,630 dollars.
82.
Values obtained from the model are y=11.575 (x=5) and y=4.630 (x=11). Actual data values are y=11.5 (x=5) and y=4.4 (x=11)
By comparing these values, we can conclude that the model is precise, because these values are almost the same.
Learn more about Piecewise Functions here: https://brainly.com/question/13882670

What function is a vertical shift of f(x) = x?
A) g(x) = 3f(x)
B) g(x) = f(x - 3)
C) g(x) = f(x) + 4
D) g(x) = 1/2 f(x)
Answers
Answer:
C) g(x) = f(x) + 4
Step-by-step explanation:
A vertical shift is where you shift, slide or translate the whole graph up or down (on a graph) The way this shows up in the equation is just a number tacked on to the end of the equation. A +anumber (like the +4 in the answer) slides the function UP four units. A
-anumber would slide the function DOWN instead.
As for the other answers:
A) the 3multiplied in front is a vertical STRETCH.
D) the 1/2 multiplied in front is a vertical shrink (smash)
B) The -3 in close tight with the x is a horizontal shift(slide, translate) It is a RIGHT shift. A +anumber would be a LEFT shift. Horizontal shift seem kind of backwards. + goes LEFT and - goes RIGHT.
Show that cos 30 degree + sin 60 degree / 1+ sin 30 degree + cos 60 degree is equal to cos 30 degree
Answers
Answer:
cos 30
Step-by-step explanation:
cos 30 + sin 60 / 1+ sin 30 + cos 60
= root3 / 2 + root3/2 / 1 + 1/2 + 1/2
= root 6/2 by 4/2
= root 6/4
= root 3/2
= cos 30
Answer:
cos 30
Step-by-step explanation:
Are { 13 x (25+ 15) } and { (13x25) + (13x25) } equal? What do you come to know from the result?
Answers
Yes, { 13 x (25+ 15) } and { (13x25) + (13x25) } are equal.
In mathematics, a binary operation is commutative if changing the order of the operands does not change the result. It is a fundamental property of many binary operations, and many mathematical proofs depend on it.
{ 13 x (25+ 15) } = 13 x 40 = 520
{ (13x25) + (13x25) } = 325 + 325 = 650
From the result, we can conclude that both expressions yield the same value, which is 520. This demonstrates the commutative property of multiplication, which states that the order of multiplication does not affect the result.
Know more about expressions here:
https://brainly.com/question/14083225
#SPJ11
Rachel is going on a vacation. She is trying to figure out how much
money to bring along. She plans to spend $120 on hotels, $45 on
gas for her car, and $65 on food. She is also going to go to an
amusement park twice. Tickets to the amusement park cost $30
per day
If she wants to have $30 left in her wallet after the trip is over, how
much money should she bring?
Answers
Answer:
$320
Step-by-step explanation:
First, add up all of the money she expects to spend:
120 + 45 + 65 + 60
= 290
Then, add 30 to this, since she wants to have $30 left:
290 + 30
= 320
So, she should bring $320
Given m || n, find the value of x and y.
X =
(7x+18)º
y =
Do
(9x-14)°
m

Answers
Answer:
x = 16y = 130Step-by-step explanation:
You want the values of x and y when corresponding angles at parallel lines are marked (9x -14)° and (7x +18)°, and a vertical angle to those is marked y°.
Corresponding anglesCorresponding angles where a transversal crosses parallel lines are congruent:
(9x -14)° = (7x +18)°
2x = 32 . . . . . . . . . . divide by °, add 14-7x
x = 16
Then these angles are ...
(9(16) -14)° = (144 -14)° = 130°
Vertical anglesVertical angles are congruent, so ...
y° = 130°
y = 130
1. a set within a set; all the elements of one set are also contained in another set
Answers
Answer:
"subset"
Step-by-step explanation:
The "set within a set" is called a "subset."
You’re given two side lengths of 3 centimeters and 5 centimeters. Which measurement can you use for the length of the third side to construct a valid triangle?
8 centimeters
6 centimeters
4 centimeters
10 centimeters
2 centimeters
Answers
Answer:
6 centimeters and 4 centimeters
Step-by-step explanation:
According to the Triangle Inequality, the sum of the lengths of any two sides of a triangle has to be greater than the third side. The answer choices that satisfy the Triangle Inequality is 6 centimeters and 4 centimeters.
Pomnoži dva broja:
5*5=
Answers
Answer:
5 * 5 = 25
Step-by-step explanation:
hope this helps you!
the manager of a grocery store has selected a random sample of 100 customers. the average length of time it took these 100 customers to check out was 8.0 minutes. it is known that the standard deviation of the checkout time is 2 minutes. with a 0.95 probability, the sample mean will provide a margin of error of . group of answer choices A. .392 B. 1.96 C. .95 D. .2
Answers
The margin of error is A. 0.392 minutes.
Which choice represents the margin of error with a 0.95 probability?The margin of error is a measure of the precision or uncertainty associated with estimating a population parameter based on a sample.
In this case, we are interested in estimating the population mean checkout time based on the sample mean checkout time.
To calculate the margin of error, we use the standard deviation of the sample mean, which is also known as the standard error. The formula for the margin of error is:
Margin of Error = Critical Value * Standard Error
The critical value is determined based on the desired level of confidence. In this case, we want a 95% confidence level, which corresponds to a critical value of 1.96 for a standard normal distribution.
The standard error is calculated by dividing the standard deviation of the population (known in this case) by the square root of the sample size.
In this scenario, the standard deviation of the checkout time is given as 2 minutes, and the sample size is 100.
Therefore, the margin of error is 1.96 * (2 / \(\sqrt(100)\)) = 1.96 * 0.2 = 0.392.
This means that with 95% confidence, the sample mean checkout time of 8.0 minutes is expected to have a margin of error of 0.392 minutes.
Learn more about confidence intervals and margin of error
brainly.com/question/17070397
#SPJ11
What are the lengths of sides kn and nm of the kite? kn = units nm = units
Answers
the lengths of sides KN and NM of the kite KN = 15 units and NM = 20 units
What is quadrilateral?
Quadrilaterals are typically four-sided closed shapes, and there are numerous varieties of them. Square, rectangle, rhombus, kite, and trapezoid are the most typical shapes. Four closed sides make up a quadrilateral. Quadrilaterals are the following figures: a four-sided figure. The form features a single set of parallel sides and no right angles.
Given that KLMN is a kite
According to the given figure KN = KL and LM=NM
we have the equation y+10=2y+5
y = 5
So KN = KL =15
On putting x =7 for LM and MN we get
LM = MN = 20
Hence, the lengths of sides KN and NM of the kite KN = 15 units and NM = 20 units
Learn more about Quadrilaterals, by the following link.
brainly.com/question/23935806
#SPJ4
6 sprinklers are shown on gridlines. each sprinkler has a diameter of 6 feet. the irrigation design for a new backyard is shown with six sprinkler heads and two walkways. which statement is true of this design? the area covered by each sprinkler head is approximately 113.10 ft2. the area covered by all the sprinkler heads together is approximately 169.64 ft2. every square foot of the yard will be watered with this design. the sprinklers would cover more area if they were in a straight line.
Answers
The area covered by all the sprinkler heads together is approximately 169.64 ft2.
We have given
6 sprinklers are shown on gridlines.
Each sprinkler has a diameter of 6 feet.
The irrigation design for a new backyard is shown with six sprinkler heads and two walkways.
One sprinkler has a diameter of 6 feet.
So the radius is 3.
The area for one sprinkler is given by,
What is the formula for the area of the circle?
\(A=\pi r^{2}\)
\(A=3.14\times 3^2\\A=28.26\)
So the area covered by one sprinkler is 28.26 feet ^2.
For six sprinkler is given by
\(=6\times 28.26\)
=169.64 ft^2
The answer is B.
The area covered by all the sprinkler heads together is approximately 169.64 ft2.
To learn more about the area visit:
https://brainly.com/question/3948796
Answer:
b
Step-by-step explanation:
60% of the student in Mr. Reed's class wear glasses. if 15 students wear glasses , how many student are in Mr. Reeds class?
Answers
Answer:
25
Step-by-step explanation:
If times 4 with 15 to get 60%
Then divide 100% with 4 to get 25 students
7. Matéd is 41 years old. Mateo's brother
is younger than him. Mateo writes
the inequality b > 41, where b equals
the number of years, to represent
his brother's age. Is Mateo correct?
Explain.