Find the other endpoint of the line segment with the given endpoint and midpoint
Endpoint: (3,5)
Midpoint: (7, -2)
Answers
Answer:
The other endpoint is (11,-9)
Step-by-step explanation:
Midpoint Formula
\(\large\boxed{(\frac{x_1+x_2}{2},\frac{y_1+y_2}{2} )}\)
We already know the another endpoint which is (3,5). We substitute x = 3 and y = 5 in the formula. You can substitute in x1, x2 or y1, y2. I'll substitute in first x-term and first y-term instead.
\(\large{\begin{cases} \frac{3+x}{2}=7\\\frac{5+y}{2}=-2 \end{cases}}\)
Because sum of two x-coordinate (along with y-coordinate) divided by two must equal to the midpoint (Let's say that you get the value of midpoint when using midpoint formula.)
Solve the equation for both terms.
\(\large{\begin{cases} \frac{3+x}{2}=7\\\frac{5+y}{2}=-2 \end{cases}}\)
Cancel the denominator by multiplying the whole equation by 2.
\(\large{\begin{cases} \frac{3+x}{2}(2)=7(2)\\\frac{5+y}{2}(2)=-2(2) \end{cases}}\\\large{\begin{cases} 3+x=14\\ 5+y=-4 \end{cases}}\)
Isolate x-term and y-term.
\(\end{cases}}\\\large{\begin{cases} 3+x=14\\ 5+y=-4 \end{cases}}\\\end{cases}}\\\large{\begin{cases} x=14-3\\ y=-4-5 \end{cases}}\\\end{cases}}\\\large{\begin{cases} x=11\\ y=-9 \end{cases}}\)
Therefore, when x = 11, y = -9. We can write in ordered pair as (11,-9). The ordered pair (11,-9) is our other endpoint of the line segment. This can be proved by using the distance formula between midpoint and endpoints.
Note: The distance of (3,5) and (7,-2) must equal to the distance of (11,-9) and (7,-2)
Related Questions
write up four different equations for the line passing through these points
Answers
the answer is: 1° equation
\(-3x\text{ + 4y = 12}\)2° equation
\(y\text{ = }0.75x\text{ + 3}\)3° equation
\(X\text{ = }(-4,\text{ 0) + }\lambda(4,3)\)and the 4° equation
\(-3x\text{ + 4y - 12}=\text{ 0}\)You randomly choose one shirt from the shelves.
Find the probability of the event.
The probability of choosing a red shirt is

Answers
Answer:
the probaility of choosing a red shirt is 1
You decide to buy a laptop for $750. You make a down payment of $150 and the company will allow you to pay off the remainder in 12 payments over the next year at 13.5% interest with a finance charge of $25. Make a table of all payments over the next year including the initial down payment and finance charge. Show the difference between paying in cash for the laptop and this financial arrangement. Show all equations and calculations you used. Explain the benefits and downsides of financing your laptop and describe when you would advise someone to finance this kind of purchase. Upload your payment table and answers below.
Answers
Total amount paid over the next year including the initial down payment and finance charge = $856
The difference between paying in cash for the laptop and this financial arrangement = $106
Cost of laptop=$750
Down payment=$150
Finance charges = $25
Remaining amount =$600
Amount for 1 month including 13.5 % interest=600/12*13.5/100+600/12
=$56.75
Total amount paid over the next year including the initial down payment and finance charge = 56.75*12+150+25 = $ 856
The difference between paying in cash for the laptop and this financial arrangement = $106
Benefits of financing laptop:
A costly laptop can be yours without having to pay in full.
Bank loans and conventional credit card services are not required.
Numerous retailers provide rewards, flexible payment plans, zero-interest rates, and other benefits.
Downsides of financing laptop:
It's dangerous to borrow money because late payments might result in fees and excessive interest rates.
A credit score may suffer if there is no interest charged.
Learn more about financing here:
https://brainly.com/question/26011426
#SPJ1
15/3 = 45/x
solve for x
Answers
Answer:
15/3=45/x
We move all terms to the left:
15/3-(45/x)=0
Domain of the equation: x)!=0
x!=0/1
x!=0
x∈R
We add all the numbers together, and all the variables
-(+45/x)+15/3=0
We add all the numbers together, and all the variables
-(+45/x)+5=0
We get rid of parentheses
-45/x+5=0
We multiply all the terms by the denominator
5*x-45=0
We add all the numbers together, and all the variables
5x-45=0
We move all terms containing x to the left, all other terms to the right
5x=45
x=45/5
x=9
Step-by-step explanation:
When a ball is thrown or kicked, the path it travels is shaped like a parabola. Suppose a football is kicked from ground level, reaches a maximum height of 25 feet, and hits the ground 100 feet from where it was kicked. Assuming that the ball was kicked at the origin, write an equation of the parabola that models the flight of the ball.
Answers
The equation of the parabola that models the flight of the ball is y = ax^2 + 25, where a can be any non-zero real number.
The equation of the parabola that models the flight of the ball can be expressed in the standard form: y = ax^2 + bx + c.
Since the ball is kicked from the origin, the equation simplifies to y = ax^2 + c.
To find the values of a and c, we can use the given information. The ball reaches a maximum height of 25 feet, which means the vertex of the parabola is at the point (0, 25). This gives us c = 25.
Now we need to determine the value of a. Since the maximum height occurs at the vertex, the x-coordinate of the vertex is 0. Additionally, we know that the ball hits the ground 100 feet from where it was kicked. The x-coordinate at that point is 100. Therefore, we can use the vertex form of the parabola equation, which is x = -b/2a, to find a.
Substituting the known values, we have 0 = -b/2a, which implies b = 0. Therefore, a can be any non-zero value.
Know more about parabola here:
https://brainly.com/question/11911877
#SPJ11
Which value from the set {0,1,17,72} makes the equation n + 8 = 9 true?
Answers
answer: 1
step-by-step explanation: n + 8 = 9
therefore 9-8 = 1
The solution to the equation n+ 8 = 9 is 1 from the set {0,1,17,72} n = 1 satisfy the provided equation.
What is a function?It is defined as a special type of relationship, and they have a predefined domain and range according to the function every value in the domain is related to exactly one value in the range.
We have a linear function in terms of n:
n + 8 = 9
Subtract 8 both side:
n + 8 – 8 = 9 – 8
n = 1
From the set, we can see the element 1 is the solution to the given equation.
Thus, the solution to the equation n+ 8 = 9 is 1 from the set {0,1,17,72} n = 1 satisfy the provided equation.
Learn more about the function here:
brainly.com/question/5245372
#SPJ2
у is directly proportional to the square route of x
If y = 32 when x = 64 find,
x when y = 48
Answers
Sorry if it’s wrong
The weight of a bag of potatoes is 25 kg, correct to the nearest kg. (a) write down the smallest possible weight of the bag of potatoes.
Answers
a) Smallest possible weight of the bag of potatoes is 24.5 kg.
What is Rounding number?
Rounding is the process of simplifying a number while maintaining a value that is near to the original.
Given that;
The weight of the potato is 25 kg.
Now,
Since, The weight of the potato is 25 kg.
For rounding number;
24.5 ≈ 25
Thus,
a) Smallest possible weight of the bag of potatoes is 24.5 kg.
To know more about Rounding number visit,
https://brainly.com/question/24673197
#SPJ4
Given j(-8,-5) and l(6,-11) if k(r-4,2s) is the midpoint of jl which correctly gives the values of r and s?
Answers
The values of r and s is -1 and -4 respectively.
Given that:-
coordinates of j are (-8,-5)
coordinates of l are (6,-11)
coordinates of k are (r-4,2s)
Also, k is the mid-point of jl.
We have to find the values of r and s.
We know that, for (x,y) and (z,w), the mid-point is ((x+z)/2, (y+w)/2).
Here,
(x,y) = (-8.-5)
(z,w) = (6,-11)
(r-4,2s) = ((x+z)/2,(y+w)/2)
Hence,
(r-4,2s) = ((-8+6)/2,(-5-11)/2)
(r-4,2s) = (-1,-8)
Comparing the coordinates, we get:-
r - 4 = -1
r = -1+4 =3
2s = -8
s =-8/2 = -4
To learn more about mid-point, here:-
https://brainly.com/question/11302835
#SPJ4
Given mn, find the value of x.
kt
(3x-5)
m
→
(2x-25)
Please help me

Answers
Answer:
I think this is the correct solution

on a coordinate plane, a curved line with an upward arc, labeled g of x, crosses the x-axis at (negative 2, 0), and the y-axis at (0, 4). a straight horizontal line, labeled f of x, crosses the y-axis at (0, 4). which represents where f(x)
Answers
Therefore, the straight horizontal line labeled f(x) represents where f(x) is equal to 4.
Based on the given information, the function f(x) is represented by the straight horizontal line that crosses the y-axis at (0, 4). The point (0, 4) on the y-axis indicates that when x is 0, the value of f(x) is 4. Since the line is horizontal, it maintains a constant value of 4 for all values of x.
To know more about straight horizontal line,
https://brainly.com/question/16816396
#SPJ11
Solve the following story problem.
The perimeter of a rectangle is 60 inches. The length is four times the
width.
What are the length and width of the rectangle?
Define your variables.
X =
Equation 1
y =
Equation 2
What is the length?
what is the width?
Answers
Answer:
The perimeter of a rectangle is equal to 2L + 2W, where L = the length of the rectangle and W = the width of the rectangle. So, one equation is
2L + 2W = 60
From the info given in the problem, we also know that L = 4W. Therefore, we can use the Substitution Method to re-write the first equation as:
4W + 2W = 60
You should be able to solve this equation for W. Once you find W, you can then calculate L.
Step-by-step explanation:
Can someone please help me with both these questions? You will get 20pts

Answers
Answer:
#1) -3 feet
#2) 5hours
Step-by-step explanation:
#1) you need to find the rate of change- feet per minute
To find the rate, divide -12 feet by 4 minutes (the twelve in negative because it decreases, almost like subtraction)
-12/4= -3 feet per minute.
Now, multiply by amount of minutes- -3x1= -3 feet in 1 minute
#2) To find the maount of degrees each hour,
divide 10 degrees by 2 degrees per hour.
10/2=5 hours
Hope this helps :)- please give me brainliest!!
I’m not sure I need help

Answers
Answer:
D) \(1 < x\leq 4\)
Step-by-step explanation:
1 is not included, but 4 is included, so we can say \(1 < x\leq 4\)
Finding the side length of a cube from its Volume in liters A technical machinist is asked to build a cubical steel tank that will hold 275 L of water. Calculate in meters the smallest possible inside length of the tank. Round your answer to the nearest 0.001 m. X 5 ?
Answers
The smallest possible inside length of the cubical steel tank that can hold 275 liters of water is approximately 0.640 meters.
The side length of the cube is found by converting the volume of water from liters to cubic meters, as the unit of measurement for the side length is meters.
Given that the volume of water is 275 liters, we convert it to cubic meters by dividing it by 1000 (1 cubic meter = 1000 liters):
275 liters / 1000 = 0.275 cubic meters
Since a cube has equal side lengths, we find the side length by taking the cube root of the volume. In this case, we find the cube root of 0.275 cubic meters:
∛(0.275) ≈ 0.640
Rounded to the nearest 0.001 meters, the smallest possible inside length of the tank is approximately 0.640 meters.
To know more about smallest possible inside length, refer to the link :
https://brainly.com/question/17304098#
#SPJ11
If 40% of x + 20% of x + 1/10 = 75% of x, then find the value of x. Please give answers with steps
Answers
Answer:
x = 13/12
Step-by-step explanation:
explain on image

What is the equation in standard form of the line that passes through the point (6,-1) and is parallel to the line represented by 8x+3y=15.
Answers
The equation of line that passes through the point (6, -1) & is parallel to the line represented by 8x + 3y = 15, in standard form is: 8x + 3y = 45.
Equation of a line in standard form is:
Ax + By = C
where A, B & C are constants.
As the required line is parallel to the given line, that is 8x + 3y = 15, the slope of the required line will be same as that of the given line.
Slope intercept form of a line is:
y = mx + b
where, m is slope of the line, b is a constant.
Re-writing the equation of the given line in slope-intercept form:
⇒ 3y = -8x + 15
⇒ y = (-8/3)x + 5
⇒ slope = m = -8/3
Now as the required line is parallel to the given line, hence its slope-intercept form will be
y = (-8/3)x + b
As the line passes through the point (6, -1),
⇒ -1 = (-8/3)×6 + b
⇒ b = 15
Hence, the slope-intercept form of the required line is
y = (-8/3)x + 15
Re-writing it in standard form, the equation comes out to be
8x + 3y = 45
To learn more about standard form https://brainly.com/question/12452575
#SPJ4
Please help me with this question

Answers
Answer:
Either 36.35 or 16.00173
Step-by-step explanation:
the reason im saying its either is due to not remembering whether do divide by inverse cosine to get on other side of equal sign or to just divide the cosine as is.
IF you are supposed to use inverse cosine:
16.00173
IF NOT:
36.35182
The total number of students in a community class is 140. Of these students, 60 are male.
Don't forget to write all fraction answers in simplest form!!!
a) Give the ratio of males to females as a fraction.
b) Give the ratio of females to males as a fraction.
c) Give the ratio of males to total number of students as a fraction.
d) Give the ratio of total number of students to females as a fraction.
Answers
Step-by-step explanation:
a fraction is x/y.
so, now we need to put the numbers in as defined. yes, and then simplify.
140 students, 60 are male. that means 140 - 60 = 80 are female.
a)
60/80 = 6/8 = 3/4
b)
80/60 = 8/6 = 4/3
c)
60/140 = 6/14 = 3/7
d)
140/80 = 14/8 = 7/4
Graph a right triangle with the two points forming the hypotenuse. Using the sides, find the distance between the two points, to the nearest tenth (if necessary ). (-1,2) and (2,5)
Answers
The length of the two points (-1 , 2) and (2 , 5) forming a hypotenuse to a right triangle is 4.2 units.
Here we have been given 2 points (-1,2) and (2,5).
Two find a right triangle with a hypotenuse from these two points, we will first plot these points in the Cartesian plane.
Let A be (-1 , 2) and B be (2 , 5)
After this, we will join these points to make the hypotenuse AB.
The best way to proceed after this is to trace out a line from point B parallel to the Y-axis and a line from point B parallel to the X axus.
The intersection of these 2 points will obviosly be a right angle.
Hence, we get out right triangle ABC where C is (2 , 2)
Now we need to use sides AC and BC to find the distance between A and B.
This can be done using the Pythogaras Theorem which states
the sum of squares of perpendicular and base = square of the hypotenuse
Hence we get
AC² + BC² = AB²
AC is clearly the difference between the x coordinates of A and C which gives us
2 - (-1) = 2 + 1 = 3
Similarly, BC is the difference between the Y-coordinates of B and C which is
5 - 2 = 3
Hence
AB² = 3² + 3²
or, AB² = 9 + 9 = 18
or AB = √18
or, AB = 4.2 units.
The length of the two points (-1 , 2) and (2 , 5) is 4.2 units.
To learn more about Co-ordinate Geometry visit
https://brainly.com/question/28974670
#SPJ4

From the top of a tree 20 metres high,the angle of depression at a point on the opposite bank of a river is found to be 30° .Find the breadth of the river.
Answers
Answer:
17.3
Step-by-step explanation:
sin(30)=0.5
0.5*20=100
20*20+x*x=100
x=17.3
Use the given minimum and maximum data entries, and the number of classes, to find the class width, the lower class limits, and the upper class limits. minimum = 8, maximum=79, 7 classes
Answers
The upper class limits are- 29, 39, 49, 59, 69, 79, 89
The lower class limits are- 20, 30, 40, 50, 60, 70, 80
The class midpoints are- 24.5, 24.5, 44.5, 54.5, 64.5, 74.5, 84.5
The class boundaries are- 29.5, 39.5, 49.5, 59.5, 69.5, 79.5
And the number of individuals included in the summary is 84.
Here, we are given the following dataset-
Age (yr) when Frequency
award was won
20-29 27
30-39 32
40-49 15
50-59 3
60-69 5
70-79 1
80-89 1
Upper class limit is the largest data value that can go in a class.
Thus, the upper class limits are- 29, 39, 49, 59, 69, 79, 89
Lower class limit is the smallest data value that can go in a class.
Thus, the lower class limits are- 20, 30, 40, 50, 60, 70, 80
The class midpoint is the average of the upper and lower limits of a class. Class midpoint = (upper limit + lower limit)/ 2
Thus, the class midpoints are- 24.5, 24.5, 44.5, 54.5, 64.5, 74.5, 84.5
Class boundary is the midpoint of the upper class limit of a class and the lower class limit of the previous class.
Thus, the class boundaries are- 29.5, 39.5, 49.5, 59.5, 69.5, 79.5
Frequency gives us the number of individuals/ objects belonging to a particular class.
Thus, the number of individuals included in the summary = 27 + 32 + 15 + 3 + 5 + 1 + 1 = 84
Learn more about frequency distribution here-
https://brainly.in/question/1123422
#SPJ1
complete question:
Identify the lower class limits, upper class limits,
class width, class midpoints, and class boundaries for
the given frequency distribution. Also identify the
number of individuals included in the summary.
Age (yr) when
award was won
20-29
30-39
40-49
50-59
60-69
70-79
80-89
Frequency
27
32
15
3
5
1
1
How many radians is -89 degrees
Answers
Answer:
-1.5533
Step-by-step explanation:
-89 degrees x Pi/180 degrees
=-0.49444444444Pi rad
=-1.5533430342 rad
Znnzbamamaksmsnsnnsnsnsshhsjsjdhdndndhdbndjdbdbd
give the velocity vector for wind blowing at 10 km/hr toward the northeast. (assume north is the positive y-direction.)
Answers
The velocity vector for wind blowing at 10 km/hr toward the northeast can be represented as \((v_x, v_y)\) = (7.071, 7.071) km/hr.
To find the velocity vector for wind blowing at 10 km/hr toward the northeast, we need to break down the velocity into its x and y components. Since the wind is blowing toward the northeast, we can consider it as a combination of motion in the positive x-direction and positive y-direction.
The magnitude of the velocity is given as 10 km/hr. Since the wind is blowing at an angle of 45° with the positive x-axis (northeast direction), we can use trigonometry to determine the x and y components of the velocity. The x-component (\(v_x\)) can be calculated as\(v_x\) = magnitude * cos(angle) = \(10 * \left(\frac{{\sqrt{2}}}{2}\right)\)= 10 * 0.7071 ≈ 7.071 km/hr.
Similarly, the y-component (\(v_y\)) can be calculated as \(v_y\) = magnitude * sin(angle) = \(10 * \left(\frac{{\sqrt{2}}}{2}\right)\) ≈ 7.071 km/hr. Therefore, the velocity vector for wind blowing at 10 km/hr toward the northeast is (\(v_x, v_y\)) = (7.071, 7.071) km/hr.
Learn more about vector here:
https://brainly.com/question/29740341
#SPJ11
HELPPP!!!!
ANSWER ONLY IF YOU KNOW IF THE QUESTION IS CORRECT I'LL GIVE 30 EXTRA POINT (50 point total) EXPLAIN HOW
Given EP = FP and GQ = FQ, what is the perimeter of ΔEFG?

Answers
Answer:
38 units
Step-by-step explanation:
EP = FP , then
4y + 2 = 2x → (1)
GQ = FQ , then
4y + 4 = 3x - 1 ( subtract 4 from both sides )
4y = 3x - 5 → (2)
substitute 4y = 3x - 5 into (1)
3x - 5 + 2 = 2x ( subtract 2x from both sides )
x - 3 = 0 ( add 3 to both sides )
x = 3
substitute x = 3 into (1)
4y + 2 = 2(3) = 6 ( subtract 2 from both sides )
4y = 4 ( divide both sides by 4 )
y = 1
Then
EP = 4y + 2 = 4(1) + 2 = 4 + 2 = 6 = FP
GQ = 4y + 4 = 4(1) + 4 = 4 + 4 = 8 = FQ
PQ is a midsegment and is half the length of EG
PQ = x + 2y = 3 + 2(1) = 3 + 2 = 5 , so
EG = 2 × 5 = 10
Then summing the parts for perimeter (P)
P = 6 + 6 + 8 + 8 + 10 = 38
See image for question.
Please show workings and diagrams.

Answers
Answer:
a) 7 kmb) 298°Step-by-step explanation:
See attached diagram (not to scale).
All given details reflected.
a) Find the measure of XZ using the law of cosines:
y = √(5² + 3² - 2*5*3*cos 135°) = 7 km (rounded)b) Now using the law of sines find the measure of angle X:
7 / sin 135° = 5 / sin X sin X = 5 sin 135° / 7m∠X = arcsin (5 sin 135° / 7 ) = 28° (rounded)The bearing of Z from X is:
270° + 28° = 298°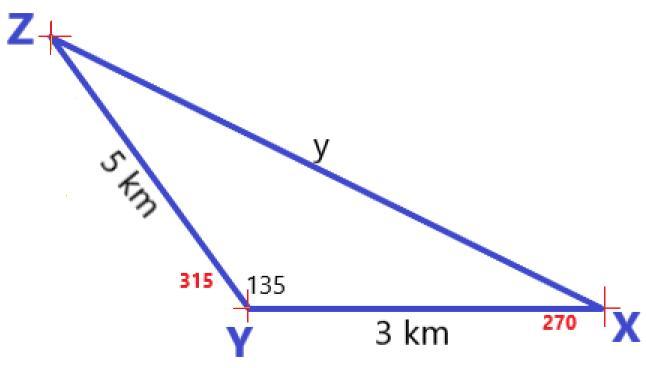
Lucy places five cards that are labeled 1 to 6 face down on the table and mixes them up.
What is the likelihood that her friend Harry will draw an even numbered card?
Answers
as likely as not
If a, b, and c are positive prime numbers, in the equation a - b = c, either b or c must represent which number?
Answers
The value of prime numbers c in the equation is c = 2.
What is prime numbers?
Those greater than one are known as prime numbers. They simply have two variables, the number itself and factor 1.
We have given that a - b = c requires all of the integers to be positive prime numbers,
c can only have one outcome, which is 2.
\(e.g., 13 - 11 = 7 - 5 = 2.\)
Hence in the given prime numbers c in the equation is c = 2.
To learn more about prime numbers visit,
https://brainly.com/question/145452
#SPJ13
What is the average rate of change for [-1,2]? I need a answer please!!!!!!!!!
Answers
Answer: -2
Hope this helps :)
Draw the isosceles triangle shown, divide each leg into eight congruent segments. connect the highest point of one leg with the lowest point of the other leg. then connect the second highest point of one leg to the second lowest point of the other leg. continue this process. write a quadratic function whose graph models the shape that appears

Answers
The equation of the graph or function y=-1/9x²
From the given instruction we obtain a parabola with an x-coordinate equal to the midpoint of I of the endpoints of the base or −6+6/2 =0
And y-coordinate equal to the midpoint of the y-coordinates of the vertex and an endpoint of the base or 4+(−4)/2=0
So the vertex is (0,0)
From the function y=x² above graph is the reflection about x-axis
so we have y=−ax²
So, to find "a" we will put (6,-4) in equation 1 we get
-4=-a(6)²
a=1/9
Hence, the equation of the graph is y=-1/9x²
To learn more on Triangles click:
https://brainly.com/question/2773823
#SPJ1