Find the population in Cherryton if the population in Appleton is people?
Answers
Answer:
The population of Appleton is 175% of the population of Cherryton.
Related Questions
7-1/5-2=10-1/5+-1/5+6-1/5

Answers
Answer: 7x-2=10x+x+6x
Step-by-step explanation:
sub in and solve
or solve the equation
7x-2=17x
10x=-2
x=-2/10
x=-1/5
7x-2=10x+x+6x is true
error error error error
Answers
Answer:
I really like helping people so can you take this app more seriously ..?
Step-by-step explanation:
AB is a diameter in a circle whose centre is the origin where A =(2,0) then B=
Answers
Answer:
(-2,0)
Step-by-step explanation:
\(\lim _{n\to \infty \:}\left(\sqrt[n]{\frac{n^2+1}{n+1}}\right)\)
Please show your work!
Thank you! :)
Answers
Answer:
\( \displaystyle \lim _{n\to \infty }\sqrt[n]{\frac{n^2+1}{n+1}} = \boxed1\)
Step-by-step explanation:
we want to compute the following limit:
\( \displaystyle \lim _{n\to \infty }\sqrt[n]{\frac{n^2+1}{n+1}}\)
well, remember that, for limits to infinity, terms less than the highest degree of the numerator or denominator can be disregarded, hence we can drop 1 which yields:
\( \displaystyle \lim _{n\to \infty }\sqrt[n]{\frac{n^2}{n}}\)
reduce fraction:
\( \displaystyle \lim _{n\to \infty }\sqrt[n]{n}\)
by using limit formula, we acquire:
\( \displaystyle \lim _{n\to \infty }\sqrt[n]{n} = \boxed1\)
and we're done!
Can someone help me please?

Answers
67.5
Step-by-step explanation:
Ann wants to buy a used car and needs to have a down payment of 35%. If the car Ann wants to buy costs $3,000, how much down payment will she need?
Answers
Answer:
Ann will need a down payment of 1050 dollars
Step-by-step explanation:
The answer is 1050 because 35% of 3000 is 1050.
P.S Can I have brainliest?
The speed with which utility companies can resolve problems is very important. GTC, the Georgetown Telephone Company, reports it can resolve customer problems the same day they are reported in 75% of the cases. Suppose the 13 cases reported today are representative of all complaints.How many of the problems would you expect to be resolved today? (Round your answer to 2 decimal places.)What is the standard deviation? (Round your answer to 4 decimal places.)What is the probability 10 of the problems can be resolved today? (Round your answer to 4 decimal places.)What is the probability 10 or 11 of the problems can be resolved today? (Round your answer to 4 decimal places.)What is the probability more than 8 of the problems can be resolved today? (Round your answer to 4 decimal places.)
Answers
The expected number of problems to be resolved today is 10, the standard deviation is 1.3693, the probability that 10 problems can be resolved today is 0.2146, the probability that 10 or 11 problems can be resolved today is 0.3246, and the probability that more than 8 problems can be resolved today is 0.816.
To answer these questions, we will use the binomial distribution since we are dealing with a fixed number of independent trials (the 13 cases reported) with only two possible outcomes (resolved or not resolved).
Let's start with the first question:
Expected number of problems resolved today:
E(X) = n * p = 13 * 0.75 = 9.75
So we would expect about 9.75 problems to be resolved today, but since we cannot have a fraction of a problem, we should round this to 10.
Now let's move on to the second question:
Standard deviation:
σ = sqrt(np(1-p)) = sqrt(13 * 0.75 * 0.25) = 1.3693 (rounded to 4 decimal places).
For the third question:
Probability that 10 of the problems can be resolved today:
P(X=10) = (13 choose 10) * (0.75)^10 * (1-0.75)^(13-10) = 0.2146 (rounded to 4 decimal places).
For the fourth question:
Probability that 10 or 11 of the problems can be resolved today:
\(P(X=10 or X=11) = P(X=10) + P(X=11) = (13 choose 10) * (0.75)^10 * (1-0.75)^(13-10) + (13 choose 11) * (0.75)^11 * (1-0.75)^(13-11) = 0.3246 (rounded to 4 decimal places).\)
For the fifth question:
Probability that more than 8 of the problems can be resolved today:
P(X>8) = 1 - P(X<=8) = 1 - (P(X=0) + P(X=1) + ... + P(X=8))
\(= 1 - ∑(13 choose i) * (0.75)^i * (1-0.75)^(13-i), for i=0 to 8.\)
= 1 - 0.0003 - 0.0033 - 0.0191 - 0.0672 - 0.1562 - 0.2529 - 0.2897 - 0.2072 - 0.0881
= 0.816 (rounded to 4 decimal places).
Therefore, the probability more than 8 of the problems can be resolved today is 0.816 (rounded to 4 decimal places).
To know more about standard deviation, refer here:
https://brainly.com/question/23907081
#SPJ11
2. Megan's aquarium measures 20 inches long, 14 inches wide, and 18 inches high. How many cubic inches of water would it take to completely fill the aquarium?
Answers
It would take 5040 cubic inches of water to completely fill the aquarium.
We know that the formula for the volume of cuboid :
V = length × width × height
Let us assume that l represents the length of the aquarium, w represent the width and h represents the height.
Here, l = 20 inches
w = 14 inches
and h = 18 inches
Using the formula for the volume of cuboid, the volume of aquarium would be,
V = l × w × h
V = 20 × 14 × 18
V = 5040 cu.in.
Therefore, it would take 5040 cu.in. of water.
Learn more about the volume of cuboid here:
https://brainly.com/question/29568631
#SPJ4
A dilation has center (0,0). Find the image of the point A(,-5,5) for the scale factor 1.8
Answers
The image of point A after the dilation is (-9, 9)
How to determine the image point of the dilation?From the question, we have the following parameters that can be used in our computation:
Point A = (-5, 5)
Center of dilation = (0, 0)
Scale factor of dilation = 1.8
From the question, we understand that:
We are to calculate the image of point A after the dilation.
This means that
Image point = Scale factor of the dilation * Point A
Substitute the known values in the above equation, so, we have the following representation
Image point = (-5., 5) * 1.8
Evaluate the products
Image point = (-9, 9)
Hence, the image point is (-9, 9)
Read more about dilation at
brainly.com/question/3457976
#SPJ1
a.) A population of values has a normal distribution with μ=27.5 and σ=71.5. You intend to draw a random sample of size n=180.What is the mean of the distribution of sample means?μ¯x=What is the standard deviation of the distribution of sample means?(Report answer accurate to 2 decimal places.)σ¯x=
Answers
For a population with a normal distribution, the mean (μ) is 27.5 and the standard deviation (σ) is 71.5. When drawing a random sample of size n=180, the mean of the distribution of sample means (μ¯x) is equal to the population mean (μ). Therefore, μ¯x = 27.5.
The standard deviation of the distribution of sample means (σ¯x) is calculated by dividing the population standard deviation (σ) by the square root of the sample size (n).
σ¯x = σ / √n = 71.5 / √180 ≈ 5.33 (rounded to 2 decimal places)
So, the mean of the distribution of sample means is 27.5, and the standard deviation of the distribution of sample means is 5.33.
For more questions like Normal distribution visit the link below:
https://brainly.com/question/29509087
#SPJ11
There are 14 dogs at the dog park on a busy Saturday. 2 of them are springer spaniels.
What is the probability that a randomly selected dog is a springer spaniel?
Write your answer as a fraction or whole number.
Answers
Answer:1/7
Step-by-step explanation: You take the amount that are springer spaniels and you put them with the whole of the dogs in the park 14 so you would have 2/14. You can reduce this fraction to 1/7 by dividing both the top and bottom of the fraction by 2.
!solve this!
−4x + 10 < 26
Answers
Answer:
−4x + 10 < 26
Step 1: Subtract 10 from both sides.
−4x + 10 − 10 < 26 − 10
−4x < 16
Step 2: Divide both sides by -4.
-4x/−4 < 16 /−4
x > −4
Answer:
x > −4
assuming you're solving for x:
solve using pemdas backwards, so basically sadmep :)
first subtract 10, and you end up with the equation -4x < 16.
Next is dividing the -4. Remember dividing by a negative number switches the sign direction, so the < becomes a >. So, your answer would be x > -4
pls help im doing geo and i dont want 2 fail again

Answers
Answer:
Angle DEC = 82
Step-by-step explanation:
All the angles in a triangle added together will always equal 180. Look at the picture for all the work.

Check the picture below.

All above -the line adjustments that do not have corresponding input lines on Schedule 1 ( Form 1040 are indicated as
A. Write -in adjustment
B. Write -in deductions
C. Miscellaneous adjustments
D. Miscellaneous deductions
Answers
The correct option is A. Write-in adjustments All above-the-line adjustments that do not have corresponding input lines on Schedule 1 (Form 1040) are indicated as write-in adjustments.
All above-the-line adjustments that do not have corresponding input lines on Schedule 1 (Form 1040) are referred to as write-in adjustments. Line 36 of Schedule 1 is where all write-in adjustments are reported. You have to provide a brief explanation of the adjustment and the corresponding amount for each write-in adjustment.If the IRS has developed an input line for a particular write-in adjustment, taxpayers must use that input line to report the adjustment.
When writing in adjustments, taxpayers must ensure that the amount they enter is calculated and that they have a reasonable explanation for the adjustment. Taxpayers may be required to provide documentation to support the adjustment if the IRS requests it.
Miscellaneous adjustments and miscellaneous deductions are not used to describe all above-the-line adjustments that do not have corresponding input lines on Schedule 1 (Form 1040).
Therefore, options C and D are incorrect. The correct option is A. Write-in adjustments.
To know more about corresponding visit:-
https://brainly.com/question/12454508
#SPJ11
The local gym want to expand the building by doubling the size find the perimeter of the entire rectangle.
Answers
The perimeter of a rectangle is calculated by adding all of the sides of a rectangle. The formula for the perimeter of a rectangle is 2(l + w).
To calculate the perimeter of a rectangle, you must first find the length and width of the rectangle. The local gym wants to double the size of its building, so the new length and width will be double the original length and width. Therefore, if the original length was l and the original width was w, then the new length and width will be 2l and 2w, respectively. The new perimeter of the rectangle will be:P = 2(2l + 2w)P = 4l + 4w
The local gym wants to double the size of its building. To find the perimeter of the entire rectangle, you must first find the length and width of the rectangle. Let's assume that the original length of the gym was l and the original width was w. To double the size of the gym, the new length and width will be 2l and 2w, respectively.The perimeter of a rectangle is calculated by adding all of the sides of a rectangle. The formula for the perimeter of a rectangle is 2(l + w). Therefore, the new perimeter of the rectangle will be:P = 2(2l + 2w)P = 4l + 4wSo, the perimeter of the entire rectangle will be 4l + 4w.
To know more about perimeter visit :-
https://brainly.com/question/7486523
#SPJ11
6b - 11 = -23 what is b
Answers
Answer:
8b-5=6b+23
8b-6b=23+5
2b=28
b=14
Step-by-step explanation:
find minimum number of coins that make a given value
Answers
The given coins [1, 2, 5] and the value 11, the minimum number of coins needed is 2.
Here are the steps to find the minimum number of coins:
1. First, we create an array of size equal to the given value, initialized with a very large number. This array will store the minimum number of coins needed to make each value from 0 to the given value.
2. We set the first element of the array to 0, as it doesn't require any coins to make a value of 0.
3. Next, we iterate through all the coins available and for each coin, we iterate through all the values from the coin value to the given value.
4. For each value, we calculate the minimum number of coins needed by taking the minimum of the current minimum and the value obtained by subtracting the coin value from the current value and adding 1 to it.
5. Finally, we return the value stored in the last element of the array, which represents the minimum number of coins needed to make the given value.
Let's consider an example to better understand the process:
Given coins: [1, 2, 5]
Given value: 11
1. Initialize the array with [INF, INF, INF, INF, INF, INF, INF, INF, INF, INF, INF, INF] (INF represents infinity).
2. Set the first element of the array to 0, so it becomes [0, INF, INF, INF, INF, INF, INF, INF, INF, INF, INF, INF].
3. For the first coin (1), iterate through the array from index 1 to 11.
- For index 1, the minimum number of coins needed is 0 + 1 = 1.
- For index 2, the minimum number of coins needed is 0 + 1 = 1.
- For index 3, the minimum number of coins needed is 0 + 1 = 1.
- ...
- For index 11, the minimum number of coins needed is 0 + 1 = 1.
The array becomes [0, 1, 1, 1, 1, 1, 1, 1, 1, 1, 1, 1].
4. For the second coin (2), iterate through the array from index 2 to 11.
- For index 2, the minimum number of coins needed is 1 (minimum of 1 and 0 + 1 = 1).
- For index 3, the minimum number of coins needed is 1 (minimum of 1 and 0 + 1 = 1).
- For index 4, the minimum number of coins needed is 1 (minimum of 1 and 1 + 1 = 2).
- ...
- For index 11, the minimum number of coins needed is 1 (minimum of 1 and 1 + 1 = 2).
The array becomes [0, 1, 1, 1, 2, 2, 2, 2, 2, 2, 2, 2].
5. For the third coin (5), iterate through the array from index 5 to 11.
- For index 5, the minimum number of coins needed is 2 (minimum of 2 and 0 + 1 = 1).
- For index 6, the minimum number of coins needed is 2 (minimum of 2 and 1 + 1 = 2).
- ...
- For index 11, the minimum number of coins needed is 2 (minimum of 2 and 2 + 1 = 3).
The array becomes [0, 1, 1, 1, 2, 1, 2, 2, 2, 2, 3, 2].
6. The minimum number of coins needed to make the given value (11) is 2.
To know more about coins here:
brainly.com/question/32385642
#SPJ11
factorise 4px-3my-2pm-6xy
Answers
Answer:
E
Step-by-step explanation:
NO
Determine two values of n that allow each polynomial to be a perfect squad trinomial. Then, factor: x^2 + nx +25
Answers
The factored form of x² + 10x + 25 is (x + 5)².
To determine two values of n that allow the polynomial x² + nx + 25 to be a perfect square trinomial, we need to consider the general form of a perfect square trinomial:
(ax + b)² = a²x² + 2abx + b²
Comparing this form with the given polynomial x² + nx + 25, we can see that:
a²x² = x² (So, a = 1)
2abx = nx (So, 2ab = n)
b² = 25 (So, b = ±5)
Since we have b = ±5, the values of n can be obtained by substituting b = 5 and b = -5 into 2ab = n.
For b = 5:
2(1)(5) = n
10 = n
For b = -5:
2(1)(-5) = n
-10 = n
The two values of n that allow the polynomial x² + nx + 25 to be a perfect square trinomial are n = 10 and n = -10.
Now let's factor the polynomial x² + nx + 25 using one of the determined values of n (let's use n = 10 as an example):
x² + 10x + 25
We can factor this trinomial as a perfect square trinomial:
(x + 5)²
For similar questions on factored
https://brainly.com/question/25829061
#SPJ8
Someone help me with this question

Answers
the answer is 7 because thats what photo math told me
Answer:
7
Step-by-step explanation:
(8+56)÷4-3^2
PEMDAS:
(64)÷4-3^2
PEMDAS:
(64)÷4-9
PEMDAS:
(16)-9
7
hope this helps
three percent of the customers of a mortgage company default on their payments. a sample of five customers is selected. what is the probability that exactly two customers in the sample will default on their payments?
Answers
The probability that exactly two customers in the sample will default on their payments is 0.9997.
What is the probability that exactly two customers in the sample will default on their payments?
This is a binomial probability problem with n = 5 trials and p = 3% = 0.03 probability of success (default) on each trial.
The probability of exactly two defaults can be calculated using the binomial probability formula:
P(x = r) = nCr * p^r * q^n-r
where q = 1 - p = 1 - 0.03 = 0.97
P(x = 2) = (5C0 × 0.03⁰ × 0.97⁵⁻⁰) + (5C1 × 0.03¹ × 0.97⁵⁻¹) + (5C2 × 0.03² × 0.97⁵⁻²)
P(x = 2) = 0.9997
Learn more about binomial distribution on:
brainly.com/question/29220138
#SPJ1
HELP I NEED HELP ASAP HELP I NEED HELP ASAP HELP I NEED HELP ASAP HELP I NEED HELP ASAP
HELP I NEED HELP ASAP HELP I NEED HELP ASAP HELP I NEED HELP ASAP HELP I NEED HELP ASAP

Answers
Answer:
2100
Step-by-step explanation:
What is 1 and 2/5 as an Improper faction in its simplest form
Answers
Answer:
\(\frac{7}{5}\)
Step-by-step explanation:
1 \(\frac{2}{5}\)
= 1 + \(\frac{2}{5}\)
= \(\frac{5}{5}\) + \(\frac{2}{5}\)
= \(\frac{5+2}{5}\)
= \(\frac{7}{5}\)
The fraction is:
7/5
Step-by-step explanation:
The number we're given is:
\(\large\pmb{1\dfrac{2}{5}}\)
To convert it into an improper fraction, we first multiply the whole number part (1) times the denominator (5). Then, we add 2, the numerator. We get 7.
That is the numerator of the improper fraction. As for the denominator, we just copy it.
\(\pmb{\dfrac{7}{5}}\)
Therefore, the answer is 7/5.
how can relative frequencies be used to help us estimate porbailities occuring in sampling distriubution
Answers
Relative frequencies can be used to estimate probabilities occurring in a sampling distribution through the concept of the Law of Large Numbers.
Define the event of interest Determine the specific event or outgrowth for which you want to estimate the probability in the slice distribution. Conduct repeated trials Perform a large number of independent trials or compliances. Each trial should be done under the same conditions. Count circumstances Record the number of times the event of interest occurs within the total number of trials.
Calculate relative frequence Divide the count of circumstances by the total number of trials to gain the relative frequence. This represents the proportion of times the event of interest passed relative to the total number of trials. reprise way 2- 4 Repeat the process of conducting trials, counting circumstances, and calculating relative frequentness multiple times. The further trials you perform, the more accurate your estimates will come.
Learn more about relative frequencies at
https://brainly.com/question/8804679
#SPJ4
Select the correct answer from each drop-down menu.
A code is formed using four of these letters: A, B, I, K, N, O, and T. (Note that the order of the letters in the code matters; for example, ABIK is a different code from BAIK.)
The size of the sample space is
. The probability that the first three letters of the four-letter code are vowels is
.
Answers
The Probability that the first three letters of the four-letter code are vowels is 0/35 = 0.
The size of the sample space, we need to find the number of ways to choose 4 letters from 7 letters without repetition and without regard to order. This is given by the combination formula:
nCr = n! / r!(n-r)!
Where n is the total number of letters, and r is the number of letters in each code.
So, the size of the sample space is:
7C4 = 7! / 4!(7-4)! = 7! / 4!3! = (7x6x5) / (3x2x1) = 35
Therefore, there are 35 possible four-letter codes that can be formed from the given letters.
Next, we need to find the probability that the first three letters of the four-letter code are vowels. There are two vowels in the given letters: A and O. To form a four-letter code with the first three letters as vowels, we need to choose 3 vowels from 2 vowels and 1 consonant from the remaining 5 consonants. This can be done in:
2C3 x 5C1 = 0 x 5 = 0ways.
Therefore, the probability that the first three letters of the four-letter code are vowels is 0/35 = 0.The size of the sample space is 35. The probability that the first three letters of the four-letter code are vowels is 0.
To know more about Probability .
https://brainly.com/question/13604758
#SPJ11
Triangle ABC has coordinates A(-2, -3), B(1, 1), and C(2, -1). If the triangle is
translated 1 unit right and 3 units up, what are the coordinates of A?
Answers
Graph the compound inequality.
f ≤ –0.8 or f ≥ 1.6


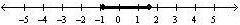

Answers
Answer:
f ≥ 1.6
Step-by-step explanation:
I am also learning this in school rn :)
*PLS HELP*
I need help on this and please provide an explanation
Thank you!
Question: What is the value of X°
In this complementary angle?

Answers
Answer:
x=30
Yes, they are complementary
Step-by-step explanation:
The two angles shown add up to the right angle marked. Right angles are 90 degrees. Set up the following equation:
2x°+x°=90
3x°=90
x=30
Complementary angles are two angles that add up to 90°. Since the two angles add up to 90 degrees, they are complementary.
Answer:
\(\boxed{\boxed{ \tt \: x = 30 {}^{ \circ}}} \)
Step-by-step explanation:
The Given two angles are complementary angles .
The Two angles are 2x° and x°.[Given]
[Two angles are called complementary if their sum is 90°. Each angle is a complement to each other.]
We need to find the value of x.
So,\( \tt2x {}^{ \circ} + {x}^{ \circ} = 90{}^{ \circ} \)
Solve this equation.
\(\tt \implies(2x + {x} ){}^{ \circ} = 90{}^{ \circ} \)
Combine the like terms:
\(\tt3x{}^{ \circ} = 90{}^{ \circ} \)
Divide both sides by 3 :
\( \tt \implies \cfrac{3x{}^{ \circ} }{3{}^{ \circ} } = \cfrac{90{}^{ \circ} }{3{}^{ \circ} } \)
Cancel the LHS and RHS:
\( \tt \implies \cfrac{ \cancel{3x{}^{ \circ}} }{ \cancel{3{}^{ \circ}} } = \cfrac{ \cancel{90}{}^{ \circ} }{ \cancel{3{}^{ \circ}} } \)
\( \tt \implies \cfrac{1x{}^{ \circ} }{1{}^{ \circ} } = \cfrac{30{}^{ \circ} }{1{}^{ \circ} } \)
\( \tt \implies 1x{}^{ \circ} = 30{}^{ \circ} \)
\( \tt \implies x{}^{ \circ} = 30{}^{ \circ} \)
\( \tt \implies x{}^{ \circ} = 30{}^{ \circ} \)
Hence, the value of x° would be 30°.
\( \rule{225pt}{2pt}\)
I hope this helps!
if a = 7 and b = 2, find the value of (a - b) ²
Answers
Answer:
substitute it into the value that they give then expand the formula which is
a^2-2ab+b^2
Step-by-step explanation:
Evaluate the following expression:
30 = 5(8 – 6 + 1) - (2^2 - 3)^3
Answers
Answer:
30=15
Step-by-step explanation:
30=40-30+6-4-27
30=10+2-27
40=12-27
30=-15