Answers
All the correct statements are,
2) AH ≅ HA Symmetry property of ≅
3) MA ≅ TA Definition of kite
HT ≅ MH
4) ΔΑΜΗ = ΔΑΤΗ By SSS post
We have to given that;
MATH is a kite
And, To Prove;
∠AMH ≅ ∠ATH
Now, We can prove with all the statements as,
Statement Reason
1) MATH is a kite Given
2) AH ≅ HA Symmetry property of ≅
3) MA ≅ TA Definition of kite
HT ≅ MH
4) ΔΑΜΗ = ΔΑΤΗ By SSS post
5) ∠AMH ≅ ∠ATH CPCTC
Hence, Prove of all the statement are shown above.
Learn more about the kite visit;
https://brainly.com/question/11808140
#SPJ1
Related Questions
What are the 6 trigonometric identities?
Answers
The six trigonometric identities are:
cosecant(x) = 1/sin(x) secant(x) = 1/cos(x) csc(x) = 1/sin(x) sec(x) = 1/cos(x) tan(x) = sin(x)/cos(x) cot(x) = cos(x)/sin(x)Trigonometry is the branch of mathematics that deals with the relationships between the angles and sides of triangles. In trigonometry, there are six basic functions, sine, cosine, tangent, cosecant, secant, and cotangent, each of which is represented by a specific letter. These functions are related to each other through a set of identities known as the trigonometric identities.
The six trigonometric identities listed above, are the most commonly used identities, and provide the relationships between the six trigonometric functions and each other. They are useful for solving trigonometric equations, simplifying trigonometric expressions, and solving problems in geometry, physics, engineering and other sciences.
The Pythagorean identity states that the sum of the squares of the sine and cosine of an angle is equal to 1, which is a fundamental relationship between the two functions. The reciprocal identities state that the reciprocal of the sine and cosine of an angle is equal to the cosecant and secant of that angle respectively.
The quotient identities state that the tangent of an angle is equal to the sine of that angle divided by the cosine of that angle, and the cotangent of an angle is equal to the cosine of that angle divided by the sine of that angle.
It's important to note that these identities are valid for all values of the angle, and are true in any angle measurement system (degrees or radians).
To know more about trigonometric identities on the link below:
https://brainly.com/question/24377281#
#SPJ11
In 2021, the Internal Revenue Service (IRS) decreased the deductible mileage cost to 56 cents from 57.5 cents. Find the percent decrease. (Round to the nearest hundredth of a percent)
Answers
The deductible mileage cost has reduced by 2.16%
What is a percentage change?
A percentage change compares the difference between the new amount and the old amount to the old amount.
I mean the percentage change is the new deductible mileage cost minus the old deductible mileage cost divided by the old deductible mileage cost
New deductible mileage cost= 57.5 cents
Old deductible mileage cost= 56 cents
percentage decrease=(56-57.5)/57.5
percentage decrease=-2.61%
The negative sign in -2.61% means that there was a percentage decrease in deductible mileage cost by 2.61%
In essence, the deductible mileage cost has been reduced by 2.16%
Hundredth of a percent means two decimal places.
Find out more on percent change on:https://brainly.com/question/2085058
#SPJ1
let the universal set u be all the letters of the english alphabet. what is the complement of the empty set? (note: the empty set is a subset of every set.)
Answers
The complement of the empty set is the set of all possible elements in the universal set U, which is the English alphabet in this context.
The universal set U is defined as the set of all possible elements or values under consideration for a given context. On the other hand, the complement of a set A is defined as the set of all elements that are not in A but are in U.
The complement of the empty set is defined as the set of all elements in U since the empty set is a subset of every set.
Therefore, the complement of the empty set in this context would be the entire set of all letters in the English alphabet.
This is because the empty set contains no elements, and therefore, its complement would be the set of all possible elements in U, which in this case is the English alphabet.
For more questions on empty set
https://brainly.com/question/1632391
#SPJ8
A researcher was interested in utilities provided by city governments. The researcher randomly selected 20 counties from a list of all counties in the U.S. From each of these counties the researcher then contacted each city government (a total of 192) and found that 12 (6.25%) of them provided electricity to their residents. In this situation the sampling frame is :_________
a. the list of all counties in the U.S.
b. the 316 cities.
c. the 20 counties.
d. 6.25%.
Answers
The sampling frame refers to the list or population from which the sample is selected. In this situation, the researcher randomly selected 20 counties from a list of all counties in the U.S. Therefore, the sampling frame is c. the 20 counties.
The sampling frame in this situation is the list of all counties in the U.S. The researcher randomly selected 20 counties from this list to conduct their study. From each of these counties, the researcher contacted every city government, resulting in a total of 192 city governments.
Among these city governments, the researcher found that 12, or 6.25%, provided electricity to their residents. However, it's important to note that the sampling frame specifically refers to the list of all counties in the U.S., as it represents the population from which the sample was selected.
To know more about governments visit-
brainly.com/question/14477132
#SPJ11
in a box of 10 electrical parts, 2 are defective. (a) if you choose one part at random from the box, what is the probability that it is not defective? (b) if you choose two parts at random from the box, without replacement, what is the probability that both are defective?
Answers
The probabilities are :
a) 0.8
b) 1/45
Probability refers to potential. This branch of mathematics deals with the occurrence of a random event. The value's range is 0 to 1. Probability has been applied into mathematics to predict the likelihood of different events.
Total no. of electrical parts = 10
No. of defective parts = 2
No. of non-defective parts = 10 - 2
= 8
a) The probability of choosing a non-defective part from the box is 8/10 or 0.8.
b) The probability of choosing two defective parts in a row without replacement is (2/10) * (1/9) = 2/90 = 1/45.
To learn more about probabilities here:
https://brainly.com/question/30034780
#SPJ4
Write an equation in slope-intercept form for the line that passes through the given
point and is perpendicular to the graph of the equation.
24. (−5, 2), y = ¹⁄2x − 3
Please explain how to get the answer too.
Answers
Answer:
Step-by-step explanation:
perp. -2
y - 2 = -2(x + 5)
y - 2 = -2x - 10
y = -2x - 8
3x+5(2+x)=4x-7 help me out here?
Answers
Answer:
x = -17/4
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightDistributive Property
Equality Properties
Multiplication Property of Equality Division Property of Equality Addition Property of Equality Subtraction Property of EqualityAlgebra I
Terms/CoefficientsStep-by-step explanation:
Step 1: Define
Identify
3x + 5(2 + x) = 4x - 7
Step 2: Solve for x
[Distributive Property] Distribute 5: 3x + 10 + 5x = 4x - 7[Addition] Combine like terms: 8x + 10 = 4x - 7[Subtraction Property of Equality] Subtract 4x on both sides: 4x + 10 = -7[Subtraction Property of Equality] Subtract 10 on both sides: 4x = -17[Division Property of Equality] Divide 4 on both sides: x = -17/4Answer:
x=\(\frac{-17}{4}\)
Step-by-step explanation:
Step 1: Simplify both sides of the equation.
3x+5(2+x)=4x−7
3x+(5)(2)+(5)(x)=4x+−7(Distribute)
3x+10+5x=4x+−7
(3x+5x)+(10)=4x−7(Combine Like Terms)
8x+10=4x−7
8x+10=4x−7
Step 2: Subtract 4x from both sides.
8x+10−4x=4x−7−4x
4x+10=−7
Step 3: Subtract 10 from both sides.
4x+10−10=−7−10
4x=−17
Step 4: Divide both sides by 4.
\(\frac{4x}{4}\)=\(\frac{-17}{4}\)
x=\(\frac{-17}{4}\)
Factorise fully 3x-9x^2
Answers
Answer:
\(3x(1 - 3x)\)
Step-by-step explanation:
We can factor the expression by using the common factor.
Pull out 3x from the expression.
\(3x - 9 {x}^{2} \\ 3x(1 - 3x)\)
Common Factor is similar to dividing by the term we pull out. When we pull out the term, we divide the whole expression and keep the term out of the expression.
Write the equation of the line in fully simplifipe-intercept form.
12
11
10
9
8
7
6
5
4
3
2
1
-12-11-10 -9 -8 -7 -6 -5 -4 -3 -2 -1
1 2 3 4 7 8 9 10 11 12
-2
-3
-4
-5
-6
-7
-O
-9
-10
-11
-12
Answers
Answer: Y = Z!
Step-by-step explanation: Hopes This Helps!
Which best fits in those boxes to find 46x93?

Answers
Answer:
Step-by-step explanation:
1.24
Answer:
3600
540
120
18
4278
Step-by-step explanation:
i am trying to remember how to do Write a two-column proof,
Given: a = 2b + 6
a = 9b - 8
Prove: b = 2
Answers
9514 1404 393
Explanation:
Make use of the properties of equality.
a = 2b +6 . . . . . given
a = 9b -8 . . . . . given
2b +6 = 9b -8 . . . . . . . substitution property of equality
6 = 7b -8 . . . . . . . . . . . subtraction property of equality
14 = 7b . . . . . . . . . . . . . addition property of equality
2 = b . . . . . . . . . . . . . . . division property of equality
b = 2 . . . . . . . . . . . . . . symmetric property of equality
can someone write me the equation please???

Answers
Hope this helps
To determine if "A" students tend to sit in a particular part of the classroom, a teacher recorded (1 point) the locations of the students who received grades of A. He found that 17 sat in front, 9 sat in the middle, and 5 sat in the beEk of the classroom, when testing the assumption that the"A" stadents are distributed evenly throughout the room, he obtained the test statistic of -7.226. If using a 0.05 significance level, is there sufficient evidence to support the claim that the"A" students are not evenly distributed throughout the classroom? Then state the conclusion. OFail to reject the null hypothesis; there is sufficient evidence to support the claim that the A" students are not evenly distributed throughout the OFail to reject the null hypothesis; there is sufficient evidence to support the claim that the "A" students are evenly distributed throughout the classroom OReject the tull hypothesis; there is sufficient evidence to support the claim that the "A" students are not evenly distributed throughout the classroom OReject the null hypothesis; there is sufficient evidence to support the claim that the "A students are evenly distributed throughout the classroom. Dc
Answers
The test statistic (-7.226) is less extreme (i.e., more negative) than the critical value (5.991), we fail to reject the null hypothesis.
To determine whether the "A" students are evenly distributed throughout the classroom, we can perform a chi-square goodness-of-fit test. The null hypothesis (H₀) assumes that the "A" students are distributed evenly, while the alternative hypothesis (H₁) suggests that they are not evenly distributed.
We are given the observed frequencies of "A" students in different parts of the classroom:
17 students sat in front
9 students sat in the middle
5 students sat in the back
To conduct the chi-square test, we need to calculate the expected frequencies under the assumption of an even distribution. Since there are three possible locations (front, middle, back), and assuming an equal distribution, we would expect approximately 31.67% of students in each location.
Expected frequencies:
Front: (31.67% * total number of "A" students) = 31.67% * (17 + 9 + 5) = 10.01
Middle: (31.67% * total number of "A" students) = 31.67% * (17 + 9 + 5) = 10.01
Back: (31.67% * total number of "A" students) = 31.67% * (17 + 9 + 5) = 10.01
Now, we can set up the chi-square test:
H₀: "A" students are evenly distributed throughout the classroom.
H₁: "A" students are not evenly distributed throughout the classroom.
We can calculate the test statistic using the formula:
χ² = ∑ ((Observed frequency - Expected frequency)² / Expected frequency)
Plugging in the values, we get:
χ² = [(17 - 10.01)² / 10.01] + [(9 - 10.01)² / 10.01] + [(5 - 10.01)² / 10.01]
= 35.7022 + 0.1009 + 24.3079
= 60.111
Now, with the test statistic of -7.226 provided, we need to determine the critical value or p-value to make a decision. Since the significance level is 0.05, we can look up the critical value for a chi-square distribution with (3-1) = 2 degrees of freedom. The critical value is approximately 5.991.
Since the test statistic (-7.226) is less extreme (i.e., more negative) than the critical value (5.991), we fail to reject the null hypothesis.
Therefore, the correct conclusion is:
Fail to reject the null hypothesis; there is sufficient evidence to support the claim that the "A" students are evenly distributed throughout the classroom.
for such more question on test statistic
https://brainly.com/question/17142834
#SPJ8
____relate the side lengths of a right triangle to an acute angle
Answers
Sine function relates side lengths of a right triangle to an acute angle.
In a right triangle, the side lengths are related to the acute angles through the trigonometric functions. The side opposite the acute angle is related to the angle through the sine function, the side adjacent to the acute angle is related to the angle through the cosine function, and the hypotenuse is related to the angle through the tangent function.
More specifically, if θ is an acute angle in a right triangle and a and b are the lengths of the side opposite and adjacent to the angle respectively, and c is the length of the hypotenuse, we have:
sin(θ) = a/c
cos(θ) = b/c
tan(θ) = a/b
Thus, we can use these functions to find the length of any side of the right triangle if we know the measure of one of the acute angles and the length of one of the other sides.
To know more about sine function click below:
https://brainly.com/question/22288720#
#SPJ4
If a=3+√7/2, then find the value of a²+1/a²
Answers
The value of a² + 1/a² is 17 - 7.5√7.
To find the value of a² + 1/a², let's first simplify the expression for a.
Given: a = (3 + √7) / 2
Now, let's square both sides of the equation to eliminate the square root:
a² = [(3 + √7) / 2]²
= (3 + √7)² / 4
= (9 + 6√7 + 7) / 4
= (16 + 6√7) / 4
= 4 + (6√7) / 4
= 1 + (3√7) / 2
Next, let's find the reciprocal of a:
1/a = 1 / [(3 + √7) / 2]
= 2 / (3 + √7)
= [2 * (3 - √7)] / [(3 + √7) * (3 - √7)]
= [6 - 2√7] / (9 - 7)
= [6 - 2√7] / 2
= 3 - √7
Now, let's square both sides of the equation to find the value of 1/a²:
(1/a)² = (3 - √7)²
= 9 - 6√7 + 7
= 16 - 6√7
Finally, we can calculate the value of a² + 1/a²:
a² + 1/a² = (1 + (3√7) / 2) + (16 - 6√7)
= 1 + 16 + (3√7) / 2 - 6√7
= 17 - (3√7) / 2 - 6√7
= 17 - (15√7) / 2
= 17 - 7.5√7.
For similar question on expression .
https://brainly.com/question/24734894
#SPJ11
Please Help Me Answer These Question
Need Help With Question 2 And 3

Answers
Answer:
2. 9
Step-by-step explanation:
Plug in 20 for the perimeter the, subtract 2 which gets you 18 and divide by 2 and you get 9
Suppose you want to test the claim that μ ≠3.5. Given a sample size of n = 47 and a level of significance of α = 0.10, when should you reject H0 ?
A..Reject H0 if the standardized test statistic is greater than 1.679 or less than -1.679.
B.Reject H0 if the standardized test statistic is greater than 1.96 or less than -1.96
C.Reject H0 if the standardized test statistic is greater than 2.33 or less than -2.33
D.Reject H0 if the standardized test statistic is greater than 2.575 or less than -2.575.
Answers
Using a t-distribution table, here with 46 degrees of freedom (n-1), the critical value for a two-tailed test with a level of significance of α = 0.10 is ±2.575. Therefore, we reject H0 if the standardized test statistic is greater than 2.575 or less than -2.575. The correct answer is D. Reject H0 if the standardized test statistic is greater than 2.575 or less than -2.575.
To determine whether to reject H0, we need to calculate the standardized test statistic using the formula (sample mean - hypothesized mean) / (standard deviation / square root of sample size). Since the null hypothesis is that μ = 3.5, the sample mean and standard deviation must be used to calculate the standardized test statistic.
Assuming a normal distribution, with a sample size of n=47 and a level of significance of α = 0.10, we can use a two-tailed test with a critical value of ±1.645. However, since we are testing for μ ≠ 3.5, this is a two-tailed test, and we need to use a critical value that accounts for both tails.
Learn more about t-distribution here, https://brainly.com/question/17469144
#SPJ11
In 2015 the cost of a complete bathroom package represented_____ of the monthly average household expenditures of the bottom 40% of the poorest population. Group of answer choices 40% 5% 27% 14%
Answers
In 2015, the cost of a complete bathroom package represented D. 14% of the monthly average household expenditures for the bottom 40% of the poorest population.
This means that out of the total monthly expenses of these households, 14% was spent on bathroom packages. This percentage highlights the financial burden that bathroom expenses placed on these families, as they had to allocate a significant portion of their limited resources towards this essential facility.
Among the given answer choices - a. 40%, b. 5%, c. 27%, and d. 14% - the correct answer is d. 14%, as this is the percentage mentioned in the question. The other percentages do not apply to the context of the question and therefore can be disregarded.
In summary, the cost of a complete bathroom package in 2015 accounted for 14% of the monthly average household expenditures of the bottom 40% of the poorest population. This percentage reflects the financial strain on these households to meet their basic needs, including essential facilities like bathrooms. Therefore the correct option is D
Know more about financial burden here:
https://brainly.com/question/29975552
#SPJ11
Solve the system of equations:
(1) x - y = 3
(2) 7x - y = -3
Answers
Answer:
x= 70
and y = 67 then
-70-67 =-3
Answer:
x=-1 y=-4
Step-by-step explanation:
answer is in the photo above

Greatest to least 9,354 , 9,012 , 9,1 , 9,157
Answers
Answer:
9,354,9,157,9,012,9,1
Step-by-step explanation:
First I do thousands place so 3 numbers have 9 for thousands place.
Numbers are
9,354
9,157
9,012
it cant be 9,012 because this number don't have a hundreds place.
But in hundred place 9,354 would be better than 9,157 because 9,354 had 300 while 9,157 only has 100.
So greatest=9,354
Second greatest=9,157
And last 9,000 number third greatest=9,012.
Our last numbers don't have hundreds or thousands,they have ones but 9 is greater than 1 because...
Say you had 9 pizzas and your friend had 1 you would have more or a greater amount so.
Least=1
and second least=9
Meaning order=
9,356=greatest
9,157
9,012
9
1=least
Hope it helps have a great day:)
3|2+x|-4=14
Solve this absolute value equation.
Answers
Answer:
Its a number
Step-by-step explanation
trapezoid abcd is proportional to trapezoid efgh. the height of trapezoid abcd is 6 cm. the length of line dc is twice the height of trapezoid abcd, and four times the length of ab. what is the area of trapezoid efgh, in cm2?
Answers
the area of trapezoid efgh is given by the expression 3 * 12^2 / (x + 12) cm^2.
Let's denote the length of ab as x. Since line dc is twice the height of trapezoid abcd and four times the length of ab, its length is 2 * 6 = 12 cm. Additionally, line dc is also the sum of the lengths of ef and gh. Thus, we have ef + gh = 12 cm.
Since trapezoid abcd is proportional to trapezoid efgh, the ratio of their areas is equal to the square of the ratio of their corresponding side lengths. Therefore, (Area of efgh) / (Area of abcd) = (ef + gh)^2 / (ab + cd)^2.
Plugging in the values, we have (Area of efgh) / (Area of abcd) = (12)^2 / (x + 12)^2.
Given that the height of abcd is 6 cm, its area is (1/2) * (ab + cd) * 6 = (1/2) * (x + 12) * 6 = 3(x + 12) cm^2.
Multiplying both sides of the proportionality equation by the area of abcd, we get (Area of efgh) = (Area of abcd) * [(ef + gh)^2 / (ab + cd)^2].
Substituting the values, we find (Area of efgh) = 3(x + 12) * [(12)^2 / (x + 12)^2].
Simplifying further, we get (Area of efgh) = 3 * 12^2 / (x + 12).
Therefore, the area of trapezoid efgh is given by the expression 3 * 12^2 / (x + 12) cm^2.
Learn more about trapezoid here:
https://brainly.com/question/29193735
#SPJ11
please help its very important

Answers
Answer:
8) B is steeper.
9) the equation is (30,7)
Every matrix transformation is a linear transformation.a. Trueb. false
Answers
True , Every matrix transformation is a linear transformation.
Is there a linear transformation for every matrix operation?
Despite the fact that every linear transformation is a matrix transformation, not all linear transformations are matrix transformations.
Because of this, it's possible that we'll encounter a linear transformation in which we're unable to locate a matrix to carry out the mapping.
What alteration does matrix entail?
In a two-dimensional or three-dimensional space, the transformation matrix converts one vector into another vector, which may be comprehended geometrically.
Stretching, squeezing, rotation, reflection, and orthogonal projection are some of the often utilized transformations.
Learn more about transformation matrix
brainly.com/question/16956653
#SPJ4
of the 20 students in the math club, five are randomly selected to participate in a competition. how many different groups of students could be selected for the competition?
Answers
There are a total of 15,504 different groups of five students that could be selected for the competition from the 20 students in the math club.
To arrive at this answer, we can use the combination formula, which is given by:
nCk = n! / (k! * (n-k)!)
where n is the total number of students in the math club (20), and k is the number of students selected for the competition (5).
Substituting these values into the formula, we get:
20C5 = 20! / (5! * (20-5)!) = 15,504
Therefore, there are 15,504 different groups of students that could be selected for the competition.
Learn more about Combination Formula here: brainly.com/question/19916016
#SPJ11
Latrell has $8 to spend on postcards. He wants to buy one large postcard and some small ones. Write an inequality to determine how many small postcards Latrell can purchase. Postcards Large $2. 00 Medium $1. 50 Small $1. 25
Answers
The inequality to determine how many small postcards Latrell can purchase is: 1.25s + 2.00 ≤ 8.00
In the inequality 1.25s + 2.00 ≤ 8.00 s represents the number of small postcards and the left-hand side of the inequality represents the total cost of the postcards, including one large postcard costing $2.00.
To solve for s, we can begin by subtracting 2.00 from both sides of the inequality to isolate the term with s:
1.25s ≤ 6.00
Then, we can divide both sides of the inequality by 1.25 to solve for s:
s ≤ 4.8
Since s represents a whole number, the largest number of small postcards that Latrell can purchase is 4.
In summary, the inequality 1.25s + 2.00 ≤ 8.00 can be used to determine the maximum number of small postcards (s) that Latrell can purchase with $8 while also buying one large postcard. By solving the inequality, we find that s ≤ 4.8, so the largest whole number of small postcards that Latrell can purchase is 4.
learn more about inequality here:
https://brainly.com/question/30231190
#SPJ1
Factor the algebraic expression
24q - 76u + 104i - 52b - 36i
(help?)
Answers
Answer:
4(6q-19u-13b+17i)
Step-by-step explanation:
each of the variables is divisble by 4, thus allowing you to factor out 4.
Step-by-step explanation:
24q-76u+104i-52b-36i
24q-76u-68i-52b
4(6q-19u-17i-13b)

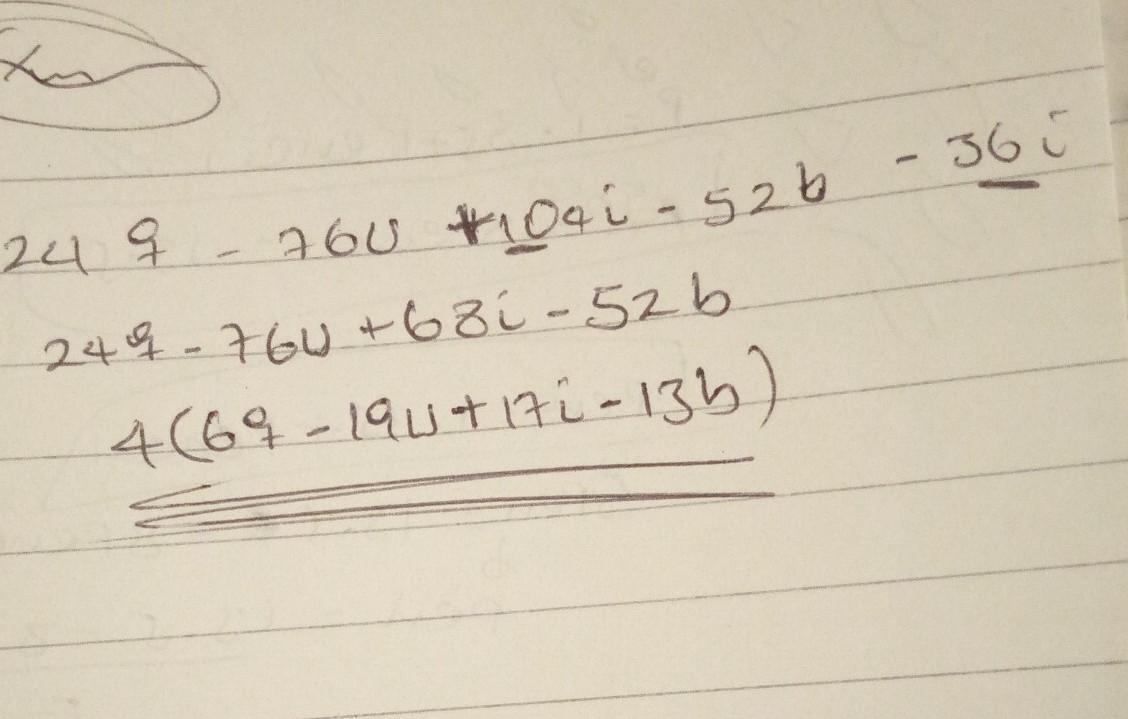
What is the slope of the line that passes through (-5,6) and (-2,4)
Answers
The slope of the line that passes through the given points (-5, 6) and (-2, 4) as required in the task content is; -2 / 3.
What is the slope of the line that passes through the given points; (-5,6) and (-2,4)?It follows from the task content that the slope of the line that passes through the given points; (-5,6) and (-2,4) be determined as required.
On this note, since the slope, otherwise termed the average rate of change is given by the formula;
Slope, m = ( y₂ - y₁ ) / ( x₂ - x₁ )
Therefore, given the points; (-5, 6) and (-2, 4), the required slope of the line is;
m = ( 4 - 6 ) / ( -2 - (-5) )
m = -2 / (-2 + 5)
m = -2 / 3
Therefore, the required slope of the line which passes through the given points is; -2 / 3.
Read more on slope of the line;
https://brainly.com/question/28645794
#SPJ1
Selena and Drake are evaluating the expression (StartFraction r s Superscript negative 2 Baseline Over r squared s Superscript negative 3 Baseline EndFraction) Superscript negative 1, when r = negative 1 and s = negative 2. Selena’s Work Drake’s Work (StartFraction r s Superscript negative 2 Baseline Over r squared s Superscript negative 3 Baseline EndFraction) Superscript negative 1 Baseline = (r Superscript negative 1 Baseline s) Superscript negative 1 Baseline = StartFraction r Over s EndFraction = StartFraction negative 1 Over negative 2 EndFraction = one-half (StartFraction (negative 1) (negative 2) Superscript negative 2 Baseline Over (negative 1) squared (negative 2) Superscript negative 3 EndFraction) Superscript negative 1 = (StartFraction (negative 1) (negative 2) cubed Over (negative 1) squared (negative 2) squared EndFraction) Superscript negative 1 = (StartFraction negative 8 Over 4 Endfraction) Superscript negative 1 Baseline = StartFraction 4 Over negative 8 EndFraction = negative one-half Who is correct and why? Selena is incorrect because she should have substituted the values for the variables first, and then simplifed. Selena is correct because she simplified correctly and then evaluated correctly after substituting the values for the variables. Drake is incorrect because he should have simplified first, before substituting the values for the variables. Drake is correct because he substituted the values for the variables first, and simplified correctly.
Nevermind it's b
Answers
B) Selena is correct because she simplified correctly and then evaluated correctly after substituting the values for the variables.
I just took the quiz on edge
Answer:
(B) Selena is correct because she simplified correctly and then evaluated correctly after substituting the values for the variables.
Step-by-step explanation:
Three boxes are shipped on a truck. The base of each box is 16 square feet. Two boxes have a hight of 3 feet and one box has a height of 5 feet. What is the volume in cubic feet of the three boxes
Answers
Answer:
176 cubic feet
Step-by-step explanation:
Three boxes are shipped on a truck.
The base of each of the three boxes is 16 square feet
Two of the boxes have a height of 3 feet
One of the box have a height of 3 feet
The first step is to calculate the volume of each boxes
Volume= height × base
First box= 16 × 3
= 48
Second box= 16 × 3
= 48
Third box= 16 × 5
= 80
Therefore the volume of the three boxes in cubic feet can be calculated as follows
= 48 + 48 + 80
= 176 cubic feet
Hence the volume of the three boxes is 176 cubic feet
