Find the sum of 1/3+1/8
Answers
Answer:
11/24
Step-by-step explanation:
1/3 + 1/8
find the common denominator
that would be 24
now cross multiply
8/24+ 3/24= 11/24
Answer:11/24
Step-by-step explanation:
When adding fractions, you need to find the lcm of the denominator.
In this case, the lcm for 3 and 8 is 24 so you would do:
1*8
3*8
and
1*3
8*3
so you would get
8/24+3/24
which equals 11/24
Related Questions
A passenger on a stranded lifeboat shoots two distress flares into the air. The first flare travels a maximum height 196 feet and lands in the water after 7 seconds. The height (feet) of the second flare above the water is given by f(t) = -16t(t-8), where t is time (seconds) since the flare was shot. Which flare travels higher? Which remains in the air longer?
Answers
Answer:
The second flare travels higher, 256 feet, and remains in the air longer, 8 seconds, than the first flare
Step-by-step explanation:
The given parameters are;
The height of the first flare = 196 feet
The duration the first flare spends in the air = 7 seconds
The function representing the height of the second flair is f(t) = -16t(t - 8)
Where;
t = The time since the passenger shot the second flare
The time the flair stays i the air is given by the time it takes for the height, f(t), to be 0 as follows;
f(t) = 0 = -16t(t - 8)
-16t(t - 8) = 0
t = 0, or t = 8
The time the second flare stays in the air = 8 seconds
The maximum height reached by the second flair is given by the finding the maximum point of the curve of the function as follows;
d(f(t))/dt = 0 = d(-16t(t - 8))/dt = -32t + 128 at the maximum point (the coefficient of t² is negative in the quadratic equation)
-32t + 128 = 0
128 = 32t
32t = 128
t = 128/32 = 4
t = 4 at maximum height and f(4) = -16 × 4 × (4 - 8) = 256 feet
The maximum height reached by the second flare f(4) = -16 × 4 × (4 - 8) = 256 feet.
Therefore, the second flare travels higher, 256 feet, and remains in the air longer, 8 seconds, than the first flare, with maximum height of 196 feet, and time in the air of 7 seconds.
three points t, u, and v on the number line have coordinates t, u, and v, respectively. is point t between points u and v ?
Answers
We can determine coordinates if point t is between points u and v by checking if u < t < v or v < t < u.
To determine if point t is between points u and v, we need to compare their coordinates. If u < v, then point t is between points u and v if and only if u < t < v. On the other hand, if v < u, then point t is between points u and v if and only if v < t < u.
Whether or not point t is between points u and v depends on the relationship between the coordinates of u and v. If u < v, t must fall between them, and if v < u, t must also fall between them.
To know more about coordinates follow the link:
https://brainly.com/question/31306451
#SPJ11
someone with great knowledge of this please help its due asap please help.


Answers
Answer: x = 2 hours y= 7 hours = 9 hours a week, 80$ a week
Step-by-step explanation:
5$x2=10$ 10$x7=70$ 70$ + 10$ = 80 $.
hopefully, maybe that helps for the first question.
Select all statements that correctly interpret the relationship shown on the scatter plot.plz help!!!

Answers
Answer:
The data shows a linear association between the variables.
The data shows a negative association between the variables.
Yesterday, sam had 146 baseball cards. today, he gave d away. Using d, write an expression for the number of cards same has left
Answers
I need help with this its like a fraction

Answers
\(\frac{10^8}{10^6} = 10^{8-6} = 10^2 = 100\)
The rule used is
\(\frac{a^b}{a^c} = a^{b-c}\)
Subtract the exponents when dividing exponentials like this of the same base.
According to Foolproof, by not negotiating the price of your first car purchase, you have a $1,000 "bad" money burn. How long would it take you to earn back the thousand dollars you just threw away if you take home $10.00 per hour? State in terms of how many hours it would take to earn back that money, and explain how you calculated this answer.
Answers
Answer:
Time required to save 1000$ is 100 hours
Step-by-step explanation:
Money wasted = 1000$
savings = 10.00$ per hour
using unitary method
if i am saving 10.00 $ per hour then let in t hours i save 1000$
⇒ 10.00 x t = 1000
dividing above equation by 10 we get,
⇒ t = 100 hours
Therefore, Time required to save 1000$ is 100 hours
PLZ HELP WILL GIVE BRAINLIST IF CORRECT!!! IGNORE THE METHODS

Answers
1) 25%of50=$12.5. 50-12.5=37.5
2)20%of21=$4.2. 21-4.2=16.8
3)5%of12=$0.6. 12-0.6=11.4
HOPE THIS HELPED!!!
Answer:
1. $37.50
2.$16.80
3. 0.41
Step-by-step explanation:
Let x and y be real numbers, determine the magnitude and angle of the following complex numbers. (15 points) (a) 2e^-5jx (b) e^x +1 C) e^j2x/e^-jy
Answers
The magnitude and angle of the complex number e^j2x/e^-jy are sqrt(e^(j4x) + e^(-j2y)) and 2.3562, respectively.
To determine the magnitude and angle of the given complex numbers, we need to use the following formulas:
Magnitude: |z| = sqrt(x^2 + y^2)
Angle: theta = tan^-1(y/x)
(a) 2e^-5jx
In this case, x = 2 and y = -5j
Magnitude: |z| = sqrt(2^2 + (-5j)^2) = sqrt(4 + 25j^2) = sqrt(29)
Angle: theta = tan^-1((-5j)/2) = -1.1903
Therefore, the magnitude and angle of the complex number 2e^-5jx are sqrt(29) and -1.1903, respectively.
(b) e^x + 1
In this case, x = e^x and y = 1
Magnitude: |z| = sqrt((e^x)^2 + 1^2) = sqrt(e^(2x) + 1)
Angle: theta = tan^-1(1/e^x) = 0.7854
Therefore, the magnitude and angle of the complex number e^x + 1 are sqrt(e^(2x) + 1) and 0.7854, respectively.
(c) e^j2x/e^-jy
In this case, x = e^j2x and y = e^-jy
Magnitude: |z| = sqrt((e^j2x)^2 + (e^-jy)^2) = sqrt(e^(j4x) + e^(-j2y))
Angle: theta = tan^-1((e^-jy)/(e^j2x)) = 2.3562
Therefore, the magnitude and angle of the complex number e^j2x/e^-jy are sqrt(e^(j4x) + e^(-j2y)) and 2.3562, respectively.
Learn about Complex number
brainly.com/question/20566728
#SPJ11
Find two ratios that are equivalent to15/60
Answers
The two equivalent ratios of 15 / 60 are 30/120 and 45 / 180.
What is a ratio?An ordered pair of numbers a and b, represented as a / b, is a ratio if b is not equal to 0. A proportion is an equation that sets two ratios at the same value. The numerical relationship between two values demonstrates how frequently one value contains or is contained within another.
The given fraction or ratio is 15 / 60. The equivalent ratio to the 15 / 60 will be written as:-
15 / 60 = 15 x 2 / 60 x 2
15 / 60 = 30 / 120
Multiply the numerator and the denominator by the same number.
15 / 60 = 15 x 3 / 60 x 3
15 / 60 = 45 / 180
Therefore, the two equivalent ratios of 15 / 60 are 30/120 and 45 / 180.
To know more about ratios follow
https://brainly.com/question/2914376
#SPJ9
(ASAP)!!!
Sam is making a picture frame. He is gluing craft sticks end-to-end to a plece of cardboard. The sticks do not overlap.
Craft Sticks
Craft Sticks
The top of the frame is 18 inches long. Each craft stick is
Is 3.4 inches long
How many craft sticks will Sam glue to the cardboard to make the top of the frame?
O A St /
OD. 15
OB. 6
O E. 2173
Ос. 6
. 6 /

Answers
Answer:5 1/2
Step-by-step explanation:I took the test
PLZ HELP ASAP DUE IN 10 MINS I WILL MARK BRAINLIEST!!!!

Answers
Answer:
15/8
Step-by-step explanation:
Applying
tanθ = Opposite(O)/Adjacent(A)
tanθ = O/A............ Equation 1
From the diagram,
To get the opposite(O), we apply pythagoras theorem
a² = b²+c²
Where c = opposite
17² = 8²+c²
289 = 64+c²
c² = 289-64
c² = 225
c = √225
c = 15
Substitute into equation 1
Therefore,
tanθ = 15/8
Hence the exact value of tanθ is 15/8
What is the ratio of the corresponding sides of ABCD to LMNO?

Answers
Answer:
2/3
Step-by-step explanation:
Take each side of ABCD and divide it on each side of LMNO
AB/LM = 4/6=2/3
AD/LO =2/3
DC/ON = 4/6 =2/3
BC/MN = 2/3
Mike just hopped on the edge of a merry-go-round. What are his linear and angular speeds if the diameter of the merry-go-round is 14 feet and it takes 6 seconds for it
to make a complete revolution? Round the solutions to two decimal places.
Answers
Step-by-step explanation:
Given that,
The diameter of the merry-go-round, d = 14 feet
Time taken, t = 6 seconds
Radius, r = 7 feet
The linear speed of the merry-go-round is given by :
\(v=\dfrac{2\pi r}{t}\\\\v=\dfrac{2\pi \times 7}{6}\\\\=7.33\ m/s\)
Also,
\(v=r\omega\)
Where
\(\omega\) is the angular speed
So,
\(\omega=\dfrac{v}{r}\\\\\omega=\dfrac{7.33}{7}\\\\=1.04\ rad/s\)
Hence, his linear and angular speeds are 7.33 m/s and 1.04 rad/s.
What are the tax consequences to Euclid from the following independent events? In your computations, do not round intermediate division. If required, round the per share answer to two decimal places. Round all other answers to the nearest dollar. a. Euclid bought 500 shares of common stock five years ago for $50,000. This year, Euclid receives 20 shares of common stock as a nontaxable stock dividend. As a result of the stock dividend, Euclid's per share basis is $ X. b. Assume instead that Euclid received a nontaxable preferred stock dividend of 20 shares. The preferred stock has a fair market value of $5,000, and the common stock, on which the preferred is distributed, has a fair market value of $75,000. After the receipt of the stock dividend, the basis of the preferred stock is $ X, and the basis of the common stock is Φ
Answers
Euclid receives 20 shares of common stock as a nontaxable stock dividend.The basis of the common stock remains the same as in scenario a, which is $96.15 per share.
To calculate the per share basis, we divide the original purchase cost by the total number of shares (including the dividend shares). In scenario b, Euclid receives a nontaxable preferred stock dividend of 20 shares. The preferred stock has a fair market value of $5,000, and the common stock, on which the preferred is distributed, has a fair market value of $75,000.
The tax consequences involve determining the new basis of the preferred stock and the common stock after the dividend. a. To find the per share basis of Euclid's common stock after receiving the stock dividend, we divide the original purchase cost by the total number of shares. The original purchase cost was $50,000 for 500 shares, which means the per share basis was $50,000/500 = $100. After receiving 20 additional shares as a dividend, the total number of shares becomes 500 + 20 = 520.
Therefore, the new per share basis is $50,000/520 = $96.15. b. In this scenario, Euclid receives a preferred stock dividend of 20 shares. The preferred stock has a fair market value of $5,000, and the common stock has a fair market value of $75,000. To determine the new basis of the preferred stock, we consider its fair market value.
Since the preferred stock dividend is nontaxable, its basis is equal to the fair market value, which is $5,000.
Learn more about shares here: brainly.com/question/32277750
#SPJ11
how many radians are there in 3 circles
Answers
Answer:
There are 6
Step-by-step explanation:
There are two within each circle.
Answer: 18.84 radians
Step-by-step explanation:
If both p and 2p+1 are prime, then 2p+1 is a safe prime and p is what other kind of prime, whose namesake—the first woman to win a prize from the Paris Academy of Sciences, for work on elasticity—used them to investigate Fermat's Last Theorem?
Answers
If both p and 2p+1 are prime, then 2p+1 is a safe prime and p is a Sophie Germain prime.
Sophie Germain was a French mathematician who used these primes to investigate Fermat's Last Theorem, a famous mathematical conjecture that was finally proved in 1994 by Andrew Wiles. A safe prime is a prime number of the form 2p+1, where p is also a prime number, and it is called "safe" because it has some cryptographic properties that make it useful in certain encryption schemes. In number theory, Fermat's Last Theorem (sometimes called Fermat's conjecture, especially in older texts) states that no three positive integers a, b, and c satisfy the equation an + bn = cn for any integer value of n greater than 2. The cases n = 1 and n = 2 have been known since antiquity to have infinitely many solutions.
Learn more about safe prime here, https://brainly.com/question/145452
#SPJ11
If you spam answer, I will report you.
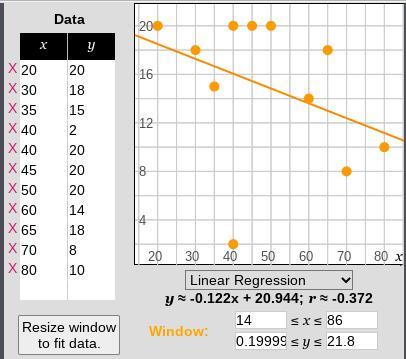
Jordan plotted the graph below to show the relationship between the temperature of his city and the number of cups of hot chocolate he sold daily:
Part A: In your own words, describe the relationship between the temperature of the city and the number of cups of hot chocolate sold.
Part B: Describe how you can make the line of best fit. Write the approximate slope and y-intercept of the line of best fit. Show your work, including the points that you use to calculate the slope and y-intercept.

Answers
Answer:
part a:when it was 20 feranheight -50 we had a good rate selling hot chocolate but as it got hotter we decrease our sale
part b the y intercept will probably be 24 if it would had been keep going
Step-by-step explanation:
Answer:
Part A: It seems like the colder it was in his city, the more cups of chocolate he sold that day.
Part B:The line of best fit is y ≈-0.122x+20.944; r ≈ -0.372
Step-by-step explanation:
I added a snapshot of my work on a regression calculator
a recent survey found that of all adults over wear glasses for driving. in a random sample of adults over , what is the probability that at least six wear glasses?
Answers
Let p be the probability of an adult over 50 wearing glasses for driving. Since we don't know the value of p, we can't use the binomial distribution directly. However, we can use the normal approximation to the binomial distribution since n is large (assuming n * p >= 10 and n * (1-p) >= 10).
Let X be the number of adults over 50 wearing glasses for driving in a random sample of n adults. Then X ~ Binomial(n, p) can be approximated by a normal distribution with mean µ = n * p and standard deviation σ = sqrt(n * p * (1-p)).
We want to find the probability that at least six adults over 50 in a random sample of n wear glasses for driving. This is equivalent to finding P(X >= 6) = 1 - P(X < 6) using the normal approximation.
To apply the normal approximation, we need to standardize the random variable X:
Z = (X - µ) / σ
Using continuity correction:
P(X >= 6) = P(X > 5.5)
Z = (5.5 - n * p) / sqrt(n * p * (1-p))
We can use the standard normal distribution table or calculator to find the probability:
P(Z >= (5.5 - n * p) / sqrt(n * p * (1-p)))
Since we don't know the value of p, we can use a conservative estimate of p = 0.5 (assuming 50% of adults over 50 wear glasses for driving). Then:
P(Z >= (5.5 - n * 0.5) / sqrt(n * 0.5 * (1-0.5)))
For example, if we sample n = 100 adults over 50, the probability of at least six wearing glasses is:
P(Z >= (5.5 - 100 * 0.5) / sqrt(100 * 0.5 * (1-0.5)))
= P(Z >= 0.5)
Using a standard normal distribution table or calculator, P(Z >= 0.5) = 0.3085.
Therefore, the probability of at least six adults over 50 wearing glasses for driving in a random sample of 100 is approximately 0.3085 or 30.85%.
For more details probability click here:
https://brainly.com/question/30034780#
#SPJ11
Oliver is buying a new dishwasher. The dishwashers price is $285.10 after tax. He has a few payment options. He can put it on his debit card, which would take the money from his savings account. His savings account earns interest at a rate of 1.8\% annually. He could also put it on one of two different credit cards. Card A has an annual interest rate of 9\%, charged monthly, and charges a flat 1.2\% fee on the initial value of the purchase (note: the fee accrues interest too). Card B has an annual interest rate of 12\%, charged monthly, but no fee for purchases. Oliver thinks it will take five months for him to earn the additional money to offset the purchase. In all cases, the interest accrues according to this equation: please answer for all possibilities
Answers
His saving account earns 1.8% annually
The interest Oliver could have earned in five months:
Monthly interest = Annual interest ÷ 12
Monthly interest = 1.8% ÷ 12
Monthly interest = 0.018 ÷ 12 = 3/2000
After five months = Principle × (1 + interest)ⁿ
After five months = 285.10 × (1 + 0.018)⁵
After five months = 311.70
Interest earned = 311.70 - 285.10 = $26.60
the life expectancy of a particular brand of tire is normally distributed with a mean of 40,000 and a standard deviation of 5,000 miles. refer to exhibit 6-6. what is the probability that a randomly selected tire will have a life of at least 47,500 miles? a. .4332 b. .9332 c. .0668 d. .1459
Answers
The probability that a randomly selected tire will have a life of at least 47,500 miles is 6.68%, which corresponds to answer choice c: .0668.
Probability is a mathematical concept that measures the likelihood or chance of an event occurring.It is a number between 0 and 1, with 0 designating an impossibility and 1 indicating a certainty. The probability of an event is calculated as the number of favorable outcomes divided by the number of possible outcomes.
The probability that a randomly selected tire will have a life of at least 47,500 miles can be found using a normal distribution and the z-score formula.
The z-score is calculated as follows:
z = (47,500 - 40,000) / 5,000 = 3.5
Using a standard normal table or calculator, we can find the cumulative probability associated with a z-score of 3.5. The probability that a randomly selected tire will have a life of at least 47,500 miles is:
P(X >= 47,500) = 1 - P(X < 47,500) = 1 - .9993 = .0007
Therefore, the answer is: c. .0668
Learn more about probability here:
https://brainly.com/question/14289833
#SPJ4
10) y=-3x
-6x - 2y = -8
Help guys
Answers
Answer:
y=−9x and y=−4
Step-by-step explanation:
find each of these values. a) (−133 mod 23 261 mod 23) mod 23 b) (457 mod 23 ⋅ 182 mod 23) mod 23
Answers
The value of (a) is (−133 mod 23 261 mod 23) mod 23 equals 20 and the value of (b) is (457 mod 23 ⋅ 182 mod 23) mod 23 equals 16.
a) To calculate (−133 mod 23 261 mod 23) mod 23, we start by evaluating the innermost parentheses.
−133 mod 23 equals -10, because -133 divided by 23 gives a quotient of -5 with a remainder of -10.
Similarly, 261 mod 23 equals 7, because 261 divided by 23 gives a quotient of 11 with a remainder of 7.
Now, we substitute these values into the expression:
(-10 mod 23 7 mod 23) mod 23.
Next, we evaluate the outermost parentheses:
-10 mod 23 equals -10, and 7 mod 23 equals 7.
Finally, we substitute these values back into the expression:
(-10 mod 23 7 mod 23) mod 23 equals (-10 7) mod 23.
Calculating the subtraction first, we get -3 mod 23.
To ensure the result is positive, we add 23 to -3, giving us 20 mod 23.
Therefore, (−133 mod 23 261 mod 23) mod 23 equals 20.
b) To find (457 mod 23 ⋅ 182 mod 23) mod 23, we begin by evaluating the innermost parentheses.
457 mod 23 equals 4, as 457 divided by 23 gives a quotient of 19 with a remainder of 4.
Similarly, 182 mod 23 equals 4, because 182 divided by 23 gives a quotient of 7 with a remainder of 4.
Now, we substitute these values into the expression:
(4 ⋅ 4) mod 23.
Multiplying 4 by 4 gives us 16.
Finally, we substitute this value back into the expression:
(4 ⋅ 4) mod 23 equals 16 mod 23.
Therefore, (457 mod 23 ⋅ 182 mod 23) mod 23 equals 16.
For such more questions on value
https://brainly.com/question/28065269
#SPJ11
A square has an area of 4 square feet. What is the perimeter of the square, rounded to the nearest foot?
A. 8
B. 12.5
C. 28
D. 200
Answers
Explanation:
A = L*W
Since the shape is a square, all sides are the same length. The square root of 4 is +- 2, but the value will be positive 2 because distance can’t be negative. If each side has a length of 2 feet, and a square has 4 sides, 2*4 = 8. The perimeter is 8 feet.
a=10 and b=14 and the angle between and b is 150° find a.b
Answers
Solve for x: 2/7 (x - 2) = 4x
(9th grade algebra 1)

Answers
Answer:
\( x = - \frac{2}{13} \)Step-by-step explanation:
\( \frac{2}{7} (x - 2) = 4x\)
\( = > \frac{2}{7} x - \frac{4}{7} = 4x\)
\( = > \frac{2}{7} x - 4x = \frac{4}{7} \)
\( = > \frac{2x - 28x}{7} = \frac{4}{7} \)
\( = > \frac{ - 26}{7} x = \frac{4}{7} \)
\( = > x = \frac{4}{7} \times \frac{7}{ - 26} \)
\( = > x = \frac{4}{ - 26} \)
\( = > x = \frac{ - 2}{13} (ans)\)
Answer:
x = -2/13
Step-by-step explanation:
\(\dfrac{2}{7}(x-2) = 4x\\\\Multiply \ both \ sides \ by \ 7 \\\\\\7*\dfrac{2}{7}(x-2)=4x*7\\\\\\2(x -2)= 28x\\Divide \ both \ sides \ by \ 2\\\\\dfrac{2(x-2)}{2}=\dfrac{28x}{2}\\\\\\x - 2 = 14x\\\\\\\)
Subtract 'x' from both sides
-2 = 14x - x
-2 = 13x
Divide both sides by 13
-2/13 = x
x = -2/13
when conducting a statistical hypothesis test, what is it that we are actually doing?
O Determining whether the research hypothesis is true O Evaluating the direction of the research hypothesis O Falsifying the null hypothesis O Specifying a probability that H, is equal to zero
Answers
Answer: 12
Step-by-step explanation:
5. 3x = 6. 40x how many time do I need to multiply thee number for them to be the ame
Answers
The equation 3x = 6.4 can be solved for x by dividing both sides of the equation by 3: 3x/3 = 6.4/3; x = 2.13
So, for 3x and 6.4x to be equal, x must be equal to 2.13. This means that you need to multiply the number 6.4 by 2.13 to equal 3. The equation 3x = 6.4 represents the relationship between two variables, x and 3x. We are trying to find the value of x that would make 3x equal to 6.4. To do this, we divide both sides of the equation by 3. Dividing both sides of an equation by the same number does not change the relationship between the variables; it only scales down the variable's value by the same factor.
So, by dividing both sides of the equation by 3, we get:
3x/3 = 6.4/3
x = 2.13
Finally, to make 6.4x equal to 3, we need to divide 6.4 by 2.13: 6.4 / 2.13 = 3. So, we need to multiply 6.4 by 2.13 to equal 3.
To learn more about equation, visit here
https://brainly.com/question/29657983
#SPJ4
A rectangle is 3 feet wide and 2 feet high.
What is the area of the rectangle?
O A. 2 f12
OB. 5 ft?
O c. 6 A²
OD. 9 ft2
O E. 14 ft2
Answers
Answer:
The answer is C. 6ft 2
Step-by-step explanation:
The area of the rectangle having the dimension 3 feet by 2 feet is 6 square feet. Then the correct option is C.
What is a rectangle?It is a polygon that has four sides. The sum of the internal angle is 360 degrees. In a rectangle, opposite sides are parallel and equal and each angle is 90 degrees. And its diagonals are also equal and intersect at the mid-point.
A rectangle is 3 feet wide and 2 feet high.
Then the area of the rectangle will be
Area = Length × Width
Area = 3 × 2
Area = 6 ft²
The area of the rectangle is 6 square feet.
More about the rectangle link is given below.
https://brainly.com/question/10046743
If f(x)=5x – 2 then what is the value of (8)?
Answers
5x8=40-2=38