Find the surface area of this cuboid.
4 cm
2 cm
2 cm
Answers
explanation:
Answer:
40 cm²
Step-by-step explanation:
Let,
length ( l ) = 4 cm
Height ( h ) = Width ( w ) = 2 cm
To find : Surface are of cuboid : -
Formula : -
Surface are of cuboid = 2 [ lh + hw + wl ]
Surface are of cuboid
= 2 [ ( 4 )( 2 ) + ( 2 )( 2 ) + ( ( 2 )( 4 ) ]
= 2 [ 8 + 4 + 8 ]
= 2 [ 20 ]
= 2 x 20
= 40 cm²
Related Questions
Given the function: \( m(w)=3 \sqrt[7]{w^{5}}-8 \sqrt[7]{w^{4}} \). Calculate: \( \frac{d(4)}{d w}= \) If you solution is a decimal, include two decimal places.
Answers
To calculate
�
(
4
)
�
�
dw
d(4)
, we need to find the derivative of the function
�
(
�
)
=
3
�
5
7
−
8
�
4
7
m(w)=3
7
w
5
−8
7
w
4
with respect to
�
w.
To find the derivative of the given function, we can use the power rule and the chain rule of differentiation. Applying the power rule, we differentiate each term separately and multiply by the derivative of the inner function.
The derivative of
3
�
5
7
3
7
w
5
is
3
7
⋅
5
�
5
7
−
1
=
15
7
�
−
2
7
7
3
⋅5w
7
5
−1
=
7
15
w
7
−2
.
Similarly, the derivative of
8
�
4
7
8
7
w
4
is
8
7
⋅
4
�
4
7
−
1
=
32
7
�
−
3
7
7
8
⋅4w
7
4
−1
=
7
32
w
7
−3
.
Combining these derivatives, we get
�
(
4
)
�
�
=
15
7
�
−
2
7
−
32
7
�
−
3
7
dw
d(4)
=
7
15
w
7
−2
−
7
32
w
7
−3
.
Since we are only interested in the derivative itself, we don't need to evaluate it at a specific value of w.
Learn more about function here: brainly.com/question/30660139
#SPJ11
Two ferries start moving towards each other from shores A and B. When they pass each other for the first time the distance to shore B is 100 meters. Once they reach their destinations and start traveling back at the same time. When they meet for the second time, the distance to shore A is 50 meters. What is the distance between the shores A and B? HELP will give brainliest! Its not 150 or 1700
Answers
The distance between the shores A and B is 250 meters.
Distance between shore A and BWidth of the river= D
Distance covered by boat A and B= a and b
Distance covered by A and B =Width of the river for the first meet.
D = a + b..............(1)
Hence:
b = 100 meters.............(2)
Sum of the distances traveled is 3times with width of the river as they both went 3 times after they first passed.
Second time= 50 meters
Hence:
3a = D+ 50...........(3)
Put the value of equation 2 into equation 1
3 ×100 = D+ 50
D=300-50
D =250 meters
Therefore the distance between the shores A and B is 250 meters.
Learn more about distance between the shores A and B here:https://brainly.com/question/14763249
#SPJ1
does the assumption of normality seem appropriate for the fill volume data
Answers
Based on the information provided in the question, it is not clear what the fill volume data refers to. However, the assumption of normality is generally considered appropriate for data sets that have a large enough sample size and follow a symmetrical, bell-shaped distribution.
If the fill volume data meets these criteria, then the assumption of normality may be appropriate. However, if the data is skewed or has a small sample size, the assumption of normality may not be appropriate and alternative statistical methods may need to be used.
It is important to always check the distribution of the data before making any assumptions about normality. This can be done through visual inspection of the data or by conducting formal tests, such as the Shapiro-Wilk test or the Kolmogorov-Smirnov test.
In conclusion, the assumption of normality may be appropriate for the fill volume data if it meets the criteria of a large sample size and a symmetrical, bell-shaped distribution. However, it is always important to check the distribution of the data before making any assumptions.
Know more about normality here:
https://brainly.com/question/30379223
#SPJ11
The ____________ assumption requires that all variation around the regression line should be equal at all possible values (levels) of the ___________variable.
A. control variance, dependent
B. constant variance, independent
C. constant variance, dependent
D. control variance, independent
Answers
The B) constant variance assumption requires that all variation around the regression line should be equal at all possible values (levels) of the independent variable.
The constant variance assumption, also known as homoscedasticity, requires that the variance of the residuals (i.e., the differences between observed and predicted values) should be approximately the same across all levels of the independent variable.
This assumption is necessary for valid statistical inference in linear regression analysis because violations of constant variance can result in biased estimates of the regression coefficients and incorrect hypothesis tests.
The independent variable is the variable that is used to predict the dependent variable. The constant variance assumption applies to the residuals at all possible values of the independent variable. Therefore, the correct answer is B. constant variance, independent.
For more questions like Variable click the link below:
https://brainly.com/question/17344045
#SPJ11
select all values that make the equation true 2(x-8)=x+5x
x= -4
x= -16
x= -2
x= -0
Answers
Answer:
x = -4
Step-by-step explanation:
2(x-8)=x+5x
Combine like terms
2(x-8) = 6x
Divide by 2
2(x-8)/2 = 6x/2
x-8 = 3x
Subtract x from each side
x-8-x = 3x-x
-8 = 2x
Divide by 2
-8/2 = 2x/2
-4 =x
2(x-8)=x+5x
2x-16=6x
Subtract 2 from each side
-16=4x
Divide both side by 4
-4=x
Find the nth term of this
quadratic sequence
2, 8, 18, 32, 50, ..
Answers
Answer:
The nth term is 2n^2.
Step-by-step explanation:
We see that each term is twice a square number. So it follows that
\(a(n )= 2 {n}^{2} \)
for n = 1, 2, 3,.....
Consider the points below. P(θ),−4,0),Q(5,1,−2),R(6,4,1) (a) Find a nonzero vector orthogonal to the plane through the points P,Q, and R. (b) Find the area of the triangle PQR.
Answers
(a) A nonzero vector orthogonal to the plane through the points P, Q, and R is (9, -17, 35). (b) The area of triangle PQR is \(\sqrt\)(811) / 2.
(a) To determine a nonzero vector orthogonal to the plane through the points P, Q, and R, we can first find two vectors in the plane and then take their cross product. Taking vectors PQ and PR, we have:
PQ = Q - P = (5, 1, -2) - (-4, 0, 0) = (9, 1, -2)
PR = R - P = (6, 4, 1) - (-4, 0, 0) = (10, 4, 1)
Taking the cross product of PQ and PR, we have:
n = PQ x PR = (9, 1, -2) x (10, 4, 1)
Evaluating the cross product gives n = (9, -17, 35). Therefore, (9, -17, 35) is a nonzero vector orthogonal to the plane through points P, Q, and R.
(b) To determine the area of triangle PQR, we can use the magnitude of the cross product of vectors PQ and PR divided by 2. The magnitude of the cross product is given by:
|n| = \(\sqrt\)((9)^2 + (-17)^2 + (35)^2)
Evaluating the magnitude gives |n| = \(\sqrt\)(811).
The area of triangle PQR is then:
Area = |n| / 2 = \(\sqrt\)(811) / 2.
To know more about nonzero vector refer here:
https://brainly.com/question/32673773#
#SPJ11
Math. Help I really need this giving brainliest to the person with the correct answer, please explain your answer..

Answers
Answer:
It is A
Step-by-step explanation:
You have 4 muffins or 4m with a 2 dollar off coupon so therefore it is 4m-2
Answer:
4m-2
Step-by-step explanation:
The cost, m, is subtracted by 2. There are 4 muffins being bought, so the term would be 4m, making the equation 4m-2.
Show that (n + 3)7 ∈ Θ(n7) for
non-negative integer n.
Proof:
Answers
To show that `(n + 3)7 ∈ Θ(n7)`, we need to prove that `(n + 3)7 = Θ(n7)`.This can be done by showing that `(n + 3)7 = O(n7)` and `(n + 3)7 = Ω(n7)` .Now, let's prove the two parts separately:
Proof for `(n + 3)7 = O(n7)`.
We want to prove that there exists a positive constant c and a non-negative constant k such that `(n + 3)7 ≤ cn7` for all `n ≥ k`.Using the Binomial theorem, we can expand `(n + 3)7` as:```
(n + 3)7
= n7 + 7n6(3) + 21n5(3)2 + 35n4(3)3 + 35n3(3)4 + 21n2(3)5 + 7n(3)6 + 37
≤ n7 + 21n6(3) + 21n5(3)2 + 35n4(3)3 + 35n3(3)4 + 21n2(3)5 + 7n(3)6 + n7
≤ 2n7 + 21n6(3) + 21n5(3)2 + 35n4(3)3 + 35n3(3)4 + 21n2(3)5 + 7n(3)6
≤ 2n7 + 84n6 + 441n5 + 2205n4 + 10395n3 + 45045n2 + 153609n + 729
```Thus, we can take `c = 153610` and `k = 1` to satisfy the definition of big-Oh notation. Hence, `(n + 3)7 = O(n7)`.Proof for `(n + 3)7 = Ω(n7)`We want to prove that there exists a positive constant c and a non-negative constant k such that `(n + 3)7 ≥ cn7` for all `n ≥ k`.Using the Binomial theorem, we can expand `(n + 3)7` as:```
(n + 3)7
= n7 + 7n6(3) + 21n5(3)2 + 35n4(3)3 + 35n3(3)4 + 21n2(3)5 + 7n(3)6 + 37
≥ n7
```Thus, we can take `c = 1` and `k = 1` to satisfy the definition of big-Omega notation. Hence, `(n + 3)7 = Ω(n7)`.
As we have proved that `(n + 3)7 = O(n7)` and `(n + 3)7 = Ω(n7)`, therefore `(n + 3)7 = Θ(n7)`.Thus, we have shown that `(n + 3)7 ∈ Θ(n7)`.From the proof, we can see that we used the Binomial theorem to expand `(n + 3)7` and used algebraic manipulation to bound it from above and below with suitable constants. This technique can be used to prove the time complexity of various algorithms, where we have to find the tightest possible upper and lower bounds on the number of operations performed by the algorithm.
Hence, we have shown that `(n + 3)7 ∈ Θ(n7)` for non-negative integer n.
To know more about Binomial theorem :
brainly.com/question/30095070
#SPJ11
what point appears to be the solution to the system of the equations shown in the graph
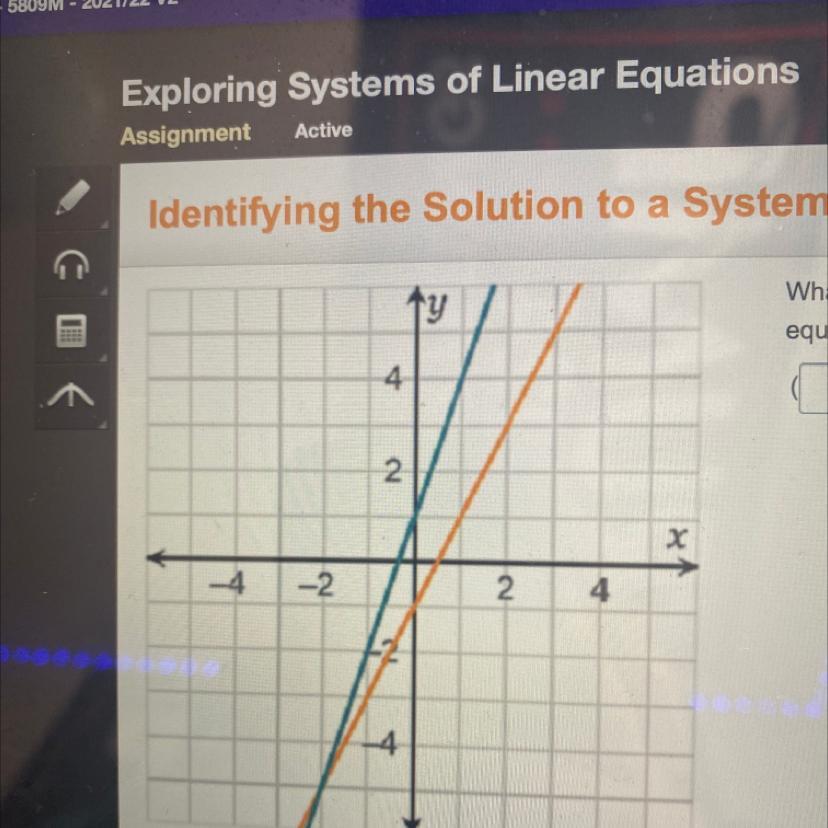
Answers
Answer:
(-2, -5) appears to fit both equation
Madelyn has a home-based business making and selling scented soaps. She initially spent $50 to purchase soap-making equipment, and the materials for each kilogram of soap cost $6. Madelyn sells the soap for $8 per kilogram. Eventually, she will sell enough soap to cover the cost of the equipment. Write a system of equations.
Answers
Answer:
25
Step-by-step explanation:
$50
Cost=$6
Selling price=$8
Profit=$8-$6=$2
50/2=25
Which expression has a value of 7/12?
Pls help

Answers
Answer:
the answer is the third one
Step-by-step explanation:
first of all you have to find the right denominator, it would be 12 since it's the lowest number 3 and 4 will go into. you then multiply 1 by 4, and 1 by 3, you then get 4/12 and 3/12, add them together and you get 7/12. hope this helped!
A car travels 60 miles in 50 minutes. Calculate the average speed of the car in mph.
Answers
Explanation:
To get the car's average speed, all you really need to know is
the total distance travelled by the car
the total time it needed to cover that distance
In your case, you know that the car travelled for a total of 60 miles and that it needed 50 minutes to do so.
Since you need to express the average speed in miles per hour, not miles per minute, you're going to have to use a simple conversion factor to get you from minutes to hours.
First, calculate the average speed in miles per minute
v
avg
=
d
total
t
total
v
avg
=
60 miles
50 minutes
=
1.2 mi/min
Now convert this value to miles per hour
1.2 miles
1
minute
⋅
60
minutes
1 hour
=
72 mph
Answer:
1.2mph
5x10=50
so it 1
if 2x5=10=.2
then 2x5=10+50=1.2 due to the .1=5
or just do 60/50=1.2
Step-by-step explanation:
The floor of a rectangular living room is 12 feet by 16 feet. What is the distance between
opposite corners of the living room?
Answers
Answer:
The width of Dana's living room is 16 feet.
Explanation:
Because Dana's living room is rectangular and we are given the length of one side and the length of the diagonal we can use the Pythagorean Theorem to solve this problem.
For a right triangle which the length, width and diagonal make up the Pythagorean Theorem states:
a
2
+
b
2
=
c
2
Let the length of 12 be
a
and because the diagonal is the hypotenuse of the triangle (the side opposite the right angle) we let
c
be 20. Substituting and solving gives:
12
2
+
b
2
=
20
2
144
+
b
2
=
400
144
−
144
+
b
2
=
400
−
144
0
+
b
2
=
256
b
2
=
256
√
b
2
=
√
256
b
=
16
help me baaaaaaaaaaaaaaaalls

Answers
aaaaa
Answer:
The correct scale faction the first pentagon dilated by to create the second pentagon is 1.25.
Step-by-step explanation:
Since the second pentagon is a dilation of the first pentagon, we can conclude these pentagons are similar, meaning the shape retained all of its previous angles, and all the corresponding sides are dilated by a common scale factor. Since we know the values of a pair of corresponding sides, we can use those to find the scale factor of dilation.
The corresponding side of the original pentagon is 4 units, and the corresponding side of the dilated pentagon is 5 units. Since the first pentagon dilated to become the second, in order to solve the scale factor the pentagon dilated by, we'd have to divide the side given on the second pentagon by the corresponding side of the first, which will be 5 ÷ 4 = 1.25. Therefore, that will be the scale factor the pentagon dilated by.
Have a great day! Feel free to let me know if you have any more questions :)
Rewrite 0.10 as a power of 10
Rewrite 0.07 as a product of a power of 10
Answers
\(0.10 = 10^{-1}\\\\0.07 = 7 \times 10^{-2}\)
Find the area of the triangle below.
Be sure to include the correct unit in your answer.

Answers
The area of the triangle with a base of 15 yards and height 6 yards is 45 sqaure yards.
What is the area of the triangle?A triangle is simply three-sided polygon having three edges and three vertices.
The area of a triangle can be expressed as:
Area = 1/2 × base × height.
From the image:
Base = 15 yards
Height = 6 yards
Area A = ?
To solve for the area of the triangle, plug the given values into the above formula:
Area = 1/2 × base × height
Area = 1/2 × 15 yd × 6 yd
Area = 15 yd × 3 yd
Area = 45 yd²
Therefore, the area is 45 sqaure yards.
Learn more about area of triangle here: brainly.com/question/29156501
#SPJ1
How do you write 0.0045 in scientific notation?
Answers
Answer:
\(4.5 x 10^{-3} \\\)Step-by-step explanation:
4x +5 (3x2-x) - (1+ x + 6x2)
need help solving this
Answers
Answer:
9x-2x-1
Step-by-step explanation:
Determine the differential equation that governs the system described by the following transfer function: \[ \frac{Y(s)}{U(s)}=\frac{2 s^{3}+4 s^{2}-6 s+1}{5 s^{4}-9 s^{3}-3 s^{2}+5} \] Select one: a.
Answers
The differential equation that governs the system is \(\[ 5 \frac{{d^4y}}{{dt^4}} - 9 \frac{{d^3y}}{{dt^3}} - 3 \frac{{d^2y}}{{dt^2}} + 5 \frac{{dy}}{{dt}} = 2 \frac{{d^3u}}{{dt^3}} + 4 \frac{{d^2u}}{{dt^2}} - 6 \frac{{du}}{{dt}} + u \].\)
To determine the differential equation that governs the system described by the given transfer function, we need to convert the transfer function from the Laplace domain (s-domain) to the time domain.
The given transfer function is \(\[ \frac{Y(s)}{U(s)}=\frac{2 s^{3}+4 s^{2}-6 s+1}{5 s^{4}-9 s^{3}-3 s^{2}+5} \].\)
To obtain the differential equation, we need to multiply both sides of the equation by the denominator of the transfer function to eliminate the fraction.
\(\[ Y(s) \cdot (5 s^{4}-9 s^{3}-3 s^{2}+5) = U(s) \cdot (2 s^{3}+4 s^{2}-6 s+1) \].\)
Expanding both sides and rearranging the terms, we obtain:
\(\[ 5 s^{4}Y(s) - 9 s^{3}Y(s) - 3 s^{2}Y(s) + 5Y(s) = 2 s^{3}U(s) + 4 s^{2}U(s) - 6 sU(s) + U(s) \].\)
Next, we need to take the inverse Laplace transform of both sides to convert the equation back to the time domain. This will give us the differential equation that governs the system.
Taking the inverse Laplace transform of both sides yields \(\[ 5 \frac{{d^4y}}{{dt^4}} - 9 \frac{{d^3y}}{{dt^3}} - 3 \frac{{d^2y}}{{dt^2}} + 5 \frac{{dy}}{{dt}} = 2 \frac{{d^3u}}{{dt^3}} + 4 \frac{{d^2u}}{{dt^2}} - 6 \frac{{du}}{{dt}} + u \].\)
Therefore, the differential equation that governs the system is \(\[ 5 \frac{{d^4y}}{{dt^4}} - 9 \frac{{d^3y}}{{dt^3}} - 3 \frac{{d^2y}}{{dt^2}} + 5 \frac{{dy}}{{dt}} = 2 \frac{{d^3u}}{{dt^3}} + 4 \frac{{d^2u}}{{dt^2}} - 6 \frac{{du}}{{dt}} + u \].\)
The differential equation governing the system described by the given transfer function is a fourth-order linear ordinary differential equation concerning the output variable y(t) and the input variable u(t).
Learn more about Laplace Transform here:
https://brainly.com/question/31689149
#SPJ11
vocabulary finding distances using similar triangles is called ?(indirect measurement or scale drawing)
Answers
The process of finding distances using similar triangles is called "indirect measurement." This technique is commonly used in fields such as engineering, architecture, and surveying.
Indirect measurement involves creating a scale drawing of the object or space in question, using known dimensions and ratios to create a proportional representation. This drawing is then used to create similar triangles, which can be used to calculate distances and other measurements.
For example, if you want to measure the height of a building, you can create a scale drawing of the building and its surroundings, and use trigonometry to calculate the height of the building based on the length of its shadow and the angle of the sun.
Indirect measurement can be a powerful tool for solving complex problems and making accurate measurements in situations where direct measurement is not possible. However, it does require a strong understanding of geometry and mathematical concepts, as well as careful attention to detail and accuracy in creating the scale drawing.
To learn more about measurement click on,
https://brainly.com/question/28989312
#SPJ4
What is x^3=8/27? pls help
Answers
Answer:x=2/3 =0.6667
Step-by-step explanation:
...
determine whether the given function is linear. if the function is linear, express the function in the form f(x) = ax b. (if the function is not linear, enter not linear.) f(x) = 5 1 5 x
Answers
The given function is linear, and it can be expressed in form f(x) = ax + b, f(x) = 1x + 0, or simply f(x) = x.
To determine if the given function is linear, we need to check if it can be expressed in form f(x) = ax + b, where a and b are constants.
The given function is f(x) = (5/1)x.
Let's rewrite the function in the required form:
f(x) = (5/5)x
Since 5/5 = 1, we can simplify the function to:
f(x) = 1x + 0
Here, a = 1 and b = 0.
So, the given function is linear, and it can be expressed in form f(x) = ax + b, f(x) = 1x + 0, or simply f(x) = x.
In Mathematics, a linear function is defined as a function that has either one or two variables without exponents. It is a function that graphs to a straight line. In case, if the function contains more variables, then the variables should be constant, or it might be the known variables for the function to remain it in the same linear function condition.
Visit here to learn more about function:
brainly.com/question/12431044
#SPJ11
Solve the system. Give answers (x, y, z)
x-5y+4z= -5
2x+5y-z= 14
-4x+ 5y-3z= -8
Answers
The given system of equations is inconsistent, and there is no solution (x, y, z) that satisfies all three equations.
First, we write the augmented matrix for the system of equations:
[1 -5 4 | -5]
[2 5 -1 | 14]
[-4 5 -3 | -8]
Next, we apply Gaussian elimination to transform the augmented matrix into row-echelon form or reduced row-echelon form.
Performing row operations, we get:
[1 -5 4 | -5]
[0 15 -9 | 24]
[0 0 1 | -1]
The row-echelon form reveals that the third equation is 0z = -1, which is inconsistent. Therefore, the system is inconsistent, and there is no solution that satisfies all three equations simultaneously.
In conclusion, the given system of equations is inconsistent, and there is no solution (x, y, z) that satisfies all three equations.
To learn more about Gaussian elimination click here, brainly.com/question/30400788
#SPJ11
Using long division method, show that x+2 is a factor of x power 3 + 8
Answers
Using the long division method, it is proved that (x + 2) is a factor of (x³ + 8), because the result of the remainder is 0.
To show that (x + 2) is a factor of (x³ + 8) using long division, we can divide (x³ + 8) by (x + 2) and see if the remainder is 0. If the remainder is 0, then (x + 2) is a factor of (x³ + 8). Here's how the long division would look:
x² - 2x + 4
x+2 | x³ + 0x² + 0x + 8
- (x³ + 2x²)
--------------------
-2x² + 0x + 8
- (-2x² - 4x)
---------------
4x + 8
- (4x + 8)
--------
0
Since the remainder is 0, we can conclude that (x + 2) is a factor of (x³ + 8).
See more about long division at https://brainly.com/question/25289437
#SPJ11
the maximum value of 4x - 6 is 54
Answers
Answer:
x=15
Step-by-step explanation:
1. Solve
4x-6=54
4x-6+6=54+6 <=== Add 6 to both sides
4x=60
4x÷4=60÷4 <=== divide by 4 on both sides
x=15
2. Check
4x15-6=54 <=== Replace x with 15 to check
60-6=54 <=== 4x15 equals 60
54=54 <=== 60-6 equals 54 and 54=54
so it is correct!!
5. What might be the dimensions of a
cylindrical container that holds 750 mL
of juice?
Answers
Answer:
radius of 10.9254843 and height of 10.9254843
Step-by-step explanation:
The equation for the volume of a cylinder is V = 2 pi r h
If V (volume) = 750, find for r and h
(I'm just going to make the radius and height the same thing)
750 = 2 pi r r
375 = pi r^2
119.366207 = r^2
10.9254843 = r
suppose the function xn 1 = (axn c) mod m is used to generate pseudo random number. assume : m=10,a=6,c=3, x0 = 3 , what is x1, x2 and x3 ?
Answers
The first three pseudo random numbers generated using the given values are x1 = 8, x2 = 1, and x3 = 9.
How to generate pseudo random number?Using the formula xn+1 = (a*xn + c) mod m, we can generate the first few pseudo random numbers as follows:
We are given:
m = 10, a = 6, c = 3, and x0 = 3
x1 = (6x0 + 3) mod 10
= (63 + 3) mod 10
= (18) mod 10
= 8
So, x1 = 8
Now, to find x2, we use x1 as the input:
x2 = (6x1 + 3) mod 10
= (68 + 3) mod 10
= (51) mod 10
= 1
So, x2 = 1
Finally, to find x3, we use x2 as the input:
x3 = (6x2 + 3) mod 10
= (61 + 3) mod 10
= (9) mod 10
= 9
So, x3 = 9
Therefore, the first three pseudo random numbers generated using the given values are x1 = 8, x2 = 1, and x3 = 9.
Learn more about pseudo random numbers
brainly.com/question/14547186
#SPJ11
When the positive integer k is divided by 9, the remainder is 4. Quantity A Quantity B The remainder when 3k is divided by 9 Quantity A is greater. Quantity B is greater. The two quantities are equal. The relationship cannot be determined from the information given.
Answers
The remainder when 3k is divided by 9 is 3. The relationship between Quantity A and Quantity B is that Quantity B is greater.
Given that k, when divided by 9, leaves a remainder of 4, we can express k as k = 9n + 4, where n is a positive integer. To find the remainder when 3k is divided by 9, we substitute the value of k: 3k = 3(9n + 4) = 27n + 12.
When 27n + 12 is divided by 9, the remainder is 3. Therefore, the remainder when 3k is divided by 9 is 3. Since the remainder when 3k is divided by 9 is less than the remainder when k is divided by 9, we can conclude that Quantity B (remainder when 3k is divided by 9) is greater than Quantity A (remainder when k is divided by 9).
To know more about remainders here: brainly.com/question/29019179
#SPJ11
What is the distance between the points (8,-4) and (-5, -4)
Answers
Answer: 13
Step-by-step explanation: