Finding the Slope of a Line from a Table
What is the slope of the linear function represented in
the table?
y
o
.

Hay 1200 gomas de borrar en total en las 4 cajas.

Para saber la cantidad de gomas de borrar que hay en 4 cajas, Don Jacinto necesita multiplicar la cantidad de gomas de borrar en cada caja (300) por la cantidad de cajas (4).

La operación que debe hacer es:

300 * 4

El resultado de esta multiplicación es:

300 * 4 = 1200

Es importante notar que en el escenario dado, la información inicial acerca de que Don Jacinto tiene 230 cuadernos no es relevante para encontrar el número de borradores en las 4 cajas. Solo necesitamos considerar el número de gomas de borrar en cada cuadro (300) y el número de cajas (4) para realizar la multiplicación y calcular el número total de gomas.

Para más preguntas de este tipo sobre la multiplicación

https://brainly.com/question/29793687

#SPJ8

Answer:

y = 3x + 2

Step-by-step explanation:

The equation of a line in slope- intercept form is

y = mx + c ( m is the slope and c the y- intercept )

Here m = 3 and c = 2 , then

y = 3x + 2 ← equation of line

Answer1000

Step-by-step explanation:

Answer:

The answer is 6

Step-by-step explanation:

6 - 3n + 3n

6  -   6n

 6

Option B, The measure of the major arc is 75° for a circle with center O, and the measure of a minor arc is 285°.

To find the measure of the major arc, follow these steps:

Recall that the total measure of a circle is 360 degrees.

Identify the measure of the given minor arc. In this case, it is 285 degrees.

Subtract the measure of the minor arc from 360 degrees to find the measure of the major arc.

Therefore, the measure of the major arc is:

360 degrees - 285 degrees = 75 degrees

So, the measure of the major arc is 75 degrees, which corresponds to option (b) in the given answer choices.

Learn more about minor arc at

https://brainly.com/question/20511450

#SPJ4

The question is -

In a circle with center O, the measure of a minor arc is 285°. What is the measure of the major arc?

a. 17°

b. 75°

c. 149°

d. 211°

Answer:

\(V=6.66\ ft^3\)

Step-by-step explanation:

The perimeter of the square base of a pyramid = 7.3 ft

The height of the pyramid, h = 6ft

The perimeter of a square is given by :

P = 4s, s is the side of square

7.3 = 4s

s = 1.825 ft

The volume of a pyramid is given by :

\(V=\dfrac{lbh}{3}\)

Here, l = b = 1.825 ft

So,

\(V=\dfrac{1.825\times 1.825\times 6}{3}\\\\V=6.66\ ft^3\)

So, the volume of the pyramid is equal to \(6.66\ ft^3\).

Answer:

39 maybe i don't know

Step-by-step explanation:

Answer:

8. a , c

9. b

10. c

Answer:

(-4,0)

Step-by-step explanation:

coordinate (x,y)

x=-4

y=0

Answer: (-4,0)

Step-by-step explanation: so its set up as (x,y) for coordinating points!

Answer:

Shown in the picture

The solution is 42.43.

What is Division method?

Division method is used to distributing a group of things into equal parts.

Given that;

The expression is;

'7 divided by 297'

Now, After divide we get;

7 ) 297 ( 42.428

   28

 --------

     17

     14

  --------

      30

      28

     -------

         20

          14

        -----

            60

            56

           ------

                4

Hence, 297 ÷ 7 = 42.428

After rounding we get;

297 ÷ 7 = 42.43

Thus, The solution is 42.43.

Learn more about the division visit:

https://brainly.com/question/672962

#SPJ1

The value of x is 4 for the isosceles triangle has a base that measures x+2 with legs that measure 4x-3 and 2x+5.

Therefore, an isosceles triangle has two equal sides as well as two equal angles. An equilateral triangle is one in which all of its sides are equal, and a scalene triangle is one in which none of its sides are equal. The characteristics of an isosceles triangle are as follows: There is agreement between two sides. The base of an isosceles triangle refers to the third side of the triangle, which is unequal to the other two sides. The two angles that are opposite the equal sides line up perfectly.

Here,

Since the adjacent sides of isosceles triangles are equal,

4x-3=2x+5

4x-2x=5+3

2x=8

x=4

The isosceles triangle has a base that measures x+2 and legs that measure 4x-3 and 2x+5, so x is equal to 4.

To know more about isosceles triangle,

https://brainly.com/question/28412104?referrer=searchResults

#SPJ4

I apologize, I am unable to create tables or upload scanned documents. However, I can assist you in calculating the amount each investor will receive in each year based on the given information.

To calculate the amount received by each investor in each year, we need to follow these steps:

Calculate the total profit earned by the bank in each year by subtracting the loss values from zero.

Year 1: 0 - (-78,000) = 78,000

Year 2: 0 - (-23,000) = 23,000

Year 3: 29,000

Year 4: 63,000

Year 5: 103,500

Calculate the total profit to be distributed among the investors in each year, which is 60% of the total profit earned by the bank.

Year 1: 0.6 * 78,000 = 46,800

Year 2: 0.6 * 23,000 = 13,800

Year 3: 0.6 * 29,000 = 17,400

Year 4: 0.6 * 63,000 = 37,800

Year 5: 0.6 * 103,500 = 62,100

Calculate the profit share for each investor based on their respective share of the investment.

Year 1:

Fahad: (30/100) * 46,800

Yashara: (50/100) * 46,800

Saud: (20/100) * 46,800

Fariha: (40/100) * 46,800

Younus: (25/100) * 46,800

Asif: (35/100) * 46,800

Similarly, calculate the profit share for each investor in the remaining years using the same formula.

By following the calculations above, you can determine the amount each investor will receive in each year based on their share of the investment.

To know more about Investor, visit

brainly.com/question/29797771

#SPJ11

The cost is the cost per pound times the number of pounds minus the coupon.

If \(p\) pounds are purchased, she will pay \(3.50p-3.25\).

If 3 pounds are purchased, she will pay 3.50(3)-3.25=7.25.

Answer:

1. ∠C ≅ ∠B  by definition of congruency

2. m∠Y by definition of obtuse angle

3. ∠C ≅ ∠A by definition of congruency

4. ∠N and ∠P  are  complementary angles by definition of complementary angles

Step-by-step explanation:

1. The given parameters are;

Statement                         \({}\)                        Reason

ΔABC is a right triangle                         \({}\)   Given

∠A is a right angle                         \({}\)            Given

m∠B = 45°                        \({}\)                          Given

m∠A + m∠B + m∠C = 180°                        \({}\) Sum of interior angles of a triangle

m∠C = 180° - (m∠A + m∠B)               \({}\)        

m∠C = 180° - (90° + 45°) = 45°               \({}\)     Substitution property

m∠C = 45° = m∠B                                 \({}\)      Substitution property

∠C ≅ ∠B                              \({}\)                        Definition of congruency

2. Statement                         \({}\)                      Reason

m∠X = 4·a + 2                        \({}\)                      Given

m∠Y = 21·a + 3                       \({}\)                      Given

∠X and ∠Y are linear pair                       \({}\)    Given

m∠X + m∠Y = 180°                     \({}\)                  Sum of angles of linear pair                  

4·a + 2 + 21·a + 3 = 180°              \({}\)                 Substitution property      

25·a + 5 = 180°                    \({}\)                          

a = (180 - 5)/25 = 7

m∠Y = 21 × 7 + 3 = 150°              \({}\)                 Substitution property      

m∠Y = 150° >  90

m∠Y is an obtuse angle             \({}\)                 Definition of obtuse angle

3. Statement                         \({}\)                         Reason

∠A and ∠B are complementary angles    \({}\)    Given

∠A + ∠B = 180°                        \({}\)                 Definition of complementary angles

∠B and ∠C are complementary angles    \({}\)    Given

∠B + ∠C = 180°                        \({}\)                 Definition of complementary angles

∠B + ∠C = ∠A + ∠B                 \({}\)                 Transitive property

∠C = ∠A                       \({}\)                      Reverse of addition property of equality

∠C ≅ ∠A                    \({}\)                        Definition of congruency

4. Statement                         \({}\)                Reason

ΔMNP is a right triangle                  \({}\)      Given

∠M is a right angle                     \({}\)           Given

∠M + ∠N + ∠P = 180°                        \({}\)     Sum of interior angles of a triangle

∠N + ∠P = 180° - ∠M                          \({}\)    Subtraction property of equality

∠N + ∠P = 180° - 90° = 90°                \({}\)    Substitution property of equality

∠N + ∠P = 90°                          \({}\)              Substitution property of equality

∠N and ∠P  are  complementary angles  \({}\)  Definition of complementary angles.

To estimate (mu1 - mu2) with a sampling error of 3.6 and 95% confidence, n1 and n2 should be determined based on the known standard deviations (sigma1 = 13, sigma2 = 22), to estimate (mu1 - mu2) with a sampling error of 6 and 99% confidence, n1 and n2 should be determined based on the range of each population (60 and to achieve a 90% confidence interval of width 1.3, the appropriate values of n1 and n2 need to be calculated.

a) To estimate (mu1 - mu2) with a sampling error of 3.6 and 95% confidence, we can use the formula:

\[ n = \left(\frac{{Z * \sqrt{{\sigma_1^2 + \sigma_2^2}}}}{{E}}\right)^2 \]

where Z is the Z-score corresponding to the desired confidence level (95% corresponds to Z = 1.96), sigma1 and sigma2 are the known standard deviations (sigma1 = 13, sigma2 = 22), and E is the desired sampling error (E = 3.6).

By plugging in the values, we get:

\[ n = \left(\frac{{1.96 * \sqrt{{13^2 + 22^2}}}}{{3.6}}\right)^2 \]

Simplifying this expression will give us the appropriate value for n1 and n2.

b) To estimate (mu1 - mu2) with a sampling error of 6 and 99% confidence, we can use the formula:

\[ n = \left(\frac{{Z * R}}{{2 * E}}\right)^2 \]

where Z is the Z-score corresponding to the desired confidence level (99% corresponds to Z = 2.58), R is the range of each population (R = 60), and E is the desired sampling error (E = 6).

By substituting the values, we get:

\[ n = \left(\frac{{2.58 * 60}}{{2 * 6}}\right)^2 \]

Simplifying this expression will give us the appropriate value for n1 and n2.

c) To achieve a 90% confidence interval of width 1.3, we can use the formula:

\[ n = \left(\frac{{Z * \sigma}}{{E}}\right)^2 \]

where Z is the Z-score corresponding to the desired confidence level (90% corresponds to Z = 1.645), sigma is the unknown standard deviation, and E is the desired interval width (E = 1.3).

Since the standard deviation (sigma) is unknown, we don't have enough information to calculate the appropriate values for n1 and n2.

Learn more about sampling error here:

brainly.com/question/15375591

#SPJ11

The critical value that should be used in constructing the confidence interval is 1.645.

We are constructing a 90% confidence interval, so the alpha level is 1 - 0.90 = 0.10. The z-score that corresponds to an alpha level of 0.10 is 1.645.

We can find the critical value using the following steps:

1. We can look up the z-score in a z-table.

2. We can use a statistical calculator to find the z-score.

The following is the z-table for a two-tailed test with an alpha level of 0.10:

```

z-score | Probability

------- | --------

1.645 | 0.9500

```

As we can see, the z-score that corresponds to an alpha level of 0.10 is 1.645.

We can also use a statistical calculator to find the z-score. For example, in Excel, we can use the following formula:

```

=NORMSINV(0.95)

```

This will return the value 1.645.

Once we have found the critical value, we can use it to construct the confidence interval.

to learn more about  confidence interval click here:

brainly.com/question/14366786

#SPJ11

To find the general solutions of the given differential equations using D-operator methods, we will use the fact that D-operator (D) represents differentiation with respect to x.

3.1 For the differential equation (D² - 5D + 6)y = e^(-x) + sin(2x), we can factorize the characteristic equation as (D - 2)(D - 3)y = e^(-x) + sin(2x). Solving each factor separately, we have: (D - 2)y = e^(-x) => y₁ = Ae^(2x) + e^(-x) (where A is a constant). (D - 3)y = sin(2x) => y₂ = Bsin(2x) + Ccos(2x) (where B and C are constants). The general solution is y(x) = y₁ + y₂ = Ae^(2x) + e^(-x) + Bsin(2x) + Ccos(2x).

3.2 For the differential equation (D² + 2D + 4)y = e^(2x)sin(2x), the characteristic equation is (D + 2i)(D - 2i)y = e^(2x)sin(2x). Solving each factor separately, we have: (D + 2i)y = e^(2x)sin(2x) => y₁ = Ae^(-2ix)e^(2x)sin(2x) = Ae^(2x)sin(2x)

(D - 2i)y = e^(2x)sin(2x) => y₂ = Be^(2ix)e^(2x)sin(2x) = Be^(2x)sin(2x)

The general solution for the first differential equation is y(x) = Ae^(2x) + e^(-x) + Bsin(2x) + Ccos(2x), and the general solution for the second differential equation is y(x) = Ae^(2x)sin(2x) + Be^(2x)sin(2x), where A, B, and C are constants.

To learn more about differential equation click here: brainly.com/question/31492438

#SPJ11

The general solution to the differential equation dy/dx = 1/3(sin x − xy^2), y(0)=5 is: y = ±√[(sin x - e^(x/2)/25)/x], if sin x - xy^2 > 0 and y(0) = 5

To solve this differential equation, we can use separation of variables.

First, we can rearrange the equation to get dy/dx on one side and the rest on the other side:

dy/dx = 1/3(sin x − xy^2)

dy/(sin x - xy^2) = dx/3

Now we can integrate both sides:

∫dy/(sin x - xy^2) = ∫dx/3

To integrate the left side, we can use substitution. Let u = xy^2, then du/dx = y^2 + 2xy(dy/dx). Substituting these expressions into the left side gives:

∫dy/(sin x - xy^2) = ∫du/(sin x - u)

= -1/2∫d(cos x - u/sin x)

= -1/2 ln|sin x - xy^2| + C1

For the right side, we simply integrate with respect to x:

∫dx/3 = x/3 + C2

Putting these together, we get:

-1/2 ln|sin x - xy^2| = x/3 + C

To solve for y, we can exponentiate both sides:

|sin x - xy^2|^-1/2 = e^(2C/3 - x/3)

|sin x - xy^2| = 1/e^(2C/3 - x/3)

Since the absolute value of sin x - xy^2 can be either positive or negative, we need to consider both cases.

Case 1: sin x - xy^2 > 0

In this case, we have:

sin x - xy^2 = 1/e^(2C/3 - x/3)

Solving for y, we get:

y = ±√[(sin x - 1/e^(2C/3 - x/3))/x]

Note that the initial condition y(0) = 5 only applies to the positive square root. We can use this condition to solve for C:

y(0) = √(sin 0 - 1/e^(2C/3)) = √(0 - 1/e^(2C/3)) = 5

Squaring both sides and solving for C, we get:

C = 3/2 ln(1/25)

Putting this value of C back into the expression for y, we get:

y = √[(sin x - e^(x/2)/25)/x]

Case 2: sin x - xy^2 < 0

In this case, we have:

- sin x + xy^2 = 1/e^(2C/3 - x/3)

Solving for y, we get:

y = ±√[(e^(2C/3 - x/3) - sin x)/x]

Again, using the initial condition y(0) = 5 and solving for C, we get:

C = 3/2 ln(1/25) + 2/3 ln(5)

Putting this value of C back into the expression for y, we get:

y = -√[(e^(2/3 ln 5 - x/3) - sin x)/x]

So the general solution to the differential equation dy/dx = 1/3(sin x − xy^2), y(0)=5 is:

y = ±√[(sin x - e^(x/2)/25)/x], if sin x - xy^2 > 0 and y(0) = 5

y = -√[(e^(2/3 ln 5 - x/3) - sin x)/x], if sin x - xy^2 < 0 and y(0) = 5

Note that there is no solution for y when sin x - xy^2 = 0.

Visit here to learn more about differential equation  : https://brainly.com/question/14620493
#SPJ11

4x-8=-2x + 20

4x+2x= 20 + 8

6x=28

x=28 : 6

x= 4,6

Arima model fits this pattern of autocorrelations is ARIMA(5,0,0).

The Autoregressive Integrated Moving Average (ARIMA) is an acronym that stands for the Autoregressive Integrated Moving Average model.

It is a statistical model for time series data that describes the correlation between points in a time series and provides insights into the temporal behavior of a variable.

The ARIMA model is a forecasting technique that uses time series data to make predictions. It is widely used in finance, economics, and other fields where it is necessary to predict the future behavior of a variable.

ARIMA models have the advantage of being able to capture trends, seasonality, and other patterns that can be difficult to detect using other methods.

The ARIMA model is made up of three parts:

the autoregressive (AR) component, the integrated (I) component, and the moving average (MA) component. The AR component takes into account the relationship between the current observation and the previous observations.

The I component deals with the trend and seasonality of the data. The MA component takes into account the relationship between the current observation and the previous errors.

For the series of length 169, we find that r1 = 0.41, r2 = 0.32, r3 = 0.26, r4 = 0.21, and r5 = 0.16. The ARIMA model that fits this pattern of autocorrelations is ARIMA(5,0,0), which means that there are five autoregressive terms in the model and no moving average or integrated terms are needed.

To learn more about Autoregressive Integrated Moving Average (ARIMA)

https://brainly.com/question/29744923

#SPJ11

Given:

A social security number contains nine digits.

As there are 10 digits from 0 to 9.

There are 9 positions and every position has 10 possibilities.

If repetition is allowed the number of ways are,

Answer: the number of ways are,

Answer:2r

Step-by-step explanation:

1/4( -12+4r)

1/4(12)= -3

1/4(4r)= 1r

-3+1r= 2r

Answer:

Step-by-step explanation:

-3+1r

The written form of the quotient as a simplified fraction as required in the task content is; Choice Choice B; 18 1/2.

It follows from the task content that the quotient as given in the task content is; 851/46.

On this note, it follows that the result of the division is; 18 with a remainder of 23.

Hence, we have; 18 23/46 which is equivalent to 18 1/2.

Read more on simplified fractions;

https://brainly.com/question/23965611

#SPJ1

The Perimeter of polygon ABCD = 21.6 units.

The perimeter of figure ABCD = sum of all its sides = AB + BC + CD + AD.

To find each of the sides, apply the distance formula, d = \(\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\).

A(-5, 5)

B(0, 3)

C(-2, -2)

D(-7, 0)

AB = √[(0−(−5))² + (3−5)²]

AB = √29

AB ≈ 5.4 units

BC = √[(0−(−2))² + (3−(−2))²]

BC = √29

BC ≈ 5.4 units

CD = √[(−7−(−2))² + (0−(−2))²]

CD = √29

CD ≈ 5.4 units

AD = √[(−7−(−5))² + (0−5)²]

AD = √29

AD ≈ 5.4 units

The Perimeter of polygon ABCD = 5.4 + 5.4 + 5.4 + 5.4 = 21.6 units.

Therefore, the Perimeter of polygon ABCD = 21.6 units.

Learn more about the perimeter of a figure on:

https://brainly.com/question/28164415

#SPJ1

The number of gallons that is obtained is 5389 gallons.

We have to look at the fact that we are told that the water sprinkler that sprays out 35 feet from the center operates long enough to cover a circular area. Thus the radius of the circle would be seen as 35 feet . The area of the circle would be;

A = πr^2

r = radius

Thus;

A = 3.142 * (35)^2

A = 3849 feet^2

The total gallons that we would need to use can be obtained from;

1.4 gallons of water per square foot *  3849 feet^2

= 5389 gallons

Learn more about area of a circle:https://brainly.com/question/28642423

#SPJ1

Answer:

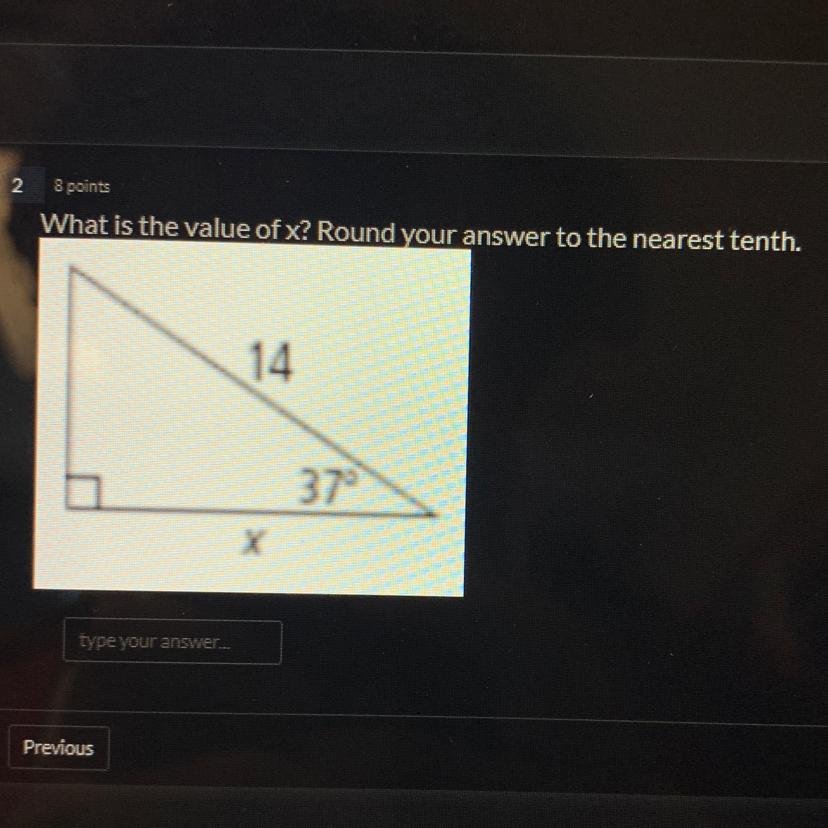
4 is the answer

Step-by-step explanation:

x+2-x+2= 4

Answer:

If you are talking about subtracting it is 4

Step-by-step explanation:

(x+2)-(X-2)=4

Step-by-step explanation:

The slope-intercept form of an equation of a line:

\(y=mx+b\)

\(m\) - slope

\(b\) - y-intercept

\(11)\ m=-1,\ b=2\\\\y=-x+2\\\\12)\ m=-\dfrac{3}{2},\ b=3\\\\y=-\dfrac{3}{2}x+3\\\\13)\ m=3,\ y=-2\\\\y=3x-2\\\\14)\ y=\dfrac{3}{4},\ b=1\\\\y=\dfrac{3}{4}x+1\\\\15)\ m=\dfrac{1}{2},\ b=1\\\\y=\dfrac{1}{2}x+1\\\\16)\ m=-\dfrac{2}{5},\ b=0\\\\y=-\dfrac{2}{5}x\\\\17)\ m=-7,\ b=2\\\\y=-7x+2\\\\18)\ m=\dfrac{4}{3},\ b=-4\\\\y=\dfrac{4}{3}x-4\)

19) and 20) you have in other your question.