for a little over two weeks in february 2005, the gates by christo and jeanne-claude were installed in new york city's central park. how long had they worked on the project?
Answers
Christo and Jeanne-Claude worked for over 26 years on "The Gates," a large-scale public art installation in Central Park consisting of thousands of saffron-colored fabric panels along 23 miles of walkways, which was on display for 16 days in February 2005.
Christo and Jeanne-Claude were artists known for their large-scale public art installations. "The Gates" was a project they worked on for over 26 years, from its conception in 1979 to its installation in Central Park in February 2005. The installation involved placing thousands of saffron-colored fabric panels along 23 miles of walkways in the park, creating a visually stunning and immersive experience for visitors.
The project was met with both praise and criticism, but it ultimately succeeded in bringing people together to appreciate art in a public space. The installation remained in place for 16 days before it was dismantled.
Learn more about Christo and Jeanne-Claude here
brainly.com/question/3500362
#SPJ4
Related Questions
How do I do these step by step thank u

Answers
Answer:
1) 24
2) 25
Step-by-step explanation:
Given:
2a + 3b = 8
4x + 10y = 50
.
1)
\( \frac{6a + 9b}{2a + 3b} = \frac{3(2a + 3b)}{2a + 3b} = 3\)
Since the second expression is 3 times greater then the previous one, the product will also be 3 times greater:
6a + 9b = 3 × 8 = 24
.
2)
\( \frac{2x + 5y}{4x + 10y} = \frac{2x + 5y}{2(2x + 5y)} = \frac{1}{2} = 0.5\)
The second expression's value is two times smaller than the first one's, so the product will also be two times smaller:
2x + 5y = 0,5 × 50 = 25
HELP ME PLEAS ON PLATO I WANT TO FINISH SUMMER SCHOOL PLEASE ITS A LIVING HELL

Answers
The parabolas with respect to their positions are y = f(x + 3), y = f(x + 2), y = f(x - 1/2), y = f(x - 4) and f = (x - 7)
How to arrange the parabolas?The parent function is given as:
f(x) = x^2
The parabolas are given as:
y = f(x - 4), y = f(x + 3), y = f(x - 7), y = f(x + 2) and f = (x - 1/2)
The transformation from the parent function to each of the parabola is a horizontal translation.
This means that the y coordinate of their vertices are the same.
Arrange the parabolas starting from the left translations
y = f(x + 3), y = f(x + 2), y = f(x - 1/2), y = f(x - 4) and f = (x - 7)
Hence, the parabolas with respect to their positions are y = f(x + 3), y = f(x + 2), y = f(x - 1/2), y = f(x - 4) and f = (x - 7)
Read more about parabolas at:
https://brainly.com/question/21685473
#SPJ1

How do you write 0.277777777777...... as a fraction?
Answers
Answer:
27777/100000
Step-by-step explanation:
i hope its right
What is the diffrent between statistical significance and practical significance
Answers
The concept of statistical significance is mathematically defined and widely accepted by those who use it to assess the usefulness of scientific claims.
However, there is no single definition for practical significance. It is subjective in nature, experiential, and each person decides what it is based on their own experiences.
Thus, it is clear that while Practical significance lacks a common definition, statistical significance has a mathematical definition and is widely accepted by those who use it to assess the usefulness of scientific claims. It is a subjective concept that each person decides for themselves based on their personal experiences.
To learn more about statistical significance visit link :
brainly.com/question/8362659
#SPJ4
What letter is located at approximately right answers only
√
22
A E
B F
C G
D H
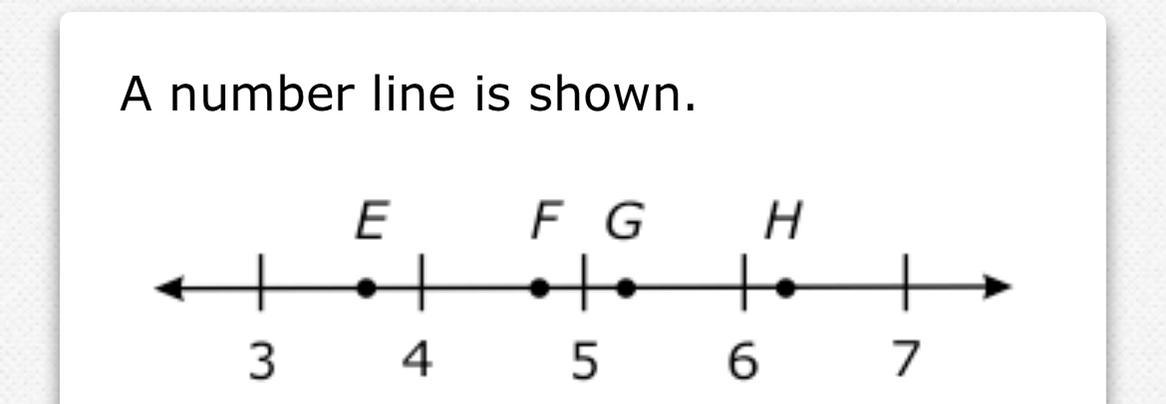
Answers
Answer: B
Step-by-step explanation:

Find the number of zeroes at the end of 26!-25!
Answers
Answer:
26-25=1
so none
I guess that is the answer
Which expression represents a negative number?
A. -4/5(-8/9)
B. 4/5*8/9
C. -4/5*8/9
D. -9/8*(-3/4)
Answers
Its C.
Negative x Negative is positive, so its not A or D.
Positive x Positive is Positive, so its not B either.
C is your option because Positive x Negative is Negative, and vice verca.
Answer:
C
Step-by-step explanation:
this is because a negative number multiplied with a negative number gives you a positive, a positive number multiplied with a positive number gives you a positive but a negative number multiplied with a positive gives you a negative so the answer is C.
for more information watch videos about the rules of positive and negative numbers.
pls help i am braindead rn and i dont know how to do this atm thank u!

Answers
Answer: Table 3
Step-by-step explanation:
exponential relationship means the y value is increasing/decreasing exponentially
In table 1: the value of y is NOT increasing/decreasing exponentially as 2 is being added continuously (linear), so not table 1
In table 2: the value of y is NOT increasing/decreasing exponentially as 4 is being subtracted continuously (linear), so not table 2
In table 3: the value of y IS increasing exponentially as the y variable is multiplied by 2, so table 3
In table 4: the value of y is NOT increasing exponentially as the y variable is only being increased by 5 constantly (linear), so not 4
Suppose that y varies directly with x and inversely with z, and y = 4 when x = 8 and z = 14. find z when x = 36 and y = 6. first, what is the equation of variation? y =
Answers
The value of z if x = 36 and y = 6 is 42
Direct and inverse variationSuppose that y varies directly with x and inversely with z, hence the required expression will be:
y = kx/z
If y = 4 when x = 8 and z = 14 then;
4 = k(8)/14
8k = 56
k = 7
To find z when x = 36 and y = 6, we will have:
6 = 7(36)/z
1 = 7(6)/z
z = 42
Hence the value of z if x = 36 and y = 6 is 42
Learn more on variation here: https://brainly.com/question/6499629
#SPJ1
Answer:
Keep it simple. Answer:
y=kx/z
k=7
z=42
Step-by-step explanation:
what 10+10+10+10+10+10+9=
Answers
10+10+10+10+10+10+9=69
Answer:
its simple girl;;;;;
ans=69
(Ohh dear don't leave the question with this big pts!!! )
The box plot represents the number of tickets sold for a school dance.
A horizontal line labeled Number of Tickets sold that starts at 8, with tick marks every one unit up to 30. The graph is titled Tickets Sold for A Dance. The box extends from 17 to 21 on the number line. A line in the box is at 19. The lines outside the box end at 10 and 27.
Which of the following is the appropriate measure of center for the data, and what is its value?
The mean is the best measure of center, and it equals 19.
The median is the best measure of center, and it equals 4.
The median is the best measure of center, and it equals 19.
The mean is the best measure of center, and it equals 4.
Answers
The median is the best measure of center, and it equals 19.
Define medianThe median is a measure of central tendency that represents the middle value of a dataset when it is arranged in order. Specifically, it is the value that separates the dataset into two halves, with half of the data falling below the median and half of the data falling above the median.
The appropriate measure of center for the given data is the median, which is the middle value when the data is arranged in numerical order.
From the given box plot, we can see that the box extends from 17 to 21 on the number line, with a line in the box at 19. This means that 50% of the data falls between 17 and 21, with the middle value (median) being 19.
Therefore, the correct answer is:
The median is the best measure of center, and it equals 19.
To know more about line, visit:
https://brainly.com/question/30003330
#SPJ1
Which function has a minimum and is transformed to the right and down from the parent function, f(x)
Answers
The parent function of a quadratic equation is f(x) = x². The function that is transformed to the right and down from the parent function with a minimum is given by f(x) = a(x - h)² + k.
The equation has the same shape as the parent quadratic function. However, it is shifted up, down, left, or right, depending on the values of a, h, and k.
For a parabola to have a minimum value, the value of a must be positive. If a is negative, the parabola will have a maximum value.To find the vertex of the parabola in this form, we use the vertex form of a quadratic equation:f(x) = a(x - h)² + k, where(h, k) is the vertex of the parabola.The vertex is the point where the parabola changes direction. It is the minimum or maximum point of the parabola. In this case, the parabola is transformed to the right and down from the parent function, f(x) = x². Therefore, h > 0 and k < 0.
Know more about quadratic equation here:
https://brainly.com/question/12186700
#SPJ11
“arizona has the highest death rate for asthma in the country. therefore, it is unsafe to go to arizona if you have asthma.”
(statistics). (misuses/misinterpretations).
Answers
Answer:
that is funny. That is where my dad was born and now he has horrible breathing issues
Step-by-step explanation:
Answer:
, Im in Arizona. Also what's this question about, that you disagree or agree?
Use a protractor to draw a quadrilateral so that three of its four sides measure 5 centimeters each. The angles between these sides have measures of 100° and 110° What is the length of the fourth side? o 5 cm 0 6.5 cm 0 7.5 cm O 8 cm
Answers
The length of the third side of the quadrilateral is 7.5 cm.
QuadrilateralA quadrilateral is a polygon that has four sides and four angles. The sum of interior angles in a quadrilateral is 360°. Types of quadrilateral are square, rectangle, rhombus, kite and so on.
Let x represent the length of the third side, hence:
x = 5 + (5 * sin(20)) + (5 * sin(10)) = 7.5 cm
The length of the third side of the quadrilateral is 7.5 cm.
Find out more on Quadrilateral at: https://brainly.com/question/16691874
Answer:
I just took the k12 quiz its 7.5 100%
Step-by-step explanation:
Yoshi buys a T-shirt for $15.95 and socks for $7.95, He pays with a gift card that has $31.19 left on it. What will the new balance on the gift card be?
Answers
the answer were to the question is 7.29
Which is the only center point that lies on the edge of a triangle?
the incenter of a right triangle
the incenter of an obtuse triangle
the circumcenter of a right triangle
the circumcenter of an obtuse triangle
Answers
Answer:
Circumcenter
Circumcenter is the only center point on the edge of a triangle. this is true in the case of the right-angle triangle. It exactly lies on the midpoint of the hypotenuse. Circumcenter is the point of intersection of the perpendicular bisector of sides of triangles.
The circumcenter of a right triangle is the only center point that lies on the edge of a triangle.
What is Coordinates?A pair of numbers which describe the exact position of a point on a cartesian plane by using the horizontal and vertical lines is called the coordinates.
Given that;
To find the only center point that lies on the edge of a triangle.
Now, We know that;
The circumcenter of a right triangle is the only center point that lies on the edge of a triangle.
Thus, The correct statement is,
The circumcenter of a right triangle.
Learn more about the coordinate visit:
https://brainly.com/question/24394007
#SPJ7

A person leaves their home at noon walking toward a restaurant located 16 miles away If the person walks constantly at 2.5 miles per hour, at what time will the person arrive at the restaurant?
Answers
A person leaves their home at noon walking toward a restaurant located 16 miles away If the person walks constantly at 2.5 miles per hour, the person arrive at the restaurant at 6:24 PM.
To determine the time it will take for the person to arrive at the restaurant, we can use the formula:
Time = Distance / Speed
Given:
Distance to the restaurant = 16 miles
Walking speed = 2.5 miles per hour
Substituting these values into the formula, we can calculate the time:
Time = 16 miles / 2.5 miles per hour
Time = 6.4 hours
Since we know the person leaves their home at noon, we can add the calculated time to noon to find the arrival time:
Arrival Time = Noon + 6.4 hours
To express the arrival time in a standard 12-hour clock format, we convert 6.4 hours into hours and minutes:
0.4 hours * 60 minutes/hour = 24 minutes
So, the person will arrive at the restaurant at approximately 6 hours and 24 minutes after noon.
Therefore, the person will arrive at the restaurant at approximately 6:24 PM.
To know more about Speed, visit:
https://brainly.com/question/17661499
#SPJ11
SSA triangles always have two solutions.
(A.) True
(B). False
Answers
Answer:
No, Ssa triangle always not have two solutions
Step-by-step explanation:
the solution can be one, two or there will be no dolution. So this statement is false.
the altitude at which the atmospheric pressure reaches 5 is approximately'
Answers
If the atmospheric pressure of 5 is measured in millibars, it would correspond to a pressure of 0.5 kPa or a pressure of approximately 1.47 inHg. However, this value should be considered as a rough estimate and would still be subject to the variability of atmospheric conditions.
It is not possible to accurately determine the altitude at which atmospheric pressure reaches 5 without additional information about the context and conditions of the atmosphere being considered. The atmospheric pressure at a given altitude can vary widely depending on factors such as temperature, humidity, and atmospheric composition.
However, in general, atmospheric pressure decreases with increasing altitude. At sea level, the standard atmospheric pressure is about 101.3 kilopascals (kPa). At an altitude of approximately 5.6 kilometers, the atmospheric pressure drops to around 50 kPa. However, this value can vary greatly depending on the conditions of the atmosphere being considered.
It is worth noting that atmospheric pressure is typically measured in units of kilopascals (kPa), millibars (mb), or inches of mercury (inHg). The conversion between these units is as follows:
1 kPa = 10 mb = 0.2953 inHg
Click the below link, to learn more about atmospheric pressure:
https://brainly.com/question/30166820
#SPJ11
Your question seems incomplete. I could not find the exact question details online so I answered in general.
A researcher wants to set up a regression equation where Y is a function X. Evaluate the researcher’s options given the following scenarios: (3)
i. Y is I(0); X is I(0)
ii. Y is I(2); X is I(0)
iii. Y is I(1); X is I(1); and the error term is I(0).
Answers
The appropriate regression model depends on the stationarity properties of both the dependent and independent variables, as well as the error term. The researcher can use a standard OLS regression model with first-order differencing of both Y and X.
In the first scenario, both Y and X are I(0), which means they are stationary time series. In this case, the researcher can perform a standard linear regression analysis, as the stationary series would lead to a stable long-run relationship. The answer from this model will be reliable and less likely to suffer from spurious regressions. In the second scenario, Y is I(2) and X is I(0). This implies that Y is integrated of order 2 and X is stationary. In this case, the researcher should first difference Y twice to make it stationary before performing a regression analysis. However, this approach might not be ideal as the integration orders differ, which can lead to biased results.
In the third scenario, Y and X are both I(1) and the error term is I(0). This indicates that both Y and X are non-stationary time series, but their combination might be stationary. The researcher should employ a co-integration analysis, such as the Engle-Granger method or Johansen test, to identify if there is a stable long-run relationship between Y and X. If co-integration is found, then an error correction model can be used for more accurate predictions.
To know more about regression visit:-
https://brainly.com/question/28168620
#SPJ11
Identify each equation has no solution, one solution, or infinitely many solutions -2x + 3 = -3x + 2- 2x + 3 = -2x + 3- 2x + 3 = 2x + 3
Answers
For equation 1;
\(\begin{gathered} -2x+3=-3x+2 \\ \text{Collect like terms} \\ -2x+3x=2-3 \\ x=-1 \\ \text{This equation has one solution} \end{gathered}\)For equation 2;
\(\begin{gathered} -2x+3=-2x+3 \\ \text{Collect like terms} \\ -2x+2x=3-3 \\ 0=0 \\ \text{This equation has infinitely many solutions} \end{gathered}\)For equation 3;
\(\begin{gathered} -2x+3=2x+3 \\ \text{Collect like terms} \\ -2x-2x=3-3 \\ -4x=0 \\ \text{Divide both sides by -4} \\ x=0 \\ \text{This equation has only one solution} \end{gathered}\)Zain bought ice cream and sherbet at the grocery store and is placing the containers in the freezer. The container of ice cream was sold in a 10" × 14" × 12" container, and the sherbet was sold in an 11" × 18" × 9" container. How much less space does the container of ice cream occupy than the container of sherbet? *
Answers
Answer:
102 in^3.
Step-by-step explanation:
Volume of the ice cream container = 10*14*12 = 1680 in^3.
Volume of the sherbet container = 11*18^9 = 1782 in^3.
So the answer is 1782 - 1680
= 102 in^3.
find the surface area of the roof of the doll house.

Answers
Answer: 480in^2
Step-by-step explanation:
The formula for the area of a triangle: \(A = \frac{h*a}{2}\)
There are 4 sides on the roof so \(A = 4*\frac{12in * 20in}{2} = 480in^{2}\)
Instructions: After interacting on your own with the model above press the "Reset" button. Use the Demand Slider in the "Settings" to have your demand curve match the equation {Demand: P=$6.00-0.100(Qd)}.
a. What is the total revenue when the price is $2.00? $
b. What is the total revenue when the price is $1.00? $
c. Is demand price elastic, price inelastic or unit elastic between these two prices:
(Click to select)
Instructions: Use the Demand Slider in the "Settings" to have your demand curve match the equation {Demand: P=$4.80-0.060(Qd)}.
d. What is the optimal price and quantity to maximize the total revenue? P = $
, Q =
, TR = $
e. What is the total revenue when the price is $3.00 and quantity is 30? $
f. What is the total revenue when the price is $1.80 and quantity is 50? $
g. If demand is price elastic and the market price is $3.00 what can be done to increase the total revenue?
Answers
Given the demand function P=$6.00-0.100(Qd)
a) at a price of $2.00, the quantity demanded is 40 units, thus, Total Revenue = $80
b) at a price of $1.00, the quantity demanded is 50 units, thus, Total Revenue = $50
c) Since the price elasticity of demand is less than 1, demand is price inelastic between these two prices.
Given the demand function P=$4.80-0.060(Qd)
d)
The optimal price to maximize total revenue is $2.40 and the optimal quantity is 40 units. TR = $96
e) When the price is $3.00 and the quantity demanded is 30, TR = $90
f) When the price is $1.80 and the quantity demanded is 50, the total revenue = $90
g) If demand is price elastic and the market price is $3.00, total revenue can be increased by reducing the price.
What is a demand function?
A demand function is a mathematical equation that describes the relationship between the price of a good or service and the quantity of that good or service that consumers are willing and able to purchase at that price.
a. When the price is $2.00, the quantity demanded can be calculated by setting Qd equal to 2.00 in the demand equation:
Qd = (6.00 - 0.100Qd)
2.00 = (6.00 - 0.100Qd)
0.100Qd = 4.00
Qd = 40
Therefore, at a price of $2.00, the quantity demanded is 40 units. The total revenue can be calculated by multiplying the price by the quantity demanded:
Total Revenue = Price x Quantity Demanded = 2.00 x 40 = $80
b. When the price is $1.00, the quantity demanded can be calculated in the same way:
Qd = (6.00 - 0.100Qd)
1.00 = (6.00 - 0.100Qd)
0.100Qd = 5.00
Qd = 50
Therefore, at a price of $1.00, the quantity demanded is 50 units. The total revenue can be calculated as:
Total Revenue = Price x Quantity Demanded = 1.00 x 50 = $50
c. To determine the price elasticity of demand between these two prices, we need to compare the percentage change in quantity demanded to the percentage change in price.
At a price of $2.00, the quantity demanded is 40 units. At a price of $1.00, the quantity demanded is 50 units. The percentage change in quantity demanded can be calculated as:
% Change in Quantity Demanded = (New Quantity Demanded - Old Quantity Demanded) / Old Quantity Demanded x 100%
= (50 - 40) / 40 x 100% = 25%
The percentage change in price can be calculated as:
% Change in Price = (New Price - Old Price) / Old Price x 100%
= (1.00 - 2.00) / 2.00 x 100% = -50%
The price elasticity of demand between these two prices can be calculated as:
Price Elasticity of Demand = % Change in Quantity Demanded / % Change in Price
= 25% / -50%
= -0.5
Since the price elasticity of demand is less than 1, demand is price inelastic between these two prices. This means that a change in price will result in a proportionately smaller change in quantity demanded.
d). To find the optimal price and quantity to maximize total revenue, we need to take the derivative of the total revenue function with respect to quantity and set it equal to zero.
Total Revenue = Price x Quantity Demanded
TR = (4.80 - 0.060Qd)Qd
TR = 4.80Qd - 0.060Qd^2
Taking the derivative of TR with respect to Qd:
dTR/dQd = 4.80 - 0.120Qd
Setting dTR/dQd equal to zero:
4.80 - 0.120Qd = 0
Qd = 40
Substituting Qd = 40 into the demand equation to find the optimal price:
P = 4.80 - 0.060(40)
P = 2.40
Therefore, the optimal price to maximize total revenue is $2.40 and the optimal quantity is 40 units. The total revenue can be calculated as:
TR = P x Q = 2.40 x 40 = $96
e. When the price is $3.00 and the quantity demanded is 30, the total revenue can be calculated as:
TR = P x Q = 3.00 x 30 = $90
f. When the price is $1.80 and the quantity demanded is 50, the total revenue can be calculated as:
TR = P x Q = 1.80 x 50 = $90
g. If demand is price elastic and the market price is $3.00, total revenue can be increased by reducing the price. This is because the percentage increase in quantity demanded will be greater than the percentage decrease in price, resulting in a net increase in total revenue.
Therefore, the company could consider lowering the price from $3.00 to a price closer to the optimal price of $2.40 to increase total revenue.
Learn more about demand function on:
https://brainly.com/question/16965973
#SPJ1
This extreme value problem has a solution with both a maximum value and a minimum value. Use Lagrange multipliers to find the extreme values of the function subject to the given constraint. f(x, y) = x2 − y2; x2 + y2 = 9
Answers
The maximum value of the function is 9, and the minimum value of the function is −9.
We have function is f(x,y)=x²−y² and constraint is x²+y²=9,
For getting the extreme values of the function subject to the given constraint using Lagrange multipliers
Firstly we will form the Lagrangian function L (x,y,λ) as follows:
L (x,y,λ)=f(x,y)+λ(g(x,y))
Where f(x,y) = x²−y²and g(x,y) = x²+y² = 9
Thus, L (x,y,λ) = x²−y² + λ(x²+y² = 9)
Now we have to find the partial derivatives of L (x,y,λ) with respect to x,y and λ and equate them to 0 for finding the critical points.
\(L_x= 2x+2 \lambda x = 0\) .......(1)
\(L_y= -2y+2 \lambda y = 0\) ........(2)
\(L_{\lambda}= x^2+y^2-9 = 0\) (3)
From the above equations (1) and (2),we get
2x+2λx = 0 ⇒ x(1+λ) = 0
Thus, x = 0 and λ = -1
Similarly, y = 0 and λ = 1
From equations (1) and (2), we have
2λx = -2x ⇒ λ = -1
Therefore, x = 0 is not acceptable
From equations (1) and (2), we have
2λy = 2y ⇒ λ = 1
Therefore, y = 0 is not acceptable
Now using equation (3),we have
x²+y²-9 = 0
⇒ x²+0-9 = 0
⇒ x = ±3
Substituting x = 3 in equation (1), we get λ = -1
Hence, the first solution is (x,y) = (3,0)
Similarly, substituting x = -3 in equation (1), we get λ = -1
Hence, the second solution is (x,y) = (-3,0)
Now we will classify the obtained critical points as maxima or minima or saddle points
For finding the nature of critical points, we will form a Hessian matrix H of second partial derivatives of the Lagrangian function L at the critical point (x,y) as follows:
\($$ H = \left[\begin{array}{ccc} L_{xx}&L_{xy}\\L_{yx}&L_{yy}\\\end{array}\right] \\\)
On evaluating the Hessian matrix H at the critical point (x,y) = (3,0), we get the Hessian matrix as
\($$ H =\left[\begin{array}{ccc}4&0\\0&-2\\\end{array}\right]\)
Here, the determinant of the Hessian matrix,
\($$ D(H) = \left[\begin{array}{ccc} L_{xx}&L_{xy}\\L_{yx}&L_{yy}\\\end{array}\right] \\\)
= (4)(-2)-(0)(0)
= -8 < 0
Hence, Hessian matrix H is negative definite at the critical point (3,0)
Therefore, the function has a local maximum at (3,0)
On evaluating the Hessian matrix H at the critical point (x,y) = (-3,0), we get Hessian matrix as
\($$ H =\left[\begin{array}{ccc}4&0\\0&-2\\\end{array}\right]\)
Here, the determinant of the Hessian matrix,
\($$ D(H) = \left[\begin{array}{ccc} L_{xx}&L_{xy}\\L_{yx}&L_{yy}\\\end{array}\right] \\\)
= (4)(-2)-(0)(0)
= -8 < 0
Hence, Hessian matrix H is negative definite at the critical point (-3,0)Therefore, the function has a local maximum at (-3,0)Hence, the extreme values of the function are as follows:
The maximum value of the function f(x,y) = x² - y², subject to the given constraint x²+ y² = 9, is 9 at (3,0) and (-3,0)
Hence, the solution is -9.
To know more about the "Lagrange multipliers": https://brainly.com/question/4609414
#SPJ11
The organizer of a late night street fair in a popular tourist city wants to analyze the relationship between daily revenue and the following variables: the number of male visitors, the number of female visitors, the number of retail stands, the number of food (and beverage) stands, and the number of performances that take place on a given night. The regression output table is provided below. Based on these results and using a 10% significance level, the organizer thinks he can improve the model. He wants to try removing at least one variable from the analysis to create and compare new models. Which variable or variables would you recommend that he consider removing from the regression model? SELECT ALL THAT APPLY.
Answers
The variables that the organizer can consider removing from the regression model are the Number of male visitors and Number of performance.
Based on the regression table provided, we can answer this question. The following is the regression table:Regression output table for night street fair variableNameCoefficientStandard Errort-StatP-ValueConstantb0.340.1402.430.023Number of male visitorsb10.6130.51320.750.462Number of female visitorsb10.9070.57918.830.000Number of retail standsb0.1160.0452.570.016Number of food (and beverage) standsb0.3160.0575.500.000Number of performanceb0.1460.1071.360.184The null hypothesis is rejected if p-value < 0.1; that is, there is a significant difference between the independent and dependent variables. The p-value of the Number of male visitors, the Number of female visitors, the Number of retail stands, the Number of food (and beverage) stands, and the Number of performances variables are 0.462, 0.000, 0.016, 0.000, and 0.184, respectively. As a result, the variables that are significant at the 10% level are the Number of female visitors, the Number of retail stands, and the Number of food (and beverage) stands. Therefore, the organizer should consider removing the Number of male visitors and Number of performance variables from the regression model. The variables that the organizer can consider removing from the regression model are the Number of male visitors and Number of performance.
Learn more about Organizer
brainly.com/question/9350045
#SPJ11
Point C is on line segment BD. Given BC=x+4,BC=x+4, CD=5x,CD=5x, and BD=5x+7,BD=5x+7, determine the numerical length of line CD.
Answers
The numerical length of line CD is 15
Solving linear equationsFrom the question, we are to determine the numerical length of line CD
From the given information,
Point C is on line segment BD
Thus, we can write that
BC + CD = BD
Also, from the given information
BC = x+4
CD = 5x
BD = 5x +7
Therefore,
x+4 + 5x = 5x + 7
6x + 4 = 5x + 7
Collect like terms
6x - 5x = 7 - 4
x = 3
Now, substitute the value of x into the equation for line CD
CD = 5x
CD = 5×3
CD = 15
Hence, the numerical length of line CD is 15
Learn more on Solving linear equations here: https://brainly.com/question/18883520
#SPJ1
suppose that $1,000 is deposited into an account that yields 0.85% interest, compounded annually? how much money will be in that account at the end of 4 years?
Answers
The money in the account at the end of the 4 years is $1034
What is compound interest?
Compound interest, also known as interest on principal and interest, is the practice of adding interest to the principal amount of a loan or deposit.
Now we are given that the initial amount deposited in the account is $1000
This account is giving 0.85% And we have to figure out how much amount will be there
We use compound interest formula to find the amount
\(A= P(1+r/n)^{nt}\\\\A= 1000(1+0.0085)^{4}\\A = 1000(1.034)\\A= 1034\)
Hence the amount will be after 4 years is $1034.
To learn more about compound interest please refer the following link
https://brainly.com/question/29003086
#SPJ4
Suppose you have entered 79-mile biathlon that consists of a run and a bicycle race. During your run, your average velocity is miles 5per hour, and during your bicycle race, your average velocity is 23 miles per hour. You finish the race in 5 hours. What is the distance of the run? What is the distance of the bicycle race?
Answers
Answer:
The distance of the run is 10 miles
The distance of the bicycle race is 69 miles
Step-by-step explanation:
Let:
r = the distance for the run79 - r = the distance for the bicycle racet = the time of the run5 - t = the time of the bicycle raceThe run: r = 5t
The bicycle race: 79 - r = 23(5 - t)
Simplify the bicycle race:
79 - r = 115 - 23t-r = 36 - 23tr = 23t - 36Now we have two equations set up for r
r = 5tr = 23t - 36We know that r = r, so 5t must equal 23t - 36
5t = 23t - 365t + 36 = 23t36 = 18tt = 2Now we know that the run took 2 hours.
Plug it back into the equation for the run to find the distance for the run
r = 5tr = 5(2)r = 10The run is 10 miles
To solve for the bicycle race, we simply subtract 10 from 79, because we know that the two distances combined are 79.
79 - 10 = 69The bicycle race is 69 miles.
-Chetan K
Answer:
run: 10 milesbike race: 69 milesStep-by-step explanation:
Let r represent the distance run. Then 79-r is the distance biked. The total time is ...
time = distance/speed
5 = (r/5) +(79-r)/23 . . . . sum of time running and time biking
575 = 23r +5(79 -r) . . . . multiply by 115
180 = 18r . . . . . . . . . . . . . subtract 395
10 = r . . . . . . . . . . . divide by 18
79-r = 69 . . . . . . distance biking
The distance of the run is 10 miles; the bicycle race is 69 miles.
How do you represent real-world problems involving ratios and rates with tables and graphs?
Answers
Answer:
we can study the relationship between the two given variables or quantities