For a standard normal distribution, find:
P(z > c) = 0.1253
Find c.
(round to 2 decimal places)
Answers
The value of c is 1.15. This is found by looking up the probability 0.1253 in the standard normal table and subtracting the value from 0.A standard normal distribution is a normal distribution with a mean of 0 and a standard deviation of 1.
The standard normal table shows the probability that a standard normal variable will be less than a certain value. To find the value of c, we can look up the probability 0.1253 in the standard normal table. The table shows that the probability is 0.1253. This means that 12.53% of the values in a standard normal distribution will be greater than c.
To find the actual value of c, we can subtract the probability from 0.0:
c = 1 - 0.1253 = 0.8747
This means that c is the value that 87.47% of the values in a standard normal distribution will be less than.
Rounded to 2 decimal places, the value of c is 1.15.
To learn more about standard normal variable click here : brainly.com/question/30911048
#SPJ11
Related Questions
Hi :) I'm working with rational and irrational numbers. There's a problem .025 with the 25 repeating. If anyone could lend some help on how to solve an equation like this. It would be greatly appreciated.

Answers
Your teacher tells the class they can have a party but you have to plan it.There are 34 students in your class and you've all decided on personal-size pizza, either cheese orpepperoni. You collect $120 from the class but when you get to the pizza place, you forget how many ofeach kind of pizza you were supposed to order! Personal-size pepperoni pizzas cost $4 and personal-size cheese pizzas cost $3Find the number of each kind of pizza you must order
Answers
Let x be the number of pepperoni pizzas you must order and y be the number of cheese pizzas.
Then, we have the following system:
I) x + y = 34
II) 4x + 3y = 120
First, we multiply equation I by 3:
I) 3x + 3y = 102
II) 4x + 3y = 120
Now, we subtract equation I from equation II:
(4x - 3x) + (3y - 3y) = 120 - 102
x = 18
Then, y = 34 - 18 = 16
You must order 18 persona-size pepperoni pizzas and 16 personal-size cheese pizzas.
An art supply store sells two colors of ink. the store has 6 cases of black ink and 8 cases of blue ink. what doe the ratio 6:14 represent in this situation? explain your reasoning.
Answers
The ratio 6:14 represents the number of cases of black ink to the number of cases of blue ink. This means that for every 6 cases of black ink, there are 14 cases of blue ink. The ratio does not give any information about the total number of cases of ink, only the relative proportions of black ink to blue ink.
The ratio 6:14 represents the number of cases of black ink to the number of cases of blue ink that are being sold at the art supply store. This means that for every 6 cases of black ink that are sold, there are 14 cases of blue ink sold. The ratio does not give any information about the total number of cases of ink that are being sold, only the relative proportions of black ink to blue ink.
For example, if the art supply store is selling a total of 20 cases of ink, the ratio of 6:14 could represent 6 cases of black ink and 14 cases of blue ink, or 12 cases of black ink and 28 cases of blue ink. In both cases, the ratio of black ink to blue ink would be the same, but the total number of cases of ink being sold would be different.
Understanding ratios like 6:14 can be useful for comparing the relative proportions of different quantities, such as the sales of different products or the proportions of different ingredients in a recipe.
Learn more about the ratio at
https://brainly.com/question/13419413?referrer=searchResults
#SPJ4
Let f(x) = 3x + 2. If f(x) = 11, find x.
Answers
Answer:
x=3
Step-by-step explanation:
If f(x)=11, then 11=3x+2.
Subtract 2 on both sides of the equation.
11-2=3x+2-2
9=3x
Divide on both sides by 3
9/3 = 3x/3
3=x
given no constraints, how many possible sequences are there if five jobs are in the queue for an operation?
Answers
There are 3,125 possible sequences if five jobs are in the queue for an operation, and there are no constraints on the order or repetition of jobs.
If there are no constraints on the order of the jobs in the queue, and each job can appear more than once in the sequence, we can calculate the number of possible sequences using the concept of permutations with repetition.
In this case, we have five jobs to consider. Let's label them as Job 1, Job 2, Job 3, Job 4, and Job 5. For each position in the sequence, there are five possible jobs that can be assigned to that position. Since there are five positions in total, we can multiply these possibilities together to get the total number of sequences.
To illustrate this, let's break down the calculation step by step:
For the first position, there are five possible jobs: Job 1, Job 2, Job 3, Job 4, and Job 5.
For the second position, there are also five possible jobs: Job 1, Job 2, Job 3, Job 4, and Job 5.
Similarly, for the third, fourth, and fifth positions, there are five possible jobs each.
Since each position has five possible choices, we can multiply these choices together:
5 * 5 * 5 * 5 * 5 = 5⁵ = 3125
Therefore, there are 3,125 possible sequences if five jobs are in the queue for an operation, and there are no constraints on the order or repetition of jobs.
Learn more about sequences here:
https://brainly.com/question/30262438
#SPJ11
7. Describe the rule for each of the tables below:
a.
Row 1
Row 2
Rule:
5
7
7
11
15
37
19
35
29
55
Answers
Answer:
Step-by-step explanation:
It is difficult to determine the rule for the table without further context or information. However, based on the pattern in the table, it appears that the rule for generating the second number in each row is to add 2 to the first number in that row. The rule for generating the third number in each row is to add 6 to the second number in that row. The fourth number is the sum of the first and the third number in that row.
if f(x) = 2x^ 2 + 3x,then what is f(−9)?
Answers
Answer:
f(-9) = 135
Step-by-step explanation:
Replace x with -9, and evaluate the expression.
f(x) = 2x² + 3x
f(-9) = 2(-9)² + 3(-9)
f(-9) = 2(81) - 27
f(-9) = 162 - 27
f(-9) = 135
If 8x-4=12;then 2x - 1=
Answers
Answer: 21
Step-by-step explanation:
Step-by-step explanation:
tbh I have no idea
doing this for points
so yeah
If three out of every 200 people contract viral disease how many people will contract the viral disease among 30,000 people solve using proportions.
Answers
Step-by-step explanation:
let no of people that contracts it be p.
3/200 = p/30000
200p = 90000
p = 450
Topic: Rate and Proportions
If you like to venture further, do check out my insta (learntionary) where I regularly post useful math tips! Thank you!
With maintaining the given proportion the number of people who will contract the viral disease among 30,000 people will be 450.
What are the ratio and proportion?The ratio is the division of the two numbers.
For example, a/b, where a will be the numerator and b will be the denominator.
Proportion is the relation of a variable with another. It could be direct or inverse.
As per the given,
Three out of every 200 people contract the viral disease.
Ratio = 3/200
With the same ratio,
x/30000 = 3/200
x = (3/200)30,000
x = (3/2)300
x = 900/2 = 450
Hence "With maintaining the given proportion the number of people who will contract the viral disease among 30,000 people will be 450".
For more information about ratios and proportions,
brainly.com/question/26974513
#SPJ2
The table lists the test scores William and Andre received on five math assessments. Which student had the higher median? By how much? A: Andre by 7 points B: William by 5 points C: William by 7 points D: Andre by 5 points

Answers
Answer: c
First you organize the numbers into order then you find the middle of both. The middle of Williams was 89. Then you do the same for Andres and you get 82. To get the difference of them both you have to subtract both of them to get 7 and that’s c.
A square has a perimeter of 40 feet. Find the length of the diagonal of the square.
Answers
Answer:
10 root 2
Step-by-step explanation:
Refer to attachment.
Hope it helps.

Solve for p.
80 + 10 = 5p - 8
Answers
Answer:
80+10=5p-8
\(\mathrm{Switch\:sides}\)
\(5p-8=80+10\)
\(\mathrm{Add\:the\:numbers:}\:80+10=90\)
\(5p-8=90\)
\(\mathrm{Add\:}8\mathrm{\:to\:both\:sides}\)
\(5p-8+8=90+8\)
\(\mathrm{Simplify}\)
\(5p=98\)
\(\mathrm{Divide\:both\:sides\:by\:}5\)
\(\frac{5p}{5}=\frac{98}{5}\)
\(\mathrm{Simplify}\)
\(p=\frac{98}{5}\)
Hope it helps. Please mark me as brainliest :) ;) ^-^
Step-by-step explanation:
\(\huge\text{Hey there!}\)
\(\large\textsf{80 + 10 = 5p - 8}\)
\(\large\textsf{90 = 5p - 8}\)
\(\large\textsf{5p - 8 = 90}\)
\(\large\text{ADD 8 to BOTH SIDES}\)
\(\large\textsf{5p - 8 + 8 = 90 + 8}\)
\(\large\text{CANCEL out: -8 + 8 because gives you 0}\\\\\large\text{KEEP: 90 + 8 because helps solve for the p-value}\)
\(\large\textsf{90 + 8 = \bf 98}\)
\(\large\text{New EQUATION: \textsf{5p = 98}}\)
\(\large\text{DIVIDE 5 to BOTH SIDES}\)
\(\large\boxed{\mathsf{\dfrac{5p}{5} = \dfrac{98}{5}}}\)
\(\large\text{Cancel out: }\rm{\dfrac{5}{5}}\large\text{ because that gives you 1}\\\\\large\text{KEEP: }\rm{\dfrac{98}{5}}\large\text{ because it gives you the value of p or the answer of p}\)
\(\large\text{SIMPLIFY ABOVE AND YOU HAVE YOUR ANSWER OF P}\)
\(\huge\boxed{\rm{Therefore, your\ answer: p = \dfrac{98}{5}}}\huge\checkmark\)
\(\huge\text{Good luck on your assignment \& enjoy your day!}\)
~\(\frak{Amphitrite1040:)}\)
The area in the trapezoid in square units 10 inches, 8 inches, 12 inches.

Answers
Answer: 100
Step-by-step explanation:
The formula for the area of a trapezoid is (B1+B2)/2*h
so substitute:
(8+12)/2*10
20/2*10
10*10
100
Answer:
100 sq inches
Step-by-step explanation:
Simply use the formula for finding the area:
\(\frac{1}{2} * (sum of parallel sides) * height\\= \frac{1}{2} * (8 + 12) * 10\\= \frac{1}{2} * 20 * 10\\= 100 sq. inches\)
A cabin cruiser traveling with the current went 45 mi in 3 h. Traveling against the current, it took 5 h to go the same distance. Find the rate of the cabin cruiser in calm water and the rate of the current.
Answers
The rate of cabin cruiser in calm water id 12 miles/h and the rate of the current is 3 miles/h.
let the speed of cabin cruiser in still water be x miles/h
let the speed of current be y miles/h
during travelling with the current, speed of cabin cruiser = (x+y) miles/h
during travelling against the current, speed of cabin cruiser =(x-y) miles/h
time taken during case 1 = \(\frac{45}{x+y}\) =3 h --->given
=> x+y = 45/3
after solving x+y=15 ------ (i)
time taken during case 2(against current) = \(\frac{45}{x-y}\) =5 --->given
=> x-y = 45/5
after solving x-y =9 -------- (ii)
adding equation (i) and (ii) we get
x+y+x-y = 15+9
=> 2*x=24
=> x= 12
so x= 12 miles/h
so y=15-x= 15- 12 = 3miles/h
so x = 12 miles/h ,y= 3miles/h
To know more about equation click the link below:
https://brainly.com/question/29514785#
#SPJ4
this cuboid is made from cm squares
a)write down the volume of the cuboid
b)the cuboid is then split apart into these smaller cuboids. how many of these can be made?

Answers
Answer:
Step-by-step explanation:
Each signal unit cubes are 1 cm to each side
the dimensions of the large cube is 4 by 6 by 3 width height and depth
a) volume of the cube Vol = area of the base times height
area of the base = the width times the depth
Volume = Base Depth
= Width x Depth x Height
= 4 x 3 x 6
= 12 x 6
Vol = 72 cm ³
b ) the volume of the smaller cuboid has different dimensions
the smaller cube is 2 by 2 by 1 width height and depth
Volume = Base Depth
= Width x Depth x Heigh
= 2 x 2 x 1
Vol smaller cuboid = 4
How many smaller cubes can be made?
The obvious answer might be to straight up divide the large cube volume by the smaler cuboid volume. That might work IF all of the cuboid dimensions divide evenly into the cube dimensions.
Number of cuboid = Volume of the cube / Volume of the cuboid
= 72 / 4
= 18
Check this answer by dividing the dimension of the cube by the cuboid
4 by 6 by 3
2 by 2 by 1
(4/2) by (6/2) by (3/1)
2 by 3 by 3 = 2 x 3 x 3 = 18
Classify the quadric surface. 4x2 - y2 - z2 = 1 a. hyperbolic paraboloid b. elliptic cone c. Hyperboloid of two sheets d. hyperboloid of one sheet e. ellipsoid
Answers
The given equation (4x² - y² - z² = 1) is classified under Hyperboloid of two sheets.
What is Hyperboloid equation?
When a hyperbola is rotated around one of its axes, an open surface is created that is known as a hyperboloid. The surface's general equation is written as (x²/ a²) + (y² / b²) - (z² / c²) = 1
if the surface's transverse axis is along the x axis, its centre is at the origin, and a, b, and c are its primary semi-axes.
As per question given that,
here is the equation of the quadratic surface is,
4x² - y² - z² = 1
Thus surface is a hyperboloid of two sheets.
The sketch of this quadratic surface is the sketch in the 2nd option which is shown below.
i.e. in the upper right corner.
To learn more about hyperboloid from the given link.
https://brainly.com/question/3351710
#SPJ4

You want to estimate the probability that 15 or more flights out of 20 will be on-time at this airport. You simulate 20 flights 25 times and get the following numbers of on-time flights: 11 15 14 8 17 14 16 15 17 17 15 9 13 16 12 18 14 16 13 15 16 14 18 149 What is your estimate of the probability?
Answers
An estimated 69.8% chance exists that 15 or more out of 20 planes will arrive on schedule.
The 20 flights were simulated 25 times in this scenario, and the outcomes were 11, 15, 14, 8, 17, 14, 16, 15, 17, 15, 9, 13, 16, 12, 18, 14, 16, 13, 15, 16, 14, 18, and 9. These figures add up to a total of 349 on-time departures out of 500 simulated departures.
It might be difficult to determine the likelihood that 15 or more aircraft out of 20 will depart on time. Thankfully, several approaches may be utilized to gauge the chance. One such approach is to simulate the issue under consideration several times, analyze the results, and then determine an estimated likelihood.
Therefore, an estimated 69.8% chance exists that 15 or more out of 20 planes will arrive on schedule.
Learn more about probability here:
https://brainly.com/question/13604758
#SPJ4
how 4 exceed negative 16
Answers
The solution is, Our required inequality would be (8x - 4) > 16.
What is inequality?An inequality is a relation which makes a non-equal comparison between two numbers or mathematical expressions.
here, we have,
Let the number be 'x'.
Eight times a number is expressed as
8x
Sum of eight times a number and negative four is expressed as
8x - 4
the above exceeds 16 is expressed as
8x - 4 > 16
Hence, our required inequality would be (8x - 4) > 16
To learn more on inequality click:
brainly.com/question/24853349
#SPJ1
What value of y makes this true? 12+y>17
Answers
Answer:
5 if the value of y become 5 it makes this true
Hi! Let's solve this inequality!
Move 12 to the right side, using the opposite operation.
y>17-12
y>5
Thus, any value of y greater than 5 will make this inequality true.
Let's try 6, since 6 is greater than 5.
12+6>17
18>17
Yeah, it worked :)
Thus, any value of y greater than 5 will make this inequality true.
Hope it helps!
Enjoy your day!
Please feel free to ask if you have any doubts.
\(\bf{M^a^g^i^c^a^l\)
answer this geometry question and show work!!

Answers
Answer:
x = 58°
Step-by-step explanation:
Label the point where the diagonals cross as T
Diagonals always meet at right angles
m∠SRT = 32
Sum of interior angles of ΔSRT = 180
x = 180 - 90 - 32 = 58
for what values of x is the inequality -5x - 3 + 2x > -18 true
Answers
The solution to the inequality is all values of x less than 5. We can write the solution set using interval notation as (-∞, 5).
How to solve this inequality ?To solve the inequality -5x - 3 + 2x > -18, we need to isolate the variable x on one side of the inequality sign.
Starting with the given inequality:
-5x - 3 + 2x > -18
Simplifying the left-hand side by combining the like terms:
-3x - 3 > -18
Adding 3 to both sides:
-3x > -15
Dividing both sides by -3, and reversing the inequality sign since we are dividing by a negative number:
x < 5
Therefore, the solution to the inequality is all values of x less than 5. We can write the solution set using interval notation as (-∞, 5).
Learn more about inequality here : brainly.com/question/18881247
#SPJ1
find the value of 6.4⋅(8+15)
Answers
Answer:
The Answer is 147.2
Step-by-step explanation:
Hope this helps Have a great day and Sry if i'm wrong :)
You are testing against based on an srs of 15 observations from a normal population. what values of the t statistic are statistically significant at the level
Answers
According to the concept of hypothesis, the value of t statistics is 0.5000 > a = 0.05
The term hypothesis is written as an assumption, an idea that is proposed for the sake of argument so that it can be tested to see if it might be true.
Here we have given that You are testing with the null hypothesis H0 => μ = 10 against the alternative hypothesis H1 => μ = 10 based on an SRS of 15 observations from a Normal population.
Then the t statistic are statistically significant at the α=0.005 level.
While we looking into the given values the Sample size n = 15
Then the Degree of freedom is calculated as
=> df = n-1 = 14
The value of t test is calculated as,
=> t-value = P(z ≤ 0.00) = .5000
Here the Rejection Rule is written as Reject H0 if t-value <a
Then the t-value 0.5000 > a = 0.05
To know more about Hypothesis here
https://brainly.com/question/29576929
#SPJ4
express this ratio in lowest fraction form
16 to 2/3
Answers
The ratio 16 to 2/3 can be expressed in its lowest fraction form as 48 to 1.
To express the ratio 16 to 2/3 in its lowest fraction form, we need to find the greatest common divisor (GCD) of the numerator and the denominator. The numerator of the ratio is 16, and the denominator is 2/3, which can be rewritten as 2 divided by 3. To find the GCD, we calculate the GCD of 16 and 2, which is 2. We then divide both the numerator and denominator by the GCD to simplify the fraction.
Dividing 16 by 2 gives us 8, and dividing 2 by 2 gives us 1. Therefore, the simplified ratio is 48 to 1. This means that for every 48 units in the first quantity, there is 1 unit in the second quantity. Simplifying the ratio to its lowest fraction form provides a clear representation of the relationship between the two quantities and allows for easier comparison and interpretation.
Learn more about ratio here:
https://brainly.com/question/30293147
#SPJ11
Suppose A is the matrix for T: R3 → R3 relative to the standard basis.
Find the diagonal matrix A' for T relative to the basis B'. A = −1 −2 0 −1 0 0 0 0 1 , B' = {(−1, 1, 0), (2, 1, 0), (0, 0, 1)}
Answers
The diagonal matrix A' for T relative to the basis \(\(B'\)\) is:
\(\[A' = \left[ \begin{array}{ccc} 3 & -4 & 0 \\ -2 & -1 & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
How to find the diagonal matrixTo find the diagonal matrix A' for the linear transformation T relative to the basis B', we need to perform a change of basis using the given matrix A and basis B'.
Let's denote the standard basis as \(\(B = \{(1, 0, 0), (0, 1, 0), (0, 0, 1)\}\).\)
To perform the change of basis, we need to find the matrix P such that P[B'] = B.
We can write the vectors in B' as column vectors:
\(\[B' = \left[ \begin{array}{ccc} -1 & 2 & 0 \\ 1 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
To find \(P\), we solve the equation P[B'] = B for P:
\(\[P \cdot B' = B\]\\\\\P = B \cdot (B')^{-1}\]\)
Calculating the inverse of \(\(B'\)\):
\(\[B'^{-1} = \left[ \begin{array}{ccc} -1 & 2 & 0 \\ 1 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right]^{-1} = \left[ \begin{array}{ccc} -1 & 1 & 0 \\ \frac{1}{2} & \frac{1}{2} & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
Now we can calculate \(\(P\)\):
\(\[P = B \cdot B'^{-1} = \left[ \begin{array}{ccc} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right] \cdot \left[ \begin{array}{ccc} -1 & 1 & 0 \\ \frac{1}{2} & \frac{1}{2} & 0 \\ 0 & 0 & 1 \end{array} \right] = \left[ \begin{array}{ccc} -1 & 1 & 0 \\ \frac{1}{2} & \frac{1}{2} & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
Now, the diagonal matrix A' for T relative to the basis B' can be calculated as:
\(\[A' = P^{-1} \cdot A \cdot P\]\)
Calculating\(\(P^{-1}\):\)
\(\[P^{-1} = \left[ \begin{array}{ccc} -1 & 1 & 0 \\ \frac{1}{2} & \frac{1}{2} & 0 \\ 0 & 0 & 1 \end{array} \right]^{-1} = \left[ \begin{array}{ccc} -1 & 2 & 0 \\ 1 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
Substituting the values into the equation for \(\(A'\)\):
\(\[A' = \left[ \begin{array}{ccc} -1 & 2 & 0 \\ 1 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right]^{-1} \cdot \left[ \begin{array}{ccc} -1 & -2 & 0 \\ -1 & 0 & 0 \\ 0 & 0 & 1 \end{array} \right] \cdot \left[ \begin{array}{ccc} -1 & 2 & 0 \\ 1 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
Performing the matrix multiplication:
\(\[A' = \left[ \begin{array}{ccc} -1 & 2 & 0 \\ 1 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right] \cdot \left[ \begin{array}{ccc} -1 & -2 & 0 \\ -1 & 0 & 0 \\ 0 & 0 & 1 \end{array} \right] \cdot \left[ \begin{array}{ccc} -1 & 2 & 0 \\ 1 & 1 & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
Calculating the matrix multiplication, we get:
\(\[A' = \left[ \begin{array}{ccc} 3 & -4 & 0 \\ -2 & -1 & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
Therefore, the diagonal matrix A' for T relative to the basis B' is:
\(\[A' = \left[ \begin{array}{ccc} 3 & -4 & 0 \\ -2 & -1 & 0 \\ 0 & 0 & 1 \end{array} \right]\]\)
Learn more about matrix at https://brainly.com/question/1279486
#SPJ4
A rectangular box weight 2.8 pounds. Width 7.70 inches,depth 7.75 inches and height 1.41 inches what is the length
Answers
The length of the rectangular box is given as follows:
1.59 inches.
How to obtain the volume of the rectangular prism?The volume of a rectangular prism, with dimensions length, width and height, is given by the multiplication of these dimensions, as follows:
Volume = length x width x height.
A rectangular box weight 2.8 pounds, and each pound has 27.68 cubic inches, hence the volume in in³ is given as follows:
V = 2.8 x 27.68
V = 77.504 in³.
The width is of 7.70 inches and the height is of 7.75 - 1.41 = 6.34 inches, hence the length is obtained as follows:
7.70 x 6.34l = 77.504
l = 77.504/[7.70 x 6.34]
l = 1.59 inches.
More can be learned about the volume of a rectangular prism at brainly.com/question/22070273
#SPJ1
Select the fractions that add to 13/3
A. 6/5 + 4/3
B. 6/2 + 4/3
C. 5/3 + 6/5
Answers
B. 6/2 +4/3= 13/3
C. 5/3 + 6/5= 43/15
Therefore B
2. The diagram shows a circle inside a rectangle. Work out the shaded region,
Give your answer to 3 significant figures.
8 cm
17 cm
32 cm

Answers
Answer:
342.938 cm²
Step-by-step explanation:
Area of shaded region = area of rectangle - area of circle
= (length*width) - (π*radius²)
= (32*17) - (π8²)
= 544 - 201.06192983
= 342.93807017 ≈ 342.938 cm²
The area of the parallelogram below is ____ square meters.
A parallelogram with height labeled with 7 meters. The top horizontal side is labeled 9 meters. The base of the left triangle formed by the height is 2 meters.
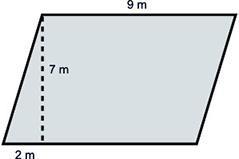
Answers
Answer:
63 m²
Step-by-step explanation:
The area of a parallelogram is given by the formula ...
A = bh . . . . . . the product of base length and height
Filling in the given values, ...
A = (9 m)(7 m) = 63 m²
The area of the parallelogram is 63 square meters.
__
Additional comment
The area of any figure with a uniform cross section is the product of the length of that cross section and the "height" measured perpendicular to it. This same formula applies to rectangles, parallelograms, or any other figure with parallel sides, regardless of the shape of those sides.
Mr. Dieter wants to tile the family room in his basement. He has selected a pattern of
square tiles that measure 9 inches by 9 inches each. The shape of the floor to be tiled is
shown below. (3 points for each part)
a. What is the area of the family room in square feet?
b. How many of the 9 inch by 9 inch tiles will it take to cover the floor? (NOTE: You
will need to convert the area in Part a from square feet to square inches.)
c. If the tile is sold only in boxes of 12 tiles per box, how many boxes will Mr. Dieter
have to buy to tile the family room?

Answers
a. The area of the family room is 162 ft².
b. 24 tiles will be needed to cover the floor.
c. Mr. Dieter will have to buy 2 boxes to tile the family room.
The solution has been obtained by using the arithmetic operations.
What are arithmetic operations?
All real numbers are thought to be sufficiently described by the four basic operations, also referred to as "arithmetic operations." The mathematical operations quotient, product, sum, and difference come after division, multiplication, addition, and subtraction.
a. There are two different shapes of which one is rectangle and other is triangle.
So,
⇒ Area of rectangle = length x width
⇒ Area of rectangle = 16 x 8
⇒ Area of rectangle = 128 ft²
Also,
⇒ Area of first triangle = \(\frac{1}{2}\) x base x height
⇒ Area of first triangle = \(\frac{1}{2}\) x 4 x 3
⇒ Area of first triangle = 6 ft²
Similarly,
⇒ Area of first triangle = \(\frac{1}{2}\) x base x height
⇒ Area of first triangle = \(\frac{1}{2}\) x 8 x 7
⇒ Area of first triangle = 28 ft²
On adding the three areas, we get
⇒ Area of family room = 128 + 6 + 28
⇒ Area of family room = 162 ft²
b. We will convert the area into inches.
We know that 1 feet = 12 inches.
So, using the multiplication, we get
162 feet = 162 * 12 in²
162 feet = 1944 in²
Now,
⇒ Area of tile = 9 * 9
⇒ Area of tile = 81 in²
So,
⇒ Number of tiles = \(\frac{1944}{81}\)
⇒ Number of tiles = 24
c. Since, tiles are sold in boxes of 12 so, using the division operation, we get
⇒ Number of boxes needed = \(\frac{24}{12}\)
⇒ Number of boxes needed = 2
Hence, the required solution has been obtained.
Learn more about arithmetic operations from the given link
brainly.com/question/30283549
#SPJ1