Answers
Related Questions
(5pt)
Find the exact value of
sin( 6
11π
)
. Explain your answer using reference angle.
Answers
The exact value of sin(6/11π) is -sin(6/11π).
The exact value of sin(6/11π) can be found by using the reference angle of 6/11π. The reference angle is the acute angle formed by the terminal side of the given angle and the x-axis. In this case, the reference angle is (6/11π) - π = (-5/11π).
To find the exact value of sin(-5/11π), we can use the fact that sin(-θ) = -sin(θ). So, sin(-5/11π) = -sin(5/11π).
Next, we can use the fact that sin(θ) = sin(π - θ) to find the exact value of sin(5/11π). So, sin(5/11π) = sin(π - 5/11π) = sin(6/11π).
Therefore, the exact value of sin(6/11π) is -sin(6/11π).
In conclusion, the exact value of sin(6/11π) is -sin(6/11π).
Learn more about Exact value
brainly.com/question/12060967
#SPJ11
Make a table of values and a graph for the functionWhat is the domain?What is the range?

Answers
Answer and Explanation:
Given:
\(f(x)=(0.2)^x-3\)Let's go ahead and chose different values of x and corresponding values of y;
When x = -2;
\(\begin{gathered} f(-2)=(0.2)^{-2}-3 \\ =25-3 \\ =22 \end{gathered}\)When x = -1;
\(\begin{gathered} f(-2)=(0.2)^{-1}-3 \\ =5-3 \\ =2 \end{gathered}\)When x = 0;
\(\begin{gathered} f(0)=(0.2)^0-3 \\ =1-3 \\ =-2 \end{gathered}\)When x = 1;
\(\begin{gathered} f(1)=(0.2)^1-3 \\ =0.2-3 \\ =-2.8 \end{gathered}\)When x = 5;
\(\begin{gathered} f(5)=(0.2)^5-3 \\ =0.00032-3 \\ =-2.99 \end{gathered}\)With the above values, we can go ahead and graph the function as seen below;
The domain of a function is the set of possible input values(x-values) for which the function is defined. On a graph, it is the set of x-values from left to right.
Looking at the given graph, we can see that the domain is;
\(Domain:(-\infty,\infty)\)The range of a function is the set of possible output values(y-values). It is the set of y-values from the bottom to the top of the graph.
Looking at the given graph, we can see that the range is;
\(Range:(-3,\infty)\)
The mass of the part of a metal rod that lies between its left end and a point x meters to the right is 3x^2 kg. Find the linear density when is 1 m, Where is the density the highest? The lowest?
Answers
Answer:
6 kg/m
Step-by-step explanation:
mass m = 3x^2.
rho ρ= dm/dx =6x
ρ(1) =6 Kg/m
linear density is the derivative of mass (m) with respect to position(x)
that is how fast is the mass changing at that position.
Given the vector v =−3/√3,1; find the direction angle of this
vector.
a) 5π6
b) 2π3
c) −π3
d) π6
e) 0
f) None of the above.
Answers
Hence, the direction angle of the vector is (c) −π/3.
Given the vector v = −3/√3, 1; we are required to find the direction angle of this vector.
The direction angle of a vector is defined as the angle made by the vector with the positive direction of the x-axis, measured counterclockwise.
Let θ be the direction angle of the vector.
Then tanθ = (y-component)/(x-component) = 1/(-3/√3)
= −√3/3
Thus, we getθ = tan−1(−√3/3)
= −π/3
Therefore, the correct option is c) −π/3.
If the angle between the vector and the x-axis is measured clockwise, then the direction angle is given byθ = π − tan−1(y-component/x-component)
To know more about vector visit:
https://brainly.com/question/24256726
#SPJ11
which quadratic equation has 2 and -3 as its roots ?
Answers
Answer:
alfa = 2
beeta = -3
product of zeros = 2x-3= -6
sum of zeros = 2+[-3] = -1
POLYNIMIAL = k[x²-[sum]+product]
=k[ x²-[-1] +[-6]]
= k[ x²+1 -6]
= x²-5
hence , the quadratic equation is x²-5
HERES UR ANS .
HOPE IT DOES HELP U
IF SO PLZ MARK THE BRAINLIESTT !!!!\
(05.05 MC) Which of the following inequalities matches the graph?
Answers
Answer: A
Step-by-step explanation:
Its A
U spend 5/12 of a day at an amusement park you spend 2/5 of the time riding water slides how many hours do u spend riding water slides
Answers
Answer:
sounds fun
Step-by-step explanation:
Third Derivative ƒ"(x) = f(x₁₁3) — 3f (x₁+2) + 3f (x₁+1) − f (x)
Answers
The third derivative of a function ƒ(x) can be expressed as ƒ"(x) = ƒ(x+3) - 3ƒ(x+2) + 3ƒ(x+1) - ƒ(x), where x₁ represents a shifted index.
This formula allows us to compute the third derivative of a function at any given point by evaluating the function at four different shifted indices. The coefficients in the formula (-1, 3, -3, 1) represent the binomial coefficients of the expansion of (x+1)³, which correspond to the coefficients of the function values in the expression for the third derivative.
The formula for the third derivative of a function ƒ(x) can be written as ƒ"(x) = ƒ(x+3) - 3ƒ(x+2) + 3ƒ(x+1) - ƒ(x). This means that to compute the third derivative of ƒ(x) at any given point, we evaluate the function at four different shifted indices: x+3, x+2, x+1, and x.
The coefficients in the formula (-1, 3, -3, 1) correspond to the binomial coefficients of the expansion of (x+1)³. These coefficients determine the weights given to the function values in the expression for the third derivative. Each coefficient is multiplied by the corresponding function value and then subtracted or added accordingly.
By using this formula, we can find the value of the third derivative of a function at any specific point by evaluating the function at the shifted indices and applying the corresponding coefficients. This provides a method for computing higher-order derivatives of functions based on function values at nearby points.
To learn more about derivative click here:
brainly.com/question/25324584
#SPJ11
Solve for n. -4(n+5)=8
Answers
Answer:
Simplify first:
-4n - 20 = 8
Add 20 to both sides:
-4n = 28
Divide by -4:
Therefore you will get:
n = -7
Answer:
n = -7.
Step-by-step explanation:
-4(n + 5) = 8
-4n - 20 = 8
-4n = 28
n = -7.
What is the value of w?

Answers
Answer:
w = 60
Step-by-step explanation:
the exterior angle of a triangle is equal to the sum of the 2 opposite interior angles.
w + 20 is an exterior angle of the triangle , then
w + 20 = w - 10 + w - 30
w + 20 = 2w - 40 ( subtract w from both sides )
20 = w - 40 ( add 40 to both sides )
60 = w
Answer:
w=60°
Step-by-step explanation:
triangle=180°
angle on a straight line=180°
using that,
we need to find the angle on the same line with that of "w+20°" angle, so we name it as "X"
so, 180-(w+20)=X
expand bracket by multiplying it with the "-"
so it'll be: 180-w-20=X
then rearrange to get like terms together
180-20-w=x
160-w=x
so the missing angle in the triangle is now (160-w)°
now using the angles in a triangle =180°
we add all the angles and equate it to 180, so:
w-30+w-10+160-w=180°
collect like terms again:
w+w-w-30-10+160=180
2w-w+120=180
w+120=180
move 120 to the other side and remember the sign changes, so:
w=180-120
therefore w=60°
now you can substitute w=60 into the triangle and see you'll get 180°
hope that helps
a track star runs two races on a certain day. the probability that she wins the first race is 0.7, the probability that she wins the second race is 0.6, and the probability that she wins both races is 0.5. what is the probability that: note: define your events, and write the probability statement before you calculate an answer. you can show your work using equations or venn diagrams. a. she wins at least once race b. she wins exactly one race c. she wins neither race
Answers
The probability that the track star wins the first race is 0.7 and the probability that she wins the second race is 0.6.
a. she wins at least one race: P(A or B) = P(A) + P(B) - P(A and B) = P(A) + P(B) - P(A) * P(B) = 0.7 + 0.6 - 0.5 = 0.8
b. she wins exactly one race: P(A and not B) + P(B and not A) = P(A) * P(not B) + P(B) * P(not A) = 0.7 * 0.4 + 0.6 * 0.3 = 0.28
c. she wins neither race: P(not A and not B) = P(not A) * P(not B) = (1 - P(A)) * (1 - P(B)) = 0.3 * 0.4 = 0.12
You could also represent this using a venn diagram, showing the overlap between the two events.
The probability that the track star wins the first race is 0.7 and the probability that she wins the second race is 0.6. The probability that she wins both races is 0.5. To find the probability that:
a. she wins at least one race = 0.8
b. she wins exactly one race = 0.28
c. she wins neither race = 0.12
These probabilities were calculated by using the probability of the events of winning each race and the probability of both events combined.
Learn more about probability here
https://brainly.com/question/11234923
#SPJ4
What is the answer to this problem -13c+8-18c+5 ?
Answers
Answer:
-31 c + 13
Step-by-step explanation:
-13c+8-18c+5
combine like terms
-18c and -13c combined is -31c
8 + 5 = 13
-31 c + 13
Answer
-31c+13 is your answer (see explanation below!)
Step-by-step explanation:
1) Add the numbers:
\(-13c + 8 - 18c + 5\\-13c + 13 -18c\\\)
2) Combine like terms:
\(-13c+13-18c\\-31c+13\\\)
\(A: -31c+13\)
Hope this helped you! Please mark me brainliest! Thanks! Have a great day! :)
Can you help me with this

Answers
Answer:
3 and 4
Step-by-step explanation:
find the slope of all of the things
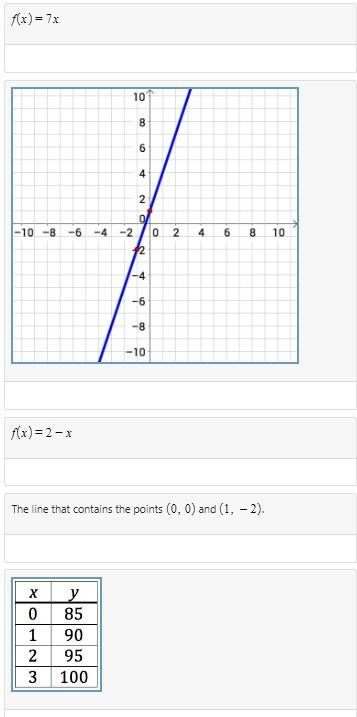
Answers
The slope of the function, f(x) = 7x, is 7
The slope of the line shown in the graph is 3
The slope of the function, f(x) = 2 - x, is -1
The slope of the line that contains points (0, 0) and (1, -2) is -2
The slope of the line given in the table is 5
Determining the slope of a lineFrom the question, we are to determine the slopes
For the function
f(x) = 7x
We will compare the equation to the slope-intercept form of the equation of a line
y = mx + b OR f(x) = mx + b
Where m is the slope
and b is the y-intercept
By comparison,
m = 7
Thus, the slope is 7
For the slope of the graph
The points on the line are (-1, -2) and (0, 1)
Using the formula,
Slope = (y₂ - y₁)/ (x₂ - x₁)
Slope = (1 - (-2)) / (0 - (-1))
Slope = (1 + 2) / (0 + 1)
Slope = 3/1
Slope = 3
The slope is 3
For f(x) = 2 - x
Compare to the slope-intercept of a line, f(x) = mx + b
m = -1
Thus, the slope of the function is -1
Slope of the line that contains points (0, 0) and (1, -2)
Using the formula,
Slope = (y₂ - y₁)/ (x₂ - x₁)
Slope = (-2 - 0) / (1 - 0)
Slope = -2/1
Slope = -2
The slope is -2
The slope of the function given in the table
Picking the points (0, 85) and (1, 90)
Using the formula,
Slope = (y₂ - y₁)/ (x₂ - x₁)
Slope = (90 - 85) / (1 - 0)
Slope = 5/1
Slope = 5
Hence, the slope is 5
Learn more on Determining the slope of a line here: https://brainly.com/question/3493733
#SPJ1
solve 6x - 3 = 3x + 12
Answers
Answer:
x=5
Step-by-step explanation:
May I have brainiest I'm trying to level up!
</3 PureBeauty
Answer:
x=5
Step-by-step explanation:
6x-3 = 3x +12
+3 +3
6x = 3x +15
-3x -3x
3x = 15
divided both sides by 3 to get your answer
(c) Problem 16: lesson 109) Find the rate of change for this two-variable equation. y = 2x + 2
Answers
Answer:2
Step-by-step explanation:
Given circle O , m∠EDF=31° . Find x .

Answers
The calculated value of x in the circle is 59
How to calculate the value of xFrom the question, we have the following parameters that can be used in our computation:
The circle
The measure of angle at the center of the circle is calculated as
Center = 2 * 31
So, we have
Center = 62
The sum of angles in a triangle is 180
So, we have
x + x + 62 = 180
This gives
2x = 118
Divide by 2
x = 59
Hence, the value of x is 59
Read more about circles at
https://brainly.com/question/32192505
#SPJ1
Math algerbra I believe I have to simplify the equation but I have no idea we’re to start

Answers
Given the equation:
\(2=(1.07)^x\)To solve this equation for x, we need to take the binary logarithm on both sides of the equation:
\(\log _22=\log \lbrack(1.07)^x\rbrack\)There are two important properties of logarithms (binary or any other kind):
\(undefined\)PLZ HELP ill mark brainlist

Answers
Answer:
x is 60 and y is 50
hope it helped have a good day mate

pitso went to buy ingredients for 48 minutes and came back at 16h15 .his friend claimed that he left half past 3p.m . verify hisd. claim
Answers
Answer:
His claim is false.
Step-by-step explanation:
People put nasty fake answers, so I will answer your question here. 16h15 minus 45 minutes is 15h30. If he was gone 48 minutes, he left at 15h27m
if mai runs 21 labs at the same rate ( 4 minutes ), how long does it take her ?
Answers
21 divided by 4
V(t)=875-50t
Find the value of V(4)?
A) 875
B) 675
825
D 200
PARTB
What is the value oft when V(t)=525
Answers
Write the equation 5x − 2y = 10 in the form y = mx + b. y equals start fraction five over two end fraction x minus 10 y equals start fraction five over two end fraction x minus five y equals start fraction five over two end fraction x plus five y equals negative start fraction five over two end fraction x minus five
Answers
Answer:
y = 5/2x - 5
Step-by-step explanation:
You have to rearrange the equation so that it is equal to y.
5x - 2y = 10
(5x - 2y) - 5x = -5x + 10
-2y = -5x + 10
(-2y)/-2 = (-5x)/-2 + (10)/-2
y = 5/2x - 5
Question 5
2 pts
(9x + 16)°
(5x - 14)
The value of
is
The measure of the exterior angle is
degrees

Answers
Answer:
Value is 15 and measure is 151
A rectangle has the following vertices: A(-1, 9), B(0, 9), C(0, -8), D(-1, -8). What is the area of rectangle ABCD?
Answers
The area of the rectangle is 17 square units.
How to find the area of the rectangle?The area of a rectangle is the product between the two dimensions (length and width) of the rectangle.
Here we know that the vertices are:
A(-1, 9), B(0, 9), C(0, -8), D(-1, -8)
We can define the length as the side AB, which has a lenght:
L = (-1, 9) - (0, 9) = (-1 - 0, 9 - 9) = (-1, 0) ----> 1 unit.
And the width as BC, which has a length:
L = (0, 9) - (0, -8) = (0 - 0, 9 + 8) = (0, 17) ---> 17 units.
Then the area is:
A = (1 unit)*(17 units) = 17 square units.
Learn more about area at
https://brainly.com/question/24487155
#SPJ1
What is the value of the expression 3m(2m) when m = 4 ?
Answers
(12)(8)
96
Derek will deposit $6,460.00 per year for 21.00 years into an
account that earns 14.00%, The first deposit is made next year. How
much will be in the account 40.00 years from today? Answer format:
Cur
Answers
The total amount that will be in the account 40.00 years from today, considering the annual deposits of $6,460.00 for 21.00 years and an annual interest rate of 14.00%, will be approximately $6,120,433.84.
Derek plans to deposit $6,460.00 per year for 21.00 years into an account with an annual interest rate of 14.00%. The first deposit will be made next year.
To calculate the total amount in the account 40.00 years from today, we need to consider the annual deposits, the interest earned, and the compounding effect over the years.
The annual deposit is $6,460.00, and the duration of deposits is 21.00 years.
Therefore, the total amount of deposits made over the 21.00 years will be 21.00 × $6,460.00 = $135,660.00.
To calculate the future value of the deposits and the interest earned, we can use the compound interest formula:
Future Value = Principal × \((1 + interest\, rate)^{number\, of\, periods}\)
In this case, the principal is $135,660.00, the interest rate is 14.00%, and the number of periods is 40.00 years.
Future Value = $135,660.00 × \((1 + 0.14)^{40}\)
Future Value = $135,660.00 × \((1.14)^{40}\)
Future Value = $135,660.00 × 45.094
Future Value = $6,120,433.84
Therefore, the total amount that will be in the account 40.00 years from today, considering the annual deposits of $6,460.00 for 21.00 years and an annual interest rate of 14.00%, will be approximately $6,120,433.84.
Learn more about compound interest formula here:
https://brainly.com/question/29008279
#SPJ11
a. Graph y = -3(x-4) using a horizontal translation.
Plot at least 5 points.
b. Translate the graph in part (a) up 5 units.
c. What is the y-coordinate of the point on the
translated line when the x-coordinate is 4?
d. The equation of the line can be written as y=-3(x-4) +5
Show that if x = 4 then y = 5.
Answers
The value of y-coordinate of the point on the translated line with 4 as the x-coordinate is 0
What is horizontal translation?
To horizontally translate a graph is simply to shift the base graph left or right in the direction of the x-axis.
The graph:
y = -3(x-4)
To determine the y - coordinate of the point on the translated line when the x-coordinate is 4
We do this by substituting the value of 'x'
Since the graph was horizontally translated up 5 points
y = -3 ( x - 4)
y = -3 ( 4 - 4)
expand the bracket
y = 0
The equation of the line is given as;
y= - 3 ( x- 4 ) + 5
We are to show that if x = 4, then y = 5
Let's substitute the value of 'x' as 4
y = - 3 ( 4 - 4) + 5
Expand the bracket
y = -3 ( 0) + 5
y = 0 + 5
y = 5
Thus, the value of y-coordinate of the point on the translated line with 4 as the x-coordinate is 0
Learn more about horizontal translation here:
https://brainly.com/question/11228569
#SPJ1
Can someone please help me to get this proof geometry

Answers
Answer:
1234
Step-by-step explanation:
Find the measure of the missing angle.
34°

Answers
The answer is 56.
As you can see, the triangle given is a right angle, meaning it is 90 degrees. To find the missing angle, we can solve 90 - 34 to get 56.
hope this helps !! have a nice day :))
