Green Frog Convenience Store supplies two types of protein bars. One protein bar has 320 calories in the 2-ounce size. How many calories are in the 3-ounce size of the bar? *
Answers
The number of calories in the 3 ounce bar is 480.
To solve this problem, we can use a proportion. If the 2-ounce protein bar has 320 calories, then we can set up the following proportion:
2 oz / 320 cal = 3 oz / x cal
where x is the number of calories in the 3-ounce protein bar.
We can solve for x by cross-multiplying and simplifying:
2 oz * x cal = 3 oz * 320 cal
2x = 960x = 480
Therefore, the 3-ounce protein bar has 480 calories.
To learn more about calories, click here:
https://brainly.com/question/22374134
#SPJ11
Related Questions
The points H, I, J and K all lie on the same line segment, in that order, such that the
ratio of HI:IJ: JK is equal to 3: 3:4. If HK - 40, find IJ.
Answers
Answer:
IJ = 12
Step-by-step explanation:
sum the parts of the ratios, 3 + 3 + 4 = 10 parts
Divide HK by 10 to find the value of one part of the ratio.
40 ÷ 10 = 4 ← value of 1 part of ratio , then
IJ = 3 parts = 3 × 4 = 12
Which ONE of the following statements is CORRECT with regards to the sequences of real numbers?
O A. If the sequence {a_n} diverges, then lim a_n n_> [infinity] = [infinity]
O B. If {a_s} is a monotone sequence with lim n->[infinity] a_n = x. then x is either an infimum or a supremum of sequence (a_s)
O C. If the sequence is monotone and bounded above, then it converges.
O D.If r<1, then lim n->[infinity] r^n = 0.
O E. None of the choices in this list.
Answers
The correct statement among the given options is:O D. If r < 1, then lim(n → ∞) r^n = 0. This statement is known as the geometric series convergence test.
It states that if the common ratio (r) of a geometric sequence is between -1 and 1 (exclusive), then the sequence converges to 0 as n approaches infinity.
The other options are not generally true:
A. The divergence of a sequence does not necessarily imply that its limit as n approaches infinity is infinity. The limit could be any real number or even undefined.
B. The limit of a monotone sequence does not have to be the infimum or supremum of the sequence. It could be any value within or outside the range of the sequence.
C. Monotonicity and boundedness alone do not guarantee convergence. Counterexamples include oscillating sequences or sequences with limit points that are not the limit of the sequence.
Therefore, option D is the correct statement.
To learn more about convergence click here:'
brainly.com/question/30974485
#SPJ11
I need the awnser for number 1

Answers
Answer:
Angel Number 1: 90
Step-by-step explanation:
As you can see the angel it is located in is a right Angel or 90 degrees angel.
Answer:
∠1
Step-by-step explanation:
Since ∠1 shares a line and vertex with ∠3, it is the angle adjacent to ∠3.
GIVING BRAINLIEST!!!PLS HELP :((
The first rule is subtract 5 starting from 30. The second rule is add 8 starting from 0. What is the fourth ordered pair using the terms in each sequence?
(16, 20)
(8, 25)
(15, 24)
(32, 10)
Answers
Answer:
D (32, 10)
Step-by-step explanation:
30 - 5 - 5 - 5 - 5 = 10
0 + 8 + 8 + 8 + 8 = 32
help pls
3n-18-2n+10+n
Answers
Answer:
Assuming that you're being asked to simplify the expression. If you are, then the answer is 2n - 8
Step-by-step explanation:
This is because the n terms:
3n -2n +n = 2n
And the integer terms:
-18+10 = -8
Together this is 2n-8
¿Cuál es la probabilidad de que una mujer que ha sido vacunada en este lapso de cuatro
meses haya recibido su segunda dosis en octubre ?
Answers
Answer: buddy if you put it in English i can do it I’m a teacher
Step-by-step explanation:
What is the value of the expression (30.72 × .05) - 1.42
Answers
Answer:
0.116
Step-by-step explanation:
\((30.72*0.05)-1.42\\1.536-1.42=\\0.116\)
Your answer is 0.116
Step-by-step explanation:
solve this please
please

Answers
Answer:
Step-by-step explanation:
cos(2a) = cos^2(a) - sin^2(a)
cos(2a) = 2cos^(a) - 1
cos(2a) = 11/25
11/25 = 2 cos^(a) - 1 Add 1 to both sides
1 + 11/25 = 2 cos^2
25/25 + 11/25 = 2 cos^2(a)
36/25 = 2 cos^2 (a) Divide by 2
36/50 = cos^2 (a) Take the square root of both sides.
6/5*sqrt(2) = cos(a)
One is given the following:
\(cos(2A)=\frac{11}{25}\)One is asked to prove the following:
\(cos(A)=\frac{6}{5\sqrt{2}}\)In order to prove the statement above, one will need to use a trigonometric identity. In this case, the following identity is the most relevant in the proof.
\(cos(2a)=2cos^2(A)-1\)
One can manipulate this identity to suit the needs of the given problem:
\(cos(2a)=2cos^2(A)-1\)
\(cos(2a)+1=2cos^2(A)\)
\(\frac{cos(2a)+1}{2}=cos^2(A)\)
\(\sqrt{\frac{cos(2a)+1}{2}}=cos(A)\)
Now substitute the given information into this identity,
\(cos(2A)=\frac{11}{25}\)
\(\sqrt{\frac{cos(2a)+1}{2}}=cos(A)\)
Substitute,
\(\sqrt{\frac{\frac{11}{25}+1}{2}}=cos(A)\)
Simplify, remember, any number over itself equals (1) and, in order to add two fractions, they must have the same denominator.
\(\sqrt{\frac{\frac{11}{25}+1}{2}}=cos(A)\)
\(\sqrt{\frac{\frac{11}{25}+\frac{25}{25}}{2}}=cos(A)\)
\(\sqrt{\frac{\frac{36}{25}}{2}}=cos(A)\)
\(\sqrt{\frac{18}{25}}=cos(A)\)
\(\frac{3\sqrt{2}}{5}=cos(A)\)
Manipulate so that it resembles the given information; remember, any number over itself is (1), multiplying an equation by (1) doesn't change it,
\(\frac{3\sqrt{2}}{5}=cos(A)\)
\(\frac{3\sqrt{2}}{5}*\frac{\sqrt{2}}{\sqrt{2}}=cos(A)\)
\(\frac{3\sqrt{2*2}}{5*\sqrt{2}}=cos(A)\)
\(\frac{6}{5\sqrt{2}}=cos(A)\)
the product of 1540 and m is a square number. find the smallest possible value of m
Answers
Answer:
Step-by-step explanation:
the smallest value is 96.25 or rounded to the nearest whole number it is 96
what i did was put in my calculator 1540 divided by 4^2 because it is squared and has 4 sides
A shopper at a local supermarket spent the following amounts in his last eight trips to the store: $32.92 $14.14 $30.80 $28.34 $75.58 $36.33 $33.51 $22.94 Deleting the amount spent of _________ would greatly reduce the mean. Give your answer as $XX.XX.
Answers
Addition of money spens=$274.56
Divided by 8=$274.56/8
=$34.32
If you have a bit of honesty and sincerity, kindly avoid spamming just for points!
A production process consists of consumption of the following material in Kgs:
49, 50, 38, 60, 75 of materials A, B, C, D. E respectively. How many angle difference between C & D in the pie chart?
a. 25.12% b. 29.12% C.38.15
Need solution with some explanation
Answers
Answer:
29.12%
Step-by-step explanation:
Given that :
A = 49
B = 50
C = 38
D = 60
E = 75
Total = (49 + 50 + 38 + 60 + 75) = 272
Pie chart % for each :
A = (49 / 272) * 360 = 64.85
B = (50 / 272) * 360 = 66.18
C = (38 / 272) * 360 = 50.29
D = (60 / 272) * 360 = 79.41
E = (75 / 272) * 360 = 99.26
Difference between C and D
(79.41 - 50.29) = 29.12%
A school surveys all 7th grade boys to determine what to seek in the school store this sample is
Answers
Answer: Biased
Step-by-step explanation:
It is biased because this survey is based off of opinions, of the 7th grade boys.
2(n + 5) - 6+n Your answer
Answers
Answer:
3n+4
Step-by-step explanation:
Answer:
3n+4 I believe
Step-by-step explanation:
Distributive...
2n+10-6+n
Combine Like terms
2n+n+10-6
3n+10-6
3n+4
the determinant of the sum of two matrices equals the sum of the determinants of the matrices. TRUE OR FALSE?
Answers
The given statement is FALSE. The determinant of the sum of two matrices does not equal the sum of the determinants of the matrices.
In fact, the determinant of the sum of two matrices is generally not even equal to the sum of the determinants of the matrices.
This property does not hold true for determinants. In general, the determinant of the sum of two matrices A and B
(det(A+B)) is not equal to the sum of their individual determinants (det(A) + det(B)).
learn more about determinants:https://brainly.com/question/16981628
#SPJ11
The area of the triangle is 22.4 cm2. Calculate the length, b, of the longest side of the triangle. 97° 3.7 cm a b

Answers
Answer:
Step-by-step explanation:

Discrete math proof
Theorem: 0.1 Let a and n be positive natural numbers. Then the following statements are equivalent. • GCDa, n) = 1 (Relatively Prime) a is not a zero divisor. (ab = 0) b=0) There exists a natural nu
Answers
The theorem states that for positive natural numbers a and n, the statements "GCD(a, n) = 1" (a and n are relatively prime), "a is not a zero divisor," and "there exists a natural number b such that ab ≡ 1 (mod n)" are all equivalent.
How to find the equivalent statements in the theorem regarding positive natural numbers a and n?The theorem establishes the equivalence of three statements concerning positive natural numbers a and n. Firstly, if the greatest common divisor (GCD) of a and n is 1, it indicates that a and n are relatively prime.
This means that they have no common factors other than 1.
The second statement states that if a is not a zero divisor, then it implies that a multiplied by any nonzero element b is not equal to zero. In other words, a does not "annihilate" any nonzero element in multiplication.
Lastly, the theorem asserts that if there exists a natural number b such that ab ≡ 1 (mod n), it signifies the existence of a multiplicative inverse of a modulo n.
This means that a and n have a modular inverse, which is a natural number that, when multiplied by a, gives a remainder of 1 when divided by n.
The theorem shows that these three statements are equivalent, meaning that if one statement is true, then the other two statements will also hold.
The proof of this theorem involves establishing the logical connections between these statements and demonstrating that they are always true under the given conditions.
Learn more about greatest common divisor (GCD)
brainly.com/question/32552654
#SPJ11
Solve for X
-2(5x-5.5) + 8= -9x+2-(-5)
Answers
Answer:
x = 12
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightAlgebra I
Equality PropertiesCombining Like TermsStep-by-step explanation:
Step 1: Define
-2(5x - 5.5) + 8 = -9x + 2 - (-5)
Step 2: Solve for x
Distribute: -10x + 11 + 8 = -9x + 2 + 5Combine like terms: -10x + 19 = -9x + 7Add 10x to both sides: 19 = x + 7Subtract 7 on both sides: 12 = xRewrite: x = 12Estimate the value of the square root of 13.
Answers
Answer:
\(\sqrt{13\\}\) ≈ 3.61
Step-by-step explanation:
The two-way table displays data collected from a random group of middle school and high school students. Which statements are
correct? (frequencies rounded to nearest tenth)
es
A)
The relative frequency of "age 10-13" and "skip breakfast" is 25.9%.
B)
The row conditional relative frequencies of "age 10-13" and "eat breakfast"
is 74.1%.
The row conditional relative frequencies of "age 14-17" and "skip
breakfast" is 66.7%.
The column conditional relative frequencies of eat breakfast" and age 14
17" is 25.5%.
The column conditional relative frequencies of "skip breakfast" and "age
10-13" is 25.6%
D)
E)
Naobra finnertions to Stats and Pre
Answers
Answer:
B) The row conditional relative frequencies of "age 10-13" and "eat breakfast" is 74.1%
C) The row conditional relative frequencies of "age 14-17" and "skips breakfast is 66.7%
D) The column conditional relative frequencies of "eat breakfast" and "age 14-17" is 25.5%
Step-by-step explanation:
PLEASE HELP!!
ILL GIVE MORE POINTS TO THE FASTEST ANSWER

Answers
Fist thing you should know ANY NUMBER WHICH IS TO POWER ZERO THE ANSWER WILL ALWAYS BE ONE!!
Part A : (7^2)^x is (49)^x
(49)^x = 1 , here is basically asking you what’s the value of X to know which number to the power of 49 to put so that it equals to 1, obviously the answer is 0 as we said before ANY NUMBER TO THE POWER OF 0 , the answer will be 1
Part A = 0
————————-
Part B IS GONNA BE WAY EASIER NOW TRUST ME!!
(7^0) = 1
(1)^x = 1
So now is asking what number to put for x for you to multiply by one to get one it’s obviously 1, because 1 x 1= 1
Part B = 1
————-
I hope you understand my explanation so good luck and best of wishes!!
Write an expression to show : 5 times the difference of x and 4.
Answers
Answer:
5(x-4)
Step-by-step explanation:
q(x) = 1⁄2x−3; q(x) = −4
Answers
We can solve with functions exactly as we do algebraic equations. Our goal is to isolate the variable.
Solving the QuestionWe're given:
\(q(x) = \dfrac{1}{2}x-3\\\\q(x) = -4\)
Solve using substitution:
\(\dfrac{1}{2}x-3=-4\)
Isolate x:
\(\dfrac{1}{2}x=-4+3\\\\\dfrac{1}{2}x=-1\\\\x=-2\)
Answerx = -2
One model for the spread of a rumor is that the rate of spread is proportional to the product of the fraction y of the population who have heard the rumor and the fraction who have not heard the rumor. Write a differential equation that is satisfied by y. a. Solve the differential equation. (Let y(0) = y0.)b. Write a differential equation that is satisfied by y.
Answers
The differential equation is equivalent to
\(\frac{dy}{dx} -\frac{3x(1-y)}{x^{2} +1}\)
If y denotes the fraction of the population who have heard the rumor, then 1 −y represents the fraction of the population who haven’t heard the rumor.
The rate of spread of the rumor (y'(t)) being proportional to the product of the fraction y of the population who have heard the rumor and the fraction who have not heard the rumor can then be rewritten as:
\(\frac{dy}{dt} =ky(1-y)\)
for some positive constant k. This is a separable differential equation, so to solve it we separate the variables
\(\frac{dy}{y(1-y)} =kdt\)
and integrate
\(\int\ \frac{dy}{y(1-y)} =\int k dt < = > ln |\frac{y}{1-y} |=kt+c\)
Exponentiating, we get
\(|\frac{y}{1-y}|=e^{kt+c}\)
Denoting ec by A, and taking into account that y ∈ (0, 1) we get
\(\frac{y}1-{y} =Ae^{kt} =\frac{Ae^{kt} }{1}\)
Adding the numerators to the denominators in the above equality of fractions we obtain
\(y=\frac{y}{(1-y)+y} =\frac{Ae^{kt} }{1+Ae^{kt} }\)
The Initial value:
\((x^{2}+1) \frac{dy}{dx} +3y(y-1)=0,y(0)=1\)
The differential equation is equal to
\(\frac{dy}{dx} -\frac{3x(1-y)}{x^2+1}\)
the initial condition of our problem is y(0) = 1, the solution must be y ≡ 1
The differential equation is equivalent to
\(\frac{dy}{dx} +\frac{3x}{x^2+1} y=\frac{3x}{x^2+1}\)
which is a linear equation, with P(x) = Q(x) =\(\frac{3x}{x^2+1}\) we have
\(\int P(x)=\frac{3}{2}\int \frac{2x}{x^2+1} dx=\frac{3}{2} ln(x^2+1)\)
It follows that the integrating factor I(x) is then
\(I(x)e^{\int P(x)dx} =e^{\frac{3}{2} ln(x^2+1)} =(x^2+1)^{\frac{3}{2} }\)
The general technique for solving linear differential equations yields
\(yI(x)=\int Q(x)I(x)dx=\int \frac{3x}{x^2+1} (x^2+1)^{\frac{3}{2} } \\\\=\int 3x(x^2+1)^{\frac{1}{2} } =(x^2+1)^{\frac{3}{2} } +C\)
Dividing by I(x) we get
\(y=1+\frac{C}{(x^2+1)^{\frac{3}{2} } }\)
The initial condition y(0) = 1 yields 1 = 1 + c, i.e. c = 0. Therefore y ≡ 1.
The differential equation is separable. We get this by separating the variables
\(\frac{dy}{y-1} -\frac{-3x}{x^2+1}\)
Integrating, we obtain
\(ln|y-1|=\frac{-3}{2} ln(x^2+1)+c\)
which yields by exponentiation
\(|y-1|=\frac{e^c}{(X^2+1)^\frac{3}{2} }\)
substituting ec by a constant A, and allowing A to also be negative or 0, we get
\(y-1=\frac{A}{(x^2+1)^\frac{3}{2} }\)
The initial condition y(0) = 1 implies that 1 − 1 = A/1 = A, hence A = 0 and y ≡ 1
To know more about the rate of speed:
https://brainly.com/question/15907252
#SPJ4
Katie wants to buy cereal bars from either
the corner shop or the supermarket.
What is the cost of 10 cereal bars at the
cheaper shop?
Give your answer in pounds (£).
Corner shop
5 cereal bars = £4
Supermarket
SUPERMARKET
Discount
2 cereal bars = £1.50
Answers
Using simple mathematical operations, we know that the cost of 10 cereal bars is cheaper in supermarkets which is £7.5.
What are mathematical operations?An operation is a function in mathematics that transforms zero or more input values into a clearly defined output value.
The operation's arity is determined by the number of operands.
A formula would be 3x - 5 = 16, for instance.
When this equation is solved, we discover that the value of the variable x is 7.
So, we know that :
Corner shop: 5 cereal bars = £4
1 cereal bar: 4/5 = £0.8
10 cereal bars: 0.8 * 10 = £8
Supermarket: 2 cereal bars = £1.50
1 cereal bar: 1.5/2 = £0.75
10 cereal bars: 0.75 * 10 = £7.5
Therefore, using simple mathematical operations, we know that the cost of 10 cereal bars is cheaper in supermarkets which is £7.5.
Know more about mathematical operations here:
https://brainly.com/question/28937023
#SPJ1
May I please receive help?
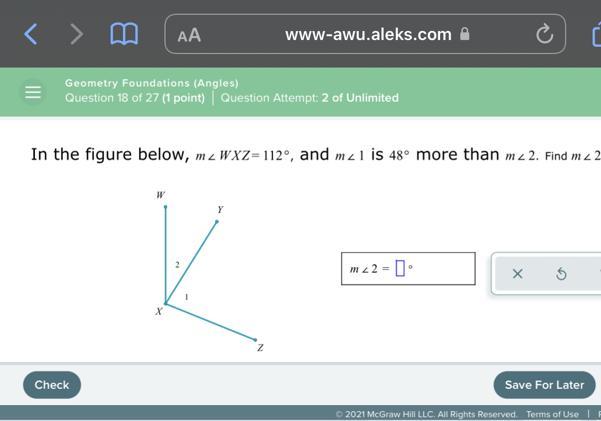
Answers
Hi there!
We know that:
m∠WXZ = 112°
Since m∠1 is 48° greater than m∠2, we can write an expression:
m∠2 = x°
m∠1 = (x + 48)°
Since both angles sum up to m∠WXZ, we can write this as:
112° = x° + (x + 48)°
Solve for x:
112 = 2x + 48
64 = 2x
x = 32°
In a second grade class containing 14 girls and 8 boys, 2 students are selected at random to give out the math papers. What is the probability that the second student chosen is a girl, given that the first one was a boy?
Answers
The required probability is 13/20.
Given that,
Number of girls = 14
Number of boys = 8
Since probability = (number of favorable outcomes)/(total outcomes)
Therefore,
The probability of selecting a boy = 8/22
= 4/11.
We have to find the probability that the second student chosen is a girl, given that the first one was a boy
Since we already know that the first student chosen was a boy,
There are now 13 girls and 7 boys left to choose from.
So,
The probability of selecting a girl as the second student = 13/20
Hence,
The probability that the second student chosen is a girl, given that the first one was a boy, is 13/20.
Learn more about the probability visit:
https://brainly.com/question/13604758
#SPJ1
Geneticists have discovered the existence of meiotic drive genes, which have alleles that, when present in a heterozygous state, are able to become incorporated into much more than 50 percent of the gametes. As a result, the offspring ratios are not what mendel would have predicted. Suppose that the short allele is a meiotic drive gene, and 90 percent of the gametes from a heterozygous individual with tall and short alleles contain short alleles. If tall is dominant to short, what percent of individuals from a cross between a heterozygous tall individual and a homozygous recessive individual will be tall?.
Answers
10% of individuals from a cross between a heterozygous tall individual and a homozygous recessive individual will be tall.
The two alleles present at a gene locus segregate from one another during gamete production, according to Mendel's first law of segregation, and each gamete has a 50% chance of harboring any one of the two alleles.
In meiotic drive genes, the alleles can become absorbed into considerably more than 50% of the gametes if they are present in a heterozygous state, which is against the law of segregation.
Here,
90% of the gametes from a heterozygous individual with tall and short alleles contain short alleles.
If tall dominates short, then 10% of individuals from a cross between a heterozygous tall individual and a homozygous recessive individual will be tall.
Learn more about genes here;
https://brainly.com/question/17088476
SPJ4
Is a fraction always less than a whole number
Answers
Answer:
Yes.
Step-by-step explanation:
A fraction is a part of a whole number, so yes a fraction is always less than a whole number, unless it's a mixed number. A mixed number is when there is a whole number before the fraction.
Plaz mark brainliest. I just need 2 more to raise my level.
Answer:
Yes unless the fraction is equal to a whole number or is an improper fraction
Step-by-step explanation:
A normal fraction like 2/4 is less than a whole number because a whole number is whole and a fraction is part of
Exceptions:
4/4 is a fraction but is also a whole number
any improper fraction ( a fraction not simplified)
5/4 it equals more than a whole number 1 1/4
So simplify the fraction and if it is equal to or more than a whole number you know, but if it is less than a whole number then you know it is less.
Hopefully this helped :)
In a sequence diagram, a lifeline is identified by a _____ line.
a.curved
b.red
c.solid
d.dashed
Answers
A lifeline in a sequence diagram is a line that is used to represent an active object, such as a class, interface, or object, and is typically identified by a solid line.
A lifeline in a sequence diagram is a graphical element used to depict the active objects in an interaction or sequence diagram. The lifeline is typically represented as a solid line running from the top of the diagram to the bottom, which represents the object's lifetime. The lifeline is used to show the messages that are sent and received between objects, as well as the sequence in which they occur. Additionally, the lifeline is often used to represent any changes of state in the objects, such as method calls, data values, and more. By depicting the lifelines of the active objects in a sequence diagram, it is possible to gain a better understanding of the interactions between the objects, and how they are related.
Learn more about sequence here
https://brainly.com/question/21961097
#SPJ4
Can someone help me with this please

Answers
Answer: get smart bro
you would know it if you was smart
Step-by-step explanation:
you wont do your work, you always cheat, and you don't listen to the teacher
you need to find the answer out on your own.
P.S. i hope you have a good day :)