Answers
Answer:
849.9 cm squared
Step-by-step explanation:
Related Questions
Madaly was extremely excited to go on vacation. HELP!!!!
The underlined part of the sentence can best be described as a/an...
adjective phrase.
prepositional phrase.
adverbial phrase.
noun phrase.
Answers
The underlined part of the sentence can best be described as an adjective phrase.
Parts of speechIn formation of sentences in English language, the words that are used usually include the following parts of speech:
nounspronounsverbsadjectivesadverbsprepositionsconjunctionsinterjectionsAn Adjective is a word that describes or modifies a noun or a pronoun. While adjective phrase is the phrase that can be used to modify or describe a noun in a sentence.
From the sentence given, extremely excited is adverbial phrase used to modify the noun vacation.
Learn more about parts of speech here:
https://brainly.com/question/721326
Ms. McNeil buys 5.2 gallons of gasoline. The total cost is $17.16.
Complete and solve the equation to find the price p in dollars of one gallon of gasoline.
p = 17.16
The price of one gallon of gasoline is $.
I need help finding the answer
Answers
Answer: 3.3
Step-by-step explanation:
Answer:
$3.30 per gallon.
Step-by-step explanation:
17.16 ÷ 5.2 = 3.3
Total cost ÷ number of gallons = price for one gallon.
a card is drawn from a standard deck of 52 playing cards. what is the probability that the card will be a spade or a club? express your answer as a fraction or a decimal number rounded to four decimal places.
Answers
The probability that the card will be a spade or a club 50%
Probability in math is referred as how likely an event is to occur, or how likely it is that a proposition is true.
Here we have given that a card is drawn from a standard deck of 52 playing cards.
And we need to find the probability that the card will be a spade or a club and we have to express your answer as a fraction or a decimal number rounded to four decimal places.
While we looking into the given question, we have identified the the total number of cards is 52.
And the number of spade cards = 13 and the number of club cards = 13
Therefore, the total number of spade and club cards
=> 13 + 13
=> 26
Therefore, the probability of getting a spade or a club from a single draw is,
=> 26/52
When we simplified this one, then we get,
=> 0.5
When we convert this into percentage then we get
=> 50%.
To know more about Probability here.
https://brainly.com/question/11234923
#SPJ4
Which cooking methods would work best if you are on a camping trip and have to build your own
fire for cooking? What types of foods would you bring along that would work best with these
cooking methods and setting?
Answers
Answer:
The best cooking methods would be to cook something simple, and reasonable for the campfire. Such as something that can cook in a pan or a pot. I wouldn't recommend frozen food. Since the best cooking method is to cook something that can cook in a pan or a pot here are some suggestions. I would suggest hot dogs, soup, beans, grilled cheese sandwich, and for dessert s'mores of course.
Step-by-step explanation:
Hope this helps!! If you need to add sum you can.
1. The best cooking method for a camping trip is Roasting using Skewers.
2. The best foods for this cooking method and setting are hot dogs and grilled cheese sandwiches.
What are the cooking methods for camping?Depending on the equipment in use, some cooking methods for camping are:
Sauteing GrillingRoastingBaking.Thus, since the foods would be roasted over an open fire, using skewers would ensure that they are well-roasted.
Learn more about camping cooking methods at https://brainly.com/question/6813887
#SPJ2
Kadita counted 30 pigs and chickens on the farm. Jack counted a total of 68 legs for the animals. How many chickens were there on the farm?.
Answers
6 chickens and 14 pigs in the farm.
Let the number of chickens in the farm = x
Let the number of pigs in the farm = y
Number of legs of chickens = 2*x (since chickens have 2 legs)
Number of legs of pigs = 4*y (since pigs have 4 legs)
We are given that there are a total of 30 animals (pigs + chickens)
so, number of chickens + number of pigs = 30
x + y = 30
We are given that there is a total of 68 legs in the farm
so, legs of chickens + legs of pigs = 68
2x + 4y = 68
Solving for number of chickens and pigs:
from the question, we have deduced that:
x + y = 30
2x + 4y = 68
taking the value of x from the first equation and using it in the second equation:
x = 30 - y
2(30-y) + 4y = 68
60- 2y + 4y = 68
40 + 2y = 68
2y = 68-40
2y = 28
y = 14
Using this value of y in the first equation:
x + y = 30
x + (14) = 20
x = 20-14
x = 6
Therefore, there are 6 chickens and 14 pigs in the farm
Learn more about to counted visit here:
https://brainly.com/question/21555185
#SPJ4
Express the statement as a formula that involves the given variables and a constant of proportionality k. r is directly proportional to the product of s and v and inversely proportional to the cube of p. Determine the value of k from the given conditions. Ifs - 2, V - S, and p 7, then r56.
Answers
\(ksv/p³r ∝ sv/p³r = ksv/p³\) (where k is the constant of proportionality)
\(56 = k × 2 × 2 × 2 / 7³(k × 8 × 7³) / (2³)\)
\(= 56k = (56 × 343)/8k\)
= 2401`. So the answer is 2401.
`r is directly proportional to the product of s and v and inversely proportional to the cube of p`We can write the above statement as a formula as follows: `r ∝ sv/p³` (where ∝ means proportional to)We can introduce a constant of proportionality k and write:\(`r = ksv/p³`\)where k is the constant of proportionality.
Now we need to find the value of k from the given conditions.s = 2,
v = s
= 2,
p = 7, and
r = 56Substituting the given values of s, v, p, and r, we get:
\(`56 = k × 2 × 2 × 2 / 7³\)`Simplifying the above equation:
\(`56 = (8k)/343`\)Multiplying both sides by `343/8`, we get:
\(`k = (56 × 343)/8k = 2401`.\)
To know more about proportionality visit:-
https://brainly.com/question/8598338
#SPJ11
A student deposited money into a savings account. The following equation models the amount of money in the account, A(1), after t years. A(1)-1575 (1.045) a. State the initial amount of money deposited into the account. b. Determine the annual interest rate being paid on the account. C. Use the equation to find the amount of money, to the nearest dollar, in the account after 15 years. d. How many years, to the nearest whole year, will it take for the account to have at least $4000?
Answers
a. The initial amount of money deposited into the account is $1575.
b. The annual interest rate being paid on the account is 4.5%.
c. The amount of money in the account after 15 years is approximately $2946.27.
d. It will take approximately 20 years for the account to reach a balance of at least $4000.
To answer these questions, let's analyze the given equation:
A(1) = 1575 * (1.045)^t
a. The initial amount of money deposited into the account is $1575. This is evident from the equation, where A(1) represents the amount of money after 1 year.
b. To determine the annual interest rate, we can compare the given equation with the general formula for compound interest:
A = P * (1 + r)^t
Comparing the two equations, we can see that the interest rate in the given equation is 4.5% (0.045) since (1 + r) is equal to 1.045.
c. To find the amount of money in the account after 15 years, we can substitute t = 15 into the equation and calculate the result:
A(15) = 1575 * (1.045)^15 ≈ $2946.27 (rounded to the nearest dollar)
Therefore, after 15 years, the amount of money in the account will be approximately $2946.
d. To find the number of years it will take for the account to have at least $4000, we need to solve the equation for t. Let's set up the equation and solve for t:
4000 = 1575 * (1.045)^t
To solve this equation, we can take the logarithm of both sides (with base 1.045):
log(4000) = log(1575 * (1.045)^t)
Using logarithm properties, we can simplify the equation:
log(4000) = log(1575) + log((1.045)^t)
log(4000) = log(1575) + t * log(1.045)
Now, we can isolate t by subtracting log(1575) from both sides and then dividing by log(1.045):
t = (log(4000) - log(1575)) / log(1.045)
Calculating this expression, we find:
t ≈ 19.56 (rounded to two decimal places)
Therefore, it will take approximately 20 years (rounded to the nearest whole year) for the account to have at least $4000.
Learn more about "amount ":
https://brainly.com/question/25720319
#SPJ11
the dotplot above shows the distribution of calf measurements for 507 adults who attend a local gym. the data is divided into equal-sized subgroups with 25% of the adults in each subgroup. which measure of center can you most accurately estimate from this information?
Answers
which measure of center can you most accurately estimate from this information: Median
The median divides the data into equal-sized groups. So approximately 50% of the data is above the median and approximately 50% of the data is below the median.
Calculating the median
The median is the value in a data set, implying that half of data focuses have a value more modest or equivalent to the median and half of data focuses have a value higher or equivalent to the median.
For a little data set, you first count the quantity of data focuses (n) and organize the data focuses in expanding request. Assuming the quantity of data focuses is lopsided, you add 1 to the quantity of places and separation the outcomes by 2 to get the rank of the data point whose value is the median.
The rank is the place of the data point after the data set has been organized in expanding request: the smallest value is rank 1, the second-smallest value is rank 2, and so forth.
to know more about median click here:
https://brainly.com/question/16408033
#SPJ4
the complete question is:
The dotplot above shows the distribution of calf measurements for 507 adults who attend a local gym. The data is divided into equal-sized subgroups with 25% of the adults in each subgroup.
Which measure of center can you most accurately estimate from this information?
1. mean
2. median
The average life of a bread-making machine is 7 years, with a standard deviation of 1 year. Assuming that the lives of these machines follow approximately a normal distribution, findb. The value of x to the right of which 15% of the means computed from a random sample of size 9 would fall
Answers
The value of x from a random sample of size 9 is approximately 7.345 years.
How to find the value of x to the right of which 15% of the means computed from a random sample of size 9 would fall?To find the value of x to the right of which 15% of the means computed from a random sample of size 9 would fall, we need to consider the sampling distribution of the sample means.
For a normal distribution, the sampling distribution of the sample means will also follow a normal distribution.
The mean of the sampling distribution will be the same as the population mean, which is 7 years in this case.
The standard deviation of the sampling distribution, also known as the standard error, can be calculated by dividing the population standard deviation by the square root of the sample size.
Standard error = σ / \(\sqrt(n)\)
Given that the population standard deviation is 1 year and the sample size is 9, we can calculate the standard error:
Standard error = 1 / \(\sqrt(9)\) = 1/3
Since the distribution is symmetric, we can find the value of x to the right of which 15% of the means fall by finding the z-score corresponding to the 85th percentile (100% - 15% = 85%).
Using a standard normal distribution table or statistical software, we can find that the z-score corresponding to the 85th percentile is approximately 1.036.
Now, we can calculate the value of x:
x = μ + z * SE
where μ is the population mean (7 years), z is the z-score (1.036), and SE is the standard error (1/3).
x = 7 + 1.036 * (1/3) = 7 + 0.345 = 7.345
Therefore, the value of x to the right of which 15% of the means computed from a random sample of size 9 would fall is approximately 7.345 years.
Learn more about sampling distribution
brainly.com/question/31465269
#SPJ11
Can anyone please help me solve for x, y, and z?

Answers
Answer:
z= 3
y = 105
x= 90
Step-by-step explanation:
y-12 = 90+z
y-18+z = 90
y = z + 102
z + 102 - 18 + z = 90
2z = 90 - 84
z = 3
Which interval notation represents the set of all numbers from -3 to 0, inclusive?

Answers
Answer:
{ - 3, 0 ]
Step-by-step explanation:
parenthesis ( ) is used when the interval does not include the end values
brackets [ ] are used when the interval includes the end values
The end values here are - 3 and 0 so brackets are used, that is
[ - 3, 0 ]
You have been making monthly deposits of $20 into a savings account with monthly compounding for 8 years, and you do not know what the account's apr is (so you cannot use the monthly deposits balance formula to compute the balance exactly). eventually you remember that the apy (not the apr) is 1.2%. use the upper bound/estimate for the balance (from the course video) to determine an upper bound for your account's balance.
Answers
Upper bound for your account's balance is $323.76
Lower bound = P * (1 + r/n)^nt
Upper bound = P * (1 + r)^t
Where:
P = monthly deposit ($20)
r = APY (1.2%)
n = number of compounding periods per year (12)
t = number of years (8)
Upper bound = 20 * (1 + 0.012)^8
Upper bound = $323.76
Learn more about Upper bound here:
https://brainly.com/question/22965427
#SPJ4
how many integers must a set s contain to be certain that at least 35 integers in s have the same remainder when divided by 4?
Answers
Set S should contain 137 number of integers so that at least 35 integers in S have same remainder when divided by 4.
What is pigeonhole principle?According to the pigeonhole principle in mathematics, at least one container must hold more than one item if n things are placed into m containers, where n > m. As an illustration, if one has three gloves (none of which are ambidextrous or reversible), at least two of them must be right-handed or left-handed as there are three items but only two categories of handedness to classify them into. It is possible to establish potentially surprising consequences using this seemingly obvious assertion, a type of counting argument.
Equating pigeonhole principle :Given: Set S = {}, least number of integers having same remainder when divided by 4=35
To find: Number of integers in set S so that at least 35 of the integers in set S have same remainder?As we know, any number when divided by 4 has following remainder = 0, 1, 2, 3.
Least number of integers having same remainder when divided by 4 = 35.
∴ Number of integers in set S = 35×4
= 140
but 3 is to be subtracted from 140 as it is the last different remainder after which the remainders repeat in manner 0, 1, 2 & 3.
∴ Total number of integers in set S = 140-3
= 137
To learn more pigeonhole principle :
brainly.com/question/13982786
#SPJ4
14. PLEASE HELP ME
Which of the quadratic functions has the narrowest graph?
A. y= 1/8x2
B. y= 1/6x2
C. y= -x2
D. y= 2x2
Answers
Answer:
D. y= 2x2
Step-by-step explanation:
y = ax^2
If the absolute value of a is <1 the graph is wider than when a = 1
If the absolute value of a is >1 the graph is narrow than when a = 1
The only function that fits that description is
y = 2x^2
B <<< answer.
Given: We are given equation of parabola ans to choose narrowest graph.
Parabola form narrowest and widest.
Larger value of a most narrowest graph.
Smaller value of a most widest graph.
Now, we will see the coefficient of x²
Now, we arrange the value of a in descending order.
2 is largest value of these.
Hence, The narrowest graph is
there are 2 more pictures i have to upload but it won't let me





help me im really bad at math

Answers
Answer:
Greater than or >
Step-by-step explanation:
Since it is negative the -1/2 is greater than the -3.75
Hope this helps! Pls give brainliest!
Convert -3.75 to fractions
\(\\ \rm\rightarrowtail \dfrac{-375}{100}\)
\(\\ \rm\rightarrowtail \dfrac{-75}{20}\)
\(\\ \rm\rightarrowtail \dfrac{-15}{4}\)
\(\\ \rm\rightarrowtail -3\dfrac{3}{4}\)
Greater than sign should be usedI need help!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!

Answers
Answer: 151/20 < 7.611 < 7 5/8 < 8.66
Step-by-step explanation:
First convert the fractions to decimals.
151/20= 7.55
7 5/8 = 7.625
The other two numbers are 7.611 and 8.66
In all the numbers the least is 7.55, then comes , 7.611, 7.625 and 8.66 .
PLEASE HELP DUE SOON NEED CALCULATIONS TOO Trevor is buying carpet for his rectangular living room the length if this living room is 3 feet more than his width the area of the living room is 88
Answers
Answer:
12
Step-by-step explanation:
5
Explain how to calculate each of these mentally.
1. 15 is what percentage of 30?
2. 3 is what percentage of 12?
3. 6 is what percentage of 10?
Answers
2. 25%
3. 60%
Example: What is 10% of 150?
Convert the problem to an equation using the percentage formula: P% * X = Y
P is 10%, X is 150, so the equation is 10% * 150 = Y
Convert 10% to a decimal by removing the percent sign and dividing by 100: 10/100 = 0.10
Substitute 0.10 for 10% in the equation: 10% * 150 = Y becomes 0.10 * 150 = Y
Do the math: 0.10 * 150 = 15
Y = 15
So 10% of 150 is 15
Double check your answer with the original question: What is 10% of 150? Multiply 0.10 * 150 = 15
Simplify
-4r + S - 2r - 3s
Answers
Answer:-6s-2r
Step-by-step explanation:
you must add all like terms in this case S -4-3 is -7. -7+1=-6.
-6s-2r
Answer:
-6r - 2s
Step-by-step explanation:
Given expression,
→ -4r + s - 2r - 3s
Let's simplify the expression,
→ -4r + s - 2r - 3s
→ (-4r - 2r) + (s - 3s)
→ -6r + (-2s)
→ -6r - 2s
Hence, the answer is -6r - 2s.
evaluate this pls quiick i have 18 min left online exams help
-0.0004 x 0.02
Answers
Given:
The expression is:
\(0.0004\times 0.02\)
To find:
The value of the given expression.
Solution:
We have,
\(0.0004\times 0.02\)
It can be written as:
\(=\dfrac{4}{10000}\times \dfrac{2}{100}\)
\(=\dfrac{8}{1000000}\)
\(=0.000008\)
Therefore, the value of the given expression is 0.000008.
A store has 125 scientific calculators.
Brand x sells for $20 each; Brand y sells
for $15 each. If the total selling price
of the calculators is $2,275, how many
of each calculator does the store have?
Answers
Answer:
Brand x: 80
Brand y: 45
Step-by-step explanation:
x + y = 125
20x + 15y = 2275
-20x - 20y = -2500
(+) 20x + 15y = 2275
--------------------------------
-5y = -225
y = 45
x + y = 125
x + 45 = 125
x = 80
Answer:
Brand x: 80
Brand y: 45
Which graph represents the function of f(x) = 4x^2-16/2x-4 ?

Answers
See the graph in the image.
y = (4x² - 16)/(2x - 4)y = [4(x-2)(x+2)]/[2(x-2)]y = 2(x+2), with a domain of all real numbers other than 2
A function is a relationship between inputs where each input is related to exactly one output.
The graph of f(x) = (4x² - 16) / (2x - 4) is given below.
What is a function?A function is a relationship between inputs where each input is related to exactly one output.
Example:
f(x) = 2x + 1
f(1) = 2 + 1 = 3
f(2) = 2 x 2 + 1 = 4 + 1 = 5
The outputs of the functions are 3 and 5
The inputs of the function are 1 and 2.
We have,
Function:
f(x) = (4x² - 16) / (2x - 4)
This can be written as,
f(x) = (2x)² - 4² / (2x - 4) [ a² - b² = (a + b)(a - b) ]
f(x) = (2x - 4)(2x + 4) / (2x - 4)
f(x) = 2x + 4
Now,
We will find the coordinates from the function f(x) = 2x + 4
x = 0, f(x) = 4
(0, 4)
x = 1, f(x) = 6
(1, 6)
x = 3, f(x) = 10
(3, 10)
And so on.....
The graph is given below,
Thus,
The graph of f(x) = (4x² - 16) / (2x - 4) is given below.
Learn more about functions here:
https://brainly.com/question/28533782
#SPJ2

bacteria in a culture increase at a rate that is proportional at any time to the number of bacteria at that time. there were 800 800800 bacteria initially, and they triple every 10 1010 hours. what is the number of bacteria after 4 44 hours? round to the nearest integer.
Answers
The number of bacteria in the culture after 4 hours is 903.
Given data:
Since the bacteria triple every 10 hours, the growth rate constant k can be determined using the exponential formula:
\(P(t)=P_0e^{kt}\)
Where:
\(P(t)\\\) is the number of bacteria at time t.
\(P_0\) is the initial number of bacteria (800 in this case)
k is the growth constant.
t is the time in hours.
Given that the bacteria triple every 10 hours, we know that after 10 hours, the number of bacteria becomes three times the initial count. Therefore, we can set up the equation:
\(3P_0=P_0e^{(10k)}\)
On simplifying the equation:
\(k = \frac{ln3}{10}\)
Now, we can use the value of k to find the number of bacteria after 4 hours:
\(P(4)=800(e^{\frac{ln3}{10}*4})\)
On simplifying the equation:
P(4) = 903
Hence, the number of bacteria is 903.
To learn more about exponential form, refer:
https://brainly.com/question/29337692
#SPJ12
about how long would it take Vicky to read 25 pages? Explain.
Answers
Answer:
so i do not have that many resources like how fast she can read, how many pages there are, and how many words are on the page
Step-by-step explanation:
if she is and older person than i can say 25 pages in 13 or 14 minutes but if she is younger than i can say 20 or 22 minutes i hope this helps with your problem
please answer with steps
txt: identify the domain and range of the following relation and determine if the relation is a function. Explain why or why not.

Answers
We need to know about relation and function to solve the problem. The domain of the relation is {-4,2,7,10} and the range is {-4}. The relation is a function.
Relation is a subset of Cartesian product or we can say that it is a set of points. It can also be described as a collection of ordered pairs.Function is a relation which follows that each input should have only one output. The domain of a relation or function is the set of values that the relation can accept, the range of a relation is the set of values that can be an output of the function or relation. The x values are the inputs and form the domain whereas the y values give the range. In the given relation the domain of the relation is {-4,2,7,10} and the range of the relation is {-4} since there is only one ouput value. The relation is a function since every input has only one output.
Therefore the domain of the relation is {-4,2,7,10} and the range is {-4} and the relation is function.
Learn more about relation and function here:
https://brainly.com/question/10377915
#SPJ1
What are the coordinates of the point on the directed line segment from (6,2) to (8,−10) that partitions the segment into a ratio of 1 to 3?
Answers
The coordinates of the point that divides the line segment from (6, 2) to (8, -10) into a ratio of 1 to 3 are (7, -1).
To find the coordinates of the point on the directed line segment that partitions it into a ratio of 1 to 3, we can use the concept of section formula.
The section formula states that if we have two points A(x₁, y₁) and B(x₂, y₂) dividing a line segment in the ratio of m₁ : m₂, then the coordinates of the dividing point P are given by:
Px = (m₁ * x₂ + m₂ * x₁) / (m₁ + m₂)
Py = (m₁ * y₂ + m₂ * y₁) / (m₁ + m₂)
In this case, the ratio is 1:3, which means m₁ = 1 and m₂ = 3. The given points are A(6, 2) and B(8, -10). Substituting these values into the formula, we can calculate the coordinates of the dividing point P:
Px = (1 * 8 + 3 * 6) / (1 + 3) = 7
Py = (1 * -10 + 3 * 2) / (1 + 3) = -2/2 = -1
Therefore, the coordinates of the point that divides the line segment from (6, 2) to (8, -10) into a ratio of 1 to 3 are (7, -1).
To find the coordinates of the point that divides the line segment between (6, 2) and (8, -10) in a 1:3 ratio, we can use the section formula. Applying the formula, where m₁ is 1 and m₂ is 3, the point P(x, y) can be determined.
By substituting the values into the formula, the x-coordinate is calculated as (1 * 8 + 3 * 6) / (1 + 3) = 7, and the y-coordinate is (1 * -10 + 3 * 2) / (1 + 3) = -1. Thus, the coordinates of the point that partitions the line segment into a ratio of 1 to 3 are (7, -1).
For more such questions segment,click on
https://brainly.com/question/28322552
#SPJ8
Which amount is largest?
1 kg
1 dg
100 g
1,000 mg
Answers
Answer:
1 kg here you go this is the answer
Can anyone please help me!!
Use the discriminant to determine the number of real solutions of the quadratic equation.
-4x^2 - 2x +1=0
Answer choices:
Zero
One
Two
SECOND Question:
Use the Quadratic Formula to solve the equation. (Enter your answers as a comma-separated list.)
24x^2 -30x +9=0
x=?

Answers
Number of real solutions of -4x²- 2x +1=0 is two
The value of x = 3/4 and 1/2
Quadratic Formula:A quadratic equation, or quadratics, is a polynomial equation with a maximum degree of two. It takes the following form: ax²+bx+c = 0.
The quadratic Formula is the formula that is used to find the roots or solution of a quadratic equation. For the standard quadratic equation, the ax²+bx+c = 0 quadratic formula is given by
x = [-b±√(b²-4ac)]/2aWhere, Discriminant, d = b²-4ac
8. Given equation -4x²- 2x +1=0
From the discriminant formula,
Discriminant, d = b² - 4ac
Compare given equation with ax²+ bx + c = 0
=> a = -4, b = -2 and c = 1
Discriminant, d = (-2)² - 4(-4)(1)
= 4 + 16 = 20
Since the discriminant is positive, the given equation will have two solutions.
9. 24x² - 30x + 9 = 0
Compare given equation with ax²+ bx + c = 0
=> a = 24, b = -30 and c = 9
From the quadratic formula x = [-b±√(b²-4ac)]/2a
x = [- (-30) ±√((-30)²- 4(24) (9)]/2(24)
x = [ 30 ±√900 - 864]/48
=> x = [ 30 + √900 - 864]/48 and x = [ 30 - √900 - 864]/48
=> x = [ 30 + √36]/48 and x = [ 30 - √36]/48
=> x = [ 30 + 6]/48 and x = [ 30 -6]/48
=> x = [ 36]/48 and x = [ 24]/48
=> x = 3/4 and x = 1/2
Therefore,
Number of real solutions of -4x²- 2x +1=0 is two
The value of x = 3/4 and 1/2
Learn more about Quadratic Formula at
https://brainly.com/question/2615966
#SPJ1
Write the quadratic equation whose roots are -6 and 6, and whose leading coefficient is 1 .
Answers
Answer:
The quadratic equation is: \(y=x^2-36\)
Step-by-step explanation:
If the roots of the quadratic equation are "-6" and "6", then it must have the following factors: \((x+6)\,\, and \,\,(x-6)\)
Therefore, we can write the equation in factor form as:
\(y=a\,(x+6)\,(x-6)\)
where a is a real number constant factor. Now, this equation in standard form will look like:
\(y=a\,(x^2+6x-6x-6^2)=a\,(x^2-36)=ax^2-36\,a\)
Therefore, using the information about the leading coefficient being "1" (one), we derive that the constant factor \(a\) must be "1". The final expression for the quadratic becomes:
\(y=x^2-36\)
Desperate
please Hurry
Question Down Below
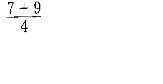
Answers
7+9/4
16/4
4/1
4 is the answer
It’s extremely blurry
