Answers
Answer:
Step-by-step explanation:
solution 1:
570 not man = 15% (cause 100% - 85%)
15% = 570
100% = w (for "whole package")
15w = 100 * 570
15w = 57000
w = 57000 / 15
w = 3800
solution 2:
15% = 570
85 = m (for "men)
15m = 570 * 85
15m = 48450
m = 48450 / 15
m = 3230
100% = 85% + 15%
100% = b (for "buch of people")
b = 3230 + 570
b = 3800
Related Questions
cat este 18 se divide la (x+1) daca x este un numar natural
Answers
Answer:
mai mic număr natural care se divide cu 24, 36 și 60. 9. ... 2. Dacă fracțiile. 12 x și. 1. 3 sunt echivalente, atunci x este: a) 36; b) 2; c) 3; ... D = Mulțimea numerelor naturale care, înmulțite cu 3, dau 18; E= Mulțimea divizorilor lui 9. 6.
Step-by-step explanation:
mai mic număr natural care se divide cu 24, 36 și 60. 9. ... 2. Dacă fracțiile. 12 x și. 1. 3 sunt echivalente, atunci x este: a) 36; b) 2; c) 3; ... D = Mulțimea numerelor naturale care, înmulțite cu 3, dau 18; E= Mulțimea divizorilor lui 9. 6.
7.) According to the quantity equation, changes in the money supply will lead directly to
changes in the price level if velocity and real GDP are unaffected by the change in the
money supply. Will velocity change over time? What factors might lead to changes in
velocity? Are those changes related to changes in the money supply?
Answers
According to the quantity theory of money, changes in the money supply will lead directly to changes in the price level if velocity and real GDP are unaffected by the change in the money supply.Velocity can change over time, and changes in velocity may be caused by various factors.
For example, changes in velocity can be caused by shifts in payment practices, changes in the use of credit, changes in the availability of bank deposits or cash, or shifts in demand patterns.Changes in velocity may be related to changes in the money supply.
For example, if the money supply increases, the demand for money may increase, causing the velocity of money to decrease. Conversely, if the money supply decreases, the demand for money may decrease, causing the velocity of money to increase.
For more question on velocity
https://brainly.com/question/25749514
#SPJ8
Gabriel finds some wooden boards in the backyard with lengths of 5 feet, 2.5 feet and 4 feet. He decides he wants to make a triangular garden in the yard and uses the triangle inequality rule to see if it will work. Which sums prove that the boards will create a triangular outline for the garden? Select all that apply. 5 + 2.5 > 4 5 + 2.5 < 4 4 + 2.5 > 5 4 + 2.5 < 5 4 + 5 > 2.5
Answers
So the correct possible triangular outline for the garden is:
5 + 2.5 > 4
4 + 2.5 > 5
4 + 5 > 2.5
Given information:
Gabriel finds some wooden boards in the backyard with lengths of 5 feet, 2.5 feet, and 4 feet.
He decides he wants to make a triangular garden in the yard and uses the triangle inequality rule to see if it will work.
The sums that prove that the boards will create a triangular outline for the garden are:
5 + 2.5 > 4
4 + 2.5 > 5
4 + 5 > 2.5
So the correct options are:
5 + 2.5 > 4
4 + 2.5 > 5
4 + 5 > 2.5
These sums satisfy the triangle inequality rule, which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the remaining side.
To learn more about triangle inequality;
https://brainly.com/question/1163433
#SPJ1
1.Write an inequality comparing the rational numbers -3/4 and -2 .
2. Using complete sentences, explain what your inequality means about the position of the two numbers on the number line.
Answers
Answer:
\(-2<x<-\dfrac{3}{4}\) Or \(x\in (-2,-\dfrac{3}{4})\)
Step-by-step explanation:
The numbers are \(-\dfrac{3}{4}\) and \(-2\).
We need an inequality that gives us the numbers between the above numbers.
The inequality will be
\(-2<x<-\dfrac{3}{4}\)
Or
\(x\in (-2,-\dfrac{3}{4})\)
This means that \(x\) is a number that is in between the rational numbers \(-\dfrac{3}{4}\) and \(-2\). Also, \(x\) is greater than \(-2\) and less than \(-\dfrac{3}{4}\)
The positions in the number line are shown in the figure where the numbers are marked by arrow.

PLEASE HELP
Suppose that the functions fand g are defined for all real numbers x as follows.
f(x) = 5x
g(x)=4x-4
Write the expressions for (g.f)(x) and (g-f)(x) and evaluate (g+f)(2).
(g•f)(x) =
(g-f)(x) =
(g+r) (2)=
Answers
= 20x^2 - 16
(g - f)(x) = (4x - 4) - (5x)
= (-1x) - 4
= -x -4
(g + f)(2) = (4(2) - 4) + (5(2))
= 4 + 10
= 14
5. Evaluate the function f (x) = 4•7^x for x=-1 and x= 2. Show your work.
Answers
Answer:
Given
f
(
x
)
=
4
⋅
7
x
For
x
=
−
1
we have
f
(
−
1
)
=
4
⋅
7
−
1
=
4
7
using
a
−
n
=
1
a
n
For x=2, we have
f
(
2
)
=
4
⋅
7
2
=
196
Step-by-step explanation:
Answer:
See below
Step-by-step explanation:
\(f(x) = 4. {7}^{x} \\ \\ plug \: x = - 1 \\ \\ f( - 1) = 4. {7}^{ - 1} \\ \\ f( - 1) = \frac{4}{7} \\ \\plug \: x = 2 \\ \\ f( 2) = 4. {7}^{ 2} \\ \\ f( 2) = 4 \times 49 \\ \\f( 2) = 196 \\ \\\)
Calculate the distance between the points P=(1, -1) and N=(5, -8) in the coordinate plane.
Round your answer to the nearest hundredth.
PLEASE PLEASE HELP ME

Answers
\(~~~~~~~~~~~~\textit{distance between 2 points} \\\\ P(\stackrel{x_1}{1}~,~\stackrel{y_1}{-1})\qquad N(\stackrel{x_2}{5}~,~\stackrel{y_2}{-8})\qquad \qquad d = \sqrt{( x_2- x_1)^2 + ( y_2- y_1)^2} \\\\\\ PN=\sqrt{(~~5 - 1~~)^2 + (~~-8 - (-1)~~)^2} \implies PN=\sqrt{(5 -1)^2 + (-8 +1)^2} \\\\\\ PN=\sqrt{( 4 )^2 + ( -7 )^2} \implies PN=\sqrt{ 16 + 49 } \implies PN=\sqrt{ 65 }\implies PN\approx 8.06\)
help! exponent grade

Answers
16a^24/ b^16c^8
Find the area of a circle with diameter 12 m.
Use the value 3.14 for n, and do not round your answer.
Be sure to include the correct unit in your answer.

Answers
Answer:
113.04m²
Step-by-step explanation:
Area of circle: \(\pi\) × r²
Radius = \(\frac{diameter}{2}\)
We can just apply the formula to the question:
Area of circle = 3.14 × (\(\frac{12}{2}\))²
= 3.14 × 36
= 113.04m²
Whats the answer we need help?its been along time since i did this

Answers
Answer:
Try either 33.6 or 24
Step-by-step explanation:
I'm not really sure but it's either one of these two. Good luck
Find the indicated confidence interval. Assume the standard error comes from a bootstrap distribution that is approximately normally distributed.

Answers
The 99% confidence interval for the population proportion p is (0.776, 0.824).
To find the 99% confidence interval for a proportion, we can use the formula:
CI = p^ ± z*(SE)
where p^ is the sample proportion, SE is the standard error, and z is the critical value from the standard normal distribution corresponding to the level of confidence.
For a 99% confidence interval, the critical value z is 2.576.
Substituting the given values into the formula, we have:
CI = 0.80 ± 2.576*(0.03/√200)
Simplifying this expression, we have:
CI = 0.80 ± 0.024
This means that we are 99% confident that the true population proportion falls between 0.776 and 0.824. We can interpret this interval as a range of plausible values for the population proportion, based on the sample data.
To learn more about confidence interval here:
https://brainly.com/question/24131141
#SPJ1
Help math ITS EASY IF U LOKE RATIO
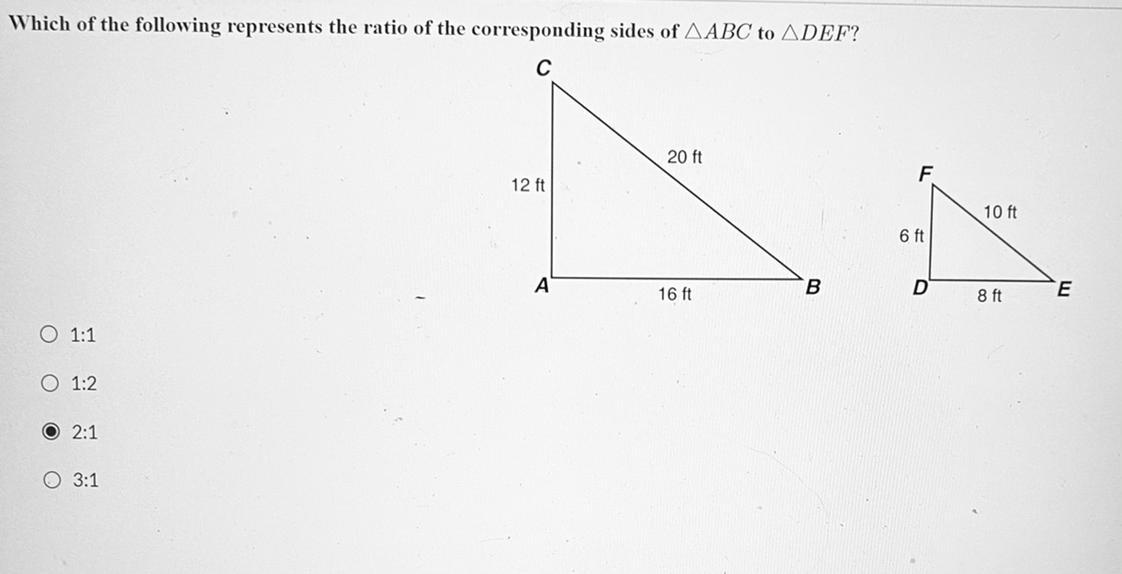
Answers
You have the right one selected.
The answer is 2:1
To find this take each side of ABC and its corresponding side on DEF, then simplify. You can treat ratios just like fractions, so simplify them in the same way.
20:10 becomes 2:1
12:6 becomes 2:1
16:8 becomes 2:1
Select all solutions to M•M•M=729
Answers
The solution to the equation given by M * M * M = 729 is 9
What is an equation?An equation is an expression that shows the relationship between two or more numbers and variables using mathematical operations. An equation can be linear, quadratic, cubic and so on, depending on the degree of the variable.
Given the equation:
M.M.M = 729
This gives:
M * M * M = 729
M³ = 729
Taking the cube root of both sides:
∛M³ = ∛729
M = 9
The solution to the equation is 9
Find out more on equation at: https://brainly.com/question/2972832
#SPJ1
2x + 2 = 5x -8 simplify
Answers
Answer, x = 1
3 1/5x+10 7/9-2 2/5x-10 8/9 (Simplify) I need the answer to this ASAP!
Answers
The simplified form of the expression 3 1/5x + 10 7/9 - 2 2/5x - 10 8/9 is 4/5x - 1/9.
To simplify the expression: 3 1/5x + 10 7/9 - 2 2/5x - 10 8/9, we need to perform the addition and subtraction of the terms.
First, let's focus on the x terms: 3 1/5x - 2 2/5x.
The whole numbers can be treated as fractions with a denominator of 1. Thus, we have:
(3 1/5)x - (2 2/5)x.
To perform the subtraction, we need to find a common denominator for the fractions involved, which is 5.
(3 1/5)x - (2 2/5)x = (16/5)x - (12/5)x.
Now we can subtract the terms:
(16/5)x - (12/5)x = (16 - 12)/5x.
Simplifying further:
4/5x.
Now let's simplify the whole number and fraction terms: 10 7/9 - 10 8/9.
10 - 10 = 0.
The fraction terms can be simplified by finding a common denominator, which is 9:
(7/9) - (8/9) = (-1/9).
Now we can combine all the simplified terms:
3 1/5x + 10 7/9 - 2 2/5x - 10 8/9
= (4/5x) + 0 + (-1/9)
= 4/5x - 1/9.
For similar question on expression.
https://brainly.com/question/29051300
#SPJ11
Which triangle is a reflection of the green triangle
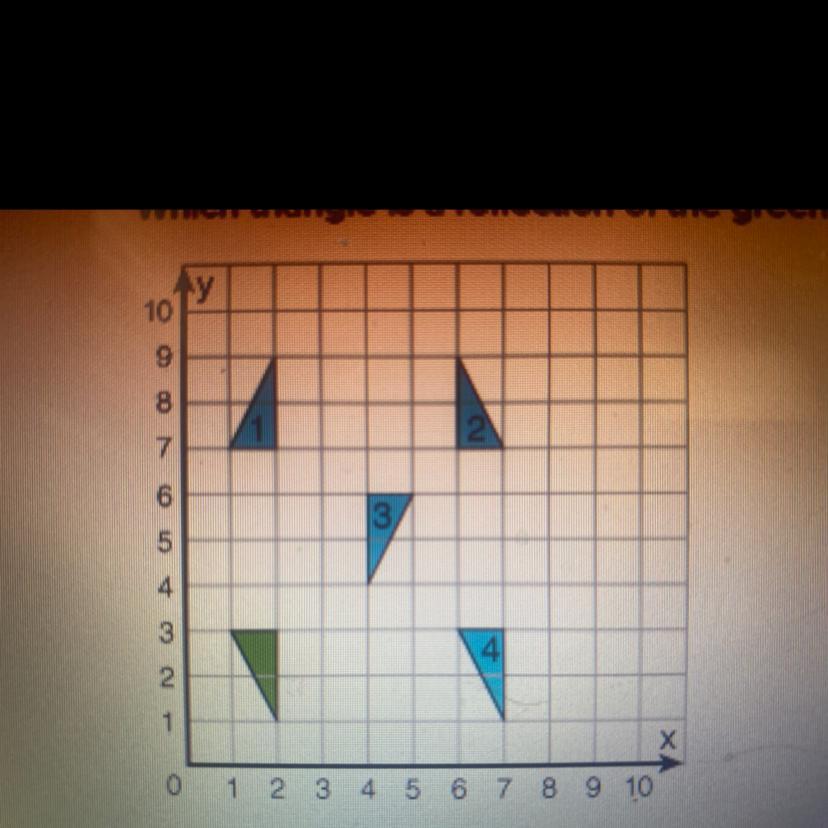
Answers
Tthe triangle that is a reflection of the green triangle is figure 1
Which triangle is a reflection of the green triangleFrom the question, we have the following parameters that can be used in our computation:
The figures
Where, we have:
Figure 1 and the green figure have the opposite orientationFigure 1 and the green figure have the same sizeThis means that the triangle that is a reflection of the green triangle is figure 1
Read more about transformation at
brainly.com/question/4289712
#SPJ1
An inch is exactly 2.54
centimeters.
Write an equation to convert the number of inches x
to the corresponding length in centimeters.
Enter the equation in the box.
Answers
Answer:
x * 2.54 = centimeters
Step-by-step explanation:
The equation to convert inches to centimeters is as follows:
x inches * 2.54 cm/inch = y centimeters
Where x is the number of inches and y is the equivalent length in centimeters.
To elaborate, the conversion factor between inches and centimeters is 2.54 cm/inch, meaning that for every inch there are 2.54 centimeters. To convert from inches to centimeters, we simply multiply the number of inches by the conversion factor. This gives us the equivalent length in centimeters.
a bike requires 2 hours of assembly and a swing 3 hours. a maximum of 250 hours of assembly time are available. the profit is $50 on a bike and $20 on a swing. if no more than 100 items can be produced how many of each should be assembled for maximum profit?
Answers
The assemble for maximum profit is mathematically given as
For maximum profit, 100 tire and 0 strings must be assembled.
How many of each should be assembled for maximum profit?Generally, the equation for the max number is mathematically given as
Mx=50x+20y
Therefore
2x+3y \leq 250
x1+x\leq=100
Therefore
with cordinates of
(0,0)
(100,0)
(50,50)
(0,250/3)
In conclusion, The mx of 50x+20y is (100,0)
Hence, for maximum profit 100 tire and 0 strings must be assembled.
Read more about Arithmetic
https://brainly.com/question/22568180
A large tank of fish from a hatchery is being delivered to a lake. The hatchery claims that the mean length of fish in the tank is 15 inches, and the standard deviation is 6 inches. A random sample of 46 fish is taken from the tank. Let x be the mean sample length of these fish. What is the probability that x is within 0.5 inch of the claimed population mean?
Answers
Answer:
0.4246
Step-by-step explanation:
Given data:
mean ( u ) = 15 inches
std ( б )= 6 inches
sample size( n ) = 46
ux = mean sample length
Determine the probability that x within 0.5 inch of the claimed population mean
ux = u = 15
бx = б/ √ 46 = 6 /√ 46 = 6 / 6.78 = 0.88
Hence the P( 14.5 < x < 15.5 )
= P ( [14.5 - 15 / 0.88 ] < z < (15.5 - 15) / 0.88) )
= P ( -0.5682 < z < 0.5682 )
= P ( z < 0.5682 ) - P ( z < -0.5682 )
= 0.7123 - 0.2877 ( from Z table )
= 0.4246
-3 (2x - 1) ≤ x - 18
Answers
Answer:
x ≥ 3
Step-by-step explanation:
- 3(2x - 1) ≤ x - 18 ← distribute parenthesis on left side
- 6x + 3 ≤ x - 18 ( subtract x from both sides )
- 7x + 3 ≤ - 18 ( subtract 3 from both sides )
- 7x ≤ - 21
divide both sides by - 7, reversing the symbol as a result of dividing by a negative quantity.
x ≥ 3
Which line segments have a positive slope PLS FAST!! THANKS!!

Answers
a-b
it’s the ones where it’s pointing up to the right!
solve for x, using the secant lines. 53° 189°
Answers
The measure of the arc opposite to 189° is 136°.
What are secant lines?Secant lines are lines that intersect a curve at two distinct points. They are used to approximate the slope of the curve by measuring the change in the y-coordinate of the two points divided by the change in the x-coordinate of the two points.
The measure of the angle opposite to 189° is x.
To solve for x, we can use the property of alternate interior angles.
The sides of the two secant lines that intersect outside the circle form alternate interior angles whose measures are equal.
Therefore, the two angles formed by the secant lines inside the circle must have the same measure.
Therefore, 53° + x° = 189°.
Subtracting 53° from both sides, we get x° = 136°.
For more questions related to arc
https://brainly.com/question/29860198
#SPJ1

Do bathroom scales tend to underestimate a person’s true weight? A 150 lb test weight was placed on each of 50 bathroom scales. The readings on 28 of the scales were too light, and the readings on the other 22 were too heavy. Can you conclude that more than half of bathroom scales underestimate weight? Find the P-value and state a conclusion. The P-value is . Round the answer to four decimal places. We cannot conclude that more than half of bathroom scales underestimate weight.
Answers
Answer:
the P-value = 0.1977
conclusion: Since the p-value is not small;
we reject null hypothesis
hence, the data does not support claim that more than half of the bathroom scales underestimate weight.
Step-by-step explanation:
Given the data in the question;
let X represent the number of successes ( the weight is underestimated ) in n independent Bernoulli trials, each with the success probability p
X - Bin( n,p).
so
Null hypothesis H₀ : p ≤ 0.5
Alternative hypothesis Hₐ : p > 0.5
now, compute np₀ and n( 1 - p₀ )
np₀ = (50)(0.5)
np₀ = 25
n( 1-p₀ ) = (50)(1 - 0.5)
n( 1-p₀ ) = 25
we can see that both values are greater than 10.
∴ the sample proportion is approximately normally distributed;
P" - N ( p₀, \(\frac{p_{0}(1-p_0)}{n}\) )
sample proportion p" = 28/50 = 0.56
standard deviation will be;
σ\(_{p"\) = √( p₀(1-p₀) / n )
σ\(_{p"\) = √( 0.5(1-0.5) / 50 )
σ\(_{p"\) = √( 0.25 / 50 )
σ\(_{p"\) = 0.07071
Next is the Z-score
z = p" - p₀ / σ\(_{p"\)
z = 0.56-0.5 / 0.07071
z = 0.06 / 0.07071
z = 0.85
from table,
the probability that a standard normal random variable takes on a value greater than 0.85 is approximately 0.1977
Therefore, the P-value = 0.1977
conclusion: Since the p-value is not small;
we reject null hypothesis
hence, the data does not support claim that more than half of the bathroom scales underestimate weight.
p = 0.197663
graph y+2=2(x+3). What is the x intercept?
x intercept :
Answers
The given equation is y + 2 = 2(x + 3) and the x-intercept is -2.
To find the x-intercept of the equation y + 2 = 2(x + 3), we need to set y equal to zero and solve for x. The x-intercept occurs when the value of y is zero, meaning the graph crosses the x-axis.
Let's substitute y with 0 in the equation:
0 + 2 = 2(x + 3)
Simplifying the equation:
2 = 2(x + 3)
Dividing both sides by 2:
1 = x + 3
Subtracting 3 from both sides:
-2 = x
The x-intercept is -2. This means that the graph of the equation y + 2 = 2(x + 3) crosses the x-axis at the point (-2, 0).
The x-intercept represents the value of x where the graph intersects or crosses the x-axis. In this case, it occurs when x is equal to -2, and the y-value is zero.
For more such questions on x-intercept
https://brainly.com/question/17932786
#SPJ8
33 + 2(5+8) - (6-2)2 =
Answers
Answer:
51
Step-by-step explanation:
33+2(5+8)-(6-2)2
open the brackets FIRST
2(5+8) = 26
33+26-(6-2)2
33+18
= 51
Evaluate the expression when m=-4
m^2-5m+2
Answers
factor -4 into the equation and simplify
In ΔFGH, the measure of ∠H=90°, the measure of ∠F=18°, and GH = 9.4 feet. Find the length of HF to the nearest tenth of a foot.
Answers
Answer:
28.9
Step-by-step explanation:
tan (18) = 9.4/x times x
x times tan(18) = 9.4 / tan(18)
tan(18)
crosses out.
On a calculator do 9.4/
tan(18).
x = 28.9
The mean height of the students in a class is 152 cm. The mean height of boys is 158 cmwith a standard deviation of 5 cm. And the mean height of girls is 148 cm with a standarddeviation of 4 cm. Find the percentage of boys in the class and also the S.D of heights of allthe students in the class?
Answers
The percentage of boys in the class and also the standard deviation of heights of all the students in the class are 78% and 9 cm respectively
How to find the percentage of boys in the class?Percentage of the boys in the class deals with a ratio of the boys to the number of students in the class
The given parameters that will help us to get the percentage are
Mean height of the class = 152 cm
Mean height of the boys = 158 cm
The standard deviation of the boys = 5 cm
Mean height of the girls = 148 cm
Standard deviation of the girls = 4 cm
(1) The percentage of boys in the class is
Total mean height = 158 +148 = 306
Percentage = 158/306 * 100 = 51.6%
Then the percentages 51.6/100 * 152 = 78%
(2) The total standard deviation of all the students
Boys + girls = 5+4 = 9 cm
Therefore, the percentage of boys in the class and also the standard deviation of heights of all the students in the class are 78 students and 9 cm respectively
Lean more about percentages on https://brainly.com/question/29306119
#SPJ1
PLEASE HELP AS SOON AS POSSIBLE

Answers
A) The slope between the points (0, -4) and (2, -1) is 1.5.
b) the slope between the points (0, -4) and (4, -1) is 0.75.
c) The slope of 0.75 indicates that for every 1 unit increase in x, there is a 0.75 unit increase in y.
Part A: To calculate the slope between two points, we use the formula:
slope = (change in y) / (change in x)
Let's choose the points (0, -4) and (2, -1).
Change in y = -1 - (-4) = 3
Change in x = 2 - 0 = 2
slope = 3 / 2 = 1.5
Therefore, the slope between the points (0, -4) and (2, -1) is 1.5.
Part B: Let's choose different points from the table, such as (0, -4) and (4, -1).
Change in y = -1 - (-4) = 3
Change in x = 4 - 0 = 4
slope = 3 / 4 = 0.75
Thus, the slope between the points (0, -4) and (4, -1) is 0.75.
Part C: The slopes from parts A and B provide information about the relationship between the points.
In part A, where the slope was calculated as 1.5, we can see that as the x-values increase by 2 units, the y-values increase by 3 units. This suggests a positive relationship between the points, meaning that as x increases, y also increases. The slope of 1.5 indicates that for every 1 unit increase in x, there is a 1.5 unit increase in y.
In part B, where the slope was calculated as 0.75, we observe a similar positive relationship between the points. As the x-values increase by 4 units, the y-values increase by 3 units. The slope of 0.75 indicates that for every 1 unit increase in x, there is a 0.75 unit increase in y.
Overall, the positive slopes in both parts A and B suggest that the points on the table exhibit a positive linear relationship. This means that as x increases, y also increases, and the rate of change is consistent based on the slope.
For more such questions on slope visit:
https://brainly.com/question/16949303
#SPJ8
Here are the prices of some items a nd the amount of sales tax charged on each in Nevada. What is the sales tax rate in Nevada?

Answers
The sale tax rate is equal to the ratio of change in sales tax to the corresponding chane in cost of item.
Determine the sales tax rate.
\(\begin{gathered} s=\frac{2.30-0.46}{50-10} \\ =\frac{1.84}{40} \\ =0.046 \end{gathered}\)So sales tax rate is 0.046 or 4.6% of cost of item.
