how many ways can you place the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 in the ten blank spaces in such an order that the indicated division will have a remainder of 1981? 3168 71 543 2 985 2 356 8 7 836 7 39997
Answers
There are 24 different ways to arrange the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 in the given order so that the indicated division will have a remainder of 1981. Each digit is used exactly once.
To determine the number of ways to arrange the digits in the given scenario, we can break down the problem into several steps
Count the number of ways to arrange the non-zero digits.
Since the division involves a quotient of 3168, we can place the digits 3, 1, 6, and 8 in the corresponding positions. There are 4! = 4 x 3 x 2 x 1 = 24 ways to arrange these digits.
There are 6 remaining digits (0, 2, 4, 5, 7, and 9) that need to be placed in the remaining positions. For each of these digits, there is only one possible position where it can be placed without affecting the remainder. Therefore, the number of ways to place these digits is 1 for each digit.
The total number of arrangements is the product of the number of arrangements from Step 1 and the number of arrangements from Step 2. Since each step is independent, we can multiply the results. So, the total number of arrangements is 24 x 1⁶ = 24.
Therefore, there are 24 different ways to place the digits such that the indicated division will have a remainder of 1981.
To know more about division:
https://brainly.com/question/2273245
#SPJ4
--The given question is incomplete, the complete question is given below " How many ways can you place the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 in the ten blank spaces in such an order that the indicated division will have a remainder of 1981?71_543_2_985_2_356_8_7_836_7_39997/ 3168 . Be sure to justify any claims you make. Note that each digit must be used exactly once and you are welcome to use a calculator."--
Related Questions
Plzzzzzz help, tysm if you do

Answers
Answer:
I think it C
Step-by-step explanation:
Let's solve your equation step-by-step.
(k−4)2=9
Step 1: Simplify both sides of the equation.
k2−8k+16=9
Step 2: Subtract 9 from both sides.
k2−8k+16−9=9−9
k2−8k+7=0
Step 3: Factor left side of equation.
(k−1)(k−7)=0
Step 4: Set factors equal to 0.
k−1=0 or k−7=0
k=1 or k=7
Answer:
k=1 or k=7
Hope this helps! :)
Square both sides:
K -4 = sqrt(9)
With the answer being a square root rewrite with a postive and negative value:
K-4 = sqrt(9)
And
K-4 = -sqrt(9)
Simplify each:
K-4 = 3
And
K-4 = -3
Solving for k in each one you get answer
C. {1,7}
determine whether an observational or experimental study is appropriate to address the following statement. a gift shop owner wants to study the average age of his clientele.
Answers
To determine the average age of a gift shop owner's clientele, an observational study is the appropriate study design to use. It involves collecting data by observing people in their usual setting, without manipulating variables.
To address the following statement, "a gift shop owner wants to study the average age of his clientele", an observational study is appropriate.
What is an observational study?
An observational study is a non-experimental research design that involves direct observation of the study subjects. It does not involve intervention from the researcher. Instead, researchers monitor subjects in their usual setting, gathering data without direct involvement.Experimental study:An experimental study, on the other hand, is a study design that involves researchers manipulating one variable to see how it affects another variable. The researcher manipulates the variable by providing a treatment or intervention.
What type of study is appropriate to determine the average age of a gift shop owner's clientele?
To determine the average age of a gift shop owner's clientele, an observational study is the appropriate study design to use. It involves collecting data by observing people in their usual setting, without manipulating variables.
To know more about observing people :
https://brainly.com/question/29425094
#SPJ11
PLEASE HELP!!! WILL GIVE BRAINLIEST!!!
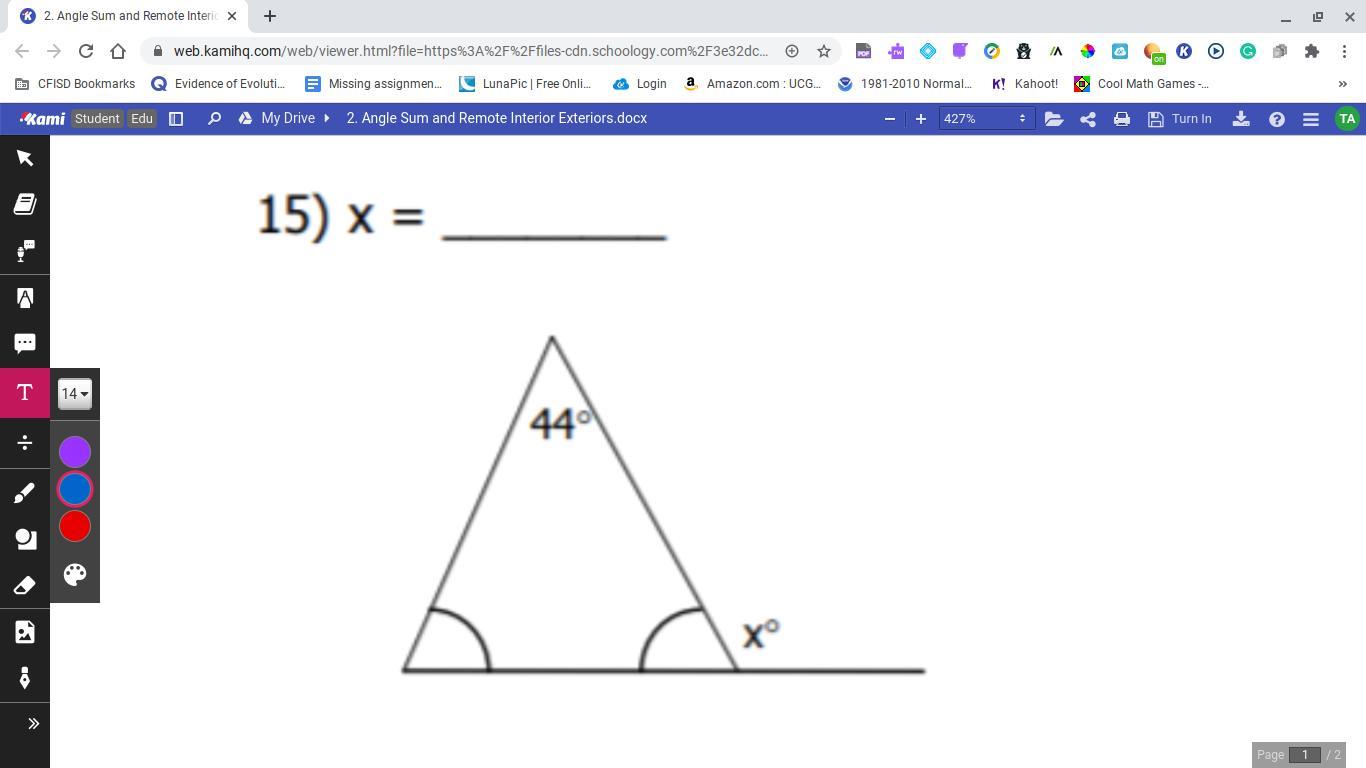
Answers
Answer:
112 degrees
Step-by-step explanation:
The other 2 interior angles are (180-44)/2 which is 68.
180 (straight line) -68 = 112
If A and B are independent events with P(A) = 0.35 and P(B) = 0.20, then, P(A U B) = _____.
SHOW ALL WORK
Answers
Answer:0.48
Step-by-step equation:
Find the area of the figure in the picture below

Answers
Answer:
183 cm^2
Step-by-step explanation:
the line through the shape line means that they are equivalent meaning the side that is empty not making the trapezoid would be 9 as well. moving the 3 over since it is right next to it. 3 times 9 equal 27cm^2. later we are gonna use this number to subtract
then make a different equation with 3+9 to the the full height of the trapezoid pretending it is not missing anything. 3+9=12
then use the formula to find the area of the trapezoid which is 1/2(h)(b1+b2)
add the 2 bases together to get 35. how did i get 35. 12+14=26 yes then include the 9 to get 35.
1/2(height=12)(35)=6(35)=210cm^2
then you used 210-27 to get 183cm^2
Determine if the following angles could be the angles in a triangle.
V = 49°, W = 56°, X = 75°
If VWX is a triangle, which side of the triangle is the longest?
Answers
Answer:V = 49°, W = 56°, X = 75°
Step-by-step explanation:V = 49°, W = 56°, X = 75°
Math, Please Help 15 points

Answers
Answer:
the second one
Step-by-step explanation:
Let us suppose a population size of 67 million, and innovation parameter of 0.005 and imitation parameter of 0.84 for Color TV. Estimate how many new users would be added during time period 7.
Answers
To estimate the number of new users that would be added during time period 7, we can use the Bass diffusion model, which is commonly used to model the adoption of new products or technologies.
The Bass diffusion model is given by the formula:
\(\[N(t) = \frac{{p \cdot q}}{{q + (p/q) \cdot e^{-((p+q) \cdot t)}}}\]\)
where:
- N(t) represents the cumulative number of adopters at time \(t\).
- p is the innovation parameter, representing the coefficient of innovation.
- q is the imitation parameter, representing the coefficient of imitation.
- e is the base of the natural logarithm.
Given a population size of 67 million, an innovation parameter of 0.005, and an imitation parameter of 0.84 for Color TV, we can substitute these values into the Bass diffusion model and calculate the number of new users added during time period 7.
\(\[N(7) - N(6) = \frac{{p \cdot q}}{{q + (p/q) \cdot e^{-((p+q) \cdot 7)}}} - \frac{{p \cdot q}}{{q + (p/q) \cdot e^{-((p+q) \cdot 6)}}}\]\)
Substituting the given values into the equation:
\(\[N(7) - N(6) = \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 7)}}} - \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 6)}}}\]\)
Evaluating the expression will give us the estimated number of new users added during time period 7.
In LaTeX, the solution can be represented as:
\(\[N(7) - N(6) = \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 7)}}} - \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 6)}}}\]\)
After evaluating this expression, you will obtain the estimated number of new users added during time period 7.
To know more about LaTex visit-
brainly.com/question/18882901
#SPJ11
a box with an open top has vertical sides, a square bottom, and a volume of 32 cubic meters. if the box has the least possible surface area, find its dimensions.
Answers
If the box has the least possible surface area, then the dimension of box is a square base of 2 meters by 2 meters, and a height of 4 meters.
Let's call the side length of the square bottom "x" and the height of the box "h". Since the box has an open top, we don't need to worry about the surface area of the top. Therefore, we only need to consider the surface area of the bottom and sides.
The volume of the box is given as 32 cubic meters, so we can set up an equation:
Volume = x²h = 32
We want to minimize the surface area, which consists of the area of the bottom and the four sides. The area of the bottom is x², and the area of each side is xh. So, the total surface area is:
Surface Area = x² + 4xh
We can use the equation for the volume to solve for h in terms of x:
h = 32/x²
Substituting this expression for h into the equation for surface area gives:
Surface Area = x² + 4x(32/x²) = x² + 128/x
To minimize the surface area, we can take the derivative of this expression with respect to x and set it equal to zero:
d(Surface Area)/dx = 2x - 128/x² = 0
Solving for x, we get:
2x = 128/x²
x⁵ = 64
x = 2 meters
Substituting this value of x back into the equation for h gives:
h = 32/x² = 4 meters
Therefore, the dimensions of the box with the least possible surface area are:
Length = Width = x = 2 meters
Height = h = 4 meters
Learn more about surface area here
brainly.com/question/29617764
#SPJ4
Find the sum of the first 10 terms of an arithmetic sequence with an eighth term of 8.2 and a common difference of 0.4.
Answers
The sum of first 10 terms is 72.
Concept: Arithmetic Progression (AP) is a sequence of numbers in order, in which the difference between any two consecutive numbers is a constant value. It is also called Arithmetic Sequence.
Arithmetic Progression Formula
= an - a. nth term of an AP: an = a + (n - 1)d. Sum of n terms of an AP: Sn = n/2(2a+(n-1)d) = n/2(a + l), where l is the last term of the arithmetic progression.
Given:
Eighth term is 8.2
Common difference is 0.4
a(8) = 8.2
a + 7d = 8.2
Since d = 0.4
a + 7(0.4) =8.2
a = 5.4
Sum of 10 terms = n/2 (2a+(n-1)d)
= 5(2*5.4 + 9*0.4)
=5(10.8 +3.6)
Sum=72
For more information about arithmetic progression, visit
https://brainly.com/question/6561461
#SPJ4
Solve the following:-
\(\sf 2 +a =7\)
Answers
Answer:
\( \sf \: a = 5\)
Step-by-step explanation:
Now we have to,
→ find the required value of a.
The equation is,
→ 2 + a = 7
Then the value of a will be,
→ 2 + a = 7
→ a + 2 = 7
→ a = 7 - 2
→ [ a = 5 ]
Hence, the value of a is 5.
use the definition to find the first five nonzero terms of the taylor series generated by the function f(x)=7tan−1x π24 about the point a=1.
Answers
The first five nonzero terms of the Taylor series for\(f(x) = \frac{7 \cdot \arctan(x)}{\frac{\pi}{24}}\) about the point a = 1 are \(7 + \frac{84}{\pi}(x - 1) - \frac{84}{\pi}(x - 1)^2 + 0 + 0\)
The first five nonzero terms of the Taylor series generated by the function \(f(x) = \frac{7 \cdot \arctan(x)}{\frac{\pi}{24}}\) about the point a = 1 can be found using the definition of the Taylor series.
The general form of the Taylor series expansion is given by:
\(f(x) = f(a) + f'(a)(x - a) + (f''(a)(x - a)^2)/2! + (f'''(a)(x - a)^3)/3! + (f''''(a)(x - a)^4)/4! + ...\)
To find the first five nonzero terms, we need to evaluate the function f(x) and its derivatives up to the fourth derivative at the point a = 1.
First, let's find the function and its derivatives:
\(f(x) = \frac{7 \cdot \arctan(x)}{\frac{\pi}{24}}\)
\(f'(x) = \frac{7}{\frac{\pi}{24} \cdot (1 + x^2)}\)
\(f''(x) = \frac{-7 \cdot (2x)}{\frac{\pi}{24} \cdot (1 + x^2)^2}\)
\(f'''(x) = \frac{-7 \cdot (2 \cdot (1 + x^2) - 4x^2)}{\frac{\pi}{24} \cdot (1 + x^2)^3}\)
\(f''''(x) = \frac{-7 \cdot (8x - 12x^3)}{\frac{\pi}{24} \cdot (1 + x^2)^4}\)
Now, let's substitute the value of a = 1 into these expressions and simplify:
\(f(1) = \frac{7 \cdot \arctan(1)}{\frac{\pi}{24}} = 7\)
\(f'(1) = \frac{7}{\frac{\pi}{24} \cdot (1 + 1^2)} = \frac{84}{\pi}\)
\(f''(1) = \frac{-7 \cdot (2 \cdot 1)}{\frac{\pi}{24} \cdot (1 + 1^2)^2} = \frac{-84}{\pi}\)
\(f'''(1) = \frac{-7 \cdot (2 \cdot (1 + 1^2) - 4 \cdot 1^2)}{\frac{\pi}{24} \cdot (1 + 1^2)^3} = 0\)
\(f''''(1) = \frac{-7 \cdot (8 \cdot 1 - 12 \cdot 1^3)}{\frac{\pi}{24} \cdot (1 + 1^2)^4} = 0\)
Now we can write the first five nonzero terms of the Taylor series:
\(f(x) = 7 + \frac{84}{\pi}(x - 1) - \frac{84}{\pi}(x - 1)^2 + \dots\)
These terms provide an approximation of the function f(x) near the point a = 1, with increasing accuracy as more terms are added to the series.
Learn more about Taylor series here:
https://brainly.com/question/31140778
#SPJ11
Use the function f(x) to answer the questions:
f(x) = 2x2 − 3x − 5
Part A: What are the x-intercepts of the graph of f(x)? Show your work. (2 points)
Part B: Is the vertex of the graph of f(x) going to be a maximum or a minimum? What are the coordinates of the vertex? Justify your answers and show your work. (3 points)
Part C: What are the steps you would use to graph f(x)? Justify that you can use the answers obtained in Part A and Part B to draw the graph. (5 points)
Answers
a) The x-intercepts of the graph of f(x) are x = 2.5 and x = -1.
b) The coordinates of the vertex are (0.75, -5.125).
c) By using the x-intercepts and vertex obtained in Parts A and B, we can accurately depict the shape and positioning of the parabolic graph of f(x).
Part A: To find the x-intercepts of the graph of f(x), we set f(x) equal to zero and solve for x.
Setting f(x) = 0:
\(2x^2 - 3x - 5 = 0\)
To solve this quadratic equation, we can either factor it or use the quadratic formula. In this case, factoring is not straightforward, so let's use the quadratic formula:
x = (-b ± √(b^2 - 4ac)) / (2a)
For our equation, a = 2, b = -3, and c = -5. Substituting these values into the quadratic formula:
x = (-(-3) ± √((-3)^2 - 4(2)(-5))) / (2(2))
x = (3 ± √(9 + 40)) / 4
x = (3 ± √49) / 4
x = (3 ± 7) / 4
This gives us two possible solutions:
x1 = (3 + 7) / 4 = 10/4 = 2.5
x2 = (3 - 7) / 4 = -4/4 = -1
Therefore, the x-intercepts of the graph of f(x) are x = 2.5 and x = -1.
Part B: To determine whether the vertex of the graph of f(x) is a maximum or minimum, we need to consider the coefficient of the x^2 term in the function f(x). In this case, the coefficient is positive (2), which means the parabola opens upward and the vertex represents a minimum point.
To find the coordinates of the vertex, we can use the formula x = -b / (2a). In our equation, a = 2 and b = -3:
x = -(-3) / (2(2))
x = 3 / 4
x = 0.75
To find the corresponding y-coordinate, we substitute x = 0.75 into the function f(x):
f(0.75) = 2(0.75)^2 - 3(0.75) - 5
f(0.75) = 2(0.5625) - 2.25 - 5
f(0.75) = 1.125 - 2.25 - 5
f(0.75) = -5.125
Therefore, the coordinates of the vertex are (0.75, -5.125).
Part C: To graph the function f(x), we can follow these steps:
Plot the x-intercepts obtained in Part A: (2.5, 0) and (-1, 0).
Plot the vertex obtained in Part B: (0.75, -5.125).
Determine if the parabola opens upward (as determined in Part B) and draw a smooth curve passing through the points.
Extend the curve to the left and right of the vertex, ensuring symmetry.
Label the axes and any other relevant points or features.
By using the x-intercepts and vertex obtained in Parts A and B, we can accurately depict the shape and positioning of the parabolic graph of f(x).
The x-intercepts help determine where the graph intersects the x-axis, and the vertex helps establish the lowest point (minimum) of the parabola. The resulting graph should show a U-shaped curve opening upward with the vertex at (0.75, -5.125) and the x-intercepts at (2.5, 0) and (-1, 0).
For more such questions on intercepts visit:
https://brainly.com/question/24212383
#SPJ8
Find the distance between (3,5)
and (7,-3). **(round to the nearest tenth.)
Answers
Answer:
The answer is
8.9 unitsStep-by-step explanation:
The distance between two points can be found by using the formula
\(d = \sqrt{( {x1 - x2})^{2} + ({y1 - y2})^{2} } \\ \)where
(x1 , y1) and (x2 , y2) are the points
From the question the points are
(3,5)and (7,-3)
The distance between them is
\(d = \sqrt{( {3 - 7})^{2} + ({5 + 3})^{2} } \\ = \sqrt{ ({ - 4})^{2} + {8}^{2} } \\ = \sqrt{16 + 64} \\ = \sqrt{80} \\ =4 \sqrt{5} \\ \: \: \: \: = 8.9442\)We have the final answer as
8.9 units to the nearest tenthHope this helps you
Step-by-step explanation:
√(7-3)^2+(-8)^2
√16+64
√80
8.94
Given sinA = 8\sqrt{73} and that angle
A is in Quadrant I, find the exact value of
tan tanA in simplest radical form using a rational denominator.
Answers
The value of \($\tan A$\) in its simplest radical form is \(8/3$.\)
What is radical form?
Radical form refers to a mathematical expression that includes radicals, which are symbols that indicate a root of a number.
To find the value of \($\tan A$\), we first need to determine the value of \(\cos A$.\)
Using the Pythagorean identity, we have:
\($$\sin^2 A + \cos^2 A = 1$$\)
Substituting the given value of \($\sin A$\), we get:
\($$\left(\frac{8}{\sqrt{73}}\right)^2 + \cos^2 A = 1$$\)
Simplifying the left-hand side, we get:
\($\frac{64}{73} + \cos^2 A = 1$$$$\cos^2 A = \frac{9}{73}$$$$\cos A = \frac{3}{\sqrt{73}}$$\)
Since angle \($A$\) is in the first quadrant, both \($\sin A$\) and \($\cos A$\) are positive.
Therefore, we have:
\($$\tan A = \frac{\sin A}{\cos A} = \frac{8/\sqrt{73}}{3/\sqrt{73}} = \frac{8}{3}$$\)
So the value of \($\tan A$\) in its simplest form is \(8/3$.\)
To learn more about radical form visit:
https://brainly.com/question/30260621
#SPJ1
if T=1/4 A^3B make A the subject of formula
Answers
Step-by-step explanation:
A³B = 4T
A³=4T/B
A = cbrt 4T/B. or
³√(4T/B)
hope this helps.
Which of these planes is NOT in the {100} family for a tetragonal crystal? (A tetragonal unit cell drawn to proportion is included below for reference.)(A) (010)(B) (001)(C) (110)(D) Both B & C(E) All of these planes are in the {100} family.
Answers
The answer is (001). This is because B (001) has h and k as non-zero integers, which does not match the criteria for the {100} family.
The question is asking which of the planes (A), (B), (C), and (D) is not part of the {100} family for a tetragonal crystal.A tetragonal crystal is a three-dimensional structure made up of four faces that intersect at right angles, forming a unit cell. Each face of the unit cell is defined by a Miller index. A Miller index is a set of three integers written in the form {hkl}, which describes the orientation of the face relative to the crystal lattice. In a tetragonal crystal, the {100} family is the set of faces described by {hkl} such that h = k = 0 and l ≠ 0.
Therefore, A (010), C (110), and E (all of these planes are in the {100} family) are all part of the {100} family for a tetragonal crystal, while B (001) is not. because B (001) has h and k as non-zero integers, which does not match the criteria for the {100} family. In conclusion, the correct answer to the question is B (001).
To know more about tetragonal crystal system, click here:
https://brainly.com/question/29645362
#SPJ11
Express the rule in function notation. (For example, the nule "square, then subtroct 5 " is expressed as the function f(x)=x2−5. ) Square, then add 3 . f(x)= [-/1 Points] SCOLALG7 2.1.010. Express the rule in function notation. (For example, the rule "square, then subtract 5 " is expressed as the function f(x)=x2−5.) Add 3, take the square root, then divide by 6 . f(x)= [-/1 Points] SCOLALG7 2.1.014. Express the function (or rule) in words. k(x)=7x2−8 Subtract 8 , then divide by 7 , then square. Square, then divide by 7 , then subtract 8 . Square, then subtract 8 , then divide by 7 Multiply by 7 , then add 8 , then take the square root. Divide by 7 , then subtract 8 , then square.
Answers
5. The rule in function notation is f(x) = x² + 3
6. The function k(x) = 7x² − 8 in words is multiply by 7, then subtract 8.
Given statement:
5. The rule in function notation.
(For example, the rule "square, then subtract 5 " is expressed as the function f(x) = x² − 5. )
The rule in function notation.
Square, then add 3 .
f(x) = x² + 3
Express the rule in function notation.
(For example, the rule "square, then subtract 5 " is expressed as the function f(x) = x² − 5.
Add 3, take the square root, then divide by 6
The rule in function notation.
f(x) = √(x + 3)/6
6. Express the function (or rule) in words. k(x) = 7x² − 8
Square, then subtract 8 , then divide by 7Therefore, the function k(x) = 7x² − 8 in words is Multiply by 7, then subtract 8.
Learn more about function here:
https://brainly.com/question/30607679
#SPJ4
4-2x=10
just fing the answer :)))
Answers
Answer:
x = -3
Step-by-step explanation:
4 - 2x = 10
-2x = 6
x = -3
What is the midpoint of the line segment with endpoints (-1,7) and (3, -3)?
A. (1, 2)
B. (1,4)
C. (2, 4)
D. (2, 2)
Answers
Fill out the table, reporting all the sample means and standard deviations.PreAlg: 1.32 (Mean), 0.96 (SD)ElemAlg: 3.18 (Mean), 0.75 (SD)InterAlg: 4.42 (Mean), 2.78 (SD)Finish the list of all three possible comparisons.1. PreAlg compared to ElemAlg2. PreAlg compared to InterAlg3. ElemAlg compared to InterAlgFind the corrected value for the significance level by dividing 0.05 by the number of comparisons.The corrected significance level is 0.0167.We have assumed that the conditions for two-sample t-tests are met. For all tests, the null hypothesis is that the two population means are the same, and the alternative hypothesis is that the two population means are different. Complete the table below. For a significant difference, the p-value must be less than the Bonferroni-corrected value for the significance level.PreAlg and ElemAlg: 5.08 (t-value), 0.000 (p-value), different (conclusion)PreAlg and InterAlg: 3.64 (t-value), 0.003 (p-value), different (conclusion)ElemAlg and InterAlg: 1.49 (t-value), 0.163 (p-value), not different (conclusion)Write a clear conclusion based on what you found. Which groups have sample means that are significantly different, and how do they differ?Ans: PreAlg students spend less time doing homework than the others.
Answers
PreAlg students spend significantly less time doing homework compared to ElemAlg and InterAlg students, while there is no significant difference in homework time between ElemAlg and InterAlg students.
What is significantly ?
Significantly" is the keyword that indicates a notable or meaningful difference or result in the context of statistical analysis. It is often used to describe findings that have a high level of confidence and statistical significance, indicating that the observed difference or relationship is unlikely to have occurred by chance.
Based on the given data and statistical analysis, the conclusion is as follows:
PreAlg compared to ElemAlg:
The sample mean for PreAlg (1.32) is significantly different from the sample mean for ElemAlg (3.18) with a t-value of 5.08 and a p-value of 0.000. Therefore, we can conclude that PreAlg students spend significantly less time doing homework compared to ElemAlg students.
PreAlg compared to InterAlg:
The sample mean for PreAlg (1.32) is significantly different from the sample mean for InterAlg (4.42) with a t-value of 3.64 and a p-value of 0.003. Hence, we can conclude that PreAlg students spend significantly less time doing homework compared to InterAlg students.
ElemAlg compared to InterAlg:
The sample mean for ElemAlg (3.18) is not significantly different from the sample mean for InterAlg (4.42) with a t-value of 1.49 and a p-value of 0.163. Therefore, we fail to reject the null hypothesis, and we cannot conclude a significant difference in homework time between ElemAlg and InterAlg students.
In summary, the PreAlg students have significantly lower homework time compared to both ElemAlg and InterAlg students. However, there is no significant difference in homework time between ElemAlg and InterAlg students.
To know more about significantly visit:
https://brainly.com/question/24621985
#SPJ4
Which of these expressions demonstrates the identity property? 25(0) = 25 25(1) = 25 25 + 0 = 25 25 + 1 = 25
Answers
The expressions which demonstrates the identity property of multiplication is; 25(1) = 25 option B
Identity Property
Identity Property of Multiplication states that any number multiplied by 1 does not change, that is, it is constant or remains the same
Check all options
25(0) = 25
0 = 25
Not true
25(1) = 25
25 = 25
True (identity property of multiplication holds)
25 + 0 = 25
25 = 25
True (Not identity property of multiplication)
25 + 1 = 25
26 = 25
Not true
Learn more about identity property:
https://brainly.com/question/2364391
#SPJ1
Craig just purchased a new car. He financed $45000 and must pay it back over 5 years with 11% interest.
How much will he have paid for his car, including interest, after 5 years?
Answers
Answer:
$49950
Step-by-step explanation:
you do 45000(.11) which equals 4950. and then you add 4,950 to 45000, and then you get your answer
I need a little help...

Answers
Answer:
10 pencils
Step-by-step explanation:
16-x = 36-3x
16-36 = -3x+
thus x = 10
Need help please (50 points at stake) The farmer's plan is to fence in a strip of his land for herding his cattle so that it forms a square. He decides to place the four corner post of the fence and then place 15 posts between each corner post so that there is a distance of 8 and 1/8 feet between each post around the entire fenced-in area. Calculate the area and perimeter of this fenced-in area.
Answers
15 posts plus 2 corner posts = 17 posts. With 17 posts there would be 16 spaces each side.
The space between each is 8 1/8 feet.
The length of one side is the number of spaces multiplied by the distance between each space.
Total length of one side = 16 spaces x 8 1/8 feet = 130 feet
Area = length x width.
It’s a square shape so area = 130 x 130 = 16,900 square feet
Perimeter is the distance around the square, this would be the length of one side x the number of sides.
Perimeter = 130 x 4 = 520 feet
give the geometrical representation of y=3 as an equation in
a) one variable
b) in two variables.
Answers
The geometrical representation of y=3 as an equation in
a) one variable is y = 3
b) in two variables is 0x + y = 3.
How to illustrate the information?Based on the information illustrated, the geometrical representation of y=3 as an equation in one variable is y = 3.
Also, the geometrical representation of y=3 as an equation in two variables is:
y = 3.
0x + y = 3
0x + y - 3 = 0
Therefore, y = 3.
Learn more about equations on:
brainly.com/question/18831322
#SPJ1
Hellllpppppppp me plsssss

Answers
Answer:
x is 38°
Step-by-step explanation:
180° - 142° = 38°.
Hope this will help❤️
Philip's bill for lunch at a restaurant was $7. He left a 15% tip. What was the amount of the
tip?
Answers
Answer:$1.05
Step-by-step explanation:
Step-by-step explanation:
:
=$7×15/100
=$1.05
Determine If The Triangles Are Similar.If They Are,Choose The Reason Why.

Answers
Answer:
because they all have 3 corners
What is the difference in minutes between the shortest collection.
Answers
The difference in the shortest collection times before and after the well installation is 20 minutes, calculated by subtracting the shortest time after installation (25 minutes) from before (45 minutes), as shown in the box and whiskers plot.
This is calculated by subtracting the shortest collection time after well installation (25 minutes) from the shortest collection time before well installation (45 minutes):
45 - 25 = 20 minutes.
This can be seen in the box and whiskers plot provided, where the lowest value for each distribution is denoted by the beginning of the whiskers.
So, the difference is before and after the well installation is 20 minutes.
To know more about box and whiskers plot:
https://brainly.com/question/15372634
#SPJ4
--The given question is incomplete, the complete question is given
" What is the difference in minutes between the shortest collection times before and after the well was installed"--
