How to find the Smallest value?
3/8
3/4
1/2
7/8
5/6

Answer:

  y =  {-180, 2 8/11}

Step-by-step explanation:

I like to solve these using a graphing calculator. I generally start by writing the equation in the form ...

  f(y) = 0

This can be done by subtracting the right-side expression from both sides. The solution will be the horizontal intercepts of the graph:

  y = -180; y = 2 8/11

__

To solve this analytically, you need to recognize that this resolves into four equations, effectively two identical pairs. Each equation comes with a set of conditions (domain) for which it is applicable. Here is the expanded set of equations:

  1/3y -7 = 2/5y +5 . . . both positive: 1/3y -7 ≥ 0 and 2/5y + 5 ≥ 0

  7 -1/3y = 2/5y +5 . . . left side negative: 1/3y -7 ≤ 0 and 2/5y +5 ≥ 0

  1/3y -7 = -2/5y -5 . . . right side negative: 1/3y -7 ≥ 0 and 2/5y +5 ≤ 0

  7 -1/3y = -2/5y -5 . . . both sides negative: 1/3y -7 ≤ 0 and 2/5y +5 ≤ 0

__

The conditions resolve to ...

  1/3y -7 ≥ 0

  y -21 ≥ 0 . . . . . multiply by 3

  y ≥ 21 . . . . . . . add 21

and ...

  2/5y +5 ≥ 0

  y +25/2 ≥ 0 . . . . multiply by 5/2

  y ≥ -25/2 . . . . . subtract 25/2

Now, we are in a position to solve the equations and determine where the solution is applicable.

__

both positive

The domain will be y ≥ 21 and y ≥ -25/2, which is y ≥ 21.

Multiplying the equation by 15 gives ...

  5y -105 = 6y +75

  y = -180 . . . . . . . . . subtract 5y+75

This solution is not in the allowed domain.

__

left side negative

The domain will be y ≤ 21 and y ≥ -25/2, which is -25/2 ≤ y ≤ 21.

Multiplying the equation by 15 gives ...

  105 -5y = 6y +75

  30 = 11y . . . . . . . . . add y-75

  y = 30/11 . . . . . .  the solution is in the allowed domain

__

right side negative

The domain will be y ≥ 21 and y ≤ -25/2. This is the empty set.

__

both sides negative

The domain will be y ≤ 21 and y ≤ -25/2, which is y ≤ -25/2.

Multiplying the equation by 15 gives ...

  105 -5y = -6y -75

  y = -180 . . . . . . . . . . add 6y-105 . . . . the solution is in the allowed domain

__

Solutions to this equation are ...

  y = 30/11 = 2 8/11

  y = -180

Answer:

Yes, the marketing manager's claim is true.

Step-by-step explanation:

p = 0.61

Phat = 545 /826 = 0.66

H0 : p = 0.61

H1 : p ≠ 0.61

The test statistic :

(Phat - p) / √pq/n

q = 1 -p = 1 - 0.61 = 0.39

(0.66 -0.61) / √(0.61*0.39)/826

0.05 / 0.0169709

Test statistic = 2.95

Using the p-value from Zscore calculator:

Pvalue at 2.95, two tailed ;

Pvalue = 0.0032

Reject H0; if Pvalue < α

Since, Pvalue < α ; Then, the marketing manager's claim is true

The value of y is 6√3 unit.

Option B is correct.

The Pythagorean theorem, or Pythagorean theorem, illustrates the relationship between the three sides of a right-angled triangle. The square of the hypotenuse is equal to the sum of the squares of the other two sides of a triangle, according to the Pythagorean theorem.

From the given figure using Pythagoras theorem

H² = 6² - 3²

H² = 36 - 9

H²  = 27

H = 3√3 unit

Now, again using Pythagoras theorem

y² = 9² + ( 3√3)²

y² = 81. + 27

y = √108

y = 6√3 unit

Thus, the value of y is 6√3 unit.

Learn more on Pythagoras theorem here:

brainly.com/question/654982

#SPJ7

The roots of the given quadratic equation are 5 and -4

Quadratics are the polynomial equation which has the highest degree of 2. Also, called quadratic equations.

Given is an equation 3x²-13x-10 = 0, we need to solve by using completing the square method,

The given equation is =

3x²-13x-10 = 0

3(x²-13x/3-10/3) = 0

3(x²-2x·13x/6-10/3) = 0

Adding and subtracting (13/6)²

3(x²-2x·13x/6+(13/6)²-(13/6)²-10/3) = 0

3[(x-13/6)²-169/36-10/3] = 0

3[(x-13/6)²-289/36] = 0

(x-13/6)²-289/36 = 0

(x-13/6)² = 289/36

Taking roots,

x-13/6 = ±17/6

x = 17/6+13/6

x = 5

Or,

x = -17/6+13/6

x = -4

Hence, the roots of the given quadratic equation are 5 and -4

Learn more about quadratic equation, click;

https://brainly.com/question/30098550

#SPJ9

⇒It is way more appropriate if I use the product rule. That states that:

⇒f(x)g(x)=f'(x)g(x)+f(x)g'(x)

\(t^{4} In(8cos(t))\\=4t^{3}In(8cos(t))+t^{4} \frac{1}{8cos(t)} *(0cos(t)+8*(-sin(t))*1)\\=4t^{3}In(8cos(t))+\frac{t^{4}-8sin(t)}{8cos(t)}\)

Note:

Given F(x)=In(x)

⇒\(F'(x)=\frac{1}{x}\)

Goodluck

Answer:

t^3 (4 ln(cos8t) - t tant)

Step-by-step explanation:

Using the Product Rule:

dy/dt = t^4 * d(ln(8cost) / dt + ln(8cost) * d(t^4)/dt

         = t^4 * 1/ (8cost) * (-8sint) + 4t^3 ln(8cost)

         = -8t^4 sint / 8 cost + 4t^3 ln(8cost)    

         = -t^4 tan t + 4t^3 ln(8cost)  

          = t^3 (4 ln(cos8t) - t tant)

The expression is simplified to 2

It is important to note that the table of exact values for trigonometric identity differ with the particular identity in study.

From the table of exact values, we have that;

sin 15 = 0. 25

sin 30 = 0. 5000

sin 45 = 0. 7071

sin 60 = 0. 8600

sin 75 = 0. 9659

sin 90 = 1

To determine the value of the expression, we have to substitute the value of sin 30 as 0. 5000

4 sin 30°

⇒ 4 × 0. 5000

multiply through

⇒ 2

The value determined is 2

Thus, the expression is simplified to 2

Learn more about trigonometric identity here:

https://brainly.com/question/7331447

#arSPJ1

Answer:

Step-by-step explanation:

First, the RS is perpendicular to TW. That is paired with "Given".

Then arc mTR = arc mRW. This is paired with, "Diameter perpendicular to a chord bisects major arcs".

After that, chord TR = chord RW. You'll pair this up with "If arcs are =, chords are =".

Finally you'll pair Triangle TRW is isosceles with "Definition".

RS perpindicular TW       Given

m RT= m RW                     Diameter perpindicular to chord bisects major arcs

TR = TW                             If arcs are =, chords are =

TRW is issocolees            Definition

The correct option D: the origin, has the symmetry of the volume of sphere for the graph of the function rule.

For the stated question.

The volume of the sphere is modeled by the power function with respect to the radius as;

V = f(r) = 4/3πr³

As, r is independent of any of the coordinate axis.

Thus, the function rule's graph, which describes the volume of the sphere, is symmetric with regard to the origin.

To know more about the symmetry along origin, here

https://brainly.com/question/1413522

#SPJ1

Answer:

a

Step-by-step explanation:

edge 2021

Answer:

0.96,1.8, 2.5, 2.75,3.03,3.21

Step-by-step explanation:

Answer:

Max exercises 13 hours a week, and Sasha 7.

Step-by-step explanation:

To find the number of hours each of them exercises during the week, we solve the system of equations.

In the second equation:

\(x = 4y - 15\)

Replacing in the first equation:

\(x + y = 20\)

\(4y - 15 + y = 20\)

\(5y = 35\)

\(y = \frac{35}{5}\)

\(y = 7\)

So Sasha exercises 7 hours per week.

Max:

\(x + y = 20\)

\(x + 7 = 20\)

\(x = 13\)

Max exercises 13 hours a week.

Using the cross product for the proff of Pythagoras theorem, the correct step is

By the cross product property, AB² =  BC multiplied by BD

The cross product property is typically used to solve equations with two values, where the product of the extremes (the outer terms) is equal to the product of the means (the inner terms).

For the similar triangles, the ratio is as follows

BD / BA = BA / BC

BA² = BD * BC

and AB = BA, hence

BA² = BD * BC

Learn more about cross product property at

https://brainly.com/question/14308624

#SPJ1

Answer:

\((x,y) = (-3,2)\) is a solution

Step-by-step explanation:

Given

\(y \le -4x + 3\)

\(x + 8y \ge 7\)

Required

Determine if \((x,y) = (-3,2)\) is a solution

\(y \le -4x + 3\) becomes

\(2 \le -4 * -3 + 3\)

\(2 \le 15\) --- this is true

\(x + 8y \ge 7\)

\(-3+ 8 * 2 \ge 7\)

\(13 \ge 7\) --- this is also true

Hence,

\((x,y) = (-3,2)\) is a solution

Answer:

x ≥ -64

Step-by-step explanation:

"3/4ths of a number is greater than or equal to -48"

3/4 of a number ≥ -48

Let's say the number is x.

(3/4)x ≥ -48

Multiply both sides by 4.

3x ≥ -192

Divide both sides by 3.

x ≥ -64

Step-by-step explanation:

27 laps.............43.2 minutes

1 lap................x minutes

cross multiply

27x=43.2

x=43.2÷27

=1.6

Answer:

Answer is in the photo so you have so look in this give wribsite

Answer:

They are not solutions

Step-by-step explanation:

To see if it is correct we have to substitute the variables for the numbers given.

x+3=30

1+3≠30

2x-y=9

2·1-10≠9

So, these solutions would be incorrect.

Answer:

Total money get from soda cans = $259.2

Step-by-step explanation:

Given:

Number of soda cases = 18

Number of soda can in each case = 24

Price of each soda can = $0.60

Find:

Total money get from soda cans

Computation:

Total money get from soda cans = Number of soda cases x Number of soda can in each case x Price of each soda can

Total money get from soda cans = 18 x 24 x 0.60

Total money get from soda cans = $259.2

f(x) = 4.1/(42.4x-10(x-2)), x-intercept = -4.878, y-intercept = (0,-2.05), asymptotes: vertical at x=2, horizontal at y=0, and slant at y=4.1/8x.

Given: f(x) = 4.1/(42.4x-10(x-2))

To find the x-intercept, we set f(x) to zero and solve for x:

0 = 4.1/(42.4x-10(x-2))

0 = 4.1/(32.4x+20)

0 = 4.1/4.08(x+4.878)

So, the x-intercept is x = -4.878.

To find the y-intercept, we set x to zero and solve for f(x):

f(0) = 4.1/(42.4(0)-10(0-2))

f(0) = -2.05

So, the y-intercept is (0,-2.05).

To write f(x) in the form f(x) = -1/(ax + b) + q, we can simplify the expression as follows:

f(x) = 4.1/(42.4x-10(x-2))

f(x) = 4.1/(32.4x+20)

f(x) = 4.1/4.08(8x+5)

So, a = 8, b = 5, and q = 4.1/4.08.

To draw the graph of f, we plot the x- and y-intercepts and the vertical asymptote x = 2.

We can find the horizontal asymptote by noting that as x becomes very large or very small, the term 10(x-2) dominates the expression, so f(x) approaches 4.1/-10x. Thus, the horizontal asymptote is y = 0.

To find the equations of the slant asymptotes, we divide the numerator by the denominator using long division:

4.1

8x + 5 | 4.1

- 4.1

0

So, the slant asymptote is y = 4.1/8x.

Therefore, the intercepts of f are x = -4.878 and (0,-2.05), and the equation of f(x) in the form f(x) = -1/(ax + b) + q is f(x) = -1/(8x + 5) + 1.0039.

The graph of f has intercepts with the x-axis at x = -4.878 and the y-axis at (0,-2.05), a vertical asymptote at x = 2, a horizontal asymptote at y = 0, and a slant asymptote at y = 4.1/8x.

To learn more about intercept visit :

https://brainly.com/question/1884491

#SPJ1

Answer:

Step-by-step explanation:

Graph the inequalities and plot the points.

See attached.

As we see, the solution is the option B, point (3, 2). All the other points are either outside or on the dashed line.

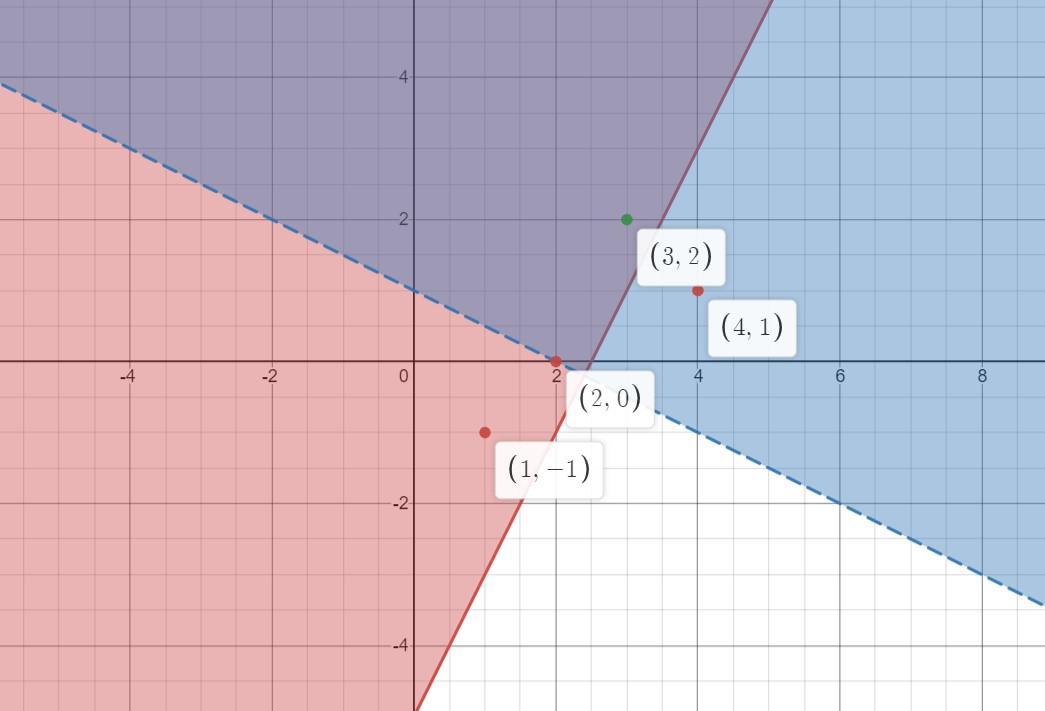
Graph attached

Second one should be 2x+2y>2

If n is a perfect square, then n +2 is not a perfect square, then The correct order is: 1, 2, 3, 4, 5, 6.

Given,

Assume n= m, for some non-negative integer m.

If m=0, then n + 2 = 2, which is not a perfect square.

The smallest perfect square greater than n is (m + 1)

Expand (m + 1)2 to obtain (m + 1)2 = m2 + 2m +1 = n + 2m +1

Simplify to n +2 +1> n +2.

Hence, n+ 2 is not a perfect square.

If n is a perfect square, then n +2 is not a perfect square, then

The correct order is: 1, 2, 3, 4, 5, 6.

For similar questions on perfect square,

https://brainly.com/question/1538726

#SPJ4

Answer:

false

Step-by-step explanation:

you only need to reverse the sign when you are dividing by a negative number

Answer: False?

Step-by-step explanation:

Answer:

24.58 km

Step-by-step explanation:

1 km = 1000 m

1 m = 1 / 1000 km

24580 m

= 24580 / 1000

= 24.58 km

The equation X^2/2 - 8 = 2X - 2 has two solutions, X = 6 and X = -2. These are the values of X that satisfy the equation and make both equations Y = X^2/2 - 8 and Y = 2X - 2 intersect on the graph.

To find the solutions to the equation X^2/2 - 8 = 2X - 2, we need to set the two equations equal to each other and solve for X.

The equation is:

X^2/2 - 8 = 2X - 2

To simplify the equation, let's multiply both sides by 2 to eliminate the fraction:

X^2 - 16 = 4X - 4

Next, we rearrange the equation to have all terms on one side:

X^2 - 4X - 12 = 0

Now, we can solve this quadratic equation by factoring, completing the square, or using the quadratic formula. Let's use factoring in this case:

(X - 6)(X + 2) = 0

Setting each factor equal to zero gives us two possible solutions:

X - 6 = 0 --> X = 6

X + 2 = 0 --> X = -2

So the solutions to the equation X^2/2 - 8 = 2X - 2 are X = 6 and X = -2.

For more such question on intersect . visit :

https://brainly.com/question/30915785

#SPJ8

The percent decrease in the boiling point of water from sea level to 7,500 feet is equal to 18.7%.

In Mathematics, a percentage simply refers to any number that is expressed as a fraction of hundred (100). This ultimately implies that, a percentage indicates the hundredth parts of any given number.

In order to determine the percent decrease in the boiling point of water from sea level to 7,500 feet, we would determine the difference in temperature as follows;

Difference in temperature = 212°F - 198°F

Difference in temperature = 14°F

Percentage decrease = 14/7500 × 100

Percentage decrease = 0.00187 × 100

Percentage decrease = 18.7%.

Read more on percentage here: brainly.com/question/11360390

#SPJ1

Answer: 70

Step-by-step explanation: First, let's find how many are worth each in numbers. Use this formula:

Part Percent * Whole number / 100

Plug in the numbers:

Red = 12 * 250 / 100

3000/100

= 30 red marbles

Blue = 16* 250 / 100

4000 / 100

= 40 blue marbles

40 + 30 = 70

White = 250 - 70

= 180 white marbles

We can check our answer by adding 12 + 16. That's 28. So by multiplying 250 by 28, we get 7000. 7000 divided by 100 = 70

70 = 70

Therefore, both statements are true

I hope this helped!

Trawler B should take a course that is 18 degrees east of north.

In order to intercept Trawler A, Trawler B must travel in a direction that will bring it closer to Trawler A. Since Trawler A is traveling due west, Trawler B must travel in a direction that is east of north. The angle that is 18 degrees east of north will bring Trawler B closest to Trawler A.

Here are the steps to find the course that Trawler B should take:

Draw a diagram of the situation.

Label the two trawlers A and B.

Draw a line representing the direction that Trawler A is traveling.

Draw a line representing the direction that Trawler B should travel.

Measure the angle between the two lines.

The angle between the two lines will be 18 degrees. Therefore, Trawler B should take a course that is 18 degrees east of north.

Learn more about angle on

https://brainly.com/question/25716982

#SPJ1