Answers
Answer:
6x^4 - 10x^3 - 28x + 15
Step-by-step explanation:
(6x^3 + 4x^2 + 6x - 5) (2x - 3)
= 6x^4 - 18x^3 + 8 x^3 - 12x^2 + 12 x^2 - 18x - 10x+ 15
= 6x^4 - 10x^3 - 28x + 15
Related Questions
The series Στα 25 (1+2) converges by the integral test. (You do not have to verify this.) Denote the sum of the series by s, and let su denote the nth partial sum of the series. (a) Write out explicitly the partial sume, and then us a calculator to compute this partial sun to four decimal places (b) Using the crror bound for the integral test, determine a value of n which ensures that is within 0.0001 of
Answers
The error between the nth partial sum and the actual sum is at most 0.0001, we need to take n to be at least 13,525.
The given series is Σ τα 25 (1+2), which can be written as Σ (3/τ^α), where α = 25. We know that this series converges by the integral test, so we can use that to estimate the value of its sum.
(a) The nth partial sum of the series is given by:
su = Σ (3/τ^α) from k = 1 to n
We can compute this sum using a calculator. For example, for n = 10, we have:
s10 = Σ (3/τ^25) from k = 1 to 10
= (3/1^25) + (3/2^25) + (3/3^25) + ... + (3/10^25)
≈ 0.6417
(b) The error bound for the integral test tells us that the error between the nth partial sum and the actual sum is at most the absolute value of the difference between the integral of the function and the nth partial sum. That is:
|s - sn| ≤ ∫(n+1, ∞) 3/τ^α dτ
We want to find a value of n such that this error bound is at most 0.0001. We can use the fact that the integral of 3/τ^α from n+1 to infinity is given by:
∫(n+1, ∞) 3/τ^α dτ = 3/(α-1) n^(1-α)
So we need to find the smallest value of n such that:
3/(α-1) n^(1-α) ≤ 0.0001
Simplifying this inequality, we get:
n ≥ [(3/(α-1)) / 0.0001]^(1/(1-α))
Plugging in α = 25, we have:
n ≥ [(3/24) / 0.0001]^(1/-24)
≈ 13,525
Therefore, to ensure that the error between the nth partial sum and the actual sum is at most 0.0001, we need to take n to be at least 13,525.
To learn more about series converges visit: https://brainly.com/question/15415793
#SPJ11
Three infinite straight wires are fixed in place and aligned parallel to the z-axis as shown. The wire at (x,y) = (-18 cm, 0) carries current 11 = 2 A in the negative z- direction. The wire at (x,y) = (18 cm, 0) carries current 12 = 1.2 A in the positive z- direction. The wire at (x,y) = (0, 31.2 cm) carries current 13 = 6.9 A in the positive z- direction. = = X d I,A) What is Fx(1), the x-component of the force exerted on a one meter length of the wire carrying current I1?
B) What is Fy(1), the y-component of the force exerted on a one meter length of the wire carrying current I1?
C)What is Fx(2), the x-component of the force exerted on a one meter length of the wire carrying current I2?
Answers
For the three infinite straight wires, the y-component force on one meter of wire carrying I1 is 2.48 × \(10^{-6}\) N, and the x-component force on one meter of wire carrying I2 is 1.96 × \(10^{-6}\) N.
A) To find Fx(1), we need to use the formula:
Fx(1) = I1 × L × Bx(1)
where L is the length of the wire, and Bx(1) is the x-component of the magnetic field at the location of the wire carrying current I1.
To find Bx(1), we can use the Biot-Savart law, which states that the magnetic field at a point due to a current-carrying wire is proportional to the current and inversely proportional to the distance from the point to the wire.
Bx(1) is the sum of the x-components of the magnetic fields due to the other two wires, which are:
B1x(1) = μ0 × I1 × I2 / (2πd)
B2x(1) = μ0 × I1 × I3 / (2πd)
where d is the distance between the wires, and μ0 is the permeability of free space.
Substituting the given values, we get:
B1x(1) = (4π × \(10^{-7}\) T·m/A) × 2 A × 1.2 A / (2π × 0.36 m) = 4.4 × \(10^{-7}\) T
B2x(1) = (4π × \(10^{-7}\) T·m/A) × 2 A × 6.9 A / (2π × 0.312 m) = 8.8 × \(10^{-7}\) T
Therefore, the total Bx(1) is:
Bx(1) = B1x(1) - B2x(1) = -4.4 × \(10^{-7}\) T
Substituting this value and the given length of 1 meter, we get:
Fx(1) = (2 A) × (1 m) × (-4.4 × \(10^{-7}\) T) = -8.8 × \(10^{-7}\) N
So the x-component of the force exerted on a one-meter length of the wire carrying current I1 is -8.8 × \(10^{-7}\) N.
B) To find Fy(1), we can use the same formula as above, but with the y-component of the magnetic field at the location of the wire carrying current I1:
Fy(1) = I1 × L × By(1)
where By(1) is the y-component of the magnetic field at the location of the wire carrying current I1, which is the sum of the y-components of the magnetic fields due to the other two wires:
B1y(1) = 0
B2y(1) = μ0 × I1 × I3 / (2πd)
Substituting the given values, we get:
B2y(1) = (4π × \(10^{-7}\) T·m/A) × 2 A × 6.9 A / (2π × 0.312 m) = 1.24 × \(10^{-6}\) T
Therefore, the total By(1) is:
By(1) = B1y(1) + B2y(1) = 1.24 × \(10^{-6}\) T
Substituting this value and the given length of 1 meter, we get:
Fy(1) = (2 A) × (1 m) × (1.24 × \(10^{-6}\) T) = 2.48 × \(10^{-6}\) N
So the y-component of the force exerted on a one-meter length of the wire carrying current I1 is 2.48 × \(10^{-6}\) N.
The x-component of the force exerted on a one-meter length of the wire carrying current I2 is 1.96 × \(10^{-6}\) N.
Learn more about the infinite straight wires at
https://brainly.com/question/12900769
#SPJ4
The JASON group and the NIH Advisory Committee to the Director have issued similar recommendations about research integrity that would allow universities and federal agencies to manage undue foreign influence more effectively. What was this recommendation
Answers
The JASON group and the NIH Advisory Committee to the Director have issued recommendations that aim to enhance the management of undue foreign influence in research integrity.
The recommendation put forth by both the JASON group and the NIH Advisory Committee to the Director revolves around strengthening measures to address undue foreign influence in research integrity. This includes enhancing the ability of universities and federal agencies to effectively manage and mitigate the risks associated with foreign influence on research activities.
The specific details of the recommendation may vary, but the overarching goal is to establish robust mechanisms for identifying and addressing potential threats to research integrity posed by undue foreign influence. This may involve implementing stricter oversight, transparency, and disclosure requirements, as well as promoting education interest among researchers.
Learn more about interest here:
https://brainly.com/question/30955042
#SPJ11
what value of z divides the standard normal distribution so that half the area is on one side and half is on the other? round your answer to two decimal places. if you would like to look up the value in a table, select the table you want to view, then either click the cell at the intersection of the row and column or use the arrow keys to find the appropriate cell in the table and select it using the space key. note: selecting a cell will return the value associated with the column and row headers for that cell.
Answers
The value of z that divides the standard normal distribution so that half the area is on one side and half is on the other is 0.00.
To understand this better, let's visualize the standard normal distribution. The graph of the standard normal distribution is a bell-shaped curve that is symmetrical around the mean of 0.
When we say that half the area is on one side and half is on the other, we mean that the area under the curve to the left of the z-score is equal to the area under the curve to the right of the z-score.
Since the standard normal distribution is symmetric, this occurs when the z-score is equal to 0.00. At this point, the area to the left of 0.00 is 0.50 (or 50%), and the area to the right of 0.00 is also 0.50 (or 50%).
To know more about distribution here
https://brainly.com/question/31197941
#SPJ4
Please Please Please help me

Answers
Answer:
18 \(\sqrt{5\\}\)
Step-by-step explanation:
Answer:
Original: 40.249223595
Simplified: 40.2
Step-by-step explanation:
3√5 = 6.7 (simplified)
15√5 = 33.5 (simplified)
6.7 + 33.5 = 40.2
Aashna thinks that the largest place value that 82.3 and 11.45 have in common is the tens place. Jake thinks that the largest place value they have in common is the tenths place.
Answer the following questions with complete sentences:
Who is correct, Aashna or Jake?
Write how you would explain your answer to the person who answered incorrectly.
Answers
Answer:
Aashna is correct
Step-by-step explanation:
I would explain it that the tens places are 8 and 1, which are about 7 apart
The tens places however are 3 and 4 which is closer.
its iready help pls im give brainiest

Answers
Answer:
The answer is 0. those types of lines NEVER touch
In a class in which the final course grade depends entirely on the average of four equally weighted 100-point tests, Joyce has scored 90, 93, and 85 on the first three. What range of scores on the fourth test will give Joyce a C for the semester (an average between 70 and 79, inclusive)? Assume that all test scores have a non-negative value.
Express your answer in interval notation.
HELP ASAP BRAINLEST AND THANKS
Answers
Answer:
[12,48]
Step-by-step explanation:
We will start by calculating the score she needs to get a 70 in the class
We will let x equal the fourth test
\(\frac{90+93+85+x}{4} =70\)
This isn't hard to solve and we will do so by multiplying 4 by both sides and subtracting 268 (90+93+85)
This means that x= 12
We will then use the same formula but set it equal to 79
\(\frac{90+93+85+x}{4} =79\)
We solve x in the exact same way and get x=48
our final answer is now [12,48]
how many bit strings of length seven either begin with two 0s or end with three 1s?
Answers
There are 40 such bit strings.
To count the number of bit strings of length seven that either begin with two 0s or end with three 1s, we need to use the principle of inclusion-exclusion.
Let A be the set of bit strings that begin with two 0s, and let B be the set of bit strings that end with three 1s.
Then, we want to find the size of the set A ∪ B, which consists of bit strings that satisfy either condition.
The size of A can be calculated as follows:
since the first two digits must be 0, the remaining five digits can be any combination of 0s and 1s,
so there are \(2^5 = 32\) possible strings that begin with two 0s.
Similarly, the size of B can be calculated as follows:
since the last three digits must be 1, the first four digits can be any combination of 0s and 1s,
so there are\(2^4 = 16\) possible strings that end with three 1s.
However, we have counted the strings that both begin with two 0s and end with three 1s twice.
To correct for this, we need to subtract the number of strings that belong to both A and B from the total count.
The strings that belong to both A and B must begin with two 0s and end with three 1s, so they have the form 00111xxx,
where the x's can be any combination of 0s and 1s.
There are \(2^3 = 8\) such strings.
Therefore, the total number of bit strings of length seven that either begin with two 0s or end with three 1s is:
|A ∪ B| = |A| + |B| - |A ∩ B| = 32 + 16 - 8 = 40.
For similar question on combination.
https://brainly.com/question/11732255
#SPJ11
Which algebraic expression represents “the quotient of a number and six”?

Answers
Answer:
The last answer w/6
Step-by-step explanation:
The quotient of a number and six is like saying a number divided by 6 which we represent with w.
Brainliest Please . . . .
Answer:
last option (w/6)
Step-by-step explanation:
Quotient=division
"the number" represents a variable, in this case W
divide it by six
that's W/6
How do I even figure this out??

Answers
The value of a is 1/64.
The rules of the exponent are
(a) \(a^m*a^n=a^{m+n\)
(b)\(a^m/a^n=a^{m-n\)
(c)\((a^b)^c=a^{bc}\)
(d) \(\sqrt[n]{a}=a^{1/n\)
(e) a⁻ⁿ=1/aⁿ
From the table it is clear that
By the rule of the exponent a⁻ⁿ=1/aⁿ
where a=2
2⁻¹ = 1/2¹ = 1/2
2⁻² =1/2² =1/4
2⁻³ =1/2³ =1/8
2⁻⁴ =1/2⁴ =1/16
2⁻⁵ =1/2⁵ =1/32
So we have to calculate the left one where it is given that we have to estimate the value for 2⁻⁶ which is a.
From the above, it is clear that 2⁻ⁿ =1/2ⁿ
using the above formula
2⁻⁶= 1/2⁶ =1/64= a
Therefore the value of a is 1/64.
Learn more about the exponent
here: https://brainly.com/question/535578
#SPJ10
a box with a square base and open top must have a volume of . we wish to find the dimensions of the box that minimize the amount of material used. first, find a formula for the surface area of the box in terms of only , the length of one side of the square base. simplify your formula as much as possible. next, find the derivative, .
Answers
The formula for the surface area of the box, in terms of the length of one side of the square base (s), is A = s^2 + 4s^2 = 5s^2.
The derivative of the surface area function with respect to s, denoted as dA/ds, gives us the rate of change of the surface area with respect to the length of one side of the base.
1. The surface area of the box consists of the area of the square base and the four equal sides. The area of the square base is s^2, and each side has a length of s. Therefore, the total surface area is given by A = s^2 + 4s^2 = 5s^2.
2. To find the derivative of the surface area function, we differentiate 5s^2 with respect to s using the power rule of differentiation. The power rule states that if we have a function f(x) = cx^n, then the derivative is f'(x) = cnx^(n-1).
Applying the power rule, we have dA/ds = d(5s^2)/ds = 10s.
3. The derivative, dA/ds = 10s, represents the rate of change of the surface area with respect to the length of one side of the square base. This means that for every unit increase in s, the surface area increases by 10s units.
The derivative does not provide information about minimizing the amount of material used. To find the dimensions of the box that minimize the amount of material used, we need to set up an optimization problem and solve for the critical points. This involves setting the derivative equal to zero and finding the values of s that satisfy this equation. However, since the problem statement does not provide a specific volume constraint or objective function, we cannot proceed with the optimization process.
To learn more about derivative, click here: brainly.com/question/23819325
#SPJ11
help pleaseeee asapppp
The value of a gold coin picturing the head of the Roman Emperor Domitian is increasing at the rate of 6% per year. If the coin is worth $155 now, what will it be worth in 14 years?
Question 6 options:
$239.00
$285.20
$294.72
$350.44
Answers
Answer:
anything :P
Step-by-step explanation:
Greg uses a triangular area of his backyard as a garden. If the area of his backyard is 1,248 square feet, what is the area of the garden?
Answers
Unfortunately, we don't have enough information to determine the area of the garden. We would need to know the dimensions of the backyard and/or the garden to calculate their areas.
To know more about calculating areas refer here:
https://brainly.com/question/30656333
#SPJ11
Can you find the domain and range and type the correct code? help me please.

Answers
The graphs are identified as follows
1. the domain is option G
2. the range is option E
3. the domain is option D
4. the range is option C
What is domain and range in coordinate geometryIn coordinate geometry, the domain and range are concepts used to describe the set of possible inputs (x-values) and outputs (y-values) of a function, respectively.
The domain of a function is the set of all possible x-values for which the function is defined. In other words, it is the set of all values that can be plugged into the function and produce a meaningful output.
The range of a function is the set of all possible y-values that the function can take on as x varies over its domain. In other words, it is the set of all values that the function can output.
Learn more about domain and range at
https://brainly.com/question/2264373
#SPJ1
Andrew plays a game where he draws one card from a well-shuffled standard deck of 52 cards 나. He wins the game if the card he draws is a Spade or a Heart Are the two events mutually exclusive? The two events are not mutually exclusive. There is not enough information to determine if the two events are mutually exclusive. The two events are mutually exclusive. What is the probability that Andrew wins the game? What is the probability that Andrew loses the game?
Answers
The probability that Andrew wins the game is 0.5, and the probability that he loses the game is also 0.5.The probability that Andrew wins the game can be calculated by finding the probability of drawing a Spade or a Heart from a standard deck of 52 cards.
There are 13 cards of each suit (Spades and Hearts), so there are a total of 26 cards that would result in a win for Andrew. The probability of winning the game is therefore 26/52, which simplifies to 1/2 or 0.5.
Similarly, the probability of losing the game can be calculated as the complement of the probability of winning. Since there are 52 cards in total and 26 of them result in a win, there are 26 cards that would result in a loss for Andrew. Therefore, the probability of losing the game is also 26/52, which is equal to 1/2 or 0.5.
In summary, the probability that Andrew wins the game is 0.5, and the probability that he loses the game is also 0.5.
Learn more about Probability here:
brainly.com/question/31828911
#SPJ11
In a group of 101 students 40 are juniors, 50 are female, and 22 are female juniors. Find the probability that a student picked from this group at random is either a junior or female.
a) 90/101
b) 22/101
c) 68/101
d) 40/101
Answers
Answer:
I would say A. as a best guess but the question is odd it does not add up to 101 students it adds up to 101 plus all of them are female juniors so they would be picked every time it would be 101/101
IV Find the citical points at which profit (pie) is maximized given the total revenue TR=4700−302 and Total CostTC =320/10,500 (2pts) 1. Compute Marginal Revenue and Marginal Cost 2. Equate MR=MC to find Q
∗
3. Verify that Q* is a relative maximum point 4. Compute the maximum profit level (pie) )
∗
by establishing (pie)* =9( (pie) (Q
∗
)
Answers
To find the critical points at which profit is maximized given the total revenue TR = 4700 - 302 and total cost TC = 320/10,500, we need to compute the marginal revenue and marginal cost, equate MR = MC to find the optimal quantity Q∗, verify if Q∗ is a relative maximum point, and compute the maximum profit level (π) by evaluating π∗ = 9(π(Q∗)).
Marginal Revenue (MR) is the derivative of the total revenue function with respect to quantity (Q). In this case, MR = dTR/dQ. By taking the derivative of TR = 4700 - 302 with respect to Q, we can find the expression for MR.
Marginal Cost (MC) is the derivative of the total cost function with respect to quantity (Q). In this case, MC = dTC/dQ. By taking the derivative of TC = 320/10,500 with respect to Q, we can find the expression for MC.
To find the optimal quantity Q∗, we equate MR and MC by setting MR = MC and solve for Q. This is because profit is maximized when MR equals MC.
Once we have found Q∗, we need to verify if it is a relative maximum point. This can be done by checking the second derivative of the profit function and determining if it is negative at Q∗. If the second derivative is negative, it confirms that Q∗ is a relative maximum point.
Finally, to compute the maximum profit level (π∗), we evaluate π(Q∗) by substituting Q∗ into the profit function. In this case, we can multiply the value of π(Q∗) by 9 to obtain the maximum profit level (π∗).
Learn more about relative maximum point here:
https://brainly.com/question/32310828
#SPJ11
an important first step in assessing the relationship of two interval level variables is to: a. calculate a correlation coefficient. b. look at a scatter plot. c. do a test of significance. d. calculate the variance of the independent variable.
Answers
Option a. calculate a correlation coefficient is the right response because a correlation coefficient measures the strength and direction of the relationship between two interval level variables.
This is because a correlation coefficient measures the strength and direction of the relationship between two interval level variables. It provides a numerical value that ranges from -1 to 1, where -1 indicates a strong negative correlation, 0 indicates no correlation, and 1 indicates a strong positive correlation.
A scatter plot is also useful in visualizing the relationship between two variables, but it does not provide a numerical value like the correlation coefficient. A test of significance and variance calculation are not typically used as first steps in assessing the relationship between two variables.
Hence, option a. calculate a correlation coefficient is the correct answer.
Learn more about "correlation ":
https://brainly.com/question/4219149
#SPJ11
A= 1/2 x 3.2 x 3.6=?

Answers
Answer:
5.76
Step-by-step explanation:
Multiply them together.
To make marbled paper, Shannon filled a rectangular 10/279 cm by 10/178 dish with water. Then they gently swirled paint on top of the water. Let A represent the area of the dish.
Select 1 multiplication and 1 division equation to represent the relationship.
A) 178/10 x A = 279/10
B) 178/10 x 279/10 = A
A) 279/10 / 178/10 = A
B) A / 178/10 = 279/10
Answers
When Shannon filled a rectangular 10/279 cm by 10/178 dish with water, the area is B) 178/10 x 279/10 = A and A / 178/10 = 279/10
How to illustrate the information?It should be noted that in order to make the paper, Shannon filled a rectangular 10/279 cm by 10/178 dish with water and then they gently swirled paint on top of the water.
Therefore, the area of the rectangular paper as illustrated will be calculated by multiplying the dimensions given. This will be:
Area = Length × Width
Area = 10/279 × 10/178
Therefore, based on the information, it should be noted that the correct option is B.
Learn more about area on:
brainly.com/question/25292087
#SPJ1
Answer:
b and d
Step-by-step explanation:
Expand 25.3(2x+3)please help
Answers
Answer:
50.6x + 75.9
Step-by-step explanation:
25.3(2x+3) = 50.6x+75.9
The Process of finding all the prime factors of a number is called _______
Answers
The Process of finding all the prime factors of a number is called prime factorization.
Prime factorization is the process of expressing a composite number as a product of its prime factors. In other words, it is the process of finding all the prime numbers that divide a given number exactly without leaving a remainder.
For example, the prime factorization of the number 20 is 2 x 2 x 5. This means that 20 is the product of the prime numbers 2 and 5, which are its prime factors.
Prime factorization is a fundamental concept in number theory and is used in many areas of mathematics, such as in the study of fractions, decimals, and rational numbers. It is also used in cryptography, where large numbers are factorized to ensure the security of encrypted messages.
Learn more about prime factorization here
brainly.com/question/29775157
#SPJ4
find k so that x+2 is a factor of x^3 - kx^2 + 2x + 7k
Answers
Answer:
k = 4
Step-by-step explanation:
If (x + 2) is a factor then f(- 2) = 0
f(x) = x³ - kx² + 2x + 7k , then
f(- 2) = (- 2)³ - k(- 2)² + 2(- 2) + 7k = 0
⇒ - 8 - k(4) - 4 + 7k = 0
⇒ - 8 - 4k - 4 + 7k = 0
⇒ 3k - 12 = 0 ( add 12 to both sides )
⇒ 3k = 12 (divide both sides by 3 )
⇒ k = 4
Answer:
k = 4
Step-by-step explanation:
If x + 2 is a factor of x³ - kx² + 2x + 7k then
the value of x = -2
Solve for k
f ( x ) = x³ - kx² + 2x + 7kplug -2 as x in the expression.
f ( -2) = ( -2)³ - k ( -2)² + 2 ( -2 ) + 7 k = 0expand the exponents
= -8 -4k -4 + 7k = 0combine like terms
= -8 -4 + -4k + 7k = 0= -12 + 3k = 0add 12 to both side
-12 + 12 + 3k = 123k = 12divide each side by 3
3k / 3 = 12/3k = 4Let n and k be positive integers, with 1 ≤ k
Answers
Answer:
Step-by-step explanation:
Step 1: Concept Introduction
Sum rule: If an event can occur either in ways or in ways (non-overlapping), the number of ways the event can occur is then m+n.
The definition of permutation (order is important) is –
No repetition allowed:
Repetition allowed:
The definition of combination (order is important) is –
No repetition allowed:
Repetition allowed:
With
.
Distributing distinguishable objects into k distinguishable boxes such that
; objects are placed in the box
can be done in
ways.
Step 2: Non-integer solution
(a)
The integer solutions of the equation
can be obtained by selecting r objects from a set with n objects such that there are
chosen from the first type,
are chosen from the second type and so on.
Thus, the number of solutions can then be obtained by using the definition of a combination (since the order of the solutions is not important) and repetition is allowed (since more than one
value can take on the same value) –
Therefore, the result is obtained as
.
Step 3: Number of non-negative integer solutions
(b)
It is given that –
It is needed to select 17 indistinguishable objects from 4 distinguishable boxes (variables).
Here n=4, r=17 .
Since repetition is allowed, so substitute the value and calculate –
Therefore, the result is obtained as 1140.
Step 4: Number of positive integer solutions
(c)
It is given that –
All variables have to be at least 1. Then redefine
and
(which are then 4 variables that are at least 0 ).
It is needed to select 13 indistinguishable objects from 4 distinguishable boxes (variables).
Here n=4, r=3 .
Since repetition is allowed, so substitute the value and calculate the –
Therefore, the result is obtained as 560.
The figures are similar. Find the missing length.

Answers
Step-by-step explanation:
Answer:-Given ,The figure is Similar.
Observation:-
Similar figures have similar sides. If we see carefully in smaller triangle, 3 has been added to each side and they are similar. We need to find y.
We have :-
5+3=8 as similar sides.
So, applying same algorithm,
\(y + 3 = 5\)
\(y = 5 - 3\)
\( \boxed{ \tt{y = 2 \ cm}}\)
is the answer.
Hope it helps :)
a triangle whose angles have measures 3x, 4x, and x-20
Answers
Answer:
All equal 180
Step-by-step explanation:
(i) The sum of all the 3 angles of a triangle is always equal to 180 degrees.
(ii) If we are given 3 angles of a triangle in terms of a variable, then we set up their sum to be 180 degrees and solve for the variable.
(iii) We substitute the value of the variable back into the given angles to find their measurements.
Suppose the future value of a \( 7.75 \% \) simple interest loan is \( \$ 1,321.17 \) at the end of 230 days. Find the present value of the loan. State your result to the nearest penny.
Answers
The present value of the loan is found to be approximately $70.28.
To find the present value of a loan, we can use the formula:
Present Value = Future Value / (1 + (interest rate * time))
Given that the future value is $1,321.17, the interest rate is 7.75%, and the time is 230 days, we can plug in these values into the formula:
Present Value = 1321.17 / (1 + (0.0775 * 230))
Calculating the expression in the parentheses first:
Present Value = 1321.17 / (1 + 17.8075)
Simplifying further:
Present Value = 1321.17 / 18.8075
Evaluating the division:
Present Value ≈ $70.28
Therefore, the present value of the loan is approximately $70.28.
Know more about the present value
https://brainly.com/question/30390056
#SPJ11
help help help pls ill give brainliest
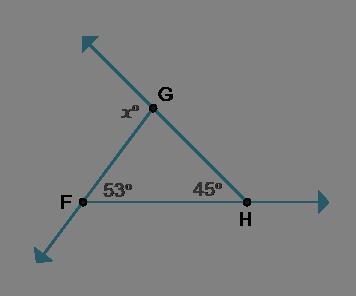
Answers
Answer:
96
Step-by-step explanation:
53+45=96
180-96=84
180-84=96
tammy divided 3 4/5 of a liter of fertilizer evenly among some smaller bottles. she put 1/3 of a liter into each bottle. how many smaller bottles did tammy fill?
Answers
Tammy divided 3 4/5 liters of fertilizer evenly among smaller bottles. To find the number of bottles she filled, we need to divide 3 4/5 by 1/3. To do this, we can convert the mixed number to an improper fraction by multiplying the whole number by the denominator and adding the numerator.
3 4/5 is equivalent to 19/5. Then, we can multiply 19/5 by the reciprocal of 1/3, which is 3/1. This gives us 19/5 x 3/1 = 57/5. Therefore, Tammy filled 57/5 or 11 2/5 smaller bottles with fertilizer. Tammy divided 3 4/5 liters of fertilizer evenly among smaller bottles, with 1/3 liter in each bottle. To find the number of smaller bottles, first convert the mixed number to an improper fraction: (3 * 5 + 4)/5 = 19/5.
Now, divide the total amount of fertilizer (19/5) by the amount in each smaller bottle (1/3): (19/5) / (1/3) = (19/5) * (3/1) = 57/5. Since 57/5 is an improper fraction, we can convert it back to a mixed number: 57 ÷ 5 = 11 with a remainder of 2. Therefore, Tammy filled 11 smaller bottles with 1/3 liter of fertilizer each.
To know more about fertilizer visit-
https://brainly.com/question/24196345
#SPJ11
