If g(x, y)=-xy2 +exy, x=rcostheta , and y=rsin theta, find dg/dr in terms of r and theta.
Answers
dg/dr in terms of r and theta dg/dr = ( \(-y^{2}\) + yex)(cos(theta)) + ( \(-y^{2}\) + yex)(-rsin(theta))+ (-2xy + ex)(sin(theta)) + (-2xy + ex)(rcos(theta))
To find dg/dr in terms of r and theta, we need to compute the partial derivatives of g(x, y) with respect to x and y, and then apply the chain rule to express them in terms of r and theta.
Given:
g(x, y) = -x\(y^{2}\) + exy
x = rcos(theta)
y = rsin(theta)
Let's start by finding the partial derivatives of g(x, y) with respect to x and y:
∂g/∂x = \(-y^{2}\) + yex
∂g/∂y = -2xy + ex
Next, we apply the chain rule to express the partial derivatives in terms of r and theta:
∂g/∂x = (∂g/∂x)(∂x/∂r) + (∂g/∂x)(∂x/∂theta)
= ( \(-y^{2}\)+ yex)(cos(theta)) + (\(-y^{2}\) + yex)(-rsin(theta))
∂g/∂y = (∂g/∂y)(∂y/∂r) + (∂g/∂y)(∂y/∂theta)
= (-2xy + ex)(sin(theta)) + (-2xy + ex)(rcos(theta))
Now, we substitute the expressions for x and y:
∂x/∂r = cos(theta)
∂x/∂theta = -rsin(theta)
∂y/∂r = sin(theta)
∂y/∂theta = rcos(theta)
Substituting these values back into the partial derivatives:
∂g/∂x = (\(-y^{2}\) + yex)(cos(theta)) + (\(-y^{2}\) + yex)(-rsin(theta))
∂g/∂y = (-2xy + ex)(sin(theta)) + (-2xy + ex)(rcos(theta))
Now, we can express dg/dr in terms of r and theta by combining the terms:
dg/dr = (∂g/∂x)(∂x/∂r) + (∂g/∂y)(∂y/∂r)
= (\(-y^{2}\) + yex)(cos(theta)) + (\(-y^{2}\) + yex)(-rsin(theta))
+ (-2xy + ex)(sin(theta)) + (-2xy + ex)(rcos(theta))
Learn more about partial derivatives;
https://brainly.com/question/2576610
#SPJ4
Related Questions
Data were collected on the distance a baseball will travel when hit by a baseball bat at a certain speed. the speed, s, is measured in miles per hour, and distance, y, is measured in yards. the line of fit is given by Å· = 3.98 53.59s. if the ball travels for a duration of 5 seconds, what is the predicted distance of the ball? 267.95 feet 271.93 feet 283.87 feet 287.85 feet
Answers
By evaluating the linear equation in t = 5 seconds, we will see that the distance traveled is 271.93 feet.
What is the predicted distance of the ball?
Here we know that the line that defines the distance traveled by the ball (y) as a function of time (t) is:
y = 3.98 + 53.59*t
Now we want to predict the distance traveled y the ball if it travels for 5 seconds, so we just need to evaluate the above linear equation in t = 5.
So we will get:
y = 3.98 + 53.59*5 = 271.93
So we conclude that the distance traveled in that time is 271.93 feet.
Learn more about linear equations:
https://brainly.com/question/1884491
#SPJ1
Answer: 271.93 feet
Step-by-step explanation:
I took the test

Solve each system by substitution
y=4x-5
y=2x-5
Need help with this asap and I need my work shown as well!!!
Answers
Answer:
equalize the both sides of the functions
Step-by-step explanation:
4x-5 = 2x-5
4x = 2x
2x = x
2 = 1 meaning phi
there is no answer for this problem
Answer:
x = 0, y = -5
Step-by-step explanation:
you're given two equations:
y = 4x - 5
y = 2x - 5
to solve, just substitute y in the second equation.
y = 2x - 5
4x - 5 = 2x - 5
now that you have this, solve for x.
4x - 5 = 2x - 5
subtract 2x from both sides
4x - 5 - 2x = 2x - 5 - 2x
2x - 5 = -5
add 5 to both sides
2x - 5 + 5 = - 5 + 5
2x = 0
divide both sides by 2
2x/2 = 0/2
x = 0
now that you know x, substitute x for 0 in the second equation.
y = 2x - 5
y = 2 * 0 - 5
y = 0 - 5
y = - 5
State which metric unit you would probably use to measure item.
Water in a bathtub
Answers
To measure the amount of water in a bathtub, you would typically use the metric unit of volume, which is litres (L) or cubic meters (m³).
Volume is a measurement of the amount of space occupied by an object or substance. In the case of water in a bathtub, you would measure the volume of water it can hold. The most commonly used metric units for volume are liters and cubic meters. Liters are commonly used for smaller quantities, while cubic meters are used for larger volumes.
To measure the volume of water in a bathtub, you can follow these steps:
1. Make sure the bathtub is empty.
2. Fill the bathtub with water until it reaches the desired level.
3. Use a measuring container marked in liters or cubic meters to scoop out the water from the bathtub.
4. Keep pouring the water into the measuring container until the bathtub is empty.
5. Read the volume measurement on the container to determine the amount of water in liters or cubic meters.
To know more about volume measurements, refer here:
https://brainly.com/question/30229789#
#SPJ11
A sweatshirt costs $48 at Hollister. The sales tax rate is 7%. How much will it cost to buy the sweatshirt?
Answers
Answer:
$ 51.36
Step-by-step explanation:
To find the sales tax multiply the tax rate by the cost of the product and then add to the cost.
Sales tax = tax rate * cost price
= 7% * 48
= 0.07 * 48
= $ 3.36
Cost price = cost of product + sales tax
= 48 + 3.36
= $ 51.36
=> Total price = 48 + 3.36
= 1284/25 or 51.36
Can you help please please helps

Answers
Answer:
2nd option
Step-by-step explanation:
Given
- np - 6 ≤ 3(c - 5) ← distribute
- np - 6 ≤ 3c - 15 ( add 6 to both sides )
- np ≤ 3c - 9
Divide both sides by - p , reversing the symbol as a result of dividing by a negative quantity.
n ≥ \(\frac{3c-9}{-p}\)
At what point (x,y) is the function f(x) = 2 - 8x closest to the origin? Enter an exact answer. Provide your answer below:
Answers
The function f(x) = 2 - 8x is closest to the origin at the point (0.25, 1.5).
Let's calculate the distance using the formula d = sqrt(x^2 + y^2)
Substitute the value of y in terms of x in the above distance formula, we get d = sqrt[x^2 + (2 - 8x)^2]
Simplify this expression d = sqrt[x^2 + 4 - 32x + 64x^2]d = sqrt[65x^2 - 32x + 4]
To find the minimum value of d, we will find its derivative with respect to x
d' = (65x^2 - 32x + 4)^1/2 - (130x - 32)x / (2 * (65x^2 - 32x + 4)^1/2)
Equating the above derivative to 0 we get, 130x - 32 = 0x = 32/130 = 0.246
Let's find the corresponding y value using the function: f(x) = 2 - 8x
Substituting x = 0.246 we get y = f(0.246) = 1.508.
Thus the function f(x) = 2 - 8x is closest to the origin at the point (0.25, 1.5).
Learn more about distance formula here:
https://brainly.com/question/25841655
#SPJ11
plzzzzz help ill mark you plzzzzzzz

Answers
Answer:
Step-by-step explanation:
a. 5 3/6 - 1 2/6 = 4 1/6
b. 8 9/12 - 5 10/12
7 21/12 - 5 10/12= 2 11/12
A: 5 1/2 - 1 1/3.
Answer: 25/6.
in mix fraction ot would be, 4 1/6
and in decimal from it will be, 4.166667.
B: 8 3/4 - 5 5/6.
Answer: 35/12.
In mix fraction it will be, 2 11/12.
and in decimal from it would be, 2.916667.
Use the segment addition postulate to write three equations using the diagram below

Answers
Answer:
\( PQ + QR = PR \) => equation 1
\( RS + ST = RT \) => equation 2
\( PR + RT = PT \) => equation 3
Step-by-step explanation:
Points P, Q, R, S, and T are collinear therefore, the following equations can be written based on the segment addition postulate:
\( PQ + QR = PR \) => equation 1
\( RS + ST = RT \) => equation 2
\( PR + RT = PT \) => equation 3
More equations can actually be written from the diagram given using the segment addition postulate. Such as:
\( PQ + QR + RS + ST = PT \)
Please help asap……..

Answers
Answer:
1. 96
2. 84
Step-by-step explanation:
I used the Circle theorems:
For 1 you use the alternate segment theory
For 2 you use the theory that opposite angles in a cyclic quadrilateral add up to 180.
I can't be asked to explain it properly I'm sorry but if no one else does it properly you can give me brainliest?
pls anwserr my quiz accurite NO EXPLIMATON PLS

Answers
Jacob and Poppy bought petrol from different petrol
stations.
a) Was Jacob's petrol or Poppy's petrol better value for
money?
b) How much would 20 litres of petrol cost from the
cheaper petrol station?
Give your answer in pounds (£).
Jacob
£18.90 for 14 litres
of petrol
1
Poppy
£22.10 for 17 litres
of petrol
Answers
a) Poppy's petrol was better value for money as it cost less per liter. b) 20 liters of petrol from the cheaper petrol station (Poppy's petrol station) would cost £26.00.
How to determine if Jacob's petrol or Poppy's petrol better value for moneya) To determine which petrol was better value for money, we need to calculate the price per liter for each petrol station:
Jacob's petrol: £18.90 / 14 litres = £1.35 per litre
Poppy's petrol: £22.10 / 17 litres = £1.30 per litre
Therefore, Poppy's petrol was better value for money as it cost less per litre.
b) To calculate the cost of 20 litres of petrol from the cheaper petrol station, we need to determine which petrol station was cheaper:
Jacob's petrol: £1.35 per litre x 20 litres = £27.00
Poppy's petrol: £1.30 per litre x 20 litres = £26.00
Therefore, 20 litres of petrol from the cheaper petrol station (Poppy's petrol station) would cost £26.00.
Learn more about word problem at https://brainly.com/question/21405634
#SPJ1
nate and lane share a 18-ounce bucket of clay. by the end of the week, nate has used 1 6 of the bucket, and lane has used 2 3 of the bucket of clay. how many ounces are left in the bucket?
Answers
Nate and lane share a 18-ounce bucket of clay. by the end of the week, Nate has used 1 6 of the bucket, and lane has used 2 3 of the bucket of clay. Therefore, there are 3 ounces of clay left in the bucket.
To find the number of ounces left in the bucket, we need to subtract the amounts used by Nate and Lane from the total capacity of the bucket.
Nate has used 1/6 of the bucket, which is (1/6) * 18 ounces = 3 ounces.
Lane has used 2/3 of the bucket, which is (2/3) * 18 ounces = 12 ounces.
To find the remaining clay in the bucket, we subtract the total amount used from the total capacity:
Remaining clay = Total capacity - Amount used
Remaining clay = 18 ounces - (3 ounces + 12 ounces)
Remaining clay = 18 ounces - 15 ounces
Remaining clay = 3 ounces
Therefore, there are 3 ounces of clay left in the bucket.
Learn more about subtract here:
https://brainly.com/question/13619104
#SPJ11
Complete the square
x^2 - 2x - 14 = 0
Answers
To complete the square for the equation x^2 - 2x - 14 = 0, follow these steps:
1. Move the constant term to the right side of the equation: x^2 - 2x = 14
2. Take half of the coefficient of x, square it, and add it to both sides of the equation: x^2 - 2x + 1 = 15
3. Factor the left side of the equation as a perfect square: (x - 1)^2 = 15
4. Take the square root of both sides of the equation, remembering to include both the positive and negative square roots: x - 1 = ±√15
5. Add 1 to both sides of the equation to isolate x: x = 1 ± √15
Therefore, the solutions to the equation x^2 - 2x - 14 = 0 are x = 1 + √15 and x = 1 - √15.
3 2/3 divided by 2/5
Answers
Answer:
9 1/6
Step-by-step explanation:
3 2/3 divided by 2/3
First convert 3 2/3 into a whole number
3 2/3 = 11/3
11/3 x 2/3 = 55/3
55/3 into a mixed number is 9 1/6
#answer please with work

Answers
=
x
2
+
8
x
+
6
Pls mark brainliest
What is the slope of the line X+ Зу = 10
Answers
Answer:
\(-\frac{1}{3}\) is the slope
Step-by-step explanation:
Slope intercept form: \(y=-\frac{1}{3}x+\frac{10}{3}\)

find the equation of the line

Answers
Answer:
\(\mathsf{y=-\dfrac13x+5}\)
Step-by-step explanation:
Slope-intercept form of a linear equation: \(\mathsf{y=mx+b}\)
(where m is the slope and b is the y-intercept)
From inspection of the graph, the y-intercept is at (0, 5)
Therefore, b = 5
Choose another point on the line, e.g. (3, 4)
Now use the slope formula to find the slope:
\(\mathsf{slope=\dfrac{y_2-y_1}{x_2-x_1}}\)
where:
\(\mathsf{(x_1,y_1)=(0,5)}\)\(\mathsf{(x_2,y_2)=(3,4)}\)\(\implies \mathsf{slope=\dfrac{5-4}{0-3}=-\dfrac13}\)
Therefore, the equation of the line is:
\(\mathsf{y=-\dfrac13x+5}\)
Answer:
\(y=-\frac{1}{3} + 5\)
Step-by-step explanation:
Pick two points (0,5) & (3,4) find the slope \(m= \frac{y_{2} - y_1 }{x_2 - x_1}\) >> \(m = \frac{4-5}{3-0} = -\frac{1}{3}\)Find y-intercept (where x is 0) >> y = 5The following figure is made of 3 triangles and 1 rectangle.
4
2
B
Figure
Triangle A
Triangle B
Rectangle C
Triangle D
Whole figure
A
2
4
T
6
2C 2D
H
2
Find the area of each part of the figure and the whole figure.
Area (square units)
19
1
I
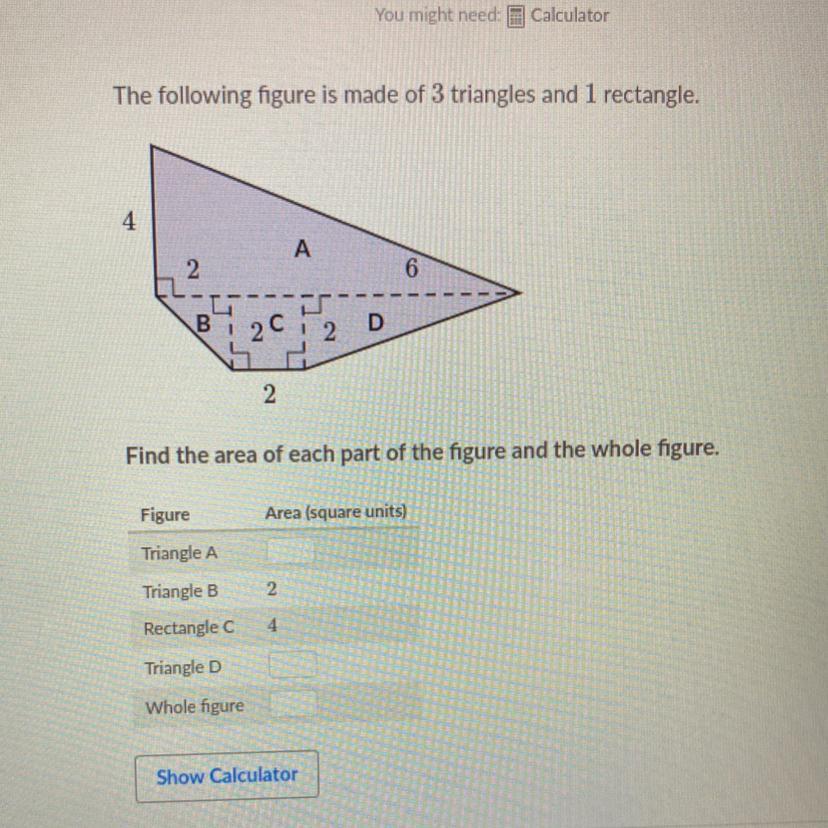
Answers
Answer:
Shape Area (units^2)
A 20
B 2
C 4
D 6
Total = 32
Step-by-step explanation:
See the attached worksheet. These calculations assume that the "6" is the length of the line segment as marked. Using the expressions for areas or traingles and rectangles, as noted, each area is calculated and the sum is 32 units^2.

The question is attached

Answers
a). The distance between A and C is derived to be 42° to the nearest degree.
b). The bearing of the point C with respect to A is equal to 72°
What is bearing?Bearing is usually measured in degrees, with 0° indicating the reference direction (usually North), and increasing clockwise to 360°. It refers to the direction or angle between a reference direction and a point or object.
Let ∆ABC be the triangle formed with bearings so that:
angle B = 30° + 90° + 15°
angle B = 135°
AB = 20km
BC = 25km
a). The distance between A and C is calculated using the cosine rule as follows:
AC² = 20² + 25² - 2(20)(25)cos135° {cosine rule}
AC² = 1025 + 707.1068
AC = √1732.1068 {take square root of both sides}
AC = 41.6186 approximately 42°
b). The bearing of the point C with respect to A is the angle from the north of A to the line AC, so;
bearing of the point C with respect to A = 30° + 42° = 72°
Therefore, the distance between A and C is derived to be 42° to the nearest degree and the bearing of the point C with respect to A is equal to 72°
Read more about bearing here:https://brainly.com/question/22518031
#SPJ1
A health researcher conducted a survey and recorded the percentage of body fat of 20 randomly selected men from two different towns. Ten of the men surveyed were from Pythagoria and 10 of the men were from Bernoullia. The following statistical information was calculated from the researcher's findings.
Answers
The statistical information from the survey conducted by the health researcher includes measures of central tendency and variability for the percentage of body fat among the 20 randomly selected men from Pythagoria and Bernoullia.
The measures of central tendency include the mean and median percentage of body fat for each town. The measures of variability include the range and standard deviation of the percentage of body fat for each town.
The mean percentage of body fat for the 10 men from Pythagoria was found to be 22%, while the median percentage was 23%. For the 10 men from Bernoullia, the mean percentage of body fat was found to be 20%, while the median percentage was 19%. This suggests that, on average, the men from Pythagoria had a slightly higher percentage of body fat than the men from Bernoullia.
The range of the percentage of body fat for the 10 men from Pythagoria was found to be 12%, ranging from 14% to 26%. The range for the 10 men from Bernoullia was found to be 19%, ranging from 8% to 27%. This suggests that the men from Bernoullia had a wider range of percentage of body fat compared to the men from Pythagoria.
The standard deviation of the percentage of body fat for the 10 men from Pythagoria was found to be 5.2%, while the standard deviation for the 10 men from Bernoullia was found to be 7.8%. This indicates that the percentage of body fat among the men from Bernoullia was more spread out compared to the men from Pythagoria.
Overall, the statistical information from the survey provides insights into the distribution of percentage of body fat among the 20 randomly selected men from the two towns.
To learn more about percentage, click here: brainly.com/question/26352729
#SPJ11
pls help will give brainliest!!

Answers
Answer:
a . 50
Step-by-step explanation:
L + 40 + 90 = 180
L + 130 = 180
L = 180 - 130
L=50
find all points having an x-coordinate of calculator
Answers
To find all points with a specific x-coordinate, you need to have the equation of the curve or the data points representing the graph. If you have an equation, you can substitute the desired x-coordinate into the equation and solve for the corresponding y-coordinate.
If you have data points, you can look for the points that have the specified x-coordinate.
For example, let's say you have the equation of a line: y = 2x + 3. If you want to find all points with an x-coordinate of 5, you can substitute x = 5 into the equation to find y. In this case, y = 2(5) + 3 = 13. So the point (5, 13) has an x-coordinate of 5.
to find points with a specific x-coordinate, you need the equation of the curve or the data points. You can substitute the desired x-coordinate into the equation or look for the points that have the specified x-coordinate in the given data.
To know more about curve follow the link:
https://brainly.com/question/31012623
#SPJ11
y = 3/2x + 2
y = 2/3x + 6
These lines are...
Answers
Answer:
As the lines are neither parallel nor perpendicular.
Therefore, These lines are neither parallel nor perpendicular.
Step-by-step explanation:
The slope-intercept form of the line equation
\(y = mx+b\)
where
m is the slopeb is the y-interceptGiven the lines
y = 3/2x + 2y = 2/3x + 6y = 3/2x + 2
Finding the slope of y = 3/2x + 2
comparing with the slope-intercept form of the line equation
The slope m₁ = 3/2
y = 2/3x + 6
Finding the slope of y = 2/3x + 6
comparing with the slope-intercept form of the line equation
The slope m₂ = 2/3
So,
m₁ = 3/2
m₂ = 2/3
We know that when two lines are parallel, they have equal slopes
But
m₁ ≠ m₂
3/2 ≠ 2/3
As the m₁ and m₂ are not equal.
Hence, the lines are not parallel.
We know that when two lines are perpendicular, the product of their slopes is -1.
Let us check the product of two slopes m₁ and m₂
m₁ × m₂ = 3/2 × 2/3
= 6/6
= 1
As
m₁ × m₂ ≠ -1
Thus, the lines are not perpendicular.
Conclusion:
As the lines are neither parallel nor perpendicular.
Therefore, These lines are neither parallel nor perpendicular.
Hi. I need help with these questions.
See image for question.

Answers
Step-by-step explanation:
Given
f(x) = 4x³ + 3x² - 2x - 1Divide it by the following:
(a) 2x + 1
4x³ + 3x² - 2x - 1 =(4x³ + 2x²) + (x² + 1/2x) - (5/2x + 5/4) + 1/4 =2x²(2x+1) + 1/2x(2x + 1) - 5/4(2x + 1) + 1/4 =(2x + 1)(2x² + 1/2x - 5/4) + 1/4Quotient = 2x² + 1/2x - 5/4
Remainder = 1/4
(b) 2x - 3
4x³ + 3x² - 2x - 1 = (4x³ - 6x²) + (9x² - 13.5x) + (11.5x - 17.25) + 16.25 = (2x -3)(2x² + 4.5x + 5.75) + 16.25Quotient = 2x² + 4.5x + 5.75
Remainder = 16.25
(c) 4x - 1
4x³ + 3x² - 2x - 1 = (4x³ - x²) + (4x² - x) - (2x - 1/2) - 3/2 =(4x - 1)(x² + x - 1/2) - 3/2Quotient = x² + x - 1/2
Remainder = - 3/2
(d) x + 2
4x³ + 3x² - 2x - 1 = (4x³ + 8x²) - (5x² + 10x) + (8x + 16) - 17 =(x + 2)(4x² - 5x + 8) - 17Quotient = 4x² - 5x + 8
Remainder = - 17
Step-by-step explanation:
Given
f(x) = 4x³ + 3x² - 2x - 1
Divide it by the following:
(a) 2x + 1
4x³ + 3x² - 2x - 1 =
(4x³ + 2x²) + (x² + 1/2x) - (5/2x + 5/4) + 1/4 =
2x²(2x+1) + 1/2x(2x + 1) - 5/4(2x + 1) + 1/4 =
(2x + 1)(2x² + 1/2x - 5/4) + 1/4
Quotient = 2x² + 1/2x - 5/4
Remainder = 1/4
(b) 2x - 3
4x³ + 3x² - 2x - 1 =
(4x³ - 6x²) + (9x² - 13.5x) + (11.5x - 17.25) + 16.25 =
(2x -3)(2x² + 4.5x + 5.75) + 16.25
Quotient = 2x² + 4.5x + 5.75
Remainder = 16.25
(c) 4x - 1
4x³ + 3x² - 2x - 1 =
(4x³ - x²) + (4x² - x) - (2x - 1/2) - 3/2 =
(4x - 1)(x² + x - 1/2) - 3/2
Quotient = x² + x - 1/2
Remainder = - 3/2
(d) x + 2
4x³ + 3x² - 2x - 1 =
(4x³ + 8x²) - (5x² + 10x) + (8x + 16) - 17 =
(x + 2)(4x² - 5x + 8) - 17
Quotient = 4x² - 5x + 8
Remainder = - 17
What is an inequality that represents the graph below

Answers
Answer:
y ≥ 4x - 6
Step-by-step explanation:
y = mx + b b=-6
pass (2,2) 2 = 2m - 6
m = 4
line y = 4x -6
equation: y ≥ 4x - 6
You and your best friend want to take a vacation to Australia. You have done some research and discovered that it will cost $2500 for the plane tickets, all-inclusive hotel and resort, and souvenirs. You have already saved $2200. If you invest this money in a savings account with a 1. 55% interest rate compounded annually, how long will it take to earn enough money to go on the trip? Use the compound interest formula A = P (1 + i)n, where A is the accumulated amount, P is the principal, i is the interest rate per year, and n is the number of years. Round your final answer to the nearest tenth
Answers
It will take approximately 4.4 years to earn enough money to go on the trip if we invest our 2200 in a savings account with a 1.55% interest rate compounded annually.
First, we need to calculate the amount of money that we need to save in order to cover the cost of the trip. This can be done by subtracting the amount we have already saved from the total cost of the trip:
Total cost of trip = 2500
Amount already saved = 2200
Amount to save = 2500 - 2200 = 300
Next, we can use the compound interest formula to calculate how long it will take to earn 300 with an interest rate of 1.55% compounded annually. We can set up the formula as follows:
A = P(1 + i)n
where:
A = accumulated amount = 300 + 2200 = 2500 (the total cost of the trip)
P = principal = 2200
i = interest rate per year = 1.55%
n = number of years we need to save for
We can now solve for n:
2500 = 2200(1 + 0.0155)n
Divide both sides by 2200:
1.13636 = 1.0155n
Take the natural logarithm of both sides:
ln(1.13636) = n ln(1.0155)
Divide both sides by ln(1.0155):
n = ln(1.13636)/ln(1.0155) ≈ 4.4 years
Therefore, it will take approximately 4.4 years to earn enough money to go on the trip if we invest our 2200 in a savings account with a 1.55% interest rate compounded annually.that we rounded our final answer to the nearest tenth as instructed.
for such more question on compound interest
https://brainly.com/question/24274034
#SPJ4
Help me please thanu you sm

Answers
Step-by-step explanation:
now you should be able to dive these things yourself.
A no
(1/4)v = 4
4×(1/4)v = 4×4
v = 16
this is not v = 4
B no
n + 4 = 25
n + 4 - 4 = 25 - 4
n = 21
this is not n = 17
C yes
2z = 28
2z/2 = 28/2
z = 14
Subtract -5 4/5 - (-7 2/5). Reduce if possible
Answers
Answer:
1 3/5 after it is reduced
Step-by-step explanation:
Guys, I’m back from nearly a year later went on hiatus on The Brainly because of myself as an anxiety and a very stressful year with A.D.H.D., and I really need help from my own schoolwork from my own school about, “A Perimeter Of The Composite Figures” with only 2 more perimeter questions left to go as soon as possible before it’s too late, please! :O
Please read it as soon as possible before answering to 2 of my own perimeter questions and thank you guys. :)
There’s only 55 points for you to answer to my own 2 of my own perimeter questions, guys! :D
Well good luck, guys! :D

Answers
Answer:
2. 26.2 m
3. 117.2 cm
Step-by-step explanation:
You want the perimeters of two figures involving that are a composite of parts of circles and parts of rectangles.
2. Semicircular archThe circumference of a circle is given by ...
C = πd . . . . . where d is the diameter
The length of the semicircle of diameter 12.6 m will be ...
1/2C = 1/2(π)(12.6 m) = 6.3π m ≈ 19.8 m
The two lighted sides of the rectangle have a total length of ...
3.2 m + 3.2 m = 6.4 m
The length of the light string is the sum of these values:
19.8 m + 6.4 m = 26.2 m
The length of the string of lights is about 26.2 meters.
3. Fan shapeThe perimeter of the figure is the sum of four quarter-circles of radius 11.4 cm, and 4 straight edges of length 11.4 cm.
Four quarter-circles total one full circle in length, so we can use the formula for the circumference of a circle:
C = 2πr
C = 2π·(11.4 cm) = 22.8π cm ≈ 71.6 cm
The four straight sides total ...
4 × 11.4 cm = 45.6 cm
The perimeter of the figure is the sum of the lengths of the curved sides and the straight sides:
71.6 cm + 45.6 cm = 117.2 cm
The design has a perimeter of about 117.2 cm.
__
Additional comment
The bottom 12.6 m edge in the figure of problem 2 is part of the perimeter of the shape, but is not included in the length of the light string.
<95141404393>
What is the difference quotient for the function f(x) = -1/5x-12?

Answers
The difference quotient for the function \(f(x) = -\frac{1}{5x-12}\) is \(\frac{5}{(5x+5h-12)(5x-12)};\) h≠0
Option B) is the correct answer.
What is the difference quotient for \(f(x) = -\frac{1}{5x-12}\) ?Given that;
\(f(x) = -\frac{1}{5x-12}\)difference quotient for f(x) = ?We know that difference quotient is expressed as;
\(\frac{f(x+h)-f(x)}{h}\)
Next, substitute x+h for x.
Hence;
\(f(x+h) = -\frac{1}{5(x+h)-12}\)
Now,
\(\frac{f(x+h)-f(x)}{h} = \frac{-\frac{1}{5(x+h)-12}-(-\frac{1}{5x-12}) }{h} \\\\= \frac{5}{(5x-12)(5h+5x-12)}\\\\= \frac{5}{(5x+5h-12)(5x-12)};\)h≠0
The difference quotient for the function \(f(x) = -\frac{1}{5x-12}\) is \(\frac{5}{(5x+5h-12)(5x-12)};\) h≠0
Option B) is the correct answer.
Learn more about difference quotient: https://brainly.com/question/18270597
#SPJ1