If OM=103 MN=108 ON=84 PQ=54 RQ=42 find the perimeter of pqr

based on the given information, we can definitively conclude that PV- is closer to 0 than to 1. The option (b) "PV- is closer to 0 than to 1" is the correct answer.

The present value (PV) represents the current value of a future cash flow or investment. If PV is approximately 1, it means that the value of the future cash flow or investment is close to its full value in the present.

When we subtract a value from PV to get PV-, the resulting value will be smaller than PV. Since PV is approximately 1, it implies that PV- is closer to 0 than to 1. This conclusion holds because subtracting a value from 1 will always yield a smaller value.

For example, if PV is exactly 1 and we subtract 0.5 from it, the resulting PV- will be 0.5, which is closer to 0 than to 1.

Learn more about present value here:

https://brainly.com/question/28304447

#SPJ11

(x, y) is an element of the set c × d, since x is an element of c and y is an element of d.

Since (x, y) was an arbitrary element in a × b, we can conclude that every element in a × b is also in c × d. Thus, we have shown that if a ⊆ c and b ⊆ d, then a × b ⊆ c × d.

To show that if a ⊆ c and b ⊆ d, then a × b ⊆ c × d, follow these steps:

Step 1: Understand the notation.
a ⊆ c means that every element in set a is also in set c.
b ⊆ d means that every element in set b is also in set d.

Step 2: Consider the Cartesian products.
a × b is the set of all ordered pairs (x, y) where x ∈ a and y ∈ b.
c × d is the set of all ordered pairs (x, y) where x ∈ c and y ∈ d.

Step 3: Show that a × b ⊆ c × d.
To prove this, we need to show that any ordered pair (x, y) in a × b is also in c × d.

Let (x, y) be an arbitrary ordered pair in a × b. This means that x ∈ a and y ∈ b.

Since a ⊆ c, we know that x ∈ c because every element in set a is also in set c.
Similarly, since b ⊆ d, we know that y ∈ d because every element in set b is also in set d.

Now, we have x ∈ c and y ∈ d, so the ordered pair (x, y) belongs to c × d.

Step 4: Conclusion
Since any arbitrary ordered pair (x, y) in a × b also belongs to c × d, we can conclude that a × b ⊆ c × d.

Learn more about Set:

brainly.com/question/28492445

#SPJ11

Answer:

y=3x^2+34

3x+y-10=0

Step-by-step explanation:

Answer:

  5.25 m

Step-by-step explanation:

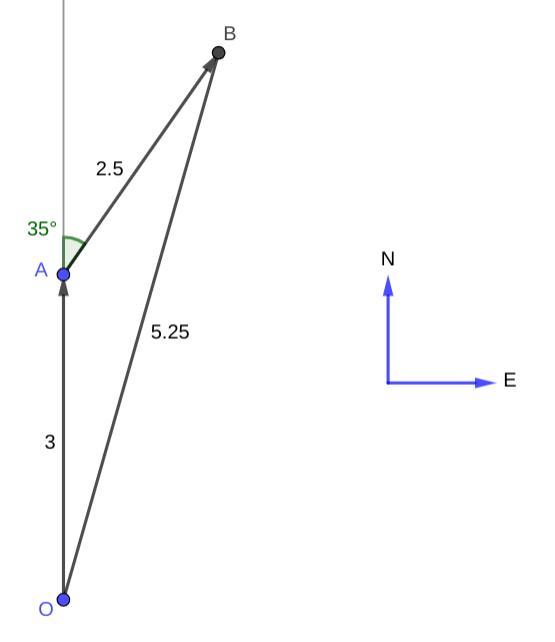
A diagram can help you understand the question, and can give you a clue as to how to find the answer. A diagram is attached. The problem can be described as finding the sum of two vectors whose magnitude and direction are known.

__

In navigation problems, direction angles are specified a couple of different ways. A bearing is usually an angle in the range [0°, 360°), measured clockwise from north. In land surveying and some other applications, a bearing may be specified as an angle east or west of a north-south line. In this problem we are given the bearing of the second leg of the walk as ...

  N 35° E . . . . . . . 35° east of north

Occasionally, a non-standard bearing will be given in terms of an angle north or south of an east-west line. The same bearing could be specified as E 55° N, for example.

A vector is a mathematical object that has both magnitude and direction. It is sometimes expressed as an ordered pair: (magnitude; direction angle). It can also be expressed using some other notations;

In the latter case, "cis" is an abbreviation for the sum cos(θ)+i·sin(θ), where θ is the direction angle.

Sometimes a semicolon is used in the polar coordinate ordered pair to distinguish the coordinates from (x, y) rectangular coordinates.

__

The first leg of the walk is 3 meters due north. The angle from north is 0°, and the magnitude of the distance is 3 meters. We can express this vector in any of the ways described above. One convenient way is 3∠0°.

The second leg of the walk is 2.5 meters on a bearing 35° clockwise from north. This leg can be described by the vector 2.5∠35°.

The final position is the sum of these two changes in position:

  3∠0° +2.5∠35°

Some calculators can compute this sum directly. The result from one such calculator is shown in the second attachment:

  = 5.24760∠15.8582°

This tells you the magnitude of the distance from the original position is about 5.25 meters. (This value is also shown in the first attachment.)

__

You may have noticed that adding two vectors often results in a triangle. The magnitude of the vector sum can also be found using the Law of Cosines to solve the triangle. For the triangle shown in the first attachment, the Law of Cosines formula can be written as ...

  a² = b² +o² -2bo·cos(A) . . . . where A is the internal angle at A, 145°

Using the values we know, this becomes ...

  a² = 3² +2.5² -2(3)(2.5)cos(145°) ≈ 27.5373

  a = √27.5373 = 5.24760 . . . . meters

The distance from the original position is about 5.25 meters.

_____

Additional comment

The vector sum can also be calculated in terms of rectangular coordinates. Position A has rectangular coordinates (0, 3). The change in coordinates from A to B can be represented as 2.5(sin(35°), cos(35°)) ≈ (1.434, 2.048). Then the coordinates of B are ...

  (0, 3) +(1.434, 2.048) = (1.434, 5.048)

The distance can be found using the Pythagorean theorem:

  OB = √(1.434² +5.048²) ≈ 5.248

Using proportions, it is found that it will take you 392 seconds = 6 minutes and 32 seconds to complete 4 laps.

A proportion is a fraction of a total amount, and the measures are related using a rule of three.

In this problem, considering the given rates, the time that is takes to complete one lap of 400 m is given by:

t = 2 x 12(sprint) + 24(half as fast as the sprint, 24 seconds per 100 meters) + 50 = 98 seconds.

Hence for 4 laps, the time is given by:

t = 4 x 98 seconds = 392 seconds = 6 minutes and 32 seconds to complete 4 laps.

More can be learned about proportions at https://brainly.com/question/24372153

#SPJ1

Taxpayers in California may need to calculate the itemized deduction limitation when filing their state income taxes. This limitation sets a cap on the amount of itemized deductions that can be claimed, based on the taxpayer's federal adjusted gross income (AGI) and other factors.

Calculating the California itemized deduction limitation involves several steps and considerations to ensure compliance with the state tax regulations. To calculate the California itemized deduction limitation, taxpayers should first determine their federal AGI. This can be found on their federal tax return. Next, they need to identify any federal deductions that are not allowed for California state tax purposes, as these will be excluded from the calculation. Once the applicable deductions are determined, taxpayers must compare their federal AGI to the threshold specified by the California Franchise Tax Board (FTB). The limitation is typically a percentage of the federal AGI, and the percentage may vary depending on the taxpayer's filing status. If the federal AGI exceeds the threshold, the itemized deductions will be limited to the specified percentage. Taxpayers should consult the official guidelines and instructions provided by the California FTB or seek professional tax advice to ensure accurate calculation and compliance with the state tax regulations. Calculating the California itemized deduction limitation is an important step in accurately reporting and calculating state income taxes. It helps determine the maximum amount of itemized deductions that can be claimed, ensuring that taxpayers adhere to the tax laws and regulations of the state.

Learn more about  adjusted gross income (AGI)  here:

https://brainly.com/question/30320694

#SPJ11

The equation of the tangent line to the curve y = ln(x^2 - 5x + 1) at the point (5,0) is y = 0.

To find the equation of the tangent line to the curve y = ln(x^2 - 5x + 1) at the point (5,0), we need to find the slope of the tangent line at that point.

The derivative of y = ln(x^2 - 5x + 1) is:

y' = (2x - 5)/(x^2 - 5x + 1)

At the point (5,0), we have:

y' = (2(5) - 5)/(5^2 - 5(5) + 1) = 0

So the slope of the tangent line at (5,0) is 0.

The equation of the tangent line can be written as:

y - y1 = m(x - x1)

where (x1, y1) is the point of tangency and m is the slope.

Since the slope is 0, we have:

y - 0 = 0(x - 5)

which simplifies to:

y = 0

Therefore, the equation of the tangent line to the curve y = ln(x^2 - 5x + 1) at the point (5,0) is y = 0.

Learn more about tangent line here

https://brainly.com/question/30162650

#SPJ11

Class limits indicate the largest and smallest data values that can be included in the class. Class limits are referred to actual data values. On the other hand, class boundaries give values that remove gaps between the classes in the frequency distribution. Class boundaries are considered to be one decimal place more accurate in comparison to the data. Options A, B, D, and F correctly describe differences between a class boundary and a class limit.

Following are the potential differences between a class boundary and a class limit.

You can learn more about class boundary and a class limit at

https://brainly.com/question/13646092

#SPJ4

Answer:

20 goody bags

Step-by-step explanation:

To solve problems like this, find the GCF of the two numbers.

Answer:

300-21=279

Step-by-step explanation:

The value of the intercept of the regression line, b, rounded to one decimal place, is 8.1.

To find the intercept of the regression line, we need to perform linear regression analysis on the given data. The regression line is an equation of the form y = mx + b, where m is the slope and b is the intercept.

We can use a statistical software or a calculator to perform linear regression analysis. Here, we will use Microsoft Excel to find the intercept of the regression line.

First, we will create a scatter plot of the data. Then, we will add a trendline and display the equation of the trendline on the chart.

After performing linear regression analysis on the given data, we get the equation of the regression line as:

y = 1.9444x + 8.1389

Here, the intercept of the regression line is the value of b, which is 8.1389. Rounding it to one decimal place, we get the intercept as 8.1.

The intercept of the regression line is the point where the regression line intersects with the y-axis. In this context, it represents the predicted value of y when x is equal to zero. In other words, it is the starting point of the regression line.

In this example, the intercept of the regression line indicates that an employee with zero years of experience would be expected to have a salary of $8.1 thousand.

To learn more about regression click on,

https://brainly.com/question/31131102

#SPJ4

Answer:

y=-4/3+2

Step-by-step explanation:

Sin the slope is going up to the left we can already infer that it is going to be a negative. Still we do the equation y2-y1/x2-x1. In this case 0 is x1 and 2 is y1, 3 is x2, and -2 is y2. Once inserted into the equation you get -2-2/3-0 which equals -4/3 and the y intercept is 2 since thats the point shown were it intercepts the y-axis

Answer:

8.533333

Step-by-step explanation:

9^(− 1/ 2 )+25− 1 2 +32^ (3/ 5) = 9 (−1 /2) +25(− 1 /2) +32 ^(3 /5)

=0.333333+25^(− 1/ 2) +32 ^(3 /5)

=0.333333+25 ^(−1 /2) +32 ^(3/ 5)

=0.333333+0.2+32^ (3/ 5)

=0.533333+32 ^(3 /5)

=0.533333+8

=8.533333

Answer:

6 Hours

Step-by-step explanation:

No.of leaflets---no.of people---work hours

1000 ————. 4. - — — — — — — 5

1500 — — — —5 — — — — — ,— —?

If work increase, no.of people needed more

So, the required ratio is 1500/1000

If work men increase, no of hours decrease

So, the required ratio is 4/5

(1500/1000)× (4/5)×5 = 6

No.of hours required to distribute 1500 leaflets by 5 people = 6 hours

Answer:

x = 23

Step-by-step explanation:

4x + 8 - 8 = 100 - 8

4x = 92

(4x) / 4 = 92 / 4

x = 23

Answer:

x = 23

Step-by-step explanation:

4x + 8 = 100 ( subtract 8 from both sides )

4x = 92 ( divide both sides by 4 )

x = 23

Total number of men and women wearing sunglasses = 290

According to the question,

Total number of men and women = 2000

half of the adults were women = 1/2 × 2000

Number of women = 1000

Number of men = (Total adults) - (Number of women )

Number of men = 2000 - 1000

= 1000

given,

20% of women were wearing sunglasses = 20/100 × 1000

= 200

9% of men were wearing sunglasses = 9/100 × 1000

= 90

Total number of adults wearing glasses = 200 + 90

= 290

In mathematics, a percentage is a number or ratio that represents a fraction of 100. It is often denoted by the symbol "%" or simply as "percent" or "pct." For example, 35% is equivalent to the decimal 0.35, or the fraction.

To learn more about percentage from the given link

https://brainly.com/question/24304697

#SPJ4

The general solution of the associated homogeneous differential equation \(y'' + 2y' + 2y = 0\) is given by

             \(y_h = c₁ e^(-x) cos(x) + c₂ e^(-x) sin(x)\)

We can use the method of undetermined coefficients or variation of parameters to find y_p, depending on the form of g(x).

For each of problems 4 through 9, we need to find the general solution of the given differential equation.

Problem:

             \(4y'' + 4y' + 13y = 0\)

By solving the auxiliary equation \(r² + 4r + 13 = 0,\)

we get

         \(r = -2 + 3i, -2 - 3i.\)

Hence, the general solution is

          \(y = c₁ e^(-2x) cos(3x) + c₂ e^(-2x) sin(3x)\)

Problem: \(5y'' + 4y' + 3y = 0\)

By solving the auxiliary equation \(r² + 4r + 3 = 0,\)

we get

          \(r = -2 + √1, -2 - √1.\)

Hence, the general solution is

     \(y = c₁ e^(-x) + c₂ e^(-3x)\)

Problem \(6y'' + y = 0\)

By solving the auxiliary equation \(r² + 1 = 0\),

we get

             r = -i, i.

Hence, the general solution is

           \(y = c₁ cos(x) + c₂ sin(x)\)

Problem\(7y'' - 3y' - 4y = 0\)

By solving the auxiliary equation \(r² - 3r - 4 = 0\),

we get

  r = 4, -1.

Hence, the general solution is

            \(y = c₁ e^(4x) + c₂ e^(-x)\)

Problem \(8y'' + 3y' + 2y = 0\)

By solving the auxiliary equation \(r² + 3r + 2 = 0,\)

we get

              r = -1, -2.

Hence, the general solution is

                  \(y = c₁ e^(-x) + c₂ e^(-2x)\)

Problem:

               \(9y'' + 2y' + 2y = g(x)\)

This is a non-homogeneous differential equation.

The general solution of the associated homogeneous differential equation \(y'' + 2y' + 2y = 0\) is given by

       \(y_h = c₁ e^(-x) cos(x) + c₂ e^(-x) sin(x)\)

For the non-homogeneous equation, the general solution is given by

      \(y = y_h + y_p\)

Where y_p is any particular solution of the non-homogeneous differential equation.

To know more about,non-homogeneous visit

https://brainly.com/question/18271118

#SPJ11

According to the given information in the question the solution of the inequality is x ≤ 4.

The term "inequality" is used in mathematics to describe a relationship between two expressions or values that is not equal to one another. Inequality results from a lack of balance. When two quantities are equal, we use the symbol '=', and when they are not equal, we use the symbol. If two values are not equal, the first value can be greater than (>) or less than (), or greater than equal to () or less than equal to ().

Here the given inequality is ,

=> x+3≤ 7

=> x+3-3≤7-3

=> x ≤ 4

Hence the solution of the inequality is x ≤ 4.

To learn more about inequality refer the below link

brainly.com/question/25275758

#SPJ1

On solving the provided question, we can say that by equation 8 trainees are in group X

An equation is a mathematical formula that connects two assertions using the equal sign (=) to denote equivalence. In algebra, an equation is a mathematical statement that establishes the equivalence of two mathematical expressions. For instance, an equal sign separates the components 3x + 5 and 14 in the equation 3x + 5 = 14. A mathematical formula is used to explain the connection between two sentences on either side of a letter. Frequently, there is just one variable, which is also the symbol. for example, 2x - 4 = 2.

Let a be the number of trainees in group X

76*a + 70 (30 - a) = 30*74

76a + 2,100 - 70a = 2,220

6a = 120

a = 20

To know more about equation visit:

https://brainly.com/question/649785

#SPJ4

No, molar mass is a constant and does not depend on pressure or temperature.

Molar mass is a physical property of a substance and is the mass of one mole of a given substance. It is a constant value that represents the amount of grams of a substance that contains 022 x 1023 atoms or molecules. Since molar mass is a constant, it does not depend on pressure or temperature. Pressure and temperature can affect the physical and chemical properties of a substance, but they do not have an effect on the molar mass. The molar mass of a substance can be useful in determining molecular and empirical formulas, as well as calculating densities and molar concentrations.

Learn more about temperature here

https://brainly.com/question/29768169

#SPJ4

Answer:

6

Step-by-step explanation:

point D = -6

point A = 18

distance between point D and A: 18 - (-6) = 24

midpoint distance = 24/2 = 12 units away from each point

-6 + 12 = 6

18 - 12 = 6

your midpoint is at 6

Answer:

6

Step-by-step explanation:

-6 to 18 is a difference of 24. half of 24 is 12.-6 + 12 is 6 so 6 is halfway between point d and point a

Answer:

gcf of 150 is 150

Step-by-step explanation:

if thats wrong then its 75

\(~~~~~~ \textit{Continuously Compounding Interest Earned Amount} \\\\ A=Pe^{rt}\qquad \begin{cases} A=\textit{accumulated amount}\dotfill & \$ 2500\\ P=\textit{original amount deposited}\\ r=rate\to 7.5\%\to \frac{7.5}{100}\dotfill &0.075\\ t=years\dotfill &8 \end{cases} \\\\\\ 2500 = Pe^{0.075\cdot 8}\implies \cfrac{2500}{e^{0.075\cdot 8}} \implies 1372.03\approx P\)

The scenario described above illustrates the Service Recovery Paradox, where a customer's satisfaction can actually increase after experiencing a service failure and receiving effective recovery efforts.

Dr. Amarkant Tripathi faced a delay and inconvenience with his suit order from B N Rao suits, but the store manager went above and beyond to rectify the situation, ultimately leading to Dr. Tripathi's increased satisfaction and loyalty.

The Service Recovery Paradox occurs when a customer's satisfaction and loyalty increase even after encountering a service failure. In the given context, Dr. Tripathi experienced a delay and fitting issue with his suit order. However, the store manager took prompt action to resolve the problem and ensure Dr. Tripathi's satisfaction.
Despite initial setbacks, the store manager exhibited proactive service recovery efforts. He communicated with Dr. Tripathi, apologized sincerely, and made efforts to rectify the situation promptly. The manager's willingness to listen, understand, and address the customer's concerns played a crucial role.
Furthermore, the store manager's personal involvement, contacting Dr. Tripathi's home, explaining the situation to his daughter, and arranging for the suit to be taken to the Domlur branch for repairs and express delivery showcased a high level of customer service and dedication to resolving the issue.
These service recovery efforts exceeded Dr. Tripathi's expectations and demonstrated B N Rao suits' commitment to customer satisfaction. As a result, when Dr. Tripathi received the suit, along with the apology note and additional handkerchiefs, he felt not only satisfied with the resolution but also impressed by the store's efforts to understand his needs and deliver a superior service experience.
The Service Recovery Paradox, in this case, highlights the importance of effective service recovery in building customer loyalty and turning a potentially negative experience into a positive one. B N Rao suits' commitment to resolving the issue and going the extra mile to deliver a satisfactory outcome ultimately strengthened the customer's trust and loyalty in the brand.

Learn more about Service Recovery paradox here
https://brainly.com/question/32533243



#SPJ11

The equation of the line passing through (9,11) and perpendicular to the line passing through (6,1) and (2,1) is x = 6

Find the slope of the line passing through (6,1) and (2,1):

The slope of a line passing through two points (x1,y1) and (x2,y2) is given by:

slope = (y2 - y1) / (x2 - x1)

Substituting the given coordinates, we get:

slope = (1 - 1) / (2 - 6) = 0

Therefore, the slope of the line passing through (6,1) and (2,1) is 0.

Find the slope of the line perpendicular to the line passing through (6,1) and (2,1):

The slope of a line perpendicular to a line with slope m is given by:

perpendicular slope = -1/m

Substituting the slope of the line passing through (6,1) and (2,1), we get:

perpendicular slope = -1/0 (which is undefined)

This means that the line perpendicular to the line passing through (6,1) and (2,1) is a vertical line passing through the point (6,1) and (2,1).

Write the equation of the line passing through (9,11) and perpendicular to the line passing through (6,1) and (2,1):

Since the line passing through (6,1) and (2,1) is a horizontal line with equation y = 1, the line perpendicular to it is a vertical line passing through the point (6,1) and (2,1). The equation of a vertical line passing through a point (a,b) is given by:

x = a

Substituting the value of x = 6 (since the vertical line passes through (6,1) and (2,1)), we get:

x = 6

Learn more about slope of the line here

brainly.com/question/16180119

#SPJ4

Answer: 58 degrees

Step-by-step explanation:

We can see that this triangle is an isosceles triangle so that means the two bottoms angles will be equal

Point S must be equal to point U

z = 58 degrees

Check if this is true:

Angle sum of a triangle: 180 degrees

58 + 58 = 116

180 - 116 = 64

Point T is 64

58 + 58 + 64 = 180

Step-by-step explanation:

To find an equation of a line in point slope form when given the slope and a point we use the formula

\(y - y_1 = m(x - x_1) \)

where

m is the slope

( x1 , y1) is the point

From the question the point is (-3, -1) and slope 2

The equation is

Hope this helps you

1. To identify the surface S, we need to analyze the given equation. However, it seems that the equation is missing, as only "z" is mentioned. Please provide the complete equation for a more accurate identification.

2. Once the equation for surface S is provided, we can find the tangent plane at the point (1, 0, 3). To find the tangent plane, we need the gradient of the surface equation.

The gradient of the surface equation gives the direction of the normal vector to the surface at any point. The equation of the tangent plane can then be written as:

(x - x1)(∂f/∂x) + (y - y1)(∂f/∂y) + (z - z1)(∂f/∂z) = 0,

where (x1, y1, z1) is the given point and (∂f/∂x), (∂f/∂y), and (∂f/∂z) are the partial derivatives of the surface equation with respect to x, y, and z, respectively.

To find the tangent plane at (1, 0, 3), we need the equation of the surface S. Please provide the equation so that we can proceed with the calculation.

Please let me know if you have any further questions or if there's anything else I can assist you with.

The numeric value of ms within is = 19.

To find the numeric value of ms within:

Given: Swithin = 70, sample size = 17 and number of groups = 3.

So, 17 × 3 = 51

70 - 51 = 19

Therefore, the numeric value of ms within is = 19.

Know more about numeric values here:

https://brainly.com/question/27922641

#SPJ4