If x+y=w+z , then prove that AOB is a line.
Answers
Answer:
it was a incomplete question
Related Questions
I know how to do this, but for some reason got it wring on a Test. Can someone demonstrate how to do it so that I know what I'm doing wrong?

Answers
Answer:
Answer on a graph

HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP
HELP I NEED HELP ASAP

Answers
Answer:
\(D.\ (3,\frac{1}{8})\)
Step-by-step explanation:
Given
\(y = (\frac{1}{2})^x\)
Required
Points that lies on the above graph
From the given options, only option D lies on the point and the proof is as follows:
\(D.\ (3,\frac{1}{8})\)
This means that:
\(x = 3;\ y = \frac{1}{8}\)
Substitute \(x = 3;\ y = \frac{1}{8}\) in \(y = (\frac{1}{2})^x\)
\(\frac{1}{8} = (\frac{1}{2})^3\)
\(\frac{1}{8} = \frac{1^3}{2^3}\)
\(\frac{1}{8} = \frac{1}{8}\)
See that the values at the right and left hand side of the equation are the same.
This means that, \(D.\ (3,\frac{1}{8})\) lies on \(y = (\frac{1}{2})^x\)
The Marted out of 10.00 He question Your answer is correct The point with cylindrical coordinates [p. p. 21-12-√3.6 has spherical coordinates [r. 0.p] [sqrt(48), pi/13, pi/6] NOTE: In the text and the lecture notes cylindrical coordinates are denoted by [r..z). So, in this question (p.) are polar coordinates in the the xy-plane. In the text and the lecture notes spherical coordinates are denoted by le, 8, 1, where is the radius, the distance from the origin to the point P, the angle is the angle between positive x-axis and the projection of anto xy-plane, and is the angle between positive - axis anda. Thus, in your answer use for r. 0 for p and for 0. Your last answer was interpreted as follows: [V answer is partially correct. The value of r is correct. The value of is incorrect. The value of op is incorrect. Please try again. You have 2 attempts remaining.
Answers
The shape with a series of parallel cross sections that are congruent circles is a cylinder.
The cross-section that results from cutting a cylinder parallel to its base is a circle that is congruent to all other parallel cross-sections. This is true for any plane that is perpendicular to the cylinder's base. The only shape that has parallel cross-sections that are congruent circles is a cylinder, for this reason.
Two parallel, congruent circular bases that lay on the same plane make up the three-dimensional shape of a cylinder. A curved rectangle connecting the bases makes up the cylinder's lateral surface. Congruent circles are produced when a cylinder is cut in half parallel to its base.
learn more about congruent circles
at brainly.com/question/9337801
#SPJ4
Franklin has delivered 26 newspapers and
can deliver 9 newspapers in an hour.
Michael has delivered 5 newspapers and can
deliver 12 newspapers in an hour. How
many hours (h) will Michael take to deliver
as many newspapers (n) as Franklin?
n = 9h+26
n = 12h + 5
[?] hours
Answers
Using linear functions, it is found that it will take 7 hours for Michael to deliver as many newspapers as Franklin.
What is a linear function?A linear function is modeled by:
y = mx + b
In which:
m is the slope, which is the rate of change, that is, by how much y changes when x changes by 1.b is the y-intercept, which is the value of y when x = 0, and can also be interpreted as the initial value of the function.The linear functions that give the amounts for Franklin and Michael after h hours are given by:
F(h) = 26 + 9h.M(h) = 5 + 12h.They will be equal when:
F(h) = M(h)
Hence:
26 + 9h = 5 + 12h
3h = 21
h = 7
It will take 7 hours for Michael to deliver as many newspapers as Franklin.
More can be learned about linear functions at https://brainly.com/question/24808124
#SPJ1
Find the area of the polygon with vertices P(5,-1), Q(-1,-4), R(-3,1), and S(0,3). If necessary, round
to the nearest tenth.
Answers
Answer:
14.3 sq units
Step-by-step explanation:
this shape is a trapezoid so we need to find height and each parallel base
to find height we can take the midpoint of RS and the midpoint of QP and then find the distance between each midpoint
midpoint of RS = (-3/2,2)
midpoint of QP = (2,-5/2)
distance between the two is 16/2 or 8
d(RS) = √2²+3² = \(\sqrt{13}\)
d(QP) = √3²+6² = √45 = \(\sqrt{9}\)\(\sqrt{5}\) or 3\(\sqrt{5}\)
put values into the formula for area:
A = 1/2(8) + (\(\sqrt{13}\) + 3\(\sqrt{5}\))
A = 4 + (\(\sqrt{13}\) + 3\(\sqrt{5}\))
A ≈ 14.3
evaluate the integral (xy y z)ds, where c is the curve given by: r(t)=2ti tj (2-2t)k.
Answers
The value of the line integral ∫(xy, y, z)·ds along the curve c is 4.
To evaluate the line integral ∫(xy, y, z)·ds, we need to parameterize the curve c and compute the dot product of the vector function (xy, y, z) with the tangent vector ds.
The curve c is given by the vector function r(t) = 2ti + tj + (2 - 2t)k, where 0 ≤ t ≤ 1. This represents a line segment in three-dimensional space.
To find the tangent vector ds, we take the derivative of r(t) with respect to t:
r'(t) = (2i + j - 2k)
Now, let's compute the dot product (xy, y, z)·ds:
(xy, y, z)·ds = (xy, y, z)·r'(t)
Substituting the values of r'(t) into the dot product expression:
(xy, y, z)·r'(t) = (2t)(2)(2) + (2)(1) + (2 - 2t)(-2) = 8t + 2 - 4 + 4t = 12t - 2
To evaluate the integral, we integrate 12t - 2 with respect to t from 0 to 1:
∫[0,1] (12t - 2) dt = [\(6t^2 - 2t\)] evaluated from 0 to 1
Plugging in the values:
\([6(1)^2 - 2(1)\)] - [\(6(0)^2 - 2(0)\)] = 4
Therefore, the value of the line integral ∫(xy, y, z)·ds along the curve c is 4.
For more such questions on curve visit:
https://brainly.com/question/30452445
#SPJ11
As part of an annual review of its accounts, a discount brokerage selects a random sample of 26 customers. Their accounts are reviewed for total account valuation, which showed a mean of $32,700, with a sample standard deviation of $9,000. (Use t Distribution Table.) what is a 98% confidence interval for the mean account valuation of the population of customers? (Round your answers to the nearest dollar amount.) 98% confidence interval for the mean account valuation is and tween
Answers
The 98% confidence interval for the mean account valuation of the population of customers is between approximately $29,086 and $36,314.
To calculate the confidence interval, we can use the t-distribution since the population standard deviation is unknown and the sample size is relatively small (26 customers). With a 98% confidence level, we need to find the critical value from the t-distribution table. For a two-tailed test, the degrees of freedom would be n - 1, which is 26 - 1 = 25.
Using the t-distribution table or statistical software, we find that the critical value for a 98% confidence level with 25 degrees of freedom is approximately 2.787.
Next, we can calculate the margin of error, which is obtained by multiplying the critical value by the standard error of the mean. The standard error of the mean (SE) is given by the sample standard deviation divided by the square root of the sample size. In this case, SE = 9000 / √26 ≈ 1766.088.
The margin of error is then 2.787 * 1766.088 ≈ 4917.936.
Finally, we can construct the confidence interval by subtracting and adding the margin of error to the sample mean. The lower bound of the confidence interval is the sample mean minus the margin of error: 32700 - 4917.936 ≈ 29,086. The upper bound is the sample mean plus the margin of error: 32700 + 4917.936 ≈ 36,314.
Therefore, the 98% confidence interval for the mean account valuation of the population of customers is approximately $29,086 to $36,314.
Learn more about confidence interval here:
https://brainly.com/question/32546207
#SPJ11
f(x)=5x+10 solve when f(x)=20
Answers
When f(x) = 20
Then
5x + 10 = 20
Therefore
5x=20-10=10
x = 10 / 5 = 2
x= 2
Find an equation of the tangent line to the astroid at the (-3√3, 1).
x²/³ + y²/³ = 4
Answers
The equation of the tangent line to the astroid at the point (-3√3, 1) is: y = -(3√3)^(1/3)x - 3(3√3)^(1/3) + 1
To find the equation of the tangent line to the astroid at the point (-3√3, 1), we need to first find the slope of the tangent line.
We can do this by taking the derivative of the equation of the astroid with respect to x, and then evaluating it at the point (-3√3, 1).
Taking the derivative of x²/³ + y²/³ = 4 with respect to x, we get:
(2/3)x^(-1/3) + (2/3)y^(-1/3) * dy/dx = 0
Solving for dy/dx, we get:
dy/dx = (-x^(1/3))/y^(1/3)
Substituting x = -3√3 and y = 1, we get:
dy/dx = (-(-3√3)^(1/3))/(1^(1/3)) = -(3√3)^(1/3)/1
So the slope of the tangent line at the point (-3√3, 1) is -(3√3)^(1/3).
Now we can use the point-slope form of the equation of a line to find the equation of the tangent line. The point-slope form is:
y - y1 = m(x - x1)
Substituting x1 = -3√3, y1 = 1, and m = -(3√3)^(1/3), we get:
y - 1 = -(3√3)^(1/3)(x + 3√3)
Simplifying, the equation of the tangent line to the astroid at the point (-3√3, 1) is:
y = -(3√3)^(1/3)x - 3(3√3)^(1/3) + 1
Learn more about derivatives here: brainly.com/question/25324584
#SPJ11
a.5÷4 divide and show work
Answers
Answer:
1.25
Step-by-step explanation:
1.25
THATS THE ANSWER
✨✨✨✨✨✨✨✨
Twelve freinds go to a resturant together to celebrate a birthday. The food bill is $192.77 and $48.19 is added on for tax and tip. If the friends plan to divide the bill evenly between the 12 people, how much does each person owe
Answers
Answer:
20.08
Step-by-step explanation:
add 192.77 and 48.19 and then divide that by 12
PLS HELPP I NEED AN ANSWER ASAP ILL GIVE BEAINLIEST

Answers
The top right graph could show the arrow's height above the ground over time.
Which graph models the situation?The initial and the final height are both at eye level, which is the reference height, that is, a height of zero.
This means that the beginning and at the end of the graph, it is touching the x-axis, hence either the top right or bottom left graphs are correct.
The trajectory of the arrow is in the format of a concave down parabola, hitting it's maximum height and then coming back down to eye leve.
Hence the top right graph could show the arrow's height above the ground over time.
More can be learned about graphs and functions at https://brainly.com/question/12463448
#SPJ1
Consider the following function. F(x) = x^3/2 Find the average rate of change over the interval [4,25]
![Consider the following function. F(x) = x^3/2 Find the average rate of change over the interval [4,25]](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/3EHdYqjCBlJIDQdGuSb7JR03EpP8g7cS.jpeg)
Answers
Given the function:
\(f(x)=x^{\frac{3}{2}}\)You can rewrite it in this form:
\(f(x)=\sqrt[]{x^3}\)Because by definition:
\(\sqrt[n]{b^m}=b^{\frac{m}{n}}\)• The formula for calculating the Average Rate of Change over an interval is:
\(m=\frac{\Delta y}{\Delta x}=\frac{f(b)-f(a)}{b-a}\)Where these two points are on the function:
\((a,f(a)),(b,f(b))\)In this case, given the interval:
\(\lbrack4,25\rbrack\)You can identify that:
\(a=4\)Then, substituting this value into the function and evaluating, you get:
\(f(a)=f(4)=\sqrt[]{(4)^3}=\sqrt[]{64}=8\)You can also identify that:
\(b=25\)Then, substituting this value into the function and evaluating, you get:
\(f(b)=f(25)=\sqrt[]{(25)^3}=125\)Now you can substitute values into the formula and then evaluate, in order to find the Average Rate of Change over the given interval:
\(\frac{\Delta y}{\Delta x}=\frac{125-8}{25-4}=\frac{39}{7}\)• In order to find the Instantaneous Rate of Change at the endpoints of the interval, you need to:
1. Derivate the function. Then, you need to find:
\(f^{\prime}(x)\)By definition:
\(\frac{d}{dx}(x^n)=nx^{n-1}\)Therefore, applying this rule, you get:
\(\frac{dy}{dx}(x^{\frac{3}{2}})=\frac{3}{2}x^{\frac{3}{2}-1}=\frac{3}{2}x^{\frac{3}{2}-1}=\frac{3}{2}x^{\frac{1}{2}}=\frac{3}{2}\sqrt[]{x}\)Then:
\(f^{\prime}(x)=\frac{3}{2}\sqrt[]{x}\)2. Now you have to substitute this value of "x" into the function derivated:
\(x=4\)In order to find:
\(f^{\prime}(4)\)Then, substituting and evaluating, you get:
\(\begin{gathered} f^{\prime}(4)=\frac{3}{2}\sqrt[]{4} \\ \\ f^{\prime}(4)=\frac{3}{2}\sqrt[]{4} \\ \\ f^{\prime}(4)=3 \end{gathered}\)3. Substitute this value of "x" into the function derivated before:
\(x=25\)In order to find:
\(f^{\prime}(25)\)Then, substituting and evaluating, you get:
\(\begin{gathered} f^{\prime}(25)=\frac{3}{2}\sqrt[]{25} \\ \\ f^{\prime}(25)=\frac{15}{2} \end{gathered}\)Hence, the answers are:
\(\frac{\Delta y}{\Delta x}=\frac{39}{7}\)\(\begin{gathered} f^{\prime}(4)=3 \\ \\ f^{\prime}(25)=\frac{15}{2} \end{gathered}\)Which of these expressions is equivalent to log ( 15 x 24 )
Answers
Answer:
15*24=360
Step-by-step explanation:
Answer:On A.p.E.X its Log (15) + Log (24)
Step-by-step explanation:
what is the mean? 43, 301, 73, or 52what is the median? 39, 43, 73, or 52what is the mode? 52, 73, 43, or 39what is the range? 73, 39, 52, or 43

Answers
The mean is calculated by the formula
\(\bar{x}=\frac{\sum ^n_{i\mathop=1}x_i}{n}\)\(\begin{gathered} \bar{x}=\frac{33+52+52+39+84+30+11}{7} \\ \bar{x}=\frac{301}{7} \\ \bar{x}=43 \end{gathered}\)The median can be found by rearranging the data from least to greater and the median will be the data exactly in the middle
\(11-30-33-39-52-52-84\)the median is 39.
The mode is the date that repeats more time over the data set, in this case the only one that repeats itself is 52.
the mode is 52.
Finally, the range is the difference between the smaller value
\(\begin{gathered} 84-11 \\ 73 \end{gathered}\)the range is 73.
Define three equivalence relations on the set of buildings on a college campus. determine the equivalence classes for each of these equivalence relations.
Answers
If a relationship R fulfills the three conditions of being transitive, symmetric, and reflexive, then R is said to be an equivalence relation.
When (a, a) € R for any element a € A, we say that R is reflexive on A.
When (b, a) €R for every (a, b) €R, we say that the relation R on A is symmetric.
If (a.b) € R and (b, c) € R implies (a, c) € R, then R is transitive on A.
If you have an a, you may think of all the components that are equivalent to it as its equivalence class.
A=College buildings
We need to define three sets of things that are the same. For instance:
R1 = "(a, b) Both a and b have the same number of rooms."
R2=(a,b): Both a and b have the same number of floors.
R3=(a,b), which means that both a and b have the same number of windows.
Note: If the statement says that a and b have the same property, the relation is probably an equivalence relation.
The set of all elements that are related to an is its equivalence class.
[a]R1,= {b|b has the same number of rooms as a}
[a]R2 = {b|b has the same number of floors as a}
[a]R3= {b|b has the same number of windows as a}
Learn more about equivalence relations here- https://brainly.com/question/15828363
#SPJ4
what is the rate of change of y to x for 25x-5y=50
Answers
Answer:0
Step-by-step explanation:
Because it says rate of change witch don’t have one
select all the expressions that equal.. *see picture for problem*

Answers
Answer:
Step-by-step explanation:
oknjbgfkdfrgn jnfbfl
If Sirius rises at 8: 00 p.m. tonight, at what time will it rise tomorrow night, to the nearest minute? Explain.
Answers
If Sirius rises at 8:00 p.m. tonight, it will rise around 7:56 p.m. tomorrow night, to the nearest minute.
Sirius, the brightest star in the night sky, rises approximately four minutes earlier each day due to Earth's rotation. Therefore, if Sirius rises at 8:00 p.m. tonight, it will rise approximately four minutes earlier tomorrow night.
To find the exact time, we need to consider the sidereal day, which is the time it takes for Earth to complete one rotation relative to a fixed point in space. The sidereal day is about 23 hours, 56 minutes, and 4 seconds. Since we are only interested in the approximate time, we can disregard the additional 4 minutes and 4 seconds.Therefore, if Sirius rises at 8:00 p.m. tonight, it will rise around 7:56 p.m. tomorrow night, to the nearest minute.
Learn more about sidereal day visit:
brainly.com/question/31456031
#SPJ11
What is the constant of proportionality in the equation y 5/4 x ?
Answers
Answer:
Firest y5/ 4x = y5 - 4 /4X -4
x= y
Answer quickly IF u love god i do please

Answers
Answer:
The answer is 11
Step-by-step explanation:
The answer is 11 because you need to add the sides up that the points are on point P is 5 Point Q is 3 and point R is 3 5+3 is 8 8+3 is 11
Answer:
19.56
Step-by-step explanation:
PR = \(\sqrt{3^{2} + 3^{2} }\) = \(\sqrt{18}\) = 3\(\sqrt{2}\)
RQ = \(\sqrt{5^{2} +5^{2} }\) = \(\sqrt{50}\) = 5\(\sqrt{2}\)
QP = \(\sqrt{8^{2} +2^{2} }\) = \(\sqrt{68}\) = 2\(\sqrt{17}\)
Perimeter = sum of the sides = 3\(\sqrt{2}\) + 5\(\sqrt{2}\) + 2\(\sqrt{17}\) = 19.56
Break the triangle PQR into 3 right triangles, with each leg being a hypotenuse. Then you can use the Pythagorean theorem to find the length of each leg. Add them all together to get the perimeter.
Can someone please help me ASAP?? It’s due tomorrow!! I will give brainliest If It’s correct.

Answers
Answer: To match the shapes produced by the slice through the triangular prism, we need to consider the orientation of the slice relative to the prism. Here are the matching options:
A. Perpendicular to the base: Rectangle
B. Parallel to the base: Triangle with dimensions equal to the base
C. Diagonal from vertex to vertex: Triangle with unknown dimensions
Which situation is best modeled by the equation 9+=16

Answers
Answer:
4th option.
Step-by-step explanation:
You pay $9 and $__ for a total of $16.
$9 + $__ = $16.
9+__=16
Eight more than the product of a number and 5 is 9.
Answers
Answer:
1/5
Step-by-step explanation:
Eight more than the product of a number and 5 is 9.
Let the number be x
More = +
Product of x and 5 is 5x
8 + 5x = 9
5x = 1
x = 1/5
The number is 1/5
Hope this helps :)
Have a nice day!
is it one solution or many solutions y=2x+7 and y=3x-1
Answers
Answer:
the answer is one solution
Answer:
One solution
Step-by-step explanation:
y=2x+7
y=3x-1 (Multiply this by -1)
-y = -3x + 1
y=2x+7
0 = -x + 8
-x = -8
x = 8
y=2x+7
y= 2(8)+7
y = 16 + 7
y = 23
(8, 23)
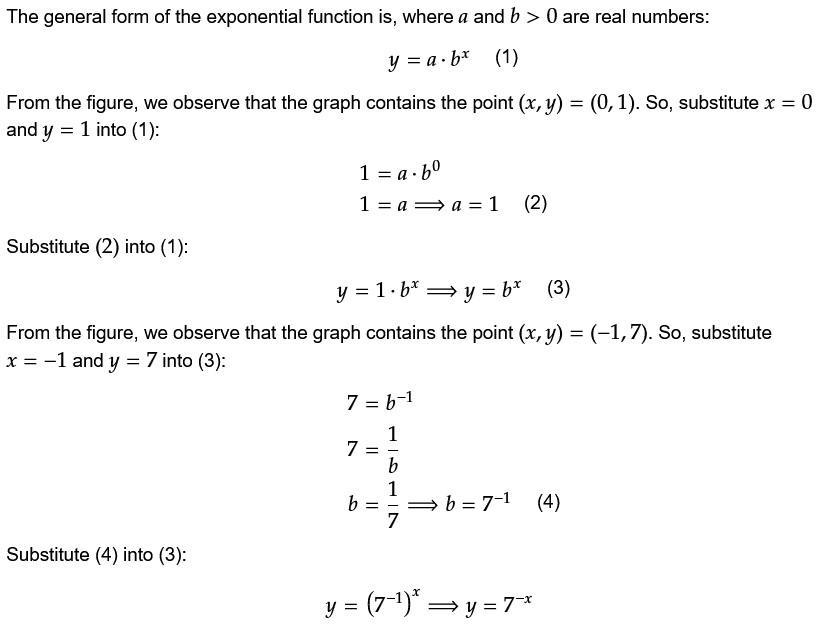
Select one of the following functions to match the graph.
A. y = (7) x
B. y = (7)-x
C. y = (-7) x
D. y = (-7)-x

Answers
Answer:
B
Step-by-step explanation:
The explanation is attached below.
Which of the following triangles are right triangles? Check all that apply. A. A triangle with side lengths 6 inches, 8 inches, 10 inches B. A triangle with side lengths 8, 15, 17 • C. A triangle with side lengths 4, 5, 6 D. A triangle with side lengths 5, 12, 13
Answers
As per the Pythagorean theorem, the right triangles are A triangle with side lengths 6 inches, 8 inches, 10 inches, A triangle with side lengths 8, 15, 17 and A triangle with side lengths 5, 12, 13 (option A, B and D)
Let's consider the four triangles given in the problem:
A. A triangle with side lengths 6 inches, 8 inches, 10 inches B. A triangle with side lengths 8, 15, 17 C. A triangle with side lengths 4, 5, 6 D. A triangle with side lengths 5, 12, 13
To determine whether each triangle is a right triangle, we need to apply the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
For triangle A, we have:
6² + 8² = 10² 36 + 64 = 100 100 = 100
Since the equation is true, we know that triangle A is a right triangle.
For triangle B, we have:
8² + 15² = 17² 64 + 225 = 289 289 = 289
Again, the equation is true, so triangle B is also a right triangle.
For triangle C, we have:
4² + 5² = 6² 16 + 25 = 36 41 ≠ 36
The equation is not true, so triangle C is not a right triangle.
Finally, for triangle D, we have:
5² + 12² = 13² 25 + 144 = 169 169 = 169
Once again, the equation is true, so triangle D is a right triangle.
To know more about triangle here
https://brainly.com/question/8587906
#SPJ1
f(x) = -2(x + 2)2 + 4
Answers
Answer:
4x4=? then we have 2x2=?
Step-by-step explanation: H-JJ-
PLEASE HELP ME PLEASE

Answers
Answer:
8.5
Step-by-step explanation:
15x+10+6x+2=180 (add up to we 180 because they are alternate eachother
21x+12=180 combine like terms
21x=178 subtract 12 from both sides
x~~8.5 divide both sides by 21
30 boys and 56 girls as a ratio
Answers
Answer:
30:56!
Step-by-step explanation:
since 30 boys is mentions first you can start it as that way
it helps me better and is way more efficient