if you see this i really need help asap! ty, 'A man pushes a 10 kg box with a force of 5 N. How much will the box accelerate?'
Answers
5kgm/s2=10kg•a
a= 1/2 m/s2
Related Questions
2=n/3 n=? Please answer as quick as possible thx
Answers
Answer:
\( \boxed{n = 6} \)
Step-by-step explanation:
\( = > 2 = \frac{n}{3} \\ \\ = > \frac{n}{3} = 2 \\ \\ = > n = 2 \times 3 \\ \\ = > n = 6\)
PLEASE PLEASE PLEASE HELP ME!!!!
A plane, 50 miles away from its
destination, is flying toward the airport at
an altitude of 30,500 feet. Later, this same
plane is 20 miles from the airport and has
descended to an altitude of 18,000 ft.
How far has the plane flown?
Answers
Answer:
Below
Step-by-step explanation:
The plane's distance is the hypotenuse of a right triangle with legs of
(50 -20)mi = 30 miles and 30500 - 18000 = 12500 ft
for consistent units 12500ft/5280ft/mile = 2.36742 miles
Now just use Pythagorean theorem
Hypotenuse^2 = leg1^2 + leg2^2
h^2 = 30^2 + 2.36742^2
h = ~ miles 30.093 miles ( note that the vertical descent distance does not matter much)
(this is 158 892 feet)
Which transformation maps quadrilateral EFGH onto JKLM?
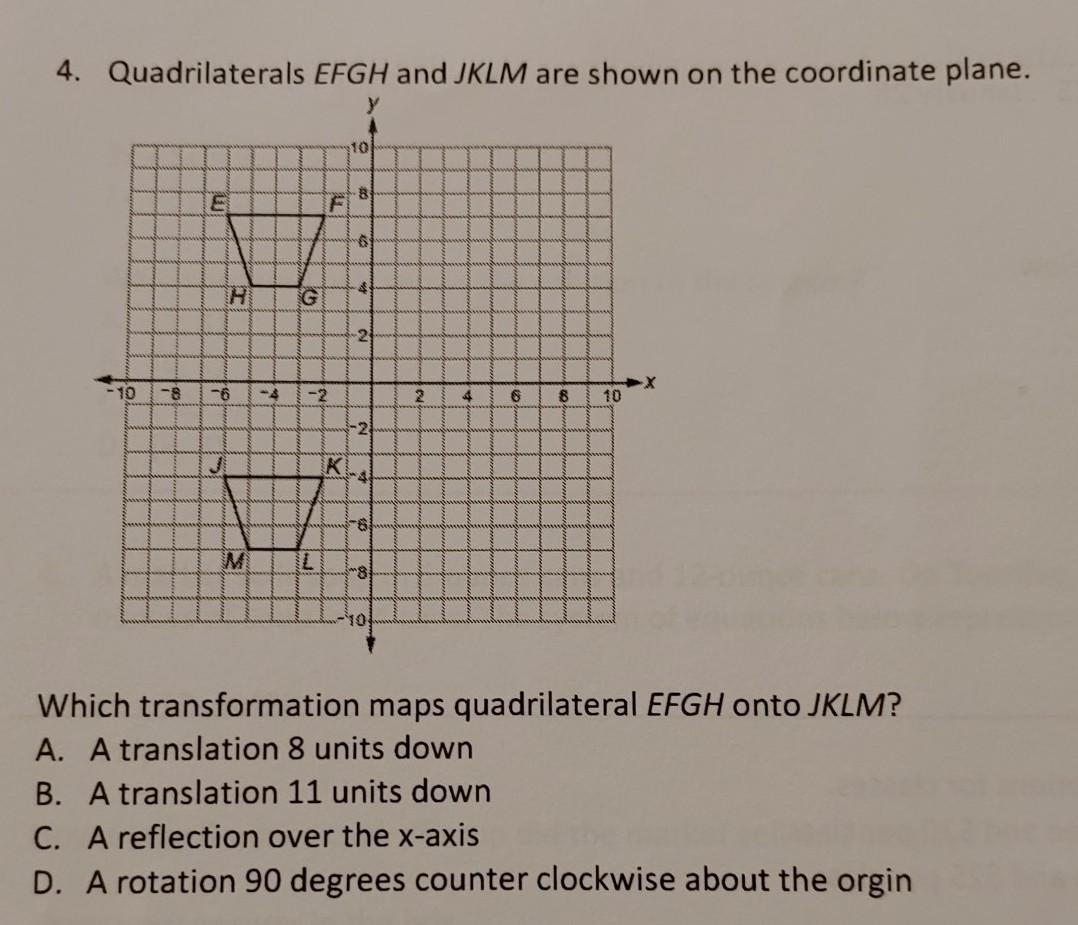
Answers
Answer:
A) A translation 8 units down
Step-by-step explanation:
It does not reflect over the x-axis because it would have flip.
Not 11 units down, only 8
it did not rotate 90 degrees, it went a little more past that
Two numbers add up to $23$ and their difference is $27$. How far apart are the two numbers
Answers
Answer:
27
Step-by-step explanation:
x + y = 23
x - y = 27
(x + y) + (x - y) = 23 + 27
2x = 50
x = 25
y = - 2
How to find distance:
|25 -(-2)| = |27| = 27
Answer:-4+27
Step-by-step explanation:
prove that in a group of 250 students, the family name of at least ten students must start with the same letter. there are 26 letters in the english alphabet
Answers
To prove that in a group of 250 students, the family name of at least ten students must start with the same letter, we can use the Pigeonhole Principle.
The Pigeonhole Principle states that if you have n pigeonholes (in this case, the 26 letters of the English alphabet) and you are placing m > n items (here, 250 students) into the pigeonholes, at least one pigeonhole must contain more than one item.
Here's a step-by-step explanation:
1. We have 26 pigeonholes, representing the 26 letters of the English alphabet.
2. We have 250 students (items) to place into these pigeonholes based on the first letter of their family name.
3. Apply the Pigeonhole Principle: Divide the total number of students (250) by the total number of pigeonholes (26).
250 ÷ 26 ≈ 9.6
4. Since we can't have a fraction of a student, we round up to the nearest whole number.
10 students per pigeonhole
5. By rounding up, we find that at least one pigeonhole (letter of the alphabet) must have 10 or more students with family names starting with that letter.
So, in a group of 250 students, the family name of at least ten students must start with the same letter, as demonstrated by the Pigeonhole Principle.
To learn more about Pigeonhole Principle - brainly.com/question/30322724
#SPJ11
The period of the function, y=cos(4x)is how many degrees?
Answers
A rectangular painting measures 1/2 yards wide and 5/4 yards long find the area of the painting in square yards.
Answers
Answer: 5/8 yards²
Step-by-step explanation:
The painting is in the shape of a rectangle as stated and the formula for finding the are of a rectangle is:
= Length * Width
The are of the rectangular painting is:
= 5/4 * 1/2
= 5/8 yards²
Witten in decimal form:
= 0.625 yards²
In which row and column will the number 83 appear?

Answers
Answer:
Row 10 and in column F
Step-by-step explanation:
rows 1 and 3 are decreasing and we are looking for an increase so we can cross those out immediately.
However we do see a pattern in rows 2 to 4 (add 8) so if we add 8 for 3 more rows than we get 37 but add 8 more and we get 45.
from 37 to 43 is 6 more than 37 so we have 4 columns but if we add 3 more we get the 6 columns also column f.
Hope this helps!
A triangle has squares on its three sides as shown below. What is the value of x? (1 point)
Three squares having side lengths 9 cm, x cm, and 12 cm joining to form right triangle at the centre
Group of answer choices
15 inches
108 inches
12 inches
144 inches
Answers
Answer:
is A: 15
Step-by-step explanation:
If you use the Pythagorean Theorem:
\(9^{2}\) + \(12^{2}\) = \(x^{2}\)
81 + 144 = \(x^{2}\)
x = \(\sqrt\)225
x = 15
Hope this helped!
Solve for x in the triangle shown.

Answers
Answer:
hmm
Step-by-step explanation:
Please someone help me with this... and please thouroughly explain, i have no clue what to do.

Answers
144. Use the formula for the volume of a cone, since the answer is in terms of pi disregard the pi in the formula and solve using (r^2)(h/3) which will give you the answer in terms of pi.
The length of a rectangle is eight more than twice it's width. the perimeter is 96 feet. find the dimensions
Variables:
equation:
Solution:
Answers
Answer:
the length and width be 13.33 feet and 34.67 feet respectively
Step-by-step explanation:
The computation is shown below:
Let us assume the width be x
So, the length be 2x + 8
As we know that
The perimeter of the rectangle = 2(length + width)
96 = 2(2x + 8 + x)
96 = 4x + 16 + 2x
96 - 16 = 6x
80 = 6x
x = 80 ÷ 6
= 13.33 feet
So, the length would be
= 2 × 13.33 + 8
= 34.67 feet
Hence, the length and width be 13.33 feet and 34.67 feet respectively
using the smallest number of pebbles possible and without using blue pebbles, what is the value in pebbles of 2 blue pebbles?
Answers
According to the combination, The value of the Two blue pebbles is a 3 red pebbles and 1 yellow pebbles.
According to the statement
We have to find that the value of the combination.
So, For this purpose, we know that the
A combination could be a mathematical technique that determines the quantity of possible arrangements in an exceedingly collection of things where the order of the choice doesn't matter.
From the given information:
A red pebble is capable 3 yellow pebbles. A blue pebble is adequate a red pebble and a couple of yellow pebbles. Therefore, the blue pebbles will be:
= 3 yellow pebble + 2 yellow pebbles
= 5 yellow pebbles or 15 red pebbles
And
Two blue pebbles will be:
= 10 yellow pebbles
= 3 red pebbles and 1 yellow pebbles.
So, According to the combination, The value of the Two blue pebbles is a 3 red pebbles and 1 yellow pebbles.
Learn more about Combination here
https://brainly.com/question/24756209
Disclaimer: This question was incomplete. Please find the content below.
Question:
A red pebble is equal to 3 yellow pebbles A blue pebble is equal to a red pebble and 2 yellow pebbles. A green pebble is equal to 3 red pebbles
Using the smallest number of pebbles possible and without using blue pebbles, what is the value in pebbles of 2 blue pebbles?
#SPJ4
If you place a 40-foot ladder against the top of a building and the bottom of the ladder is 17 feet from the bottom of the building, how tall is the building? Round to the nearest tenth of a foot.
Answers
Answer:
no se ingles pero, alguien me puede ayudar?
Answer:
The building is 36.2 ft tall.
Step-by-step explanation:
i got it right on the deltamath
At which root does the graph of f x x 5 3 * x 2 2 touch the X axis?
Answers
The root of the graph of f(x) =(x - 5)3(x + 2)2 touches the x-axis at -2,5
(x - 5)^3 has a power of 3 which is an ODD number. An ODD power means that the graph will cross through the x-axis.
(x + 2)^2 has a power of 2 which is an EVEN number. An EVEN power means that the graph will touch the x-axis.
Given: Function f(x) is (x - 5)3(x + 2)2
If a curve touches the x-axis then f(x) = 0
⇒ (x - 5)3(x + 2)2 = 0.
But if ab = 0 ⇒ either a = 0 or b = 0 or both zero.
⇒ (x - 5)3 = 0 and (x + 2)2 = 0
⇒ (x - 5) = 0 and x + 2 = 0
⇒ x = 5 and x = - 2.
To know more about the roots of functions:
https://brainly.com/question/1942920
#SPJ4

find an antiderivative of f(x) = tan−1x satisfying f(0) = 1.
Answers
The antiderivative of f(x) = tan⁻¹(x) is x tan⁻¹(x) − ln(x²+1)/2 + C
The term antiderivative in math is referred as a function that reverses what the derivative does
Here we have given the expression f(x) = tan⁻¹(x).
Now, the antiderivative of the expression is calculated by doing the integral
=> ∫tan⁻¹(x)dx
By using the integration by parts ∫udv = uv−∫vdu
Here let us consider that u=tan⁻¹(x) and dv=dx
And the value of du is calculated as,
=> du = ∫tan⁻¹(x)dx = dx/(x² + 1)
And the value of v = x.
Then the expression is written as,
=> ∫tan⁻¹(x)dx = x tan⁻¹(x) - ∫x/(x² + 1)dx
As per the concept of the constant of integration, it can be simplified as,
=> x tan⁻¹(x) − ln(x²+1)/2 + C
To know more about Expression here.
https://brainly.com/question/14083225
#SPJ4
Which number would make this number sentence true? (3 + 2) x ? = 5 x 8

Answers
Answer:
8
Step-by-step explanation:
3+2=5
5 x 8 = 5 x 8
Answer:
8
Step-by-step explanation:
2 numbers are in perenticies so do them first 3+2 = 5 Then wait dont do nothing with that do 5x8 to get what to need to get 5 x? to witch is
40 so 8 would be the correct answer
For which angles 0 mmbetween 0 and 2m is cos(0)

Answers
Answer: \(\frac{\pi }{2 }\) < ∅ < \(\frac{3}{4}\pi\)
Step-by-step explanation:
They are asking where is cos∅ negative. cos is your x value in the unit circle (your adjacent)
So cos∅ is neg between \(\frac{\pi }{2 }\) < ∅ < \(\frac{3}{4}\pi\)
theta is < but not = those values because cos ∅ is 0 (which is neither neg or pos.
Answer: \(\frac{\pi }{2 }\) < ∅ < \(\frac{3}{4}\pi\)
\(\frac{tan(\frac{-2π}{3}} {sin\frac{7π}{4}} -sec (-π)\\\)What is the exact value of the trigonometric expression? State your answer in simplified radical form
Answers
The exact value of the trigonometric expression \(\frac{tan-(2\pi/3) }{sin(7\pi /4)} - sec(-\pi )\) in the simplified radical form is √6 + 1.
\(\frac{tan-(2\pi/3) }{sin(7\pi /4)} - sec(-\pi )\)
We have the angle,
-2π/3 = -120° = (360 - 120)° = 240° = 60°
Put the value of -2π/3 in tan -2π/3, we get
tan -2π/3 = tan (60) = √3
7π/4 × 180/π = 7 × (180/4) = 7 × 45 = 315° = 45°
Put the value of 7π/4 in sin 7π/4, and we get
sin 7π/4 = sin (45°) = √2/2
sec (-π) = sec (π) = -1
Now, substitute all the values in the given equation,
= {√3/(√2/2)} - (-1)
= (2√3/√2) + 1
= (2√3 × √2/√2 × √2) +1
= (2√6/2) + 1
= √6 + 1
--The given question is incorrect, the correct question is
''What is the exact value of the trigonometric expression? \(\frac{tan-(2\pi/3) }{sin(7\pi /4)} - sec(-\pi )\) State your answer in simplified radical form."--
To know more about trigonometric expression, here
https://brainly.com/question/11659262
#SPJ4
PLEASE HELP WILL MARK BRAINLIEST

Answers
Answer:
Step-by-step explanation:
x^2 = 64
√x² = √64
x = 8, -8
answer is B
.Specifications for a piece of material used in the manufacture of a bed mattress require that the piece be between 63.76 and 64.24 inches. The process that produces the piece yields a mean of 64 and a standard deviation of 0.1 inches. The distribution of output is normal. What percentage of the pieces will meet the length specs?
Answers
The correctanswer is-the percentage of the pieces that will meet the length specs is 98.78%.
The given data may be a random variable that takes after the normal distribution with mean μ=64 and standard deviation σ=0.1
The required value is to discover the rate of the pieces that will meet the length specs which is between 63.76 and 64.24 inches.
To find the required percentage, standardize the given limits as follows: Lower Limit: (63.76 - μ) / σ= (63.76 - 64) / 0.1 = -2.4
Upper Limit: (64.24 - μ) / σ= (64.24 - 64) / 0.1 = 2.4
Using the Standard Normal Distribution Table, the probability that the value will fall between -2.4 and 2.4 is found to be 0.9878.
The required percentage is then found by multiplying the probability by 100, which is: Percentage = 0.9878 x 100% = 98.78%
Therefore, the percentage of the pieces that will meet the length specs is 98.78%.
know more about Normal Distribution
https://brainly.com/question/15103234
#SPJ11
Given: ST || UV, ST = VU
Prove: STW = VUW

Answers
Hence the given statement is proved.
Given: ST || UV, ST = VU
we are asked to prove STW = VUW
Statement(Reason)
ST || UV (Given)
Angle S = Angle V (Alternate interior angle)
Angle SWT = Angle VWU (Vertically opposite Angle)
ST = VU (Given)
Therefore, based on above, we can say that the triangle STW is congruent to VUW by AAS (i.e. Angle Angle Side congruency.
Hence the statement is proved bu angle angle side congruency.
Learn more about Parallel lines here:
brainly.com/question/24607467
#SPJ9
Can someone please please help mke i was suppose to finnish this at least 4 weeks ago please can someone answer this question for at leat 150 or 200 points.

Answers
The number of mulch sold is 108 pounds, and the number of gravel sold is 72 pounds.
What is the number of each item sold?The number of each item sold is calculated as follows;
The number of gravel found in mulch sold is calculated as;
( 2 ¹/₄ ) / ( 1 ¹/₂ ) = ( 2.25 ) / ( 1.5 ) = 1.5
mulch = 1.5 gravel
let the number of gravel = x
1.5x + x = 180
2.5x = 180
x = 180 / 2.5
x = 72
The number of mulch = 1.5 x 72 = 108
Learn more about gravel and mulch here: https://brainly.com/question/13674561
#SPJ1
true or false: if a data set is approximately normally distributed, its normal probability plot would be s-shaped.
Answers
Doug is a salesperson in a retail store and earns $75 per week, plus 15% of his weekly sales. If Doug makes $555 one week, what are his sales for that week?
A. $3,200
B. $627
C. $566
D. $1,914
Answers
Doug made sales worth A. $3,200 in the last week.
What is the percentage?A percentage is a value per hundredth. Percentages can be converted into decimals and fractions by dividing the percentage value by a hundred.
Given, Doug is a salesperson in a retail store and earns $75 per week,
Plus 15% of his weekly sales.
Doug makes $555 one week,
∴ He made $(555 - 75) = $480.
So, $480 is 15% of his weekly sales, Assuming he made sales worth $x.
∴ 15% of x is $480.
(15/100)×x = 480.
0.15x = 480.
x = 480/0.15.
x = 3200.
learn more about percentages here :
https://brainly.com/question/24159063
#SPJ2
At 1pm, there were 530 visitors in the museum. At 8pm, there were 250. What
is the constant rate of change?
Answers
The work is below

The joint distribution for the length of life of two different types of components operating in a system is given by f(y1, y2) = { 1/27 y1e^-(y1+y2)/3 , yi > 0, y2 > 0,
0, elsewhere, }
The relative efficiency of the two types of components is measured by U = y2/y1. Find the probability density function for U. f_u(u) = { ________, u >=0
________, u< 0 }
Answers
The probability density function for U is {2/(1+U)³; U≥0
0, U<0}
What is the probability?
A probability is a number that reflects how likely an event is to occur. It is expressed as a number between 0 and 1, or as a percentage between 0% and 100% in percentage notation. The higher the likelihood, the more probable the event will occur.
Here, we have
Given: The joint distribution for the length of life of two different types of components operating in a system is given by
f(y₁, y₂) = { 1/27 y₁\(e^{-(y_1+y_2)/3}\), y₁ > 0, y₂ > 0
0, elsewhere, }
Let U = y₂/y₁ and Z = y₁ and y₂ = UZ
|J| = \(\left|\begin{array}{cc}1&0\\U&Z\end{array}\right|\) = Z
The joint distribution of U and Z is
f(U,Z) = 1/27 Z²\(e^{-(Z+UZ)/3}\), Z≥0, U≥0
The marginal distribution is:
f(U) = \(\frac{1}{27} \int\limits^i_0 {Z^2e^{-(Z+UZ)/3} } \, dZ\)
f(U) = 2/(1+U)³; U≥0
f(U) = {2/(1+U)³; U≥0
0, U<0}
Hence, the probability density function for U is {2/(1+U)³; U≥0
0, U<0}
To learn more about the probability from the given link
https://brainly.com/question/24756209
#SPJ4
In the figure below, the radius ofthe inscribed circle is 6 inches.What is the perimeter of squareWXYZ?
Answers
The perimeter of square WXYZ can be determined by considering the properties of the inscribed circle. The perimeter of square WXYZ is approximately 33.94 inches
In a square, the diagonals bisect each other at right angles and intersect at the center of the square. The radius of the inscribed circle is the distance from the center to any side of the square. Since the radius is given as 6 inches, it is also the distance from the center to any corner of the square.
The radius of the inscribed circle forms a right triangle with half of one side of the square and the diagonal. The length of half a side of the square is equal to the radius of the inscribed circle, which is 6 inches. The diagonal of the square is twice the length of one side, so it is 12 inches.
Using the Pythagorean theorem, we can calculate the length of one side of the square:
(side length)^2 + (side length)^2 = (diagonal length)^2
(6 inches)^2 + (6 inches)^2 = (side length)^2
36 inches^2 + 36 inches^2 = (side length)^2
72 inches^2 = (side length)^2
Taking the square root of both sides, we get:
side length = √72 inches ≈ 8.485 inches
Since the square has four equal sides, the perimeter of the square is:
perimeter = 4 * side length = 4 * 8.485 inches = 33.94 inches
Therefore, the perimeter of square WXYZ is approximately 33.94 inches.
To learn more about Pythagorean theorem click here: brainly.com/question/14930619
#SPJ11
given δabc≅ δpqr,m∠b = 3v+4 and m∠q = 8v ― 6 , find m∠b and m∠q.
Answers
the measure of angle ∠B is 38/5 and the measure of angle ∠Q
is 18/5.
We are given that δABC ≅ δPQR. This means that the corresponding angles in the two triangles are congruent. Specifically, angle ∠B in δABC is congruent to angle ∠Q in δPQR.
Let's equate the expressions for the angle measures:
3v + 4 = 8v - 6
Simplifying the equation, we have: 6 = 5v
Dividing both sides by 5, we find: v = 6/5
Now, we can substitute this value of v back into the expressions for the angle measures:
m∠B = 3v + 4
= 3(6/5) + 4
= 18/5 + 4
= 38/5
m∠Q = 8v - 6
= 8(6/5) - 6
= 48/5 - 6
= 48/5 - 30/5
= 18/5
Learn more about triangles here:
https://brainly.com/question/29083884
#SPJ11
10 computer operators can compose 120 pages in 2 hours. How many operators should work, if the same job is to be completed in 30 minutes?
Answers
Answer:
40 Operators
Step-by-step explanation:
if 10 operators worked on 120 pages for 2 hours
P operators will work on the same pages for 30min
note: 2hrs= 120min
10×120min/120= P × 30/120
cross multiply make P subject of the formulaP= 10×120×120/120×30
P = 40 operators