If your t-test value is t=1.45 and the critical value is CV=5.66, your data is: A.significant B.different not significantly different.
Answers
If your t-test value is t=1.45 and the critical value is CV=5.66, your data is not significantly different.
In a t-test, you compare the t-value to the critical value to determine the significance of the data. If the t-value is greater than the critical value, it indicates that the data is significant and there is a difference between the groups being compared. However, if the t-value is less than the critical value, it means that the data is not significantly different, and any observed difference may be due to chance or random variation.
In this case, the t-value (1.45) is less than the critical value (5.66), which suggests that the data is not significantly different. This means that the differences observed between the groups in your study may not be meaningful, and there may not be a true difference between the groups.
It is important to consider other factors, such as sample size and effect size when interpreting the results of a t-test, but based on the provided values, your data is not significantly different.
You can learn more about critical value at: brainly.com/question/30168469
#SPJ11
Related Questions
Find the value of x with the help of following

Answers
The value of x is 22.
Given is a parallelogram ABCD, in which angle B and C are 4x+12 and 3x+14, we are asked to find the value of x,
We can make use of the fact that a parallelogram's adjacent angle sum equals 180 degrees.
According to the given information, the adjacent angles B and C are 4x + 12 and 3x + 14, respectively.
The equation:
(4x + 12) + (3x + 14) = 180
Combine like terms:
7x + 26 = 180
Subtract 26 from both sides:
7x = 154
Divide both sides by 7:
x = 22
Therefore, the value of x is 22.
Learn more about parallelogram click;
https://brainly.com/question/28854514
#SPJ1
Using an integrating factor, solve y-y-5 CD- in the method for solving a first-order linear differential equation, the first step is to put the equation in the standard form y alty bit). is the given equation in the standard form? No Yes Identify a(t) and bit)
Answers
The value of a(t) is -1 and b(t) is 55 + \(e^t\)
No, the given equation y' - y = 55 + \(e^t\) is not in the standard form of a first-order linear differential equation.
In the method for solving a first-order linear differential equation, an integrating factor is a function used to transform the equation into a form that can be easily solved.
For an equation in the standard form y' + a(t)y = b(t), the integrating factor is defined as:
μ(t) = e^∫a(t)dt
To solve the equation, you multiply both sides of the equation by the integrating factor μ(t) and then simplify. This multiplication helps to make the left side of the equation integrable and simplifies the process of finding the solution.
To put it in standard form, we need to rewrite it as y' + a(t)y = b(t).
Comparing the given equation with the standard form, we can identify:
a(t) = -1
b(t) = 55 + \(e^t\)
Therefore, The value of a(t) is -1 and b(t) is 55 + \(e^t\)
Learn more about integrating factor here
https://brainly.com/question/32554742
#SPJ4
In △RST, ∠R≅∠T, TR=7 and ST=5. Find RS.
Answers
The length of RS (or RT) can also be represented as 26 in simplified radical form.
Since ∠R ≅ ∠T, we know that △RST is an isosceles triangle and that RS = RT. Let x be the length of RS (or RT). Then we can use the Pythagorean theorem to solve for x:
\(RS^2 + ST^2 = RT^2\)(By Pythagoras theorem)
\(x^2 + 5^2 = 7^2\\x^2 + 25 = 49\\x^2 = 49 - 25\\x^2 = 24\\x = \sqrt{24\)
Therefore, the length of RS (or RT) is √24, which can also be written as 2√6 in simplified radical form (since 24 can be factored as 2^2 × 6).
Learn more about triangles here:
https://brainly.com/question/2773823
#SPJ1
pls help easy!!
There are 15 pieces of fruit in a bowl and 6 of them are apples. What percentage of the pieces of fruit in the bowl are apple?
-0.06%
-0.4%
-6%
-40%
Answers
Answer:
40%
Step-by-step explanation:
brainliest please
Answer:
D: 40%
Step-by-step explanation:
total: 15
apples: 6
6 out of the 15 fruits are apples
6/15 = 40%
I hope this helped and if it did I would appreciate it if you marked me Brainliest. Thank you and have a nice day!
What is the trigonometric ratio for sin C?
Enter your answer, as a simplified fraction,
In the boxes.

Answers
==========================================================
Explanation:
We have a triangle with these three sides.
a = 80b = unknownc = 82Use the pythagorean theorem to find b
a^2+b^2 = c^2
b = sqrt(c^2 - a^2)
b = sqrt(82^2 - 80^2)
b = sqrt(324)
b = 18
This is the missing vertical leg of the triangle. And this is also the side opposite angle C.
We have enough information to compute the sine of the angle.
sin(angle) = opposite/hypotenuse
sin(C) = AB/AC
sin(C) = 18/82
sin(C) = (9*2)/(41*2)
sin(C) = 9/41
what is the inequality of 2.2m < 11
Answers
Answer:
m<5
Step-by-step explanation:
Answer:
m < 5
Step-by-step explanation:
You bought a new video game for $65. You beat the game and want to sell it and buy a new game.
GameStop sells for $40, and they want to make 15% profit. How much will they give you for the game?
Answers
Answer:
Si es el 15% del IVA, serí.an $46 por el juego
Pero si es el 15% de descuento, ser.ían $36 por el juego
Para sacar el 15% es multiplicar el precio del juego en GameStop, sería:
40 x 0.15 = 6
Creo que es así, espero que te ayude
You have a biased coin, which has the probability of flipping heads at 70%. You flip once, and the coin comes up tails. What is the expected number of flips from that point (so counting that as flip
Answers
E(X) = P(H2)E(X | H2) + P(T2)E(X | T2)E(X) = 0.7(1) + 0.3(1 + E(X))E(X) = 0.7 + 0.3E(X)0.7E(X) = 0.7E(X) + 0.7E(X) - 0.3E(X)0.3E(X) = 0.7E(X)E(X) = 0.7 / 0.3E(X) = 2.33 (rounded to two decimal places)Therefore, the expected number of flips from the point where we flipped tails is 2.33 flips.
Given a biased coin whose probability of flipping a head is 70%, the probability of flipping a tail is 30%.After one flip, the possible outcomes are H or T. Let X be the random variable representing the number of flips from that point, given that a tail was flipped initially. Then we can define the probability of flipping heads at the ith flip as P(Hi), and the probability of flipping tails at the ith flip as P(Ti).
Since we flipped tails initially, P(H1) = 0.3 and P(T1) = 0.7. To find the expected number of flips from this point, we can use the formula: E(X) = Σ i * P(X = i)where Σ denotes the sum over all possible values of i. We can break this sum into two cases:Case 1: We flip heads on the next flip, which occurs with probability P(H2) = 0.7.E(X | H2) = 1 + 0 = 1, since we would only need to flip the coin one more time to get a head
To know more about decimal places visit:-
https://brainly.com/question/30650781
#SPJ11
Find the equation of the line below. Arrange your answer in the form y = mx + b, where b is the constant. (Note: in this answer make sure your x has a coefficient) (2,1) (-2,-1)
Answers
Answer: y = 0.5x
(b = 0)
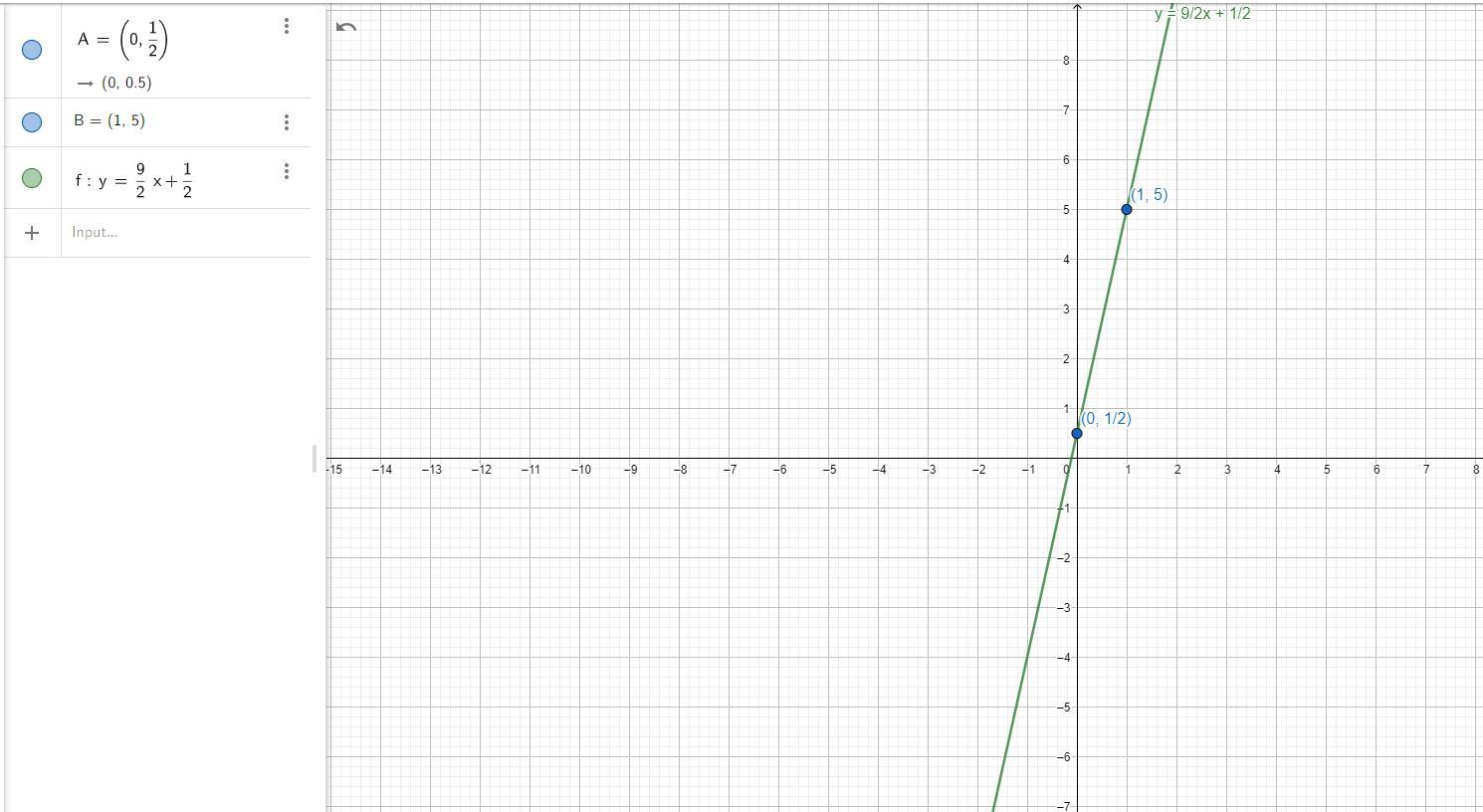
graph the linear equation using the slope intercept form . -11x+2y=1

Answers
We will have the following:
*First: We solve for y:
\(-9x+2y=1\Rightarrow2y=9x+1\)\(\Rightarrow y=\frac{9}{2}x+\frac{1}{2}\)*Second: We determine two sets of points that belong to the line. In our case we will solve for x = 0 & x = 2. So we would get:
*For x = 0:
\(y=\frac{9}{2}(0)+\frac{1}{2}\Rightarrow y=\frac{1}{2}\)So, we would have the point:
\((0,\frac{1}{2})\)*For x = 1:
\(y=\frac{9}{2}(1)+\frac{1}{2}\Rightarrow y=5\)So, we would have the point:
\((1,5)\)*Third: We graph:
two containers are used to hold liquid. these containers have exactly the same shape. the first container has a height of 20 cm, and it can hold 320 m3 of liquid. if the second container has a height of , how much liquid can it hold?
Answers
The second container can hold approximately 1715 m³ of liquid.
Two containers have exactly the same shape, we can use their height ratio to determine the liquid capacity ratio.
Let's denote the height of the first container as H1 = 20 m and its liquid capacity as C1 = 320 m³.
We want to find the liquid capacity of the second container, C2, given its height H2 = 35 m.
The ratio of the heights is H2 / H1 = 35 m / 20 m = 7/4.
The ratio of the liquid capacities is equal to the ratio of the volumes, since the containers have the same shape. Therefore, we have:
C2 / C1 = (H2 / H1)³
Substituting the given values:
C2 / 320 m³ = (7/4)³
Simplifying the exponent:
C2 / 320 m³ = 343 / 64
To find C2, we can cross-multiply:
C2 = (343 / 64) × 320 m³
Calculating the result:
C2 ≈ 1715 m³
Therefore, the second container can hold approximately 1715 m³ of liquid.
To know more about container click here:
https://brainly.com/question/430860
#SPJ4
The question is incomplete the complete question is :
two containers are used to hold liquid. these containers have exactly the same shape. the first container has a height of 20 m, and it can hold 320 m³ of liquid. if the second container has a height of 35 m, how much liquid can it hold?
What starting balance grows to $9,500 in 4 years with 6.5% simple interest?
Answers
The principal amount started at $7539.68.
Given that, amount =$9,500, rate of interest = 6.5% and time period =4 years.
What is the simple interest?Simple interest is a method to calculate the amount of interest charged on a sum at a given rate and for a given period of time.
Simple interest is calculated with the following formula: S.I. = (P × R × T)/100
Let the principal amount be x
Now, simple interest = Amount - Principal
= 9500-x
Here, 9500-x=(x×6.5×4)/100
⇒ 0.26x=9500-x
⇒ 1.26x=9500
⇒ x=9500/1.26
⇒ x=$7539.68
Therefore, the principal amount started at $7539.68.
To learn more about the simple interest visit:
https://brainly.com/question/25845758.
#SPJ1
4. Emily’s house has a chimney. What is the volume of her chimney? 4 in 9 in 12 in 3 in 4 in 6 in

Answers
Using the volume for rectangular prisms, the volume of the chimney is: 216 in.³.
What is the Volume of a Rectangular Prism?The volume of a rectangular prism = (length)(width)(height).
How to Find the Volume of Composite Shapes?The Chimney in Emily's house is a composite solid consisting of two rectangular prism. To find the volume, we have to decompose the figure into two rectangular prisms. Then solve for the volume of each before finding the total volume.
Volume of the bigger rectangular prism = (length)(width)(height) = (4)(4)(12)
Volume of the bigger rectangular prism = 192 in.³
Volume of the smaller rectangular prism = (length)(width)(height) = (4)(2)(3)
Volume of the smaller rectangular prism = 24 in.³
The volume of the chimney = volume of the two rectangular prism
The volume of the chimney = 192 + 24
The volume of the chimney = 216 in.³
Learn more about the volume of rectangular prism on:
https://brainly.com/question/12917973
#SPJ1
the president of a large company recommends that employees perform, on average, 24 hours of community service each year. the president believes that the mean number of hours of community service performed last year was different from the recommended 24 hours. to estimate the mean number of hours of community service performed last year, the president obtained data from a random sample of employees and used the data to construct the 95 percent confidence interval (20.37, 23.49). if all conditions for inference were met, does the interval provide convincing statistical evidence, at a level of significance of
Answers
If all conditions for inference were met, does the interval provide convincing statistical evidence, at a level of significance of 95%, that the mean number of hours of community service performed last year was indeed different from the recommended 24 hours.
To determine if the 95% confidence interval provides convincing statistical evidence at a level of significance that the mean number of hours of community service performed last year was different from the recommended 24 hours, we'll analyze the constructed interval (20.37, 23.49).
1. First, observe the given confidence interval: (20.37, 23.49)
2. Identify the recommended average of 24 hours by the president.
3. Check if the recommended average is within the confidence interval.
Since 24 hours is not within the interval (20.37, 23.49), it provides convincing statistical evidence, at a level of significance of 95%, that the mean number of hours of community service performed last year was indeed different from the recommended 24 hours.
Learn more about confidence interval here, https://brainly.com/question/15712887
#SPJ11
Suppose a department contains 10 men and 15 women. a) How many ways are there to form a committee of 6 people from the department? Explain your answer. b) How many ways are there to form a committee of 6 people from the department, if the number of men in the committee is equal to the number of females in the committee? Explain your answer. c) How many ways are there to form a committee of 6 people from the department, if the number of men in the committee is less than the number of females in the committee? Explain your answer.
Answers
a) The number of ways to form a committee of 6 people from the department is 177,100.
b) The number of ways to form a committee of 6 people with an equal number of men and women is 54,600.
c) The number of ways to form a committee of 6 people with more women than men is 91,455.
a) To form a committee of 6 people from the department, we can choose 6 individuals from a total of 25 people (10 men + 15 women). The order in which the committee members are chosen does not matter, and we are not concerned with any specific positions within the committee. Therefore, we can use the concept of combinations.
The number of ways to choose 6 people from a group of 25 is given by the combination formula:
C(25, 6) = 25! / (6! * (25 - 6)!) = 25! / (6! * 19!) = 177,100
Therefore, there are 177,100 ways to form a committee of 6 people from the department.
b) In this case, we need to choose an equal number of men and women for the committee. We can select 3 men from the available 10 men and 3 women from the available 15 women. Again, the order of selection does not matter.
The number of ways to choose 3 men from 10 is given by the combination formula:
C(10, 3) = 10! / (3! * (10 - 3)!) = 10! / (3! * 7!) = 120
Similarly, the number of ways to choose 3 women from 15 is:
C(15, 3) = 15! / (3! * (15 - 3)!) = 15! / (3! * 12!) = 455
To find the total number of ways to form a committee with an equal number of men and women, we multiply these two combinations:
Total = C(10, 3) * C(15, 3) = 120 * 455 = 54,600
Therefore, there are 54,600 ways to form a committee of 6 people with an equal number of men and women.
c) In this case, we need to form a committee with more women than men. We can choose 1 or 2 men from the 10 available men and select the remaining 6 - (1 or 2) = 5 or 4 women from the 15 available women.
For 1 man and 5 women:
Number of ways to choose 1 man from 10: C(10, 1) = 10
Number of ways to choose 5 women from 15: C(15, 5) = 3,003
For 2 men and 4 women:
Number of ways to choose 2 men from 10: C(10, 2) = 45
Number of ways to choose 4 women from 15: C(15, 4) = 1,365
The total number of ways to form a committee with more women than men is the sum of these two cases:
Total = (Number of ways for 1 man and 5 women) + (Number of ways for 2 men and 4 women)
= 10 * 3,003 + 45 * 1,365
= 30,030 + 61,425
= 91,455
Therefore, there are 91,455 ways to form a committee of 6 people with more women than men.
To know more about number of ways, refer here:
https://brainly.com/question/30649502
#SPJ4
crime statistics can sometimes be misleading. explain some potential problems with crime statistics that draw conclusions about the criminal behavior of certain class, age, or ethnic groups.
Answers
Crime statistics can indeed be misleading, especially when drawing conclusions about the criminal behavior of specific class, age, or ethnic groups. There are several potential problems that can arise:
Underreporting and Unreported Crimes: Crime statistics rely on reported incidents. However, many crimes go unreported due to various reasons such as fear of reprisal, lack of trust in law enforcement, or cultural factors. This can result in a distorted representation of crime rates within certain groups
Bias in Law Enforcement: Bias in law enforcement practices, including profiling or over-policing certain communities, can lead to higher arrest rates among specific groups. This can create an inflated perception of criminality within those groups in the statistics, even if the actual criminal behavior is not significantly different.
Know more about Crime statistics here:
https://brainly.com/question/14342795
#SPJ11
Given a graph for the transformation of f(x) in the format g(x) = f(x) + k, determine the k value.
k = 9
k = 6
k = 3
k = −3

Answers
The calcuated value of k from the transformation of the graphs is (b) 6
How to calculate the value of kFrom the question, we have the following parameters that can be used in our computation:
The functions f(x) and g(x)
In the graph, we can see that
The function f(x) passes through the vertex (-3, -3)The function g(x) passes through the vertex (-3, 3)The vertices of the functions have the same x-coordinate
So, we have
k = g(3) - f(3)
This means that
k = 3 + 3
Evaluate
k = 6
Hence, the value of k is (b) 6
Read more about transformation at
brainly.com/question/27224272
#SPJ1
A local children's theater sold tickets for their evening show at $5 for children and $15 for adults. For their afternoon matinee, the price was reduced to $2 for children and $5 for adults. They sold $550 worth of tickets for the evening show and $200 for the matinee.
Solve the system algebraically, and compare it with the graph you created. Explain the intersection point of the two equations in terms of the context.
Answers
The solution of the system of linear equations is \(x = 50\), \(y = 20\).
The point of intersection represents the solution found analytically and represents the scenario in which the same quantity of adults and children assisted to both shows.
Procedure - Determination of the number of people assisting to a movieConstruction of the system of equationsIn this question we need to construct a linear function for the total of people that assisted to the evening show and for the total of people that assisted to the afternoon matinee. The system of equations is described below:
Evening show
\(5\cdot x + 15\cdot y = 550\) (1)
Afternoon matinee
\(2\cdot x + 5\cdot y = 200\) (2)
Where:
\(x\) - Number of children that assisted.\(y\) - Number of adults that assisted. Resolution of the system of equations and analysis of the resultsBy algebraic means we find the following result associated with the system of equations:
\(x = 50\), \(y = 20\) \(\blacksquare\)
The graphic representation of this system is described in the image attached below.
The point of intersection represents the solution found analytically and represents the scenario in which the same quantity of adults and children assisted to both shows. \(\blacksquare\)
To learn more on systems of linear equations, we kindly invite to check this verified question: https://brainly.com/question/20379472

can you help me? im so confused

Answers
Answer:
(AB) is longer than (AC)
Step-by-step explanation:
1. Approach
Use the distance formula to find the length of the segments (AC) and (AB); substitute their endpoints into the distance formula and simplifying to solve for the length. After finding the length of each segment, compare their lengths to find out which of the statements is true. The distance formula is as follows:
\(D=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
Where (\((x_1,y_1)\)) and (\((x_2,y_2)\)) are the endpoints of the segment.
2. Find the length of (AC)
Coordinates of point (A): \((-1,1)\)
Coordinates of point (C): \((-4,4)\)
Substitute these points into the distance formula,
\(D=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
\(D=\sqrt{((-1)-(-4))^2+((1)-(4))^2}\)
Simplify,
\(D=\sqrt{((-1)-(-4))^2+((1)-(4))^2}\)
\(D=\sqrt{(-1+4)^2+(1-4)^2}\)
\(D=\sqrt{(3)^2+(-3)^2}\)
\(D=\sqrt{9+9}\)
\(D=\sqrt{18}\)
3. Find the length of (AB)
Coordinates of point (A): \((-1,1)\)
Coordinates of point (B): \((0,-4)\)
Substitute these points into the distance formula,
\(D=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
\(D=\sqrt{((-1)-(0))^2+((1)-(-4))^2}\)
Simplify,
\(D=\sqrt{((-1)-(0))^2+((1)-(-4))^2}\)
\(D=\sqrt{(-1-0)^2+(1+4)^2}\)
\(D=\sqrt{(-1)^2+(5)^2}\)
\(D=\sqrt{1+25}\)
\(D=\sqrt{26}\)
4. Find the correct statement
(AB) is longer than (AC)
This statement is true for the following reason:
\(\sqrt{18}>\sqrt{26}\)
For the following exercises, write the equation of an ellipse in standard form, and identify the endpoints of the major and minor axes as well as the foci. 9x² - 54x +9y² - 54y + 81 = 0
Answers
The equation of the ellipse in standard form is ((x - 3)²)/9 + ((y - 3)²)/9 = 1.
The center is (3, 3), the endpoints of the major axis are (0, 3) and (6, 3), the endpoints of the minor axis are (3, 0) and (3, 6), and the foci coincide with the center at (3, 3).
To write the equation of the ellipse in standard form and identify the endpoints of the major and minor axes as well as the foci, we need to manipulate the given equation into the standard form of an ellipse.
The general equation for an ellipse in standard form is:
((x - h)²/a²) + ((y - k)²/b²) = 1
where (h, k) represents the center of the ellipse, "a" represents the semi-major axis, and "b" represents the semi-minor axis.
Given equation: 9x² - 54x + 9y² - 54y + 81 = 0
First, let's group the x-terms and y-terms together:
(9x² - 54x) + (9y² - 54y) + 81 = 0
Next, we factor out the coefficients of x² and y²:
9(x² - 6x) + 9(y² - 6y) + 81 = 0
Now, complete the square for both x and y terms separately. To complete the square for x, we take half of the coefficient of x (-6) and square it (-6/2)² = 9. Similarly, for y, we have (6/2)² = 9:
9(x² - 6x + 9) + 9(y² - 6y + 9) + 81 = 0
Simplify the equation:
9(x - 3)² + 9(y - 3)² + 81 = 0
Divide the equation by 81 to get the equation in standard form:
((x - 3)²)/9 + ((y - 3)²)/9 = -1
To make the right-hand side equal to 1, we need to divide the equation by -1:
((x - 3)²)/(-9) + ((y - 3)²)/(-9) = 1
Now, the equation is in standard form, and we can identify the values:
Center: (3, 3)
Semi-major axis: sqrt(9) = 3
Semi-minor axis: sqrt(9) = 3
Endpoints of major axis: (3 - 3, 3) and (3 + 3, 3) --> (0, 3) and (6, 3)
Endpoints of minor axis: (3, 3 - 3) and (3, 3 + 3) --> (3, 0) and (3, 6)
To find the foci, we can use the formula:
c = sqrt(a² - b²)
In this case, a = 3 and b = 3, so:
c = sqrt(3² - 3²) = sqrt(0) = 0
Since c = 0, the foci coincide with the center of the ellipse at (3, 3).
In summary, the equation of the ellipse in standard form is:
((x - 3)²)/9 + ((y - 3)²)/9 = 1
The center is (3, 3), the endpoints of the major axis are (0, 3) and (6, 3), the endpoints of the minor axis are (3, 0) and (3, 6), and the foci coincide with the center at (3, 3).
Learn more about ellipse here
https://brainly.com/question/30727620
#SPJ11
How many inches in 4.11 feet?
Answers
Answer: The answer is 49.32
Step-by-step explanation:

how many ounces of pure chocolate must be added to 100 oz of chocolate topping that is 50% chocolate to make a topping that is 75% chocolate?
Answers
100 ounces of pure chocolate is required to be included in 100 ounces of chocolate topping that is 50% chocolate to prepare a topping that is 75% chocolate.
Using the given equation to determine the number of ounces needed:
0.50 . 100 + 1.00x = 0.75(100 + x)
50 + 1.00x = 75 + 0.75x
50 - 75 = 0.75x - 1.00x
- 25= -0.25x
or
0.25x= 25
x= 25/0.25
x= (25. 100)/25
x = 100.
Thus, 100 ounces of pure chocolate is required to be included in 100 ounces of chocolate topping that is 50% chocolate to prepare a topping that is 75% chocolate.
You can learn more about number of ounces at
https://brainly.com/question/17209436
#SPJ4
Three more than the product of a number and 5 is equal to 6.
Answers
5x + 3 = 6
5x = 3
x = 3/5 or .60
5x = 3
x = 3/5
What are the 4 binomial conditions?
Answers
The four conditions of binomial distribution are:
There will always be n observations.Every observation stands alone.Each observation corresponds to one of two results ("success" or "failure").Each outcome has the same chance of "success," or p.What is binomial distribution?
The discrete probability distribution known as the binomial distribution only allows for Success or Failure as the possible outcomes of an experiment.
The four conditions are described below.
1. Fixed Trials: The number of trials in the procedure under investigation must be fixed and cannot be changed during the analysis. Each experiment must be carried out consistently throughout the analysis, even though each trial's results could vary.
2. Independent trials: The results of one trial shouldn't influence those of the others.
3. Fixed probability of success: For the trials we are looking at, the likelihood of success must remain constant.
4. Two mutually exclusive outcomes: Success or failure are the only two outcomes that can occur. Even though the word "success" is typically used to refer to anything positive, it can also signify that the trial's results concur with your definition of success, whether that result is favorable or bad.
Hence, these are the four conditions.
Learn more about binomial distribution from the given link:
https://brainly.com/question/15246027
#SPJ4
how many solutions can a system of 2 linear equations in 2 variables have? give all options. explain visually, symbolically, and verbally
Answers
Symbolically, they correspond to the relationships between the coefficients of the equations.
A system of 2 linear equations in 2 variables can have one of the following three possible solutions:
One unique solution: In this case, the two lines intersect at exactly one point, and this point is the solution to the system. Visually, the two lines are not parallel, but they are not the same either. Symbolically, the system is represented as:
a1x + b1y = c1
a2x + b2y = c2
where a1, b1, c1, a2, b2, and c2 are constants, and x and y are variables.
Infinitely many solutions: In this case, the two lines coincide and are on top of each other, meaning they have the same slope and the same y-intercept. Visually, the two lines are identical. Symbolically, the system is represented as:
a1x + b1y = c1
ka1x + kb1y = kc1
where a1, b1, and c1 are constants, x and y are variables, and k is any non-zero constant.
No solution: In this case, the two lines are parallel and never intersect. Visually, the two lines are distinct and never meet. Symbolically, the system is represented as:
a1x + b1y = c1
a2x + b2y = c2
where a1, b1, c1, a2, b2, and c2 are constants, and x and y are variables, and the slope of one line is not equal to the slope of the other.
Geometrically, these cases correspond to the three possible positions of two lines in the coordinate plane. Symbolically, they correspond to the relationships between the coefficients of the equations.
Learn more about equations here:
https://brainly.com/question/29538993
#SPJ11
what is the answer -x/8>-6
Answers
Answer:
multiply both sides by -1
X/8 > 6
multiplying 8 on both sides
X > 48 is the answer!
The total number of forks dropped by customers per day at a busy restaurant is multimodal with a mean of 24.5 and a standard deviation of 3.3. If a random sample of 80 days is selected, what is the probability that the mean number of forks dropped during those days will be more than 25
Answers
The probability that the mean number of forks dropped during the 80 days will be more than 25 is approximately 0.0885 or 8.85%.
We'll be using the Central Limit Theorem, which states that the distribution of sample means approaches a normal distribution as the sample size increases, regardless of the population's distribution.
In this case, we have a multimodal distribution with a mean (μ) of 24.5 and a standard deviation (σ) of 3.3.
We want to find the probability that the mean number of forks dropped in a sample of 80 days (n) will be more than 25.
Calculate the standard error of the mean (SE).
SE = σ / √n = 3.3 / √80 ≈ 0.369.
Calculate the z-score for the desired mean (25) using the formula:
z = (x - μ) / SE = (25 - 24.5) / 0.369 ≈ 1.35
Find the probability that corresponds to the z-score.
Using a z-table or an online calculator, we find that the area to the left of z = 1.35 is approximately 0.9115.
Since we want the probability of the mean being more than 25, we'll take the complement:
Probability (mean > 25) = 1 - 0.9115 ≈ 0.0885.
For similar question on probability.
https://brainly.com/question/30390037
#SPJ11
solve the system of equations
−4x+3y=−2
y=x−1
x =
y =
Answers
Step-by-step explanation:
So if you know y = x-1 you can plug that in where y is in the first equation
-4x + 3(x-1) = -2
Distribute the 3:
-4x +3x -3 = -2
Combine like terms:
-x -3 = -2
Add 3 to both sides:
-x = 1
Multiply by -1:
X = -1
Then you plug that into the other equation to get y=(-1)-1
Which is -2
The probability that a carton of eggs taken from the cooler at a store will have at least one cracked egg is 2.5%.
What are the odds that a carton of eggs will have at least one cracked egg?
Answers
Answer: The odds of an event happening are the ratio of the number of ways the event can happen to the number of ways it cannot happen.
Odds = (probability of success) / (probability of failure)
In this case, the event is that a carton of eggs will have at least one cracked egg.
The probability of success (the event happening) is 2.5%
The probability of failure (the event not happening) is 100% - 2.5% = 97.5%
so we can find the odds by dividing the probability of success by the probability of failure.
Odds = 2.5 / 97.5 = 0.025/0.975 = 1/39
So the odds that a carton of eggs will have at least one cracked egg is 1:39, meaning that for every 39 cartons that are free of cracked eggs, one carton will have at least one cracked egg.
It means that it's 1 in 39 chance that a carton of eggs will have at least one cracked egg
Step-by-step explanation:
PLS HELP !!! i’ll mark you for 1st right answer

Answers
Answer:
130 grams
Step-by-step explanation:
Add the second amount which equals 150, and then subtract by 20.