IM MARKING BRAINLIEST
Robert wants to make a scarf and matching hat for his sister. The patterns call for 4/6 yard of fabric for the scarf and 1/6 yard of fabric for the hat. How much fabric should robert buy?
Answers
5/6 yards
Step-by-step explanation:
\( \frac{1}{6} + \frac{4}{6} = \frac{5}{6} \)
Answer:
5/6 yards of fabric
Step-by-step explanation:
4/6 yards + 1/6 yards = 5/6 yards
Related Questions
write a growth/decay function to model the situation A car was purchased in 2000 for $22,500. It has depreciated at a rate of 5% per year. What was the value of the car in 2011
Answers
If the price of the car is $22,500 in 2000. Then the value of the car in 2011 with a decay of 5% every year is $12,798.
What is an exponent?Exponential notation is the form of mathematical shorthand which allows us to write complicated expressions more succinctly. An exponent is a number or letter is called the base. It indicates that the base is to raise to a certain power. X is the base and n is the power.
A car was purchased in 2000 for $22,500.
It has depreciated at a rate of 5% per year.
Then the price of the car in 2011 will be
The decay function is modeled as
\(\rm A = P(1-r)^t\)
The number of years has been passed is 11.
Then we have
\(\rm A = 22,500(1-0.05)^{11}\\\\A = \$ \ 12798\)
More about the exponent link is given below.
https://brainly.com/question/5497425
#SPJ1
Question is attached in the photo! Please answer with the local maximums, minimums, and extrema's! Thank you :)
local maximum(s) :
local minimums(s) :
local extrema :

Answers
The number local extremas to the function is given as follows:
3.
They are classified as follows:
Local maximum: 2.Local minimum: 1.What are the local extremas of a function?The local extremas of a function are the turning points of the graph of the function, and they are classified as follows:
Local maximum: the function changes from increasing to decreasing.Local minimum: the function changes from decreasing to increasing.The graph of the function has three turning points, and they are defined as follows:
Local maximum close to x = -4, as the function changes from increasing to decreasing.Local minimum close to x = 2, as the function changes from decreasing to increasing.Local maximum close to x = 1, as the function changes from increasing to decreasing.More can be learned about the local extremas of a function at https://brainly.com/question/24367710
#SPJ1
help me asap now pls

Answers
Answer:
b=60a
Step-by-step explanation:
solve the equation1/4p-2/5=3/4p+7P=
Answers
Solve:
\(\frac{1}{4}p-\frac{2}{5}=\frac{3}{4}p+7\)The LCM of the denominators is 4*5 = 20. So we multiply each term by 20 to eliminate denominators:
\(20\cdot\frac{1}{4}p-20\cdot\frac{2}{5}=20\cdot\frac{3}{4}p+20\cdot7\)Operating:
\(5p-8=15p+140\)Adding 8 and subtracting 15p:
\(5p-15p=8+140\)Simplifying:
\(-10p=148\)Dividing by -10:
\(p=\frac{148}{-10}\)Simplifying:
\(p=-\frac{74}{5}\)Easy brainliest for correct answer 100%

Answers
Answer:
I need 1 Brainliest before I can become Virtuoso
Step-by-step explanation:
i once met six siblings whose ages are 6 consecutive whole numbers. i asked each of them the question how old is your oldest sibling. which of the following could not be the sum of their answers.
A. 95
B. 125
C. 167
D. 205
E. 233
Answers
The consecutive ages of the siblings mean that each sibling is a year older than the younger sibling
All the options cannot be the sum of their ages
How to determine the sum of their ages
Represent the age of the smallest sibling with x.
So, the sum of their ages is:
\(Sum =x + x + 1 + x + 2 + x + 3 + x + 4 + x + 5\)
Evaluate the like terms
\(Sum =6x + 15\)
When the sum is 95 (from the options), we have:
\(6x + 15 = 95\)
Subtract 15 from both sides
\(6x = 80\)
Divide by 6
\(x = 13.3\) -- this is not an integer
When the sum is 95 (from the options), we have:
\(6x + 15 = 125\)
Subtract 15 from both sides
\(6x = 110\)
Divide by 6
\(x = 6.875\) -- this is not an integer
When the sum is 167 (from the options), we have:
\(6x + 15 = 167\)
Subtract 15 from both sides
\(6x = 152\)
Divide by 6
\(x = 25.33\) -- this is not an integer
When the sum is 205 (from the options), we have:
\(6x + 15 = 205\)
Subtract 15 from both sides
\(6x = 190\)
Divide by 6
\(x = 31.67\) -- this is not an integer
When the sum is 233 (from the options), we have:
\(6x + 15 = 233\)
Subtract 15 from both sides
\(6x = 218\)
Divide by 6
\(x = 36.33\) -- this is not an integer
Hence, all the options cannot be the sum of their ages
Read more about consecutive numbers at:
https://brainly.com/question/10853762
Answer:
Step-by-step explanation:

The length of one leg of a right triangle is 3 feet more than the other leg. If the hypotenuse is 15 feet, find the length of each leg.
Answers
Answer:
5 IS THE LEGHTH
Step-by-step explanation:
srry caps xd ummm it depends u can divide 3 into 15 which will be 5
Find all solutions to the equation.
7 sin2x - 14 sin x + 2 = -5
If yall can help me for Pre-Calc, that would be great.
-Thanks.
Answers
If the equation is
\(7\sin^2x-14\sin x+2=-5\)
then rewrite the equation as
\(7\sin^2x-14\sin x+7=0\)
Divide boths sides by 7:
\(\sin^2x-2\sin x+1=0\)
Since \(x^2-2x+1=(x-1)^2\), we can factorize this as
\((\sin x-1)^2=0\)
Now solve for x :
\(\sin x-1=0\)
\(\sin x=1\)
\(\implies\boxed{x=\dfrac\pi2+2n\pi}\)
where n is any integer.
If you meant sin(2x) instead, I'm not sure there's a simple way to get a solution...
The equation y=195(1.10)x can be used to represent growth in the number of Salad Supreme restaurants since 2005. Which is the most reasonable conclusion based on this information? A. Salad Supreme had 110 restaurants in 2005. B. Salad Supreme is increasing the number of restaurants by 10% each year. C. Salad Supreme is increasing the number of restaurants by 195 each year. D. Salad Supreme is increasing the number of restaurants by 110% each year.
Answers
The most reasonable conclusion based on the given information is: Salad Supreme is increasing the number of restaurants by 10% each year. Option B
How to determine the most reasonable conclusionBased on the given equation y = 195(1.10)^x, where x represents the number of years since 2005 and y represents the number of Salad Supreme restaurants, we can draw the following conclusions:
A. Salad Supreme had 110 restaurants in 2005: This conclusion is not supported by the given equation.
B. Salad Supreme is increasing the number of restaurants by 10% each year: This conclusion is supported by the equation.
C. Salad Supreme is increasing the number of restaurants by 195 each year: This conclusion is not supported by the equation.
D. Salad Supreme is increasing the number of restaurants by 110% each year: This conclusion is not supported by the equation. The growth factor of 1.10 (or 10%) indicates a 10% increase each year, not 110%.
Therefore, the most reasonable conclusion based on the given information is: Salad Supreme is increasing the number of restaurants by 10% each year.
Learn more about equation at https://brainly.com/question/29174899
#SPJ1
Complete the expression so that it is equivalent to 2 (x+6)

Answers
The complete expression is (2 × x) + (2 × 6). This is an equivalent expression to the given expression.
What is an expression?
A number, a variable, or a combination of numbers, variables, and operation symbols make up an expression. Two expressions joined by an equal sign form an equation.
Given expression is
2 (x+6)
Distributive property: The same outcome is obtained by multiplying the sum of two or more addends by a number as it is by multiplying each addend by the number separately and combining the resulting products.
The mathematical representation is a(b+c) = ab + ac.
Apply the Distributive property in the given expression:
(2×x) + (2×6)
To learn more about expression, click on the below link:
https://brainly.com/question/26523496
#SPJ1
At a costumer service call center for a large company, the number of calls received per hour is normally distributed with a mean of 150 calls and a standard deviation of 5 calls. What is the probability that during a given hour of the day there will be between 146 calls and 163 calls, to the nearest thousandth
Answers
We have been given that at a customer service call center for a large company, the number of calls received per hour is normally distributed with a mean of 150 calls and a standard deviation of 5 calls. We are asked to find the probability that during a given hour of the day there will be between 146 calls and 163 calls.
First of all, we will use z-score formula to find z-score corresponding to 146 and 163.
\(z=\frac{x-\mu}{\sigma}\)
\(z=\frac{146-150}{5}\)
\(z=\frac{-4}{5}\)
\(z=-0.8\)
\(z=\frac{163-150}{5}\)
\(z=\frac{13}{5}\)
\(z=2.6\)
Our next step is to find percentage of data scores falls between both z-scores.
\(P(-0.8<z<2.6)=P(z<2.6)-P(z<-0.8)\)
Using normal distribution table, we will get:
\(P(-0.8<z<2.6)=0.99534-0.21186\)
\(P(-0.8<z<2.6)=0.78348\)
Let us convert our answer into percentage.
\(0.78348\times 100\%=78.348\%\)
Upon rounding our answer to nearest thousandths, we will get:
\(78.348\%\approx 78.35\%\)
Therefore, the probability that during a given hour of the day there will be between 146 calls and 163 calls is approximately \(78.35\%\).
Answer:
0.624
Step-by-step explanation:
DeltaMath
find the general solution for: cos(x+30)=-1
Answers
The required, general solution for x that satisfies the equation cos(x + 30) = -1 is x = (2n + 1)π - 30.
To find the general solution for the equation cos(x + 30) = -1, we can start by considering the general form of the cosine function. The cosine function has a period of 2π, meaning it repeats every 2π radians.
In this equation, we have cos(x + 30) = -1. Since the cosine function has a maximum value of 1 and a minimum value of -1, the only way for cos(x + 30) to equal -1 is if x + 30 is an odd multiple of π. We can write this as:
x + 30 = (2n + 1)π
Here, n is an integer representing the number of periods of the cosine function. To find the general solution, we can solve for x by subtracting 30 from both sides:
x = (2n + 1)π - 30
This equation gives us the general solution for x that satisfies the equation cos(x + 30) = -1. We can see that for each integer value of n, we have a different solution.
Learn more about trigonometric functions here:
https://brainly.com/question/25618616
#SPJ1
The profit a company makes from producing x tabletops is modeled by the equation P(x) = 480% - 2x For what
number of tabletops does the company make a profit of $0?
Answers
Answer: 240 tabletops
Select the correct answer from each drop-down menu. Given: , , , and are the vertices of quadrilateral. Prove: is a square. Using the distance formula, i found that.
Answers
WXYZ must be a square, all the sides must have a length of 5.
The vertices of the quadrilateral are:
W(-1, 1), X(3, 4), Y(6,0), and Z(2, -3)
Distance can be calculated using the formula derived from Pythagoras theorem. In coordinate geometry, the distance formula is:
\(\sqrt{[(x_2 -x_1)^2 + (y_2 - y_1)^2]}\)
We have to prove that WXYZ is a square.
Using the distance formula :
\(WX = \sqrt{(-1-3)^2+(1-4)^2}\\ \\WX = \sqrt{16 + 9}\\ \\WX = \sqrt{25}\\ \\WX = 5\)
Since WXYZ must be a square, all the sides must have a length of 5.
Learn more about Square at:
https://brainly.com/question/28776767
#SPJ4
The given question is incomplete, complete question is:
Select the correct answer from each drop-down menu.
Given: W(-1, 1), X(3, 4), Y(6,0), and Z(2, -3) are the vertices of quadrilateral WXYZ.
Prove: WXYZ is a square.
Using the distance formula, I found that
…
A. all four sides have a length of 5
B. all four sides have different lengths
C. only 2 sides have the same length
D. all four sides have a length of 10
What error did Wanda make?
None. Wanda’s work is correct.
Wanda converted the percent to a decimal incorrectly. It should be 0.073.
Wanda converted the percent to a decimal incorrectly. It should be 0.73.
Wanda has the wrong label. It should be hours, not dollars.
Answers
Answer:
C
Step-by-step explanation:
the guy above sucks
None. Wanda’s work is correct.
Wanda converted the percent to a decimal incorrectly. It should be 0.073.
Wanda converted the percent to a decimal incorrectly. It should be 0.73.
Wanda has the wrong label. It should be hours, not dollars.
Step-by-step explanation:
Find the domain. f(x) = −4x(x − 1)(x − 3)
Answers
Answer:
Step-by-step explanation:
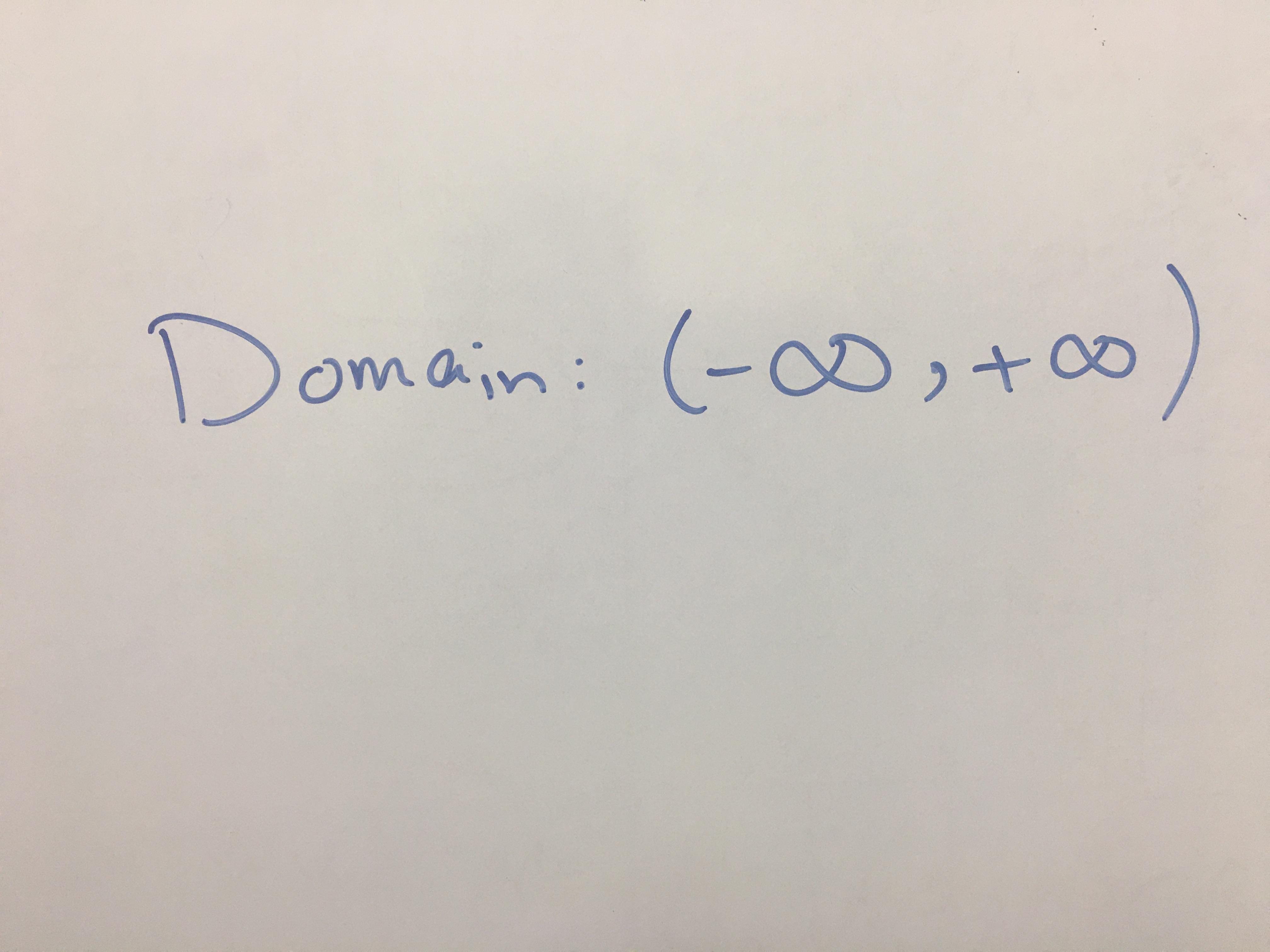
Hurry please !!!
The graph of g(x) is a translation of y = V.
Which equation represents g(x)?
ly
g(x) = - 4
5
4
g(x) = 3/X+4
3
27
900)
O g(x) = 5x +1.5
1
g(x) = -1.5
-10 -3 -6 -21
2
6
810
X

Answers
Answer:
The translation corresponds to : \(g(x)=\sqrt[3]{x-4}\)
which is the first option in your list of possible answers
Step-by-step explanation:
Notice that this new function's graph responds to a horizontal translation to the right of the original graph of \(f(x)=\sqrt[3]{x}\). Notice that the crossing of the x-axis, which for f(x) is at the origin (0, 0), has now moved to the point (4,0), which means a translation to the right in exactly 4 units.
Recall that horizontal translations are performed by subtracting from the independent variable (x) , the number of units you move (in this case 4)
Therefore the new function should look like:
\(g(x)=\sqrt[3]{x-4}\)
The answer is A.
did the test
The price of an item has been reduced by 95%. The original price was $65
Answers
The new price of the item is equivalent to $3.25.
What is percentage?In mathematics, a percentage is a number or ratio that represents a fraction of 100. A percentage is a dimensionless number i.e. it has no unit of measurement.Given is that the price of an item has been reduced by 95%. The original price was $65
Assume the new price to be $[x]. So, we can write -
x = 65 - {95% of 65}
x = 65 - {(95/100) x 65}
x = 65 - {6175/100}
x = 65 - 61.75
x = 3.25
Therefore, the new price of the item is equivalent to $3.25.
To solve more questions on percentage, visit the link below -
https://brainly.com/question/29011982
#SPJ1
solve the following inequality for z. write your answer in simplest form. -9-(2z-7)>-2z-6-5z
Answers
Answer:
z > -4/5
Step-by-step explanation:
-9 - (2z - 7) > -2z - 6 - 5z
Get rid of the parenthesis
*There is the number one in front of the parenthesis.-9 - 2z + 7 > -2z - 6 - 5z
Combine like terms:
-2 - 2z > -7z - 6
+2 > +2
Add 2 to both sides.
-2z > -7z - 4
+7z > +7z
Add 7 to both sides.
5z > -4
Divide both sides by 5 to get z.
z > -4/5
The sign stays the same unless you divide by a negative number---------------------------------
More on inequalities: https://brainly.com/question/21857626
Hope this helps! :)
Three support beams for a bridge form a pair of complementary angles. Find the measure of each angle.

Answers
Answer:
Please answer my questions you'll get points
Answer:
The smaller angle is 39, the other is 51
Step-by-step explanation:
the expression negative five eighths times k minus 8 plus the expression 2 plus one third times k
7 over 24 times k plus negative 6
negative 4 over 11 times k plus negative 10
negative 7 over 24 times k plus negative 6
negative 23 over 24 times k plus negative 10
Answers
After solving the expression, the value is negative 7 over 24 times k plus negative 6. Therefore, option C is correct.
What is an expression?Mathematical actions are called expressions if they have at least two terms that are related by an operator and include either numbers, variables, or both. Adding, subtraction, multiplying, and division are all reflection coefficient operations.
A mathematical operation such as reduction, addition, multiplication, or division is used to integrate terms into an expression.
As per the given statement for the expression,
-(5/8)k - 8 + 2 + (1/3)k
Take LCM as 24 and solve the equation,
(-7k - 144) / 24
= (-7/24)k - 6
Therefore, this concludes that option C is correct.
To know more about Expression:
https://brainly.com/question/14083225
#SPJ1
Which of the following expressions are equivalent to -r/-s

Answers
Answer:
A \(\frac{r}{8}\)
Step-by-step explanation:
-/- = +
-r/-8 = r/8
if x=-3 is the only x-incercept of the graph of a quadratic equation which statement best describes the discrinant of the equation
Answers
Answer:
The discriminant must be zero.
Step-by-step explanation:
Quadratic Equation
The standard representation of a quadratic function is:
\(f(x)=ax^2+bx+c\)
where a,b, and c are constants.
The zeros, roots, or x-intercepts can be found by solving the equation:
\(ax^2+bx+c=0\)
We can use the quadratic formula to find the roots:
\(\displaystyle x=\frac{-b\pm \sqrt{b^2-4ac}}{2a}\)
Note this formula gives us two different roots if the square root is non-zero.
The only way there can be only one x-intercept is because the square root is zero.
If the square root is zero, then
\(b^2-4ac =0\)
The expression is called the discriminant.
Thus, if x=-3 is the only x-intercept of the graph of a quadratic function, then the discriminant must be zero.
Find the volume of the cylinder.
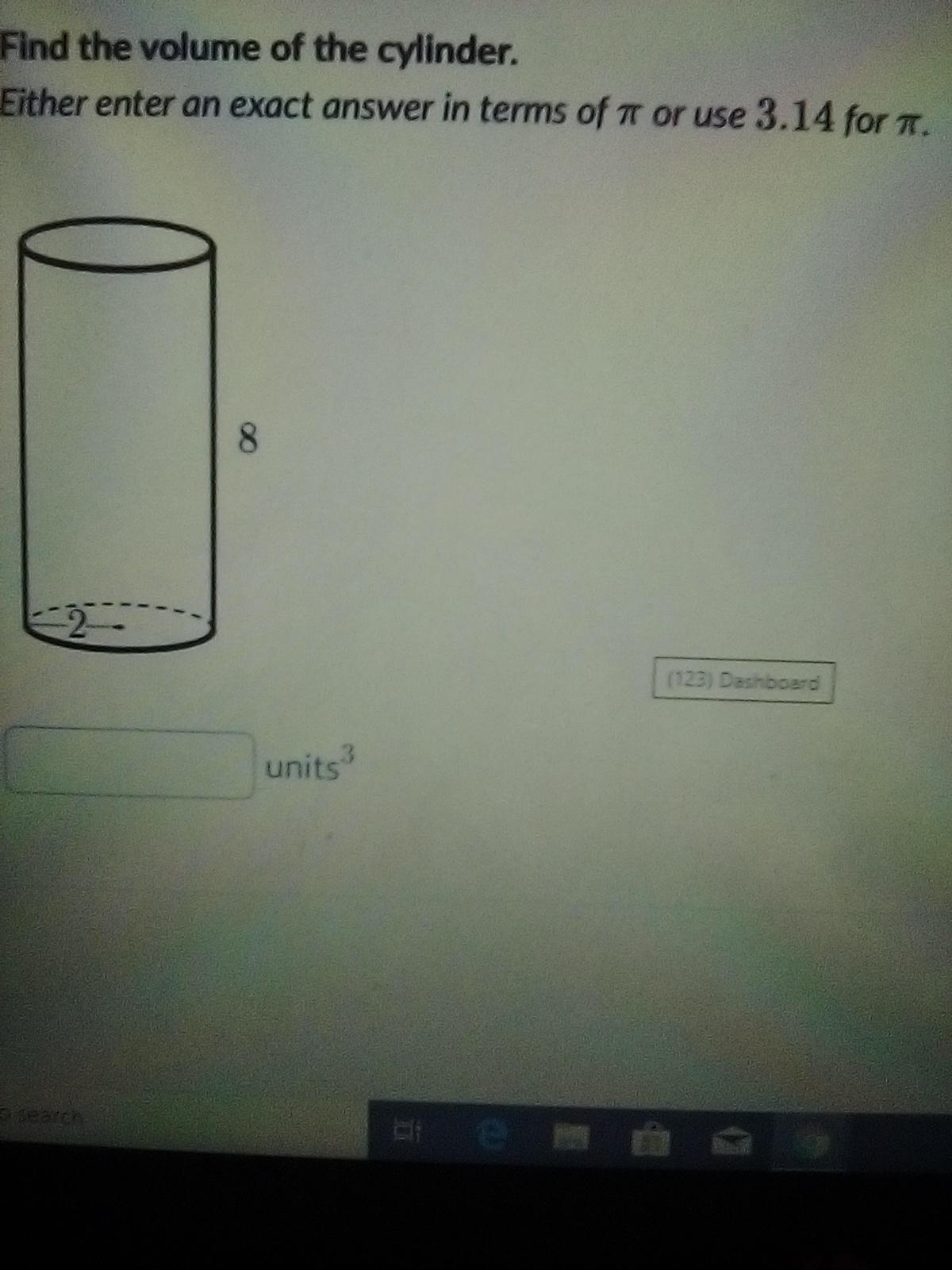
Answers
The approximate volume only applies when pi = 3.14
Use either answer, but not both of course.
===============================================
Work Shown:
V = volume of cylinder
V = pi*r^2*h
V = pi*2^2*8
V = pi*32
V = 32pi .... exact volume in terms of pi
V = 32*3.14
V = 100.48 .... approximate volume when we use pi = 3.14
The probability that a house in an urban area will develop a leak is 2%. If 21 houses are randomly selected, what is the probability that none of the houses will develop a leak?
Answers
Answer:
The probability that none of the houses will develop a leak is 0.6543.
Step-by-step explanation:
Let X denote the number of houses in an urban area that develop a leak.
The probability that a house in an urban area will develop a leak is, p = 0.02.
A random sample of n = 21 houses are selected.
Every house is independent of developing a leak.
Thus, the random variable X follows a binomial distribution with parameters n = 21 and p = 0.02.
Compute the probability that none of the houses will develop a leak as follows:
\(P(X=0)={21\choose 0}(0.02)^{0}(1-0.02)^{21-0}\\\\=1\times1\times 0.654256\\\\\approx 0.6543\)
Thus, the probability that none of the houses will develop a leak is 0.6543.
Find the area of the parallelogram 9.9in 5.5in

Answers
Answer:
54.45 in²
Step-by-step explanation:Let the base and height be 9.9 inches and 5.5 inches respectively.
Formula;
Area of parallelogram = Base × Height
Substitute the base and the height in the formula:
⇒ Area of parallelogram = Base × Height⇒ Area of parallelogram = 9.9 × 5.5Evaluate the area of the parallelogram:
⇒ Area of parallelogram = 9.9 in × 5.5 in⇒ Area of parallelogram = 54.45 in²
Answer:
54.45 in²
Step-by-step explanation:
Formula
Area of a parallelogram = base × height
If you turn the parallelogram so that the sides that measure 9.9in are the base, you will see that 5.5in is the height (refer to the attached diagram). Therefore, we can use the formula with these values to calculate the area.
Given:
base = 9.9 inheight - 5.5 in⇒ area = 9.9 × 5.5 = 54.45 in²

Find the value of x in the triangle below. Please help

Answers
Answer:
The exterior angle of 77 degrees is equal to the sum of angle M and L, so we can create an equation where (10x + 1) + (6x - 4) = 77 and solve it. First we can combine like terms and add numbers on the left side of the equation: 16x - 3 = 77. Next, we can add 3 to both sides of this equation: 16x = 80. Lastly, we can isolate the x variable by dividing both sides of this equation by 16: x = 5.
Step-by-step explanation:
Please support my answer.
As the CAPS document outlines, the Content Specification and Content Clarification for Patterns, Functions, and Algebra shows sequenced mathematics content topics and a content area spread. In the Intermediate Phase, select one topic and report on the topic sequence and content area spread. Your report should demonstrate mathematics concepts and procedures’ hierarchical and logical progression.
Answers
Answer:
Step-by-step explanation:
In the Intermediate Phase of mathematics education, one topic that demonstrates a hierarchical and logical progression in patterns, functions, and algebra is the concept of "Linear Equations."
The topic of Linear Equations in the Intermediate Phase builds upon the foundation laid in earlier grades and serves as a stepping stone towards more advanced algebraic concepts. Here is an overview of the topic sequence and content area spread for Linear Equations:
Introduction to Variables and Expressions:
Students are introduced to the concept of variables and expressions, learning to represent unknown quantities using letters or symbols. They understand the difference between constants and variables and learn to evaluate expressions.
Solving One-Step Equations:
Students learn how to solve simple one-step equations involving addition, subtraction, multiplication, and division. They develop the skills to isolate the variable and find its value.
Solving Two-Step Equations:
Building upon the previous knowledge, students progress to solving two-step equations. They learn to perform multiple operations to isolate the variable and find its value.
Writing and Graphing Linear Equations:
Students explore the relationship between variables and learn to write linear equations in slope-intercept form (y = mx + b). They understand the meaning of slope and y-intercept and how they relate to the graph of a line.
Systems of Linear Equations:
Students are introduced to the concept of systems of linear equations, where multiple equations are solved simultaneously. They learn various methods such as substitution, elimination, and graphing to find the solution to the system.
Word Problems and Applications:
Students apply their understanding of linear equations to solve real-life word problems and situations. They learn to translate verbal descriptions into algebraic equations and solve them to find the unknown quantities.
The content area spread for Linear Equations includes concepts such as variables, expressions, equations, operations, graphing, slope, y-intercept, systems, and real-world applications. The progression from simple one-step equations to more complex systems of equations reflects a logical sequence that builds upon prior knowledge and skills.
By following this hierarchical progression, students develop a solid foundation in algebraic thinking and problem-solving skills. They learn to apply mathematical concepts and procedures in a systematic and logical manner, paving the way for further exploration of patterns, functions, and advanced algebraic topics in later phases of mathematics education.
Anyone knows the answer??

Answers
Add the ratios: 3 + 7 + 5 = 15
Divide total songs by the sum:
60 / 15 = 4
Now multiply each ratio by that:
3 x 4 = 12
7 x 4 = 28
5 x 4 = 20
Answer: trap = 12, r&b = 28, reggaeton = 20
If 1 inch is 500 miles, then 4 inches is how many miles?
Answers
Answer:
2,000 miles
Step-by-step explanation:
We are given:
\(1 inch=500 miles\)
This can be rewritten as:
\(\frac{500 miles}{1inch}\)
This is representative of a unit rate, as we have a \(1\) in the denominator. To calculate the number of miles in \(4\) inches, simply multiply the unit rate by \(4\):
\(\frac{500 miles}{1inch}*4=\)
\(\frac{2000 miles}{4 inches}\)
This can rewritten as:
\(4inches=2000miles\)
Therefore, there are 2,000 miles in 4 inches.
-
We can check our solution by simplifying the fraction \(\frac{2000 miles}{4 inches}\) by dividing both \(2000\) and \(4\) by \(4\) to see if we achieve the unit rate:
\(\frac{500 miles}{1 inch}\)
Since this is in fact the unit rate, our solution is correct!